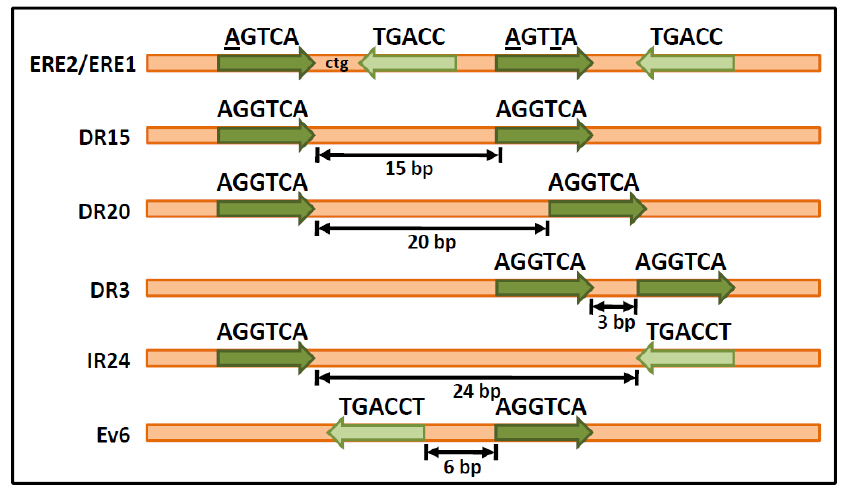
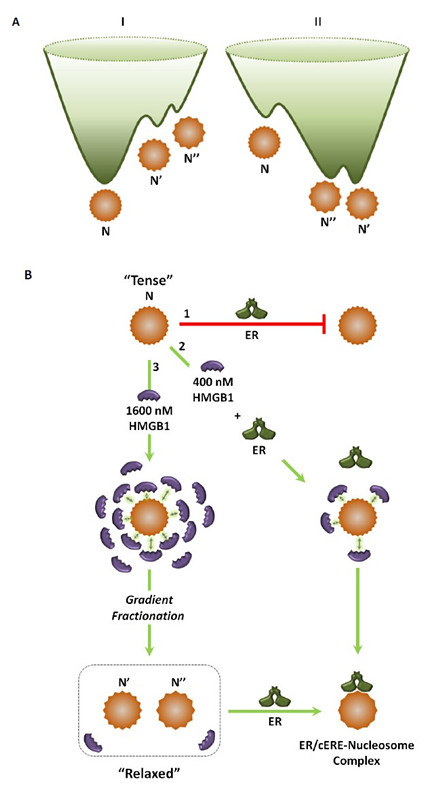
Estrogen receptor alpha (ERα) plays a major role in the expression of estrogen-responsive genes. Although its conventional binding characteristics have been considered coincident with & exclusively in the class of steroid hormone receptors,increasing evidence challenges this paradigm. ERα was shown to bind to consensus estrogen response element half-sites (cHERE) in DNA in the presence of the ubiquitous,abundant & conserved architectural protein,high mobility group protein 1 (HMGB1). It also binds to direct repeats with various spacers,in addition to everted repeats. These in vitro binding sites have been shown to be active in vivo,with both the binding affinity and transcriptional activity increased in the presence of HMGB1. Surprisingly,ERα does not bind to the optimally oriented cERE at the dyad in rotationally phased and translationally positioned nucleosomes. However,the presence of HMGB1 restructures the nucleosome to facilitate increased ERα accessibility,resulting in sequence-specific estrogen receptor binding. The finding that HMGB1 interacts with unbound ERα provides a unique avenue for enhanced ERα activity and possibly an increase in the extent of targeting at estrogen-responsive genes. The findings are consistent with ERα 1) targeting a much wider selection of genomic response elements (half-sites and inverted,direct and everted repeats) and 2) exhibiting characteristics of both steroid and non steroid nuclear receptors. Growing evidence already shows a competition occurs at the DNA level between ERα and the non steroid nuclear hormone receptor,thyroid receptor (TR). Collectively,these reports suggest a less restrictive cataloging for estrogen receptor and a broader paradigm for understanding its role in the regulation of estrogen-responsive genes and influence on non steroid hormone receptor activities.
1.
Introduction
The following inequality is named the Ostrowski type inequality [30].
Theorem 1. [10] Let $ f:\left[ a, b\right] \rightarrow \mathbb{R} $ be a differentiable mapping on $ \left(a, b\right) $ and $ f^{\prime }\in L\left[ a, b\right] $ (i.e. $ f^{\prime } $ be an integrable function on $ \left[ a, b \right] $). If $ \left\vert f^{\prime }\left(x\right) \right\vert < M $ on $
\left[ a, b\right] $, then the following inequality holds:
for all $ x\in \left[ a, b\right] $.
To prove the Ostrowski type inequality in $ \left(1.1\right) $, the following identity is used, (see [26]):
where $ f\left(x\right) $ is a continuous function on $ \left[ a, b\right] $ with a continuous first derivative in $ \left(a, b\right) $. The identity $
\left(1.2\right) $ is known as Montgomery identity.
By changing variable, the Montgomery identity $ \left(1.2\right) $ can be expressed as:
where
A number of different identities of the Montgomery and many inequalities of Ostrowski type were obtained by using these identities. Through the framework of Montgomery's identity, Cerone and Dragomir [9] developed a systematic study which produced some novel inequalities. By introducing some parameters, Budak and Sarıkaya [8] as well as Özdemir et al. [31] established the generalized Montgomery-type identities for differentiable mappings and certain generalized Ostrowski-type inequalities, respectively. Aljinović in [1], presented another simpler generalization of the Montgomery identity for fractional integrals by utilizing the weighted Montgomery identity. Furthermore, the generalized Montgomery identity involving the Ostrowski type inequalities in question with applications to local fractional integrals can be found in [32]. For more related results considering the different Montgomery identities, [2,4,7,11,12,13,15,16,21,22,23,24,25,33,34,36] and the references therein can be seen.
In the related literature of Montgomery type identity, it was not considered via quantum integral operators. The aim of this work is to set up a quantum Montgomery identity with respect to quantum integral operators. With the help of this new version of Montgomery identity, some new quantum integral inequalities such as Ostrowski type, midpoint type, etc are established. The absolute values of the derivatives of considered mappings are quantum differentiable convex mappings.
Throughout this paper, let $ 0 < q < 1 $ be a constant. It is known that quantum calculus constructs in a quantum geometric set. That is, if $ qx\in A $ for all $ x\in A, $ then the set $ A $ is called quantum geometric.
Suppose that $ f\left(t\right) $ is an arbitrary function defined on the interval $ \left[ 0, b\right] $. Clearly, for $ b > 0 $, the interval $ [0, b] $ is a quantum geometric set. The quantum derivative of $ f\left(t\right) $ is defined with the following expression:
Note that
if $ f\left(t\right) $ is differentiable.
The quantum integral of $ f\left(t\right) $ is defined as:
and
where $ 0 < c < b $ (see [3,14]).
Note that if the series in right-hand side of $ \left(1.6\right) $ is convergence, then $ \int_{0}^{b}f\left(t\right) $ $ d_{q}t $ is exist, i.e., $ f\left(t\right) $ is quantum integrable on $ \left[ 0, b\right] $. Also, provided that if $ \int_{0}^{b}f\left(t\right) $ $ dt $ converges, then one has
These definitions are not sufficient in establishing integral inequalities for a function defined on an arbitrary closed interval $ \left[ a, b\right] \subset \mathbb{R}
$. Due to this fact, Tariboon and Ntouyas in [37,38] improved these definitions as follows:
Definition 1. [37,38]. Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be a continuous function. The $ q $-derivative of $ f $ at $ t\in \left[ a, b \right] $ is characterized by the expression:
The function $ f $ is said to be $ q $-differentiable on $ \left[ a, b\right] $, if $
aDqf(t)
$ exists for all $ t\in \left[ a, b\right] $.
Clearly, if $ a = 0 $ in $ \left(1.9\right) $, then$
0Dqf(t)=Dqf(t),
$ where $ D_{q}f\left(t\right) $ is familiar quantum derivatives given in $
\left(1.4\right) $.
Definition 2. [37,38]. Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be a continuous function. Then the quantum definite integral on $ \left[ a, b \right] $ is defined as
and
where $ a < c < b $.
Clearly, if $ a = 0 $ in $ \left(1.10\right) $, then
where $ \int_{0}^{b}f\left(t\right) dqt
$ is familiar definite quantum integrals on $ \left[ 0, b\right] $ given in $
\left(1.6\right) $.
Definition 1 and Definition 2 have actually developed previous definitions and have been widely used for quantum integral inequalities. There is a lot of remarkable papers about quantum integral inequalities based on these definitions, including Kunt et al. [19] in the study of the quantum Hermite–Hadamard inequalities for mappings of two variables considering convexity and quasi-convexity on the co-ordinates, Noor et al. [27,28,29] in quantum Ostrowski-type inequalities for quantum differentiable convex mappings, quantum estimates for Hermite–Hadamard inequalities via convexity and quasi-convexity, quantum analogues of Iyengar type inequalities for some classes of preinvex mappings, as well as Tunç et al. [39] in the Simpson-type inequalities for convex mappings via quantum integral operators. For more results related to the quantum integral operators, the interested reader is directed to [5,18,20,35,41] and the references cited therein.
In [6], Alp et al. proved the following inequality named quantum Hermite–Hadamard type inequality. Also in [40], Zhang et al. proved the same inequality with the fewer assumptions and shorter method.
Theorem 2. Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be a convex function with $ 0 < q < 1 $. Then we have
2.
Main results
Firstly, we discuss the assumptions of the continuity of the function $ f(t) $ in Definition 1 and Definition 2. Also, under these conditions, we want to discuss that similar cases with $ \left(1.5\right) $ and $
\left(1.8\right) $ can exist.
By considering the Definition 1, it is not necessary that the function $ f\left(t\right) $ must be continuous on $ \left[ a, b\right] $. Indeed, for all $ t\in \left[ a, b\right] $, $ qt+\left(1-q\right) a\in \left[ a, b\right] $ and $ f\left(t\right) -f\left(qt+\left(1-q\right) a\right) \in \mathbb{R}
$. It means that $ \frac{f\left(t\right) -f\left(qt+\left(1-q\right) a\right) }{\left(1-q\right) \left(t-a\right) }\in \mathbb{R}
$ exists for all $ t \in (a, b] $, so the Definition 1 should be as follows:
Definition 3. (Quantum derivative on $ \left[ a, b\right] $) Let $ f:\left[ a, b \right] \rightarrow \mathbb{R}
$ be an arbitrary function. Then $ f $ is called quantum differentiable on $
\left(a, b\right] $ with the following expression:
and $ f $ is called quantum differentiable on $ t = a $, if the following limit exists:
Lemma 1. (Similar case with $ \left(1.5\right) $) Let $ f:\left[ a, b \right] \rightarrow \mathbb{R}
$ be a differentiable function. Then we have
Proof. Since $ f $ is differentiable on $ \left[ a, b\right] $, clearly we have
for all $ t\in \left(a, b\right] $. Since $ 0 < q < 1 $, for all $ a < t\leq b $, we have $ \left(1-q\right) \left(a-t\right) < 0 $. Changing variable in $ \left(2.2\right) $ as $ \left(1-q\right) \left(a-t\right) = h $, then $ q\rightarrow 1^{-} $ we have $ h\rightarrow 0^{-} $ and $ qt+\left(1-q\right) a = t+h $. Using $ \left(2.3\right) $, we have
for all $ t\in \left(a, b\right] $. On the other hand, for $ t = a $ we have
which completes the proof.
In Definition 2, the condition of the continuity of the function $ f(t) $ on $ [a, b] $ is not required. For this purpose, it is enough to construct an example in which a function is discontinuous on $ [a, b] $, but quantum integrable on it.
Example 1. Let $ 0 < q < 1 $ be a constant, and the set $ A $ is defined as
Then the function $ f:\left[ -1, 2\right] \rightarrow \mathbb{R}
$ defined as
Clearly, it is not continuous on $ \left[ -1, 2\right] $. On the other hand
i.e., the function $ f\left(t\right) $ is quantum integrable on $ \left[ -1, 2 \right]. $
Hence the Definition 2 should be described in the following way.
Definition 4. (Quantum definite integral on $ \left[ a, b\right] $) Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be an arbitrary function. Then the quantum integral of $ f $ on $ \left[ a, b \right] $ is defined as
If the series in right-hand side of $ \left(2.4\right) $ is convergent, then $ \int_{a}^{b}f\left(t\right) adqt
$ is exist, i.e., $ f\left(t\right) $ is quantum integrable on $ \left[ a, b \right] $.
Lemma 2. (Similar case with $ \left(1.8\right) $) Let $ f:\left[ a, b \right] \rightarrow \mathbb{R}, $ be an arbitrary function. It provided that if $ \int_{a}^{b}f\left(t\right) $ converges, then we have
Proof. If $ \int_{a}^{b}f\left(t\right) $ $ dt $ converges, then $ \int_{0}^{1}f\left(tb+\left(1-t\right) a\right) $ $ dt $ also converges. Using $ \left(1.8 \right) $, we have that
Next we present an important quantum Montgomery identity, which is similar with the identity in $ \left(1.3\right) $.
Lemma 3. (Quantum Montgomery identity) Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}, $ be an arbitrary function with $
aDqf
$ is quantum integrable on $ \left[ a, b\right] $, then the following quantum identity holds:
where
Proof. By the Definition 3, $ f\left(t\right) $ is quantum differentiable on $ \left(a, b\right) $ and $
aDqf
$ is exist. Since $
aDqf
$ is quantum integrable on $ \left[ a, b\right] $, by the Definition 4, the quantum integral for the right-side of $ \left(2.6\right) $ is exist. The integral of the right-side of $ \left(2.6\right) $, with the help of $ \left(2.1\right) $ and $ \left(2.4\right) $, is equal to
which completes the proof.
Remark 1. If one takes limit $ q\rightarrow 1^{-} $ on the Quantum Montgomery identity in $ \left(2.6\right) $, one has the Montgomery identity in $ \left(1.3\right) $.
The following calculations of quantum definite integrals are used in next result:
and
Let us introduce some new quantum integral inequalities by the help of quantum power mean inequality and Lemma 3.
Theorem 3. Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be an arbitrary function with $
aDqf
$ is quantum integrable on $ \left[ a, b\right] $. If $
|aDqf|r,
$ $ r\geq 1 $ is a convex function, then the following quantum integral inequality holds:
for all $ x\in \left[ a, b\right] $, where
and
Proof. Using convexity of $ \left\vert _{a}D_{q}f\right\vert ^{r} $, we have that
By using Lemma 3, quantum power mean inequality and $ \left(2.12\right) $, we have that
Using $ \left(2.7\right) $–$ \left(2.10\right) $ in $ \left(2.13\right) $, we obtain the desired result in $ \left(2.11\right) $. This ends the proof.
Corollary 1. In Theorem 3, the following inequalities are held by the following assumptions:
1. If one takes $ r = 1 $, one has
2. If one takes $ r = 1 $ and $ \left\vert _{a}D_{q}f\left(x\right) \right\vert < M $ for all $ x\in \left[ a, b\right] $, then one has (a quantum Ostrowski type inequality, see [27,Theorem 3.1])
3. If one takes $ r = 1 $, $ \left\vert _{a}D_{q}f\left(x\right) \right\vert < M $ for all $ x\in \left[ a, b\right] $ and $ q\rightarrow 1^{-} $, then one has (Ostrowski inequality $ \left(1.1\right) $).
4. If one takes $ r = 1 $ and $ x = \frac{qa+b}{1+q} $, then one has (a new quantum midpoint type inequality)
5. If one takes $ r = 1 $, $ x = \frac{qa+b}{1+q} $ and $ q\rightarrow 1^{-} $, then one has (a midpoint type inequality, see [17,Theorem 4])
6. If one takes $ r = 1 $ and $ x = \frac{a+b}{2} $, then one has (a new quantum midpoint type inequality)
7. If one takes $ \left\vert _{a}D_{q}f\left(x\right) \right\vert < M $ for all $ x\in \left[ a, b\right] $, then one has (a quantum Ostrowski type inequality, see [27,Theorem 3.1])
8. If one takes $ x = \frac{qa+b}{1+q} $, then one has (a new quantum midpoint type inequality)
9. If one takes $ x = \frac{qa+b}{1+q} $ and $ q\rightarrow 1^{-} $, then one has (a midpoint type inequality, see [6,Corollary 17])
10. If one takes $ x = \frac{a+b}{2} $, then one has (a new quantum midpoint type inequality)
11. If one takes $ x = \frac{a+qb}{1+q} $, then one has (a new quantum midpoint type inequality)
Finally, we give the following calculated quantum definite integrals used as the next Theorem 4.
and
Theorem 4. Let $ f:\left[ a, b\right] \rightarrow \mathbb{R}
$ be an arbitrary function with $
aDqf
$ is quantum integrable on $ \left[ a, b\right] $. If $
|aDqf|r,
$ $ r > 1 $ and $ \frac{1}{r}+\frac{1}{p} = 1 $ is a convex function, then the following quantum integral inequality holds:
for all $ x\in \left[ a, b\right] $.
Proof. By using Lemma 3, quantum Hölder inequality and $ \left(2.8 \right) $, we have that
Using $ \left(2.14\right) $–$ \left(2.17\right) $ in $ \left(2.19\right) $, we obtain the desired result in $ \left(2.18\right) $. This ends the proof.
Remark 2. In Theorem 4, many different inequalities could be derived similarly to Corollary 1.
3.
Conclusions
In the terms of quantum Montgomery identity, some quantum integral inequalities of Ostrowski type are established. The establishment of the inequalities is based on the mappings whose first derivatives absolute values are quantum differentiable convex. Furthermore, the important relevant connection obtained in this work with those which were introduced in previously published papers is investigated. By considering the special value for $ x\in [a, b] $, some fixed value for $ r $, and as well as $ q\rightarrow 1^{-} $, many sub-results can be derived from the main results of this work. It is worthwhile to mention that certain quantum inequalities presented in this work are generalized forms of the very recent results given by Alp et al. (2018) and Noor et al. (2016). With the contribution of this work, the interested researchers will be motivated to explore this fascinating field of the quantum integral inequality based on the techniques and ideas developed in this article.
Acknowledgments
The first author would like to thank Ondokuz Mayıs University for being a visiting professor and providing excellent research facilities.
Conflict of interest
The authors declare that they have no competing interests.













 DownLoad:
DownLoad: