Associated outcomes of various iterations of the dedicated orthopaedic trauma room: a literature review

1.   Introduction

The designation of “urgent” to a surgical case implies that the case is neither emergent, nor elective. Emergency status is designated as part of the ASA Physical Status classification system, whereas elective status is defined as a scheduled surgery in which the patient is specifically brought to a medical facility for the procedure. For general surgery, the ACS NSQIP protocols state that operations be labeled “urgent” when they do not meet the emergency or elective criteria [1]. The ACS Trauma Center Guidelines require for there to be an available OR for emergencies, but there are no written guidelines regarding urgent surgery [2].

Scheduling urgent trauma cases has long been a challenge for health care institutions. Traditionally, these cases are scheduled for an OR slot in the middle of the night, by “bumping” elective cases to later in the day, by adding a case on after-hours, or by delaying the case for several days until an OR becomes available. With this traditional scheduling model, urgent cases across all surgical specialties are competing for OR availability. Orthopaedic trauma is often deemed “less urgent” than trauma that falls into other specialty categories such as general or neurosurgical cases, resulting in a large disruption in orthopaedic trauma surgical care. In countries with universal health care systems, patients may wait days for urgent surgery [3],[4].

The traditional scheduling model has deleterious ramifications affecting the patient, surgeon, and institution, for both the urgent and elective cases. Surgical delays can cause increased wait time for the patient, lengthening the time spent NPO and delaying time to rehabilitation [5]. In addition, patient outcomes may be affected by undergoing an after-hours procedure [6]. The unpredictable nature of the traditional scheduling model also affects surgeons by increasing the need to operate after-hours, including cases in the middle of the night after a full day of work and during “off duty” hours. Otherwise, elective cases can be bumped at the expense of significant disruption to the flow of all ORs, the surgeon's individual daily schedule, and the patient's time. As a result of this disruption, it can be difficult to recruit and maintain fellowship trained orthopaedic traumatologists and senior, experienced surgeons due to lifestyle detriments and burnout [7]. The traditional scheduling model also has effects at the institutional level. Increased cost can occur secondary to higher overtime and nighttime staffing. In addition, patients must occupy a bed either in the emergency department or on the floor while awaiting surgery, leading to usage of finite hospital resources, further contributing to the overall cost of care.

As a solution to these challenges facing traditional scheduling models, trauma centers around the country have instituted the use of a dedicated orthopaedic trauma room (DOTR). The DOTR model was first described in the United States by Bhattacharyya et al [7]. It was originally defined as an OR scheduling model that ensures a daytime OR is available for urgent orthopaedic trauma. This is typically achieved by blocking off a certain amount of time on weekdays, with no elective cases scheduled, to be under the full control by the day's attending traumatologist. Featherall et al. surveyed the top 20 US hospitals and found that 70% use an iteration of the DOTR [5]. Various models of the DOTR have been implemented around the world. In countries such as Australia and the United Kingdom, hospital systems have implemented the use of a dedicated orthopaedic trauma list (DOTL). The DOTL operates in the same fashion as a DOTR, where an OR remains un-booked and available for trauma cases to be scheduled by the attending specialist [8]. Bhattacharyya et al. described how the DOTR was initially implemented with three goals in mind: to (1) improve quality of care, (2) improve efficiency, and (3) recruit and retain fellowship trained orthopaedic surgeons [7].

While there are multiple studies analyzing the effects of DOTRs/DOTLs on various outcomes, there is not a centralized review of said studies. This paper will serve as a review of the various models of the DOTR/DOTL as well as the effect of the DOTR/DOTL on after-hours procedures, time to surgery, duration of surgery, length of stay, cost, and surgical complications.

2.   Materials and methods

2.1.   Search strategy

An extensive review of the literature was performed through PubMed and Embase. Key words used in the search included (emergency operating room AND orthopedic trauma) OR (emergency operating room AND orthopaedic trauma) OR (urgent operating room AND orthopedic trauma) OR (urgent operating room AND orthopaedic trauma) OR (dedicated orthopaedic trauma room) OR (dedicated orthopedic trauma list) OR (dedicated orthopaedic trauma list) OR (dedicated orthopaedic operating room) OR (dedicated orthopaedic operating room). Results were limited to studies published between 2005 and 2022, in English.

2.2.   Eligibility criteria

Studies were included in the review if they were published in English, involved clinical research, and reported the use of a dedicated operating room at a regular interval throughout the week, specifically for orthopaedic trauma.

2.3.   Statistical significance

For the purpose of this review, reported outcomes were considered statistically significant if P ≤ 0.05.

3.   Results

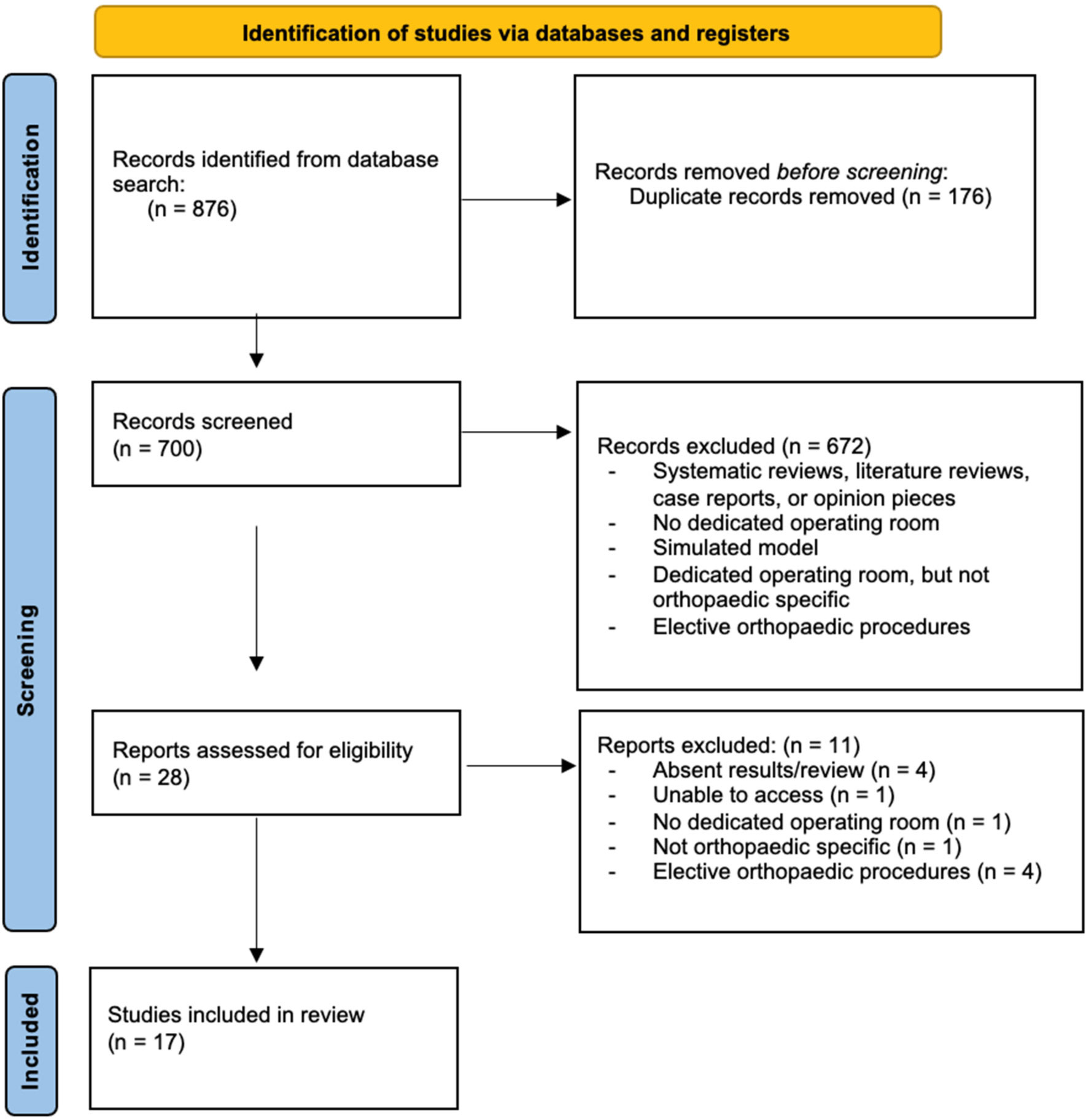
3.1.   Study selection (see Figure 1)

After the removal of duplicates, there were 700 articles left for review. Titles and abstracts were screened for all 700 articles, leaving 28 articles left for review. Full text review was performed on all 28 articles; 17 articles met the full eligibility criteria. The PRISMA flow diagram is shown in Figure 1.

3.2.   Study characteristics (see Table 1)

All 17 studies that met the inclusion criteria were retrospective in design. 14 out of 17 (82%) studies were performed at level 1 trauma centers, while the remaining 3 were at a satellite hospital of a tertiary care center, a level 2 trauma center, or a regional hospital (6%, respectively). Additional descriptive information of all 17 studies can be found in Table 1.

4.   Discussion

4.1.   Various models of DOTR (see Table 1)

The first DOTR described in the literature was instituted prior to 1991 in a Canadian tertiary care trauma center [3]. This model consisted of a DOTR Monday through Friday during the first 4-hour block of the operative day. In 1999, Massachusetts General Hospital instituted a DOTR, operating from 7:45 am to 5pm six days per week [7]. At both institutions, the DOTR was controlled by staff orthopaedic traumatologists on a rotating basis. Nighttime call was provided by the following day's surgeon. Since these initial models were described, multiple centers have implemented identical or similar DOTRs [9]–[15].

As DOTRs gained popularity, various models have been implemented to meet the needs of a specific institution. Featherall et al. described a center with a daily DOTR that remained open unless no orthopaedic trauma cases were booked by 5:00pm the night prior. At that point, elective cases were permitted for scheduling [5]. Thompson et al. reported an institution with a twice weekly DOTL, ensuring an available OR for trauma cases as they presented [4]. Runner et. al implemented a Saturday DOTR in a center that previously only had 3 active trauma ORs on the weekend, one that was reserved for general trauma and the other 2 shared by all specialties [16]. Similarly, Taylor et al. created a weekend DOTR at a level II community trauma center [17]. McDonald et al. introduced an abbreviated version of the DOTR from 6 am to 9 am, Monday through Friday, allowing for one case to be scheduled before the start of the elective schedule [18]. Rather than a general DOTR, Whitlock et al. described a dedicated fracture reduction room (FRR) in a fluoroscopy suite at a pediatric center. The FRR was operated on Tuesdays each week [19].

While all the above DOTRs are located at the primary hospital location, Waters et al. described a unique iteration of the DOTR by creating a dedicated satellite trauma room for a pediatric tertiary care center [20]. The DOTR (7:30 am to 5 pm) was at a satellite hospital for 3 days per week in the summer and 2 days per week the rest of the year. Non-emergency, non-multitrauma operative fracture cases were considered for satellite referral with an extensive list of exclusion and inclusion criteria. In addition, short elective cases were permitted when there were openings in the schedule. This study showed no technical or clinical intraoperative complications as a result of care at the satellite location, no cases of compartment syndrome, and no cases requiring transfer back to the tertiary center. The introduction of the satellite trauma room allowed for decreased volume at the tertiary center by referrals that did not require level 1 trauma care.

See Table 1 describing all the models of the DOTR/DOTL that met eligibility requirements.

4.2.   After-hours procedures (see Table 2)

One of the main challenges of the traditional scheduling model is the lack of daytime OR availability for urgent trauma, necessitating the need for after-hours procedures. After-hours procedures have been shown to be associated with worse outcomes, increased complication rates, and increased duration of surgery [6]. Ricci et al. has studied the effect of after-hours surgery on outcomes for femoral and tibial shaft fractures via a prospective comparative study of 243 cases. Results showed that unplanned reoperations were two times higher in the afterhours group compared to the daytime group. In addition, painful hardware was removed in 27% of the after-hours group versus 3% in the daytime group [6]. Another study found that the mean duration of surgery was 14 minutes longer when performed after-hours (P = 0.003) and there were statistically significant higher rates of complications including pneumonia, pulmonary embolism, and surgical site infection after-hours [18].

As a result of the data indicating worsened outcomes associated with after-hours procedures, a primary goal for the implementation of a DOTR is to reduce after-hours procedures and therefore mitigate the associated adverse effects. Of the 17 studies included in this review, 9 reported an outcome related to percent change of after-hours procedures. 6 out of 9 (67%) reported a statistically significant decrease in after-hours procedures, 2 out of 9 (22%) reported a decreasing trend of after-hours procedures, and 1 out of 9 (11%) reported number of after-hours procedures but did not analyze the data. No studies reported an increase of after-hours procedures.

Bhattacharyya et al. found that hip fracture cases performed after-hours was reduced from 29% to 8% (P < 0.01) [7]. Brusalis et. al reported a 48% reduction (P < 0.001) of after hour procedures following the development of a Monday through Friday DOTR (7:30 am to 5 pm) in a pediatric center [9]. Similarly, Wixted et al. found a 16% decrease in cases performed from 7 pm to 12 am (P = 0.0022) and a 44% decrease in cases performed between 12 am and 7 am (P = 0.003) after the development of a Monday through Friday DOTR [12]. Roberts et al. also described a decrease in after-hours operations from 66.7% to 19.3% (P < 0.001) after the creation of a Monday through Friday DOTR [14]. At an institution that only implemented a DOTR for one case per morning, there was a decrease in after-hours surgery from 32.4% to 19.6% (P = 0.036) [18]. The development of a Saturday DOTR allowed for 59% more cases to be performed on Saturdays, creating a trend towards decreased Monday caseloads and therefore a decrease in cases that must be performed after-hours [16]. A twice weekly trauma list resulted in nearly half the number of operations performed on the weekends compared to before the implementation of the trauma list (P < 0.001). In addition, operations performed after 16:00 hours reduced from 50.5% to 35.8% (P < 0.05) [4].

In summary, the majority of studies that described percent change of after-hours procedures as an outcome measure reported a statistically significant decrease.

4.3.   Time to surgery (see Table 3)

Time to surgery (TTS) is often used as a quality measure for operating room efficiency. With the traditional scheduling model, a lack of OR availability has historically led to increased TTS. It was hypothesized that a DOTR would provide an available OR for urgent trauma and therefore decrease the TTS. However, some have concerns that the guaranteed availability of a daytime OR would result in a delay of the case to the following day, regardless of the urgency [21].

Of the 17 studies included in this review, 13 reported an outcome related to time to surgery. 4 out of 13 (31%) reported a statistically significant decrease in time to surgery, 3 out of 13 (23%) reported a statistically significant increase in time to surgery, and 6 out of 13 (46%) reported no statistically significant difference.

At a pediatric center with a Monday through Friday DOTR, there was a statistically significant reduction in mean TTS for supracondylar humeral fractures, lateral condylar fractures, and tibial fractures [9]. Serving as a control, the same study demonstrated no statistically significant difference in TTS for urgent appendectomies during that time. At a different center, patients admitted on a Friday were found to have a 25.1-hour mean reduction (P = 0.06) in TTS following the development of a Saturday DOTR [16]. Similarly, Elder et al. found a decrease in TTS, with a mean of 56.5 hours at a center without a DOTR versus 29.3 hours at a center utilizing a DOTR (P < 0.001) [3]. Taylor et al. found a decreasing trend in mean TTS following the implementation of a weekend DOTR [17]. Cloud et al. demonstrated a statistically significant decrease in median time to intramedullary nailing of diaphyseal femur fractures, which allowed for a statistically significant decrease in placement of temporary external fixators from 15% to 2.9% [22]. Similarly, Denisiuk et al. demonstrated a statistically significant mean decrease in TTS for pertrochanteric hip, femoral neck, and femoral shaft and distal femur fractures (FSDF) after the implantation of a DOTR [23].

Alternatively, Steeby et al. found that following the implementation of a DOTR, the average time to debridement for open tibia and femur fractures was significantly longer in the DOTR group versus the non-DOTR group (12.9 hours vs 5.4 hours, P = 0.044) [11]. Additionally, patients were 9 times less likely to undergo debridement within 6 hours when in the DOTR group. However, rates of debridement in 24 hours were similar between the on-call OR and the DOTR and the incidence of malunion, infection, or amputation between the DOTR group and the on-call OR was equivocal. Similarly, Lemos et al. found an increase in TTS after implementing a DOTR 4 days per week, with the mean TTS 72.1 hours after implementation versus 56.5 hours before (P = 0.006) [10]. In patients with tibial fractures, Knight et al. observed an increased TTS from 11.04 hours to 29.04 hours following the creation of an orthopaedic trauma list (P = 0.01) [15]. Knight et al. also analyzed the effect of the trauma list on the time from referral to surgery for patients with hand tendon injuries, showing an increase of 1.06 days to 2.82 days after implementation (P = 0.001). As mentioned above, multiple studies reported no significant difference in TTS after the implementation of a DOTR/DOTL [4],[12],[18],[19].

In summary, there is variability in the data regarding the effect of a DOTR/DOTL on time to surgery.

4.4.   Duration of surgery (see Table 4)

With the development of DOTRs, it was hypothesized that duration of surgery (DOS) would be decreased due to availability of familiar operating room staff, presence of equipment representatives, and less surgeon fatigue.

Of the 17 studies included in this review, 10 reported an outcome related to duration of surgery. 7 out of 10 (70%) reported no statistically significant difference, 2 out of 10 (20%) reported a statistically significant decrease in duration of surgery, and 1 out of 10 (10%) reported a statistically significant increase in duration of surgery.

Chacko et al. found a statistically significant decrease in the duration of dynamic hip procedures performed during the day compared to the nighttime group, in addition to a statistically significant decrease after the DOTR became available [13]. Similarly, Elder et al. found the mean DOS for hemiarthroplasties to decrease from 77 minutes to 60 minutes (P < 0.0001) after the development of a DOTR [3]. In both cases, decreased DOS was associated with decreased blood loss in procedures.

Alternatively, Lemos et al. reported an on average 10-minute increase in the DOS for hemiarthroplasties after introducing a DOTR [10]. Similarly, Wixted et al. found a 21-minute increase in intramedullary nailing following implementation of the DOTR, however these results were not statistically significant (P = 0.27) [12]. As noted above, multiple other studies reported no significant difference in the DOS [9],[14],[15],[17],[18],[22].

In summary, the majority of studies that described DOS as an outcome measure reported no significant effect of a DOTR on DOS.

4.5.   Length of stay (see Table 5)

By providing increased OR availability, it was originally hypothesized that the implementation of a DOTR would result in a decreased length of stay (LOS) for trauma patients [7]. Of the 17 studies included in this review, 14 reported an outcome related to LOS. 7 out of 14 (50%) reported a statistically significant decrease in LOS, 1 out of 14 (7%) reported a statistically significant increase in LOS, and 6 out of 14 (43%) reported no statistically significant difference.

Brusalis et al. described a decrease in inpatient hospitalization by 5.6 hours (P < 0.001) after implementing a Monday through Friday DOTR at a pediatric center [9]. This seemingly minor decrease in LOS resulted in significant cost savings per patient (discussed further below). At a different center, patients undergoing hip hemiarthroplasty had a decreased LOS by 4.6 days (P = 0.04) after the implementation of a Monday through Friday DOTR [14]. At a center where a DOTR was only implemented on Saturdays, Runner et al. found a significant decrease of LOS by 2.7 days (P = 0.018) [16]. In addition, Taylor et al. found a significant decrease in mean LOS from 11.6 days to 9.4 days (P = 0.005) after the implementation of a weekend DOTR [17]. Thompson et al. reported that at an institution with a twice weekly DOTL, patients on the DOTL stayed on average for 3 less days than those not on the DOTL (P < 0.05) [4]. Denisiuk et al. also demonstrated a statistically significant decrease in LOS for femoral neck fractures and pertrochanteric hip fractures. Of note, after the implementation of a DOTR there was an increased emergency department LOS [23]. Three studies reported a trend towards decreased LOS, however results were not significant [11],[15],[22]. At a pediatric center that developed a weekly, dedicated fracture reduction room, time from admission to discharge decreased by 67 minutes on average (P < 0.001) [19].

Contradictorily, the LOS for patients with low energy femoral neck fractures at one institution was found to significantly increase following the development of a DOTR by an average of 4 days (P = 0.02) [3]. It should be noted however, that patients were more likely to be discharged home rather than to another health care center after the implementation of the DOTR compared to before. Lemos et al. reported a 4 day increase in LOS following the implementation of the DOTR, however results were not statistically significant [10]. As noted above, multiple studies reported no significant difference [13],[18].

Although the trend was evident for a decrease in the LOS, the majority of the studies did not report a statistically significant change in the setting of a DOTR/DOTL or did not report on this outcome measure.

4.6.   Cost

Hesitation to implement a DOTR has been centered around concerns that the opportunity cost is too high to block off time when an OR could be used for lucrative elective cases [5]. Moody et al. published the first study to document the per minute cost of running a DOTR with the intention of defining the value of DOTRs [24]. They found that the total per minute cost was ＄16.21 at their level II trauma center, without including professional fees of the anesthesiologist or surgeon.

Of the 17 studies included in this review, 4 reported an outcome related to cost. All 4 studies described a reduction in cost as a result of a DOTR/DOTL. Brusalis et al. calculated that the decreased LOS associated with the DOTR for pediatric trauma patients resulted in a mean cost reduction of ＄1,251 per patient [9]. Similarly, Cloud et al. demonstrated an annual cost savings of ＄261,678 from decreased LOS and OR savings secondary to decreased need for temporary external fixation [22]. Following the implementation of a Saturday DOTR, Runner et al. calculated a ＄1.13 million per year savings due to decreased LOS [16]. Whitlock et al. also found cost savings from a dedicated fracture reduction room as well, with patients treated in the fracture reduction room accruing charges of ＄5,299 +/− ＄1,289 versus ＄10,455 +/− ＄2,290 in the OR (P < 0.001) [19].

In summary, significant cost reduction has been demonstrated with a DOTR/DOTL, however, the majority of studies did not report on this outcome measure.

4.7.   Surgical complications

By providing traumatologists with ideal operating conditions, it was hypothesized that the availability of a DOTR would lead to decreased complications. Published literature regarding post-DOTR complications fall into many different categories, therefore, we opted to organize them as follows: mortality, unplanned reoperations, postoperative ICU admission, and miscellaneous complications.

4.7.1.   Mortality

Of the 17 studies included in this review, 7 reported an outcome related to mortality. 2 out of 7 (29%) studies reported a statistically significant decrease in mortality, while 5 out of 7 (71%) studies reported no significant difference. No studies reported an increase in mortality.

Chacko et al. published a report showing that the 1-year and 2-year mortalities of hip fracture patients were significantly less after the implementation of the DOTR, from 25% to 13% and 37% to 15%, respectively [13]. Similarly, Roberts et al. found a significant decrease in postoperative mortality in patients with femoral neck fractures following the development of a DOTR, from 5.6% before the DOTR to 0% after (P = 0.04) [14]. On the contrary, multiple studies noted no significant difference in the postoperative mortality [10],[17],[18],[22],[23].

Although the trend was evident for a decrease in the mortality associated with the respective procedures performed, the majority of the studies did not report a statistically significant change in the setting of a DOTR/DOTL.

4.7.2.   Unplanned reoperation

Of the 17 studies included in this review, 2 reported an outcome related to unplanned reoperation. Both studies reported a decreased rate of unplanned reoperation following the implementation of a DOTR/DOTL.

Brusalis et al. recorded a 53% reduction (P = 0.018) in unplanned reoperation for supracondylar humeral fractures after the implementation of a DOTR [9]. Similarly, Steeby et al. found an increased incidence of unplanned return to the OR for the on-call OR (42%) when compared to the DOTR (27.5%) (P = 0.018) [11]. While not analyzing the effects of a DOTR, Ricci et al. found that femoral or tibial shaft operations performed at night were twice as likely to require an unplanned reoperation (as discussed above) [6].

Although the trend was evident for a decrease in unplanned reoperation associated with the respective procedures performed, the majority of the studies did not study this outcome measure.

4.7.3.   Postoperative ICU admission

Of the 17 studies included in this review, 2 reported an outcome related to postoperative ICU admission. Both studies reported a decreased rate of postoperative ICU admission following the implementation of a DOTR/DOTL.

McDonald et al. found a statistically significant decrease (7.0% pre-DOTR vs 3.8% post-DOTR, P = 0.036) in hip fracture patients requiring postoperative ICU transfer following the development of a 6 am to 9 am DOTR [18]. Roberts et al. reports similar findings with a 9.3% decrease (P = 0.02) of postoperative ICU transfer for patients undergoing operative treatment of femoral neck fractures [14].

Although the trend was evident for a decrease in postoperative ICU admission, the majority of the studies did not study this outcome measure.

4.7.4.   Miscellaneous complications

Steeby et al. found that following the implementation of a DOTR, primary fracture union was two times more likely when compared to the non-DOTR group for debridement of open tibia and femur fractures (P = 0.003) [11]. Lemos et al. also found that patients had significantly more complications before the implementation of the DOTR, including urinary tract infections, pressure sores, and cardiac complications (P < 0.001, P = 0.012, P = 0.046, respectively) [10]. Roberts et al. demonstrated a statistically significant decrease in any postoperative complication by 17% following the implementation of the DOTR (P = 0.04) [14]. Contradictorily, Cloud et al. demonstrated no statistically significant difference in complications including sepsis, surgical site infection, respiratory failure, or pulmonary embolism [22].

5.   Limitations

A limitation of this review is the heterogeneity of the data collected among all the studies. There is variability in the published literature regarding the injuries and surgical methods analyzed and the model of DOTR/DOTL used by various institutions. In addition, the studies included in this review were retrospective and the majority included a before and after design. A major disadvantage of before and after studies is that large changes can be made in processes and healthcare systems that affect outcomes, confounding the outcomes related to the specific intervention being studied [25]. As a result, there is a potential for a lack of generalizability of these findings. To account for this limitation, multiple studies have run mathematical and graphical simulations to determine situations in which a dedicated emergency operating room is advantageous. Importantly, these models were analyzing trauma of all specialties and often specifically excluded orthopaedic trauma [26]. Additionally, there was no distinction between emergent and urgent trauma, a foundational aspect of this review. Findings amongst these studies were split, with some favoring a dedicated room [27],[28] while others favored dividing trauma amongst elective ORs [29]. Bowers et al. developed a simulation specifically for orthopaedic trauma and found that the utilization of trauma rooms can be increased by scheduling elective cases within the “dedicated” trauma time [30]. However, this model did report any morbidity or mortality outcomes related to potential delays in delivery of trauma care.

6.   Conclusions

This review suggests that DOTRs/DOTLs have favorable effects on after-hours procedures, cost, and surgical complications. There is variability among the data regarding the effect on TTS, DOS, and LOS.

7.   Interpretation

There is evidence to suggest that the DOTR/DOTL is beneficial in many regards for the orthopaedic patient, the orthopaedic surgeon, and in cost savings for the hospital. However, more research must be done to study the effects of the DOTR/DOTL on scheduling for other surgical specialties.