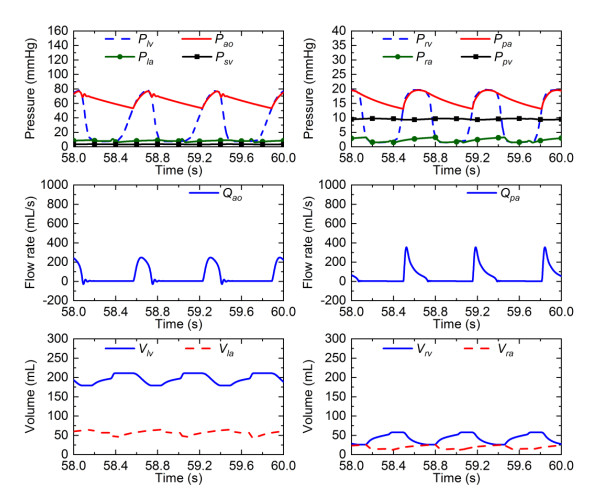
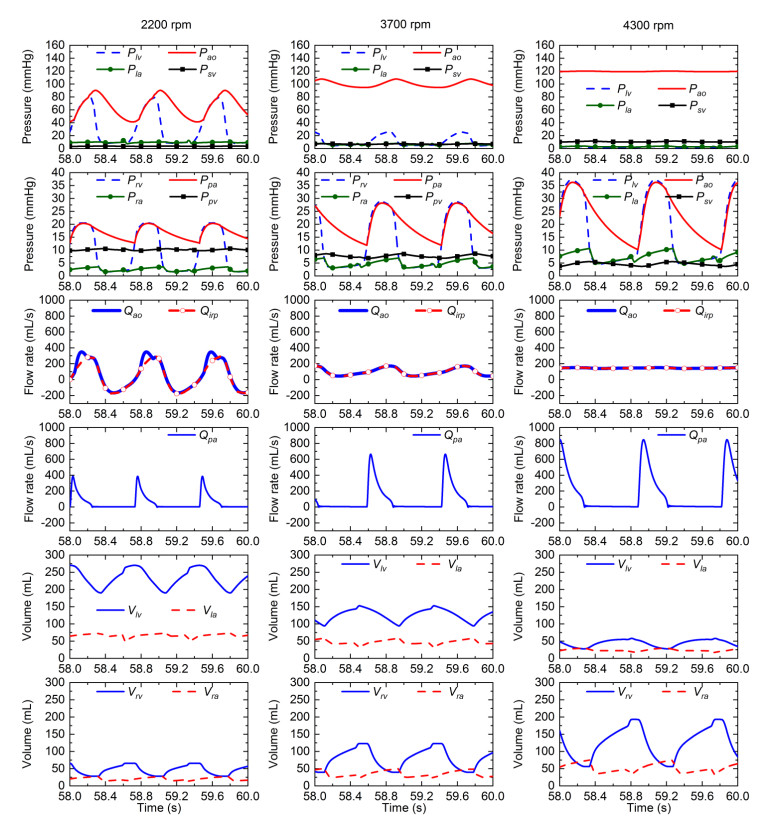
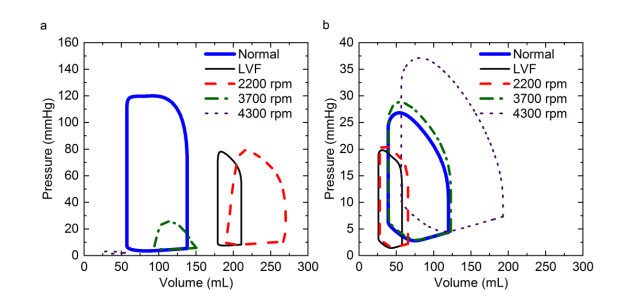
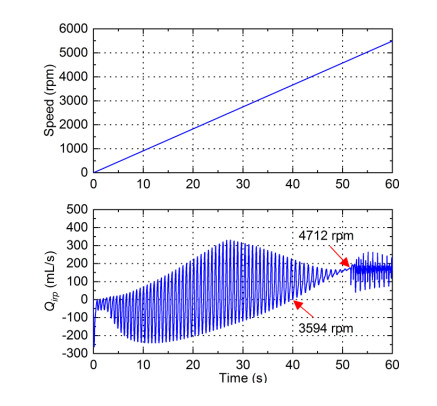
The mathematical modeling of the cardiovascular system is a simple and noninvasive method to comprehend hemodynamics and the operating mechanism of the mechanical circulatory assist device. In this study, a numerical model was developed to simulate hemodynamics under different conditions and to evaluate the operating condition of continuous-flow left ventricular assist device (LVAD). The numerical model consisted of a cardiovascular lumped parameter (CLP) model, a baroreflex model, and an LVAD model. The CLP model was established to simulate the human cardiovascular system including the left heart, right heart, systemic circulation, and pulmonary circulation. The baroreflex model was used to regulate left and right ventricular end-systolic elastances, systemic vascular resistance, and heart rate. The centrifugal pump HeartMate Ⅲ used as an example to simulate the rotary pump dynamics at different operating speeds. Simulation results show that hemodynamics under normal, left ventricular failure and different levels of pump support conditions can be reproduced by the numerical model. Based on simulation results, HeartMate Ⅲ operating speed can be maintained between 3600 rpm and 4400 rpm to avoid pump regurgitation and ventricular suction. Additionally, in the simulation system, the HeartMate Ⅲ operating speed should be between 3600 rpm and 3800 rpm to provide optimal physiological perfusion. Thus, the developed numerical model is a feasible solution to simulate hemodynamics and evaluate the operating condition of continuous-flow LVAD.
1.
Introduction
With the relentless advancement of global financial integration, financial volatilities emanating from developed economies have acquired more far-reaching consequences. Economic and financial tumults instigated by specific national events or policies possess a contagious quality, disseminating through intricate channels to other nations. Particularly for hegemonic economies such as the United States and the Eurozone, their internal economic and financial oscillations wield a potent influence on other countries, inducing a considerable degree of synchronous fluctuations in global financial systems.
The issue of monetary policy spillovers can be traced back to the 18th-century work "Essays, Moral, Political, and Literary" by David Hume (Coeure, 2016). In 2008, the subprime crisis, with the United States as its primary epicenter, engendered a domino effect causing severe perturbations and retrogressions in the global economic and financial systems. In the aftermath, the United States repeatedly adopted quantitative easing measures, while Europe and Japan successively initiated low-interest rate policies (Lenza et al., 2010). The policies aimed at mitigating the subprime crisis demonstrated strong external effects, invigorating economic recovery in other countries, especially emerging economies, through complex channels of financial flows between economies. The European sovereign debt crisis further spilled over globally (Beirne & Fratzscher, 2012), directly leading to a protracted period of negative interest rate policy in the Eurozone. Taylor (2013) retrospectively examined instances supporting or opposing coordinated monetary policy from the mid-1980s to 2013, and extensive prior research has rigorously analyzed the international spillover effects of monetary policy using theoretical models. During the spread of COVID-19, major world economies once again endeavored to stimulate economic recovery through quantitative easing, reigniting scholarly attention on the spillover effects of monetary policies among economies (Ahiadorme & Adenutsi, 2023; Filho & Neto, 2023).
Amidst the ascendance of industrial economics, China has gradually unveiled its dominant stance in international trade and diplomatic realms. However, in the sphere of international finance, China has largely remained in a receptive role, continually buffeted by the monetary policies of developed economies. The frequency and amplitude of monetary policy adjustments in major developed economies are substantially more robust compared to China. For instance, post the subprime crisis, the trajectory of the Federal Reserve's monetary policy could be succinctly delineated as follows: "Rate Cuts → Zero Interest Rates → Emergency Quantitative Easing (QE) → Recovery-Oriented QE → QE Tapering → QE Exit → Rate Hikes → Balance Sheet Reduction." In the wake of the COVID-19 outbreak, the Federal Reserve instigated various market-saving measures, including reducing the benchmark interest rate to near-zero levels, reactivating quantitative easing and launching several emergency loan initiatives. The European Central Bank has maintained an enduring unconventional negative interest rate policy.
Figure 1 illustrates the specific trends of benchmark interest rates for major developed economies (USA: Federal funds rate; JPN: Bank of Japan's overnight lending rate; EUR: European Central Bank's overnight deposit rate; CHN: People's Bank of China's demand deposit rate).
By scrutinizing Figure 1, it becomes evident that, unlike the United States and other developed economies that frequently resort to oscillations in interest rates as a monetary policy instrument, China exercises restraint in modifying its monetary policy. In this context, the flow of international capital is predominantly shaped by the central banks of developed economies, relegating China to a more passive role. As the world economy undergoes a seismic transformation unparalleled in the past century, China is ardently pursuing financial liberalization. Initiatives like easing the eligibility criteria for foreign institutional investors, as well as the inauguration of the Shanghai-Hong Kong Stock Connect and Shenzhen-Hong Kong Stock Connect, have progressively unfastened the doors to China's financial markets. However, the fluidity of the external environment remains one of the salient challenges impeding China's development in this new era. Since 2020, the Federal Reserve's reactivation of the QE policy has led to a ripple effect, engendering global liquidity and inevitably impacting other nations (Zhang et al., 2020). Unconventional monetary policies carry discernible risks of spillover and exhibit period-specific traits (Yang & Zhou, 2017; Chandrasekhar & Ghosh, 2018), which could culminate in substantial retractions during future economic recovery phases. Hence, a meticulous examination of how developed economies' monetary policies are transmitted to China's financial markets, bolstering China's resilience against financial risks and preparing for potential liquidity contractions due to future interest rate hikes are an array of topics requiring anticipatory analysis.
The effects of monetary policy, especially unconventional strategies by key western economies, have become a significant topic in contemporary research. Central banks from several nations have turned to nontraditional tools, such as QE and the Securities Markets Programme (SMP), to counteract liquidity shortages and stimulate economic growth. Studies like Rogers et al. (2014) and Meinusch & Tillmann (2016) validated the effectiveness of such tools, emphasizing their capacity to stimulate financial markets, influence real economic activity, interest rates, inflation and equity prices. On the other hand, Bhar et al. (2015) highlighted the bolstering effect of the Federal Reserve's QE on investor confidence, but left its impact on long-term rates and employment as a topic of debate. Eser & Schwaab (2016) similarly demonstrated the effectiveness of the European SMP in enhancing liquidity and reducing default risk premiums in sovereign bond markets. Inflation was also a major outcome (Seidl, 2023).
The interconnection of global financial markets has been underscored by Gai & Kapadia (2010), noting the increased complexity due to the advent of sophisticated financial instruments. This intricate network of interdependencies implies that the monetary policies of dominant economies, such as the United States or the Eurozone, can profoundly influence other nations. Despite traditional models suggesting monetary independence under flexible exchange rates, Edwards (2018) posits that true independence may be a mirage due to policy spillover effects.
In international studies, spillover effects have been extensively examined using interest rates and bond yields as indicators (Cronin, 2014; Lee et al., 2016; Sowmya et al., 2016; Garcia-de-Andoain & Kremer, 2017; Belke et al., 2018; Berisha et al., 2018; Galariotis et al., 2018). The United States stands out as a focal point in such studies given its economic prominence. Hausman & Wongswan (2011), Wongswan (2006) and Gupta et al. (2018) underscored the significant influence of the Federal Reserve's policies on global financial markets, particularly in Asia and Europe. Such effects, as identified by Nsafoah & Serletis (2019), challenge the independence suggested by conventional theories. Furthermore, Claus et al. (2016) identified spillover effects between the United States and Japan.
Regarding temporal variances, studies by Cook & Devereux (2016) and Antonakakis et al. (2019) emphasized that the intensity of spillover effects can fluctuate, being more pronounced during exceptional periods like the 2008 Global Financial Crisis. Claus et al. (2016) supported this notion, suggesting a more pronounced spillover during periods of unconventional monetary policy between the United States and Japan.
Although abundant research has been conducted in this field, the post-pandemic era warrants timely insights. Hence, this paper incorporates data ranging from January 2002 to September 2021 to yield more contemporaneously relevant conclusions. Applying advanced multi-factor analysis method TVP-FAVAR, this paper constructs a comprehensive and time-variant financial market stress index as a proxy for China's financial markets. The marginal contributions of this paper are manifested in several dimensions:
(1) Heterogeneity of Subjects: In the 21st century, the global landscape transitioned from a "uni-polar to multi-polar" framework. Consequently, the focus of financial research on China should shift from an exclusive concentration on the United States to a comprehensive analysis that encompasses all major global economies. This paper includes multiple developed economies in its analytical framework, thereby allowing for the investigation of heterogeneous monetary policy impacts on China's financial markets, emanating from major economies like the United States and the European Union, as well as smaller economies like Japan.
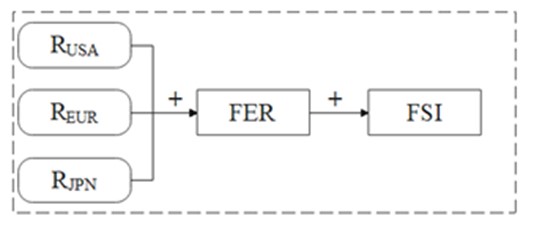
(2) Transmission Mechanisms: China's financial markets remain in a nascent stage of development, and their operational modes and regulations have yet to be fully harmonized with international standards. Given the extant capital arbitrage and irrational behaviors, mere observation of financial markets does not suffice in capturing the full scope of the spillover effects from foreign monetary policies on China's economy. Correspondingly, with the increasing internationalization of the Renminbi, greater attention must be given to the roles played by foreign exchange markets and capital flows. This paper extends its analysis to bonds, currency and exchange rates, thereby achieving more universally applicable conclusions. Two transmission pathways—"Monetary Policy → Short-term Capital Flows → Financial Markets" and "Monetary Policy →Foreign Exchange Reserve Changes → Financial Markets"—are established to elucidate the channels through which developed economies' monetary policies impact China's financial markets.
(3) Time-Variant Features: After enduring multiple large-scale economic crises, the global financial environment has undergone significant transformations. China's level of openness has also continuously expanded, rendering static evaluations of the impacts of developed countries' monetary policies insufficient. Therefore, this paper employs dynamic spillover indices based on the TVP-VAR-DY model for characterizing the time-variant nature of spillover effects. Previous studies have primarily focused on static spillovers. Furthermore, this paper identifies three critical time points for analyzing the unique characteristics of monetary policy spillover effects from developed economies on China's financial markets during general economic crises and the COVID-19 pandemic.
The study investigates the spillover effects of monetary policies in developed nations on China's financial markets. Section two introduces the research methodology, relying on economic and financial theories and utilizing the TVP-VAR-DY index for empirical evidence. Section three covers data preprocessing using the TVP-FAVAR model to create China's financial stress index (FSI) and elaborates on the data sources and variables involved. Section four delves deep into the empirical effects of major developed economies' monetary policies on China's financial sectors, analyzing different dimensions of these effects and their transmission mechanisms. Section five provides robustness checks by replacing price-based policy data with quantity-based data while maintaining a similar approach to section four. The final section concludes the findings and offers recommendations.
2.
Research design
2.1. Theoretical framework
The spillover effects of the monetary policy refer to how one economy's monetary decisions impact other nations. Two primary theoretical frameworks for such analysis are the Mundell-Fleming Dornbusch (MFD) model and the New Open Economy Macroeconomics (NEO) model. The MFD model suggests that monetary policy changes in foreign economies can impact a country's domestic economy, especially through imports, exports and exchange rates (Bouakez & Eyquem, 2015). On the other hand, the NEO model asserts that a nation's macroeconomic health is sensitive to another country's monetary policy shifts. A rise in money supply might cause temporary drops in interest and exchange rates due to price stickiness, leading to domestic currency depreciation. This depreciation makes global goods cheaper, boosting domestic exports, income and consumption (Obstfeld, 2001). China's financial markets, crucial for its economic development, are notably influenced by these spillover effects.
Developed economies' monetary strategies can create positive spillover effects for China's financial markets. These effects are transmitted through three main channels: (a) Balance sheet effects (Devereux & Yetman, 2010): When developed economies adopt a lenient monetary policy, it results in abundant liquidity. This enhanced liquidity improves the asset conditions in other countries, promoting more significant investment and enabling financial institutions to lend more. However, a liquidity crunch can lead to financial stagnation, (b) portfolio reallocation mechanisms (Van Wincoop, 2013): Easy monetary conditions in developed countries can lead to rising asset prices and falling liquidity costs. International investors aiming for profits might shift funds to countries with higher liquidity costs, raising their asset prices and stimulating their economiesand (c) trade linkages (Ammer et al., 2016): Strong trade ties mean that financial shocks in one country can affect others. The wealth effect implies that a robust financial performance in developed nations increases their imports, boosting other countries' exports. Conversely, the competitive effect states that capital inflows from financial shocks can appreciate a country's currency, making their exports less competitive. However, the benefits of the wealth effect often outweigh the competitive effect's negatives.
The spillover effects of monetary policy from developed economies can sometimes negatively influence China's financial markets. The complexities of financial cooperation influenced by differences in legal and institutional structures might hinder harmonious economic interactions between nations (Ostry & Ghosh, 2016). When there's a disparity in financial market development, the road to financial integration might inadvertently produce global financial imbalances (Mendoza et al., 2009). Individual economies tailor their monetary policies to their specific financial cycles, primarily focused on domestic stability and growth. Could these policies, seen in a zero-sum game context, amplify the instability or downturn in other countries' markets?
Three key observations support these concerns: (a) Financial integration primarily represents intertwined capital markets across countries, rather than a uniformity in financial development. While developed economies have advanced financial mechanisms that can adeptly navigate policy shifts, emerging or lesser-developed nations might struggle with significant external monetary changes, (b) financial cycles are not uniform across nations due to varying macroeconomic environments and stages of development. As a result, monetary policies suitable for one financial phase might lead to ill-timed disruptions when applied to a different phase and (c) Monetary policy application faces inherent delays, affecting the timeline of spillover effects. These delays, both internal (time between policy design and its application) and external (time between policy action and its observable impacts), exist in transnational spillovers too.
From this, we propose the hypotheses:
Hypothesis 1: The spillover effects of developed economies' monetary policy on China's financial markets differ in direction and magnitude, contingent on specific conditions, context and timing.
As global trade intensifies and more countries liberalize their markets, capital reallocates from low-yield to high-yield economies in pursuit of higher profits. Using the Federal Reserve's monetary policy as an illustration, when it tightens and raises the federal funds rate, China becomes less attractive for liquid capital if it doesn't match the rate increase. This could lead to capital flowing rapidly into American markets, potentially subjecting China to a liquidity crisis.
Monetary policy adjustments influence not only domestic interest rates but also instigate fluctuations in domestic exchange rates, impacting the relative value of other nations' currencies. A currency devaluation can impair a nation's global ventures. Consequently, countries might swiftly revise their foreign exchange reserve policies. For instance, a rate increase by the Federal Reserve boosts the U.S. dollar's value, making other currencies depreciate in comparison. This could force China to reevaluate its foreign exchange reserves, potentially unsettling its financial market stability. From this, the paper proposes:
Hypothesis 2: Monetary policies of developed economies influence China's financial markets through channels of short-term capital flows and changes in foreign exchange reserves.
2.2. Model setting
Since Sims' introduction of the VAR model in 1980, it has been extensively employed across various domains of macroeconomics. However, the model's assumption of constant coefficients falls short of capturing the nonlinear relationships between monetary policy and financial markets during periods of dramatic change. To rectify this limitation, a plethora of enhanced models has emerged since the 1990s, which includes the more extensive use of nonlinear and time-varying coefficient models. Notably, this paper employs a time-varying coefficient model to empirically examine the spillover effects of monetary policy. More specifically, the study adopts Nakajima's (2011) stochastic volatility TVP-VAR model, which posits that the parameters reflecting monetary spillover effects follow a first-order stochastic process. The volatility undergoes random fluctuations, thereby adequately capturing long-term parameter changes instigated by structural shocks and, consequently, reducing the estimation error of the spillover effects (Li et al., 2022; Chen et al., 2022).
In the first step, we utilize the TVP-VAR model to investigate the impact and time-varying characteristics of the monetary policies of major developed economies on China's FSI. The TVP-VAR model allows for coefficients and the covariance matrix of monetary policy shocks to be time-varying, thereby capturing the evolving influence of developed economies' monetary policies on China's financial markets, as well as the nonlinear dynamics between policy variables and market variables. The underlying structure of the simple VAR model is outlined as follows:
yt is K×1-dimensional observation vector containing the core elements of this article, namely RUSA, RERU, RJAPAN and FSIt. In the mechanism analysis, the channel variable is also included FERt and CFt. section three provides a detailed introduction to the above variables. A is K×K-dimensional simultaneous parameter matrix. To reduce the number of parameters to be estimated and relatively simplify model estimation, it is assumed that the A-matrix is a lower triangular matrix. Considering the availability, timeliness and richness of the data, the study period selected in this article is from January 2002 to September 2021, so t includes 237 data periods. F1 is K×K-dimensional coefficient matrix, perturbation term μt is K×1 dimensional structural impact and μt∼N(0,∅∅), where
Therefore, (1) can be abbreviated as yt=B1yt−1+⋯+Bsyt−l+A−1∅εt,εt∼N(0,IK), where Bi=A−1Fi. Straighten the elements of the B matrix to form k2s×1-dimensional vector β and define Xt=IS⊗(yt−1,⋯,yt−s), where ⊗ represents the Kronecker product. Therefore, the model is simplified as:
Subsequently, time-varying features were introduced, and Nakajima's (2011) approach was adopted to transform At nonzero and one elements in t to one column vector at. Among them, at=(a2,1,a3,1,a3,2,a4,1,⋯,ak,k−1), let ht=(h1,t,⋯,hk,t), where hi,t=log(σ2i,t),i∈[1,k],t∈[l+1,n], then the model is extended to a time-varying parameter VAR with the following form:
The parameters in Equation (3) follow a random walk process, i.e βt+1=βt+μβt,αt+1=αt+μαt,ht+1=ht+μht, t∈[l+1,n], where βl+1∼N(μβ0,∑β0), αs+1∼N(μA0,∑A0), hs+1∼N(μh0,∑h0). At this point, the model can better describe the time-varying characteristics of the impact of monetary policy on China's financial market. For the estimation of time-varying model parameters, this article constructs the Markov chain Monte Carlo (MCMC) algorithm in the background of Bayesian and continuously samples 20,000 times, using the analogue filter to sample the time-varying parameters to ensure effectiveness.
In addition, this article uses the TVP-VAR-DY model to analyze the dynamic spillover index of the impact of monetary policies in major developed economies on China's financial market pressure index. Based on the definition of spillover index in Diebold and Yilmaz (2009; 2012; 2016), this article first converts the TVP-VAR model into the form of TVP-VMA:
where y∗t=yt−¯yt,ut=A−1t∑12tεt. Coefficient matrix Bi,t can be calculated recursively as follows:
B0,t=IK,Bj,t=0 (if j<0), thus, the impulse response function of the financial market pressure index can be expressed as: IRFh=Bh,tA−1t∑12t.
We record the contribution ratio of the orthogonalization impact of the jth variable at time t to the prediction mean square error of the i-th variable in the forward H period as θij,t(H),
∑u,t=A−1t∑t(A−1t) is the disturbance term u. The covariance matrix of t, σjj,t is the jth element on the diagonal of ∑u,t, ei is the selection vector where the i-th element is one and all other elements are zero.
Due to ∑Kj=1θij,t(H)≠1, it needs to be standardized into the following form:
From this ∑Kj=1∼θij,t(H)=1, ∑Ki,j=1∼θij,t(H)=K, a corresponding one-way overflow index can be calculated FROMi,t (the i-th variable is overflowed by all other variables), as well as TOi,t (the total overflow of the i-th variable to other variables) and the total overflow index TOTAL. Therefore, this article cannot only observe the dynamic spillover index of monetary policy in developed economies on the Chinese financial market, but it also considers the monetary policy interaction spillover between developed economies as a control variable to obtain more effective estimates.
3.
Data preprocessing
3.1. Measurement of China's FSI
In prior research, obtaining indicators that sufficiently encapsulate the dynamics of China's financial markets has proven elusive, often resorting to the stock market as a rudimentary surrogate. Addressing this challenge, the current paper follows the methodologies of Koop & Korobilis (2014) as well as Wang et al. (2018), employing a TVP-FAVAR model. This model extracts fundamental factors from five dimensions—namely, bond markets, stock markets, interest rate markets, foreign exchange markets and broader macroeconomics—to analyze China's FSI from January 2002 to October 2021. The procedure is delineated as follows:
Initially, a standard VAR model is established, represented by the equation:
where Ft denotes latent variables, Yt represents observable variables and Φ(L) is a finite-order lag polynomial matrix. vt is a zero-mean error term permitted to be autocorrelated. The limitation of a conventional VAR model lies in its consumption of degrees of freedom, thereby making it difficult to comprehensively reflect financial market conditions. The enhanced FAVAR model can extract factors from large datasets and includes an equation for factor extraction:
Xt represents a large dataset with dimension N×1. N represents the number of economic data, Λf and Λy are N×K and N×M-dimensional factor load matrix, where K represents the number of factors and M represents the number of economic indicators. N is much greater than the sum of K and M, that is, N≫K+M. εt is a random error term with a mean of zero but can be autocorrelated. The result is Ft The combination of factor analysis and VAR is achieved by substituting the estimated value of t into the original general VAR.
Subsequently, akin to the approach in TVP-VAR, TVP are employed to overcome the limitations of FAVAR in capturing the time-varying features of financial markets. To dynamically adjust the coefficients in FAVAR, the following modifications are made:
The parameters Λf,Λy,Φ are allowed to vary over time, and both εi,t and vt are assumed to follow stochastic volatility processes. The MATLAB software is utilized for these estimations and subsequent empirical analyses.
Table 1 outlines the framework and data sources for the FSI, which measures the financial market risk in China. It is composed of 13 variables across five dimensions: bond markets, stock markets, money markets, foreign exchange markets and macroeconomics. These dimensions encompass one-year, five-year and 10-year government bond yields; stock market indices from the Shanghai, Shenzhen and Hang Seng markets (Zhu and Yang, 2022; Zhao and Zeng, 2023); interbank lending rates; the real effective exchange rate index of the Renminbi and macroeconomic variables such as M2 money supply, industrial value added, consumer price index and net exports. Figure 2 exhibits the trend of China's FSI as estimated through the TVP-FAVAR methodology.
3.2. Explanatory variables and transmission channels
This study employs benchmark interest rates from the central banks of the United States, Europe and Japan to represent the monetary policies of developed economies. Since 1982, Western-developed nations exemplified by the United States have shifted the focal point of their monetary policies from the control of money supply to interest rate targets (Zhang, 2012). This paradigmatic shift has galvanized a plethora of scholarly inquiries into the ramifications of interest rate-based monetary policies. For instance, Bekaert et al. (2013) contended that reductions in the federal funds rate served to diminish the equity market's risk premium, while Benchimol & Fourçans (2017) asserted that monetary policies exert analogous directional effects on output and inflation, particularly intensifying during periods of economic tumult. Coibion et al. (2017) argued that contractionary monetary policies exacerbate inequities in aggregate income, spending and consumption, with monetary shocks constituting a salient determinant of cyclical fluctuations in income and consumption inequality. However, when policy rates approach a near-zero lower bound, conventional price-based monetary policies occasionally falter in their effectiveness (Luciani, 2015; Berger & Bouwman, 2017). In accordance with conventional practice, this study selects the federal funds rate (denoted as RUSA), the European Central Bank's overnight deposit rate (denoted as RERU) and the Bank of Japan's overnight lending rate (denoted as RJAPAN) as benchmark rates. Figure 1 delineates the temporal evolution of these benchmark rates for the three principal developed economies from January 2002 through September 2021.
Regarding transmission channels, the study employs short-term capital flows (CF) and changes in foreign exchange reserves (FER) as key variables. CF is quantified through the following equation:
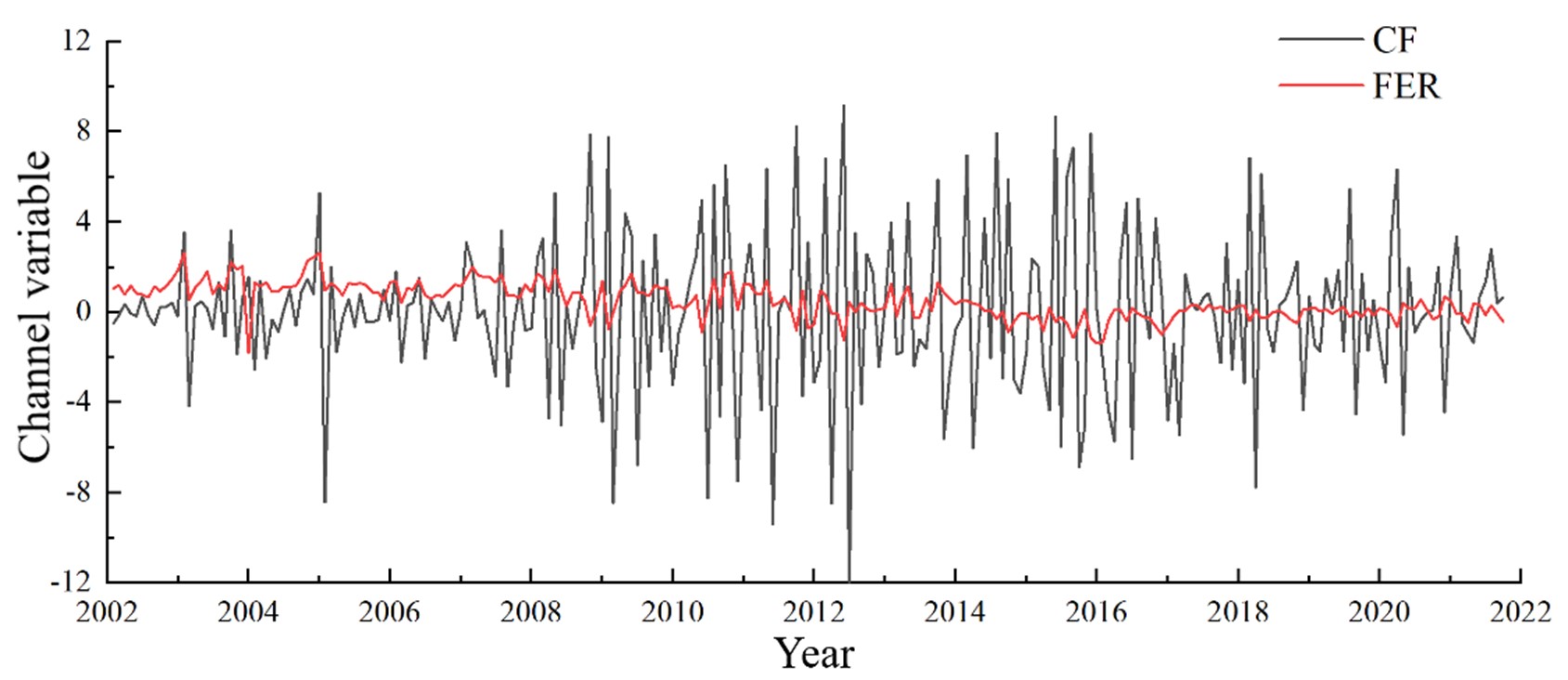
In (15), NIH represents net inflows of hot money, FDI denotes foreign direct investment, FER signifies newly acquired foreign exchange reserves and TS indicates trade surplus. CF represents short-term capital flows, which is the study's initial transmission channel. To ensure data stationarity, first-order differencing is applied. As the second transmission channel, FER is disclosed by the People's Bank of China, log-transformed and treated through first-order differencing. Figure 3 illustrates the fluctuations in the transmission channels CF and FER.
4.
Empirical examination of the spillover effects of monetary policy in major developed economies
Using a model defined by (1) to (10), this study assesses the spillover effects of monetary policies in major developed economies on the Chinese financial market. After confirming the stationarity of all variables through the augmented Dickey-Fuller (ADF) unit root test, the study determines an optimal lag order of two using information criterion methods. During the MCMC simulations, a total of 20,000 consecutive effective samples were generated.
4.1. Analysis of spillover effects based on entity differences
Figure 4 offers insights into how U.S., Eurozone and Japanese monetary policies influenced the Chinese financial market. A look at the responses across three, six, and 12 lag intervals suggests that the Federal Reserve rate fluctuations cause notable short-term shocks on the Chinese financial market, evidenced by a consistent increase in the FSI in response to positive rate shocks. This impact, however, wanes in the medium to long term. The European Central Bank rate changes also affect China's financial market across all durations. However, the potency of this influence diminishes over time, most notably after the Eurozone adopted negative interest rates in 2014. The Bank of Japan's rate adjustments have a limited overall influence on China's financial market, especially in the short to medium term. Surprisingly, the Chinese market's FSI exhibits a decrease in response to positive rate shocks from Japan.
4.2. Analysis of spillover effects based on temporal differences
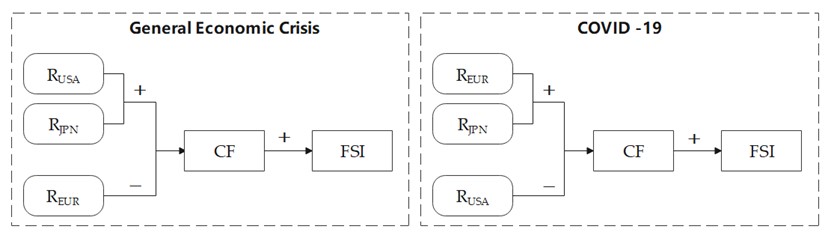
During the study period, three globally impactful and representative "crisis periods" are selected: (a) The 2008 Global Financial Crisis (GFC), pinpointed to October 2008. It is generally accepted that the global economic crisis spiraled out of control in September 2008, prompting subsequent crisis-relief measures from U.S. and European authorities (Li et al., 2022), (b) the European Sovereign Debt Crisis (EDC), dated in December 2009. On December 8, 2009, three leading global rating agencies downgraded Greece's sovereign rating, subsequently affecting other European countries. Greece ceased to be the crisis epicenter, and the debt crisis posed a severe challenge for the entire Eurozone and (c) the outbreak of the COVID-19 pandemic (Cov19), identified in February 2020. On February 28, 2020, the World Health Organization released a report on the novel coronavirus, elevating the regional and global risk level to "very high" and subsequently declaring it a global pandemic (Nguyen and Dinh, 2021; Pan et al., 2021).
Figure 5 delves into the effects of monetary policies from the U.S., Eurozone and Japan on the Chinese financial market during these periods. Post a unit rate shock, the impulse responses during all three crises from the Federal Reserve reveal a consistent pattern, showing a profound monetary policy transmission mechanism on China across varying times. The European Central Bank during the GFC and EDC, showcased a synchronized decay in its impact strength on China. Notably, this decay is faster during the COVID-19 period, hinting at diminished spillover effects amidst negative interest rate environments. The Bank of Japan's monetary policy effects on China, post a rate shock, begin with a positive impact during the first period, which soon turns negative, fading out by the eighth period.
4.3. Analysis of the spillover mechanisms in transmitting effects
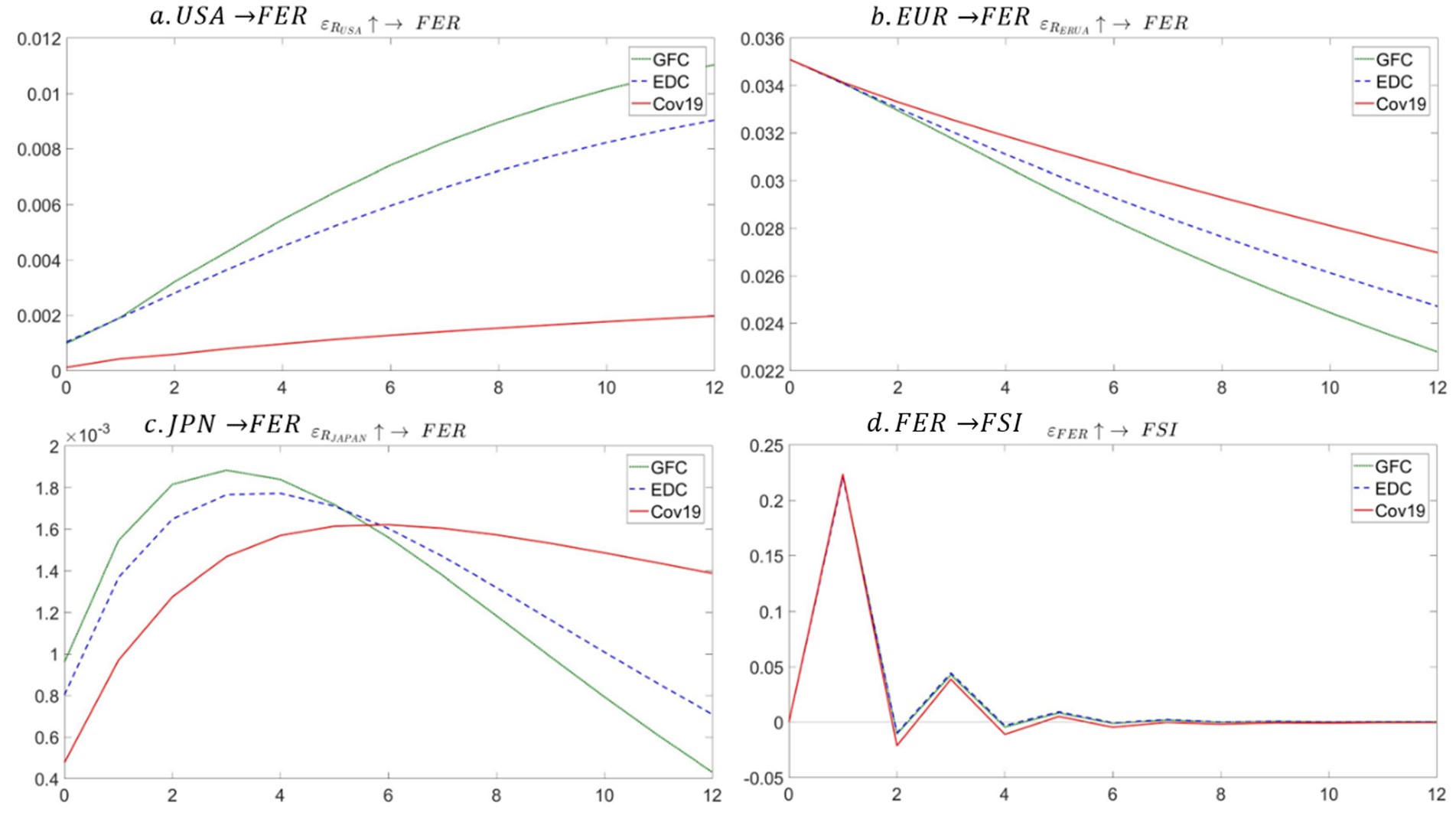
The discourse initially centers on the short-term capital flow channels that act as conduits for the spillover effects. Figure 6 delineates the dynamic alterations in China's FSI under the auspices of short-term capital flows, influenced by monetary policy decisions from major developed economies. Specifically, Figure 6-a illustrates the ramifications of the Federal Reserve's rate hikes at various junctures on China's short-term capital movements; Figure 6-b encapsulates the impact of the European Central Bank's rate adjustments on these flows; Figure 6-c depicts the repercussions of the Bank of Japan's policy shifts and while Figure 6-d outlines the influence of accelerated short-term capital flows at distinct temporal points on China's FSI.
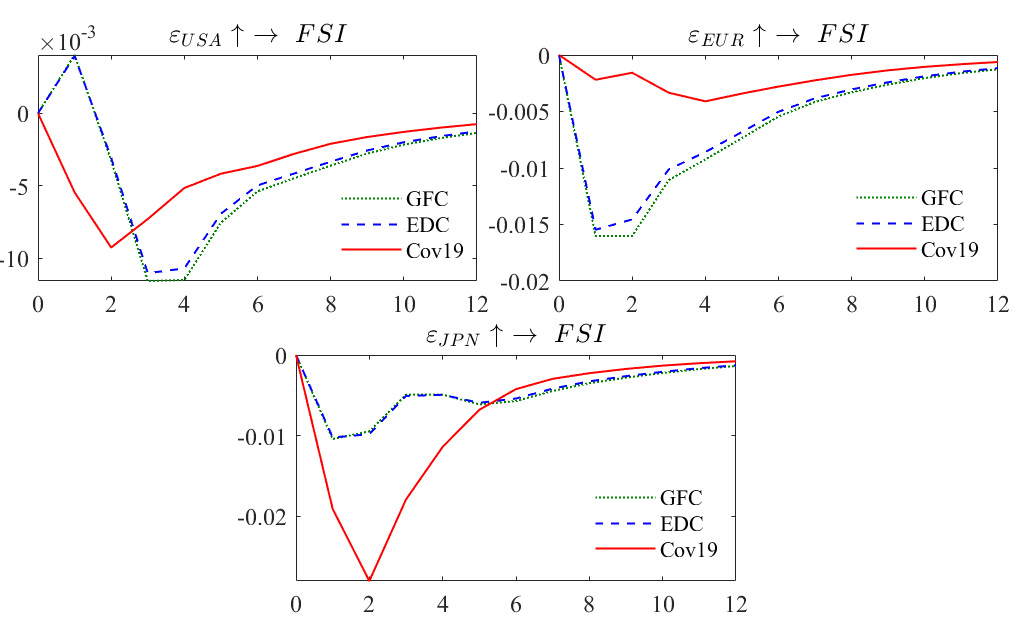
In the context of generic economic crises (represented as GFC and EDC in the figure), the following observations can be made: A standard deviation change in the Federal Reserve's benchmark interest rate engenders a stimulatory effect on China's short-term capital flows, reaching an apogee at a value of 0.005 during the fifth period (GFC), subsequently experiencing a gradual decline. In contrast, a similar fluctuation in the European Central Bank's benchmark rate slightly curtails these flows, peaking at around −0.002 during the fourth and fifth periods (GFC). Meanwhile, the Bank of Japan's interest rate shifts stimulate these flows and, as time progresses, this effect dissipates by the eighth period. Amid the COVID-19 pandemic (represented as Cov19 in the figure), the Federal Reserve's rate fluctuations exert a weak inhibitory influence on China's short-term capital flows; the European Central Bank's rate shifts stimulate these flows, peaking at approximately 0.004 during the sixth period and the Bank of Japan's influence remains congruent with its effect during conventional economic crises.
Furthermore, across various temporal frameworks, the impact of short-term capital flows on China's FSI remains largely consistent. Namely, the immediate pulse response of these flows engenders a 2.8 point upward pressure on the FSI, which reverses to a −1.4 point downward pressure during the subsequent period, thereafter, oscillating between positive and negative effects, ultimately attenuating to zero by the sixth period. Figure 7 presents the transmission mechanism through the channel of short-term capital flows.
The subsequent segment pertains to the spillover effects inherent in the transmission mechanisms of foreign exchange reserve alterations. Figure 8 portrays the impact response graphs, detailing how China's FSI changes under the aegis of foreign exchange reserve channels, influenced by monetary policies from leading developed economies. The graphical representations here align with the sequencing depicted in Figure 6.
It is evident that regardless of the economic milieu—be it in standard economic crises or during the global pandemic—the effect of foreign exchange reserve channels remains largely congruous. A standard deviation fluctuation in the Federal Reserve's benchmark interest rate consistently amplifies China's foreign exchange reserve changes, a promotional effect that escalates over the long term. The European Central Bank's rate adjustments yield a similar stimulatory effect, peaking at around 0.035 in the current period before gradually waning. The Bank of Japan's rate shifts have a promotional impact, reaching an apex at 0.002 during the third period (GFC), subsequently fading with the passage of time. Additionally, across diverse timeframes, the influence of foreign exchange reserve changes on China's FSI remains broadly consistent: The immediate pulse response is a positive 0.23 point influence in the first period, which manifests a lesser positive impact in the third period before tapering off. Figure 9 illuminates the transmission mechanisms of foreign exchange reserve changes.
4.4. Dynamic spillover index analysis
The foregoing tripartite analysis scrutinizes the spillover effects and mechanisms of monetary policies from principal developed economies. Utilizing the TVP-VAR-DY model, according to (4) to (10), we further investigate the dynamic interrelations between developed economies' monetary policies and China's financial markets.
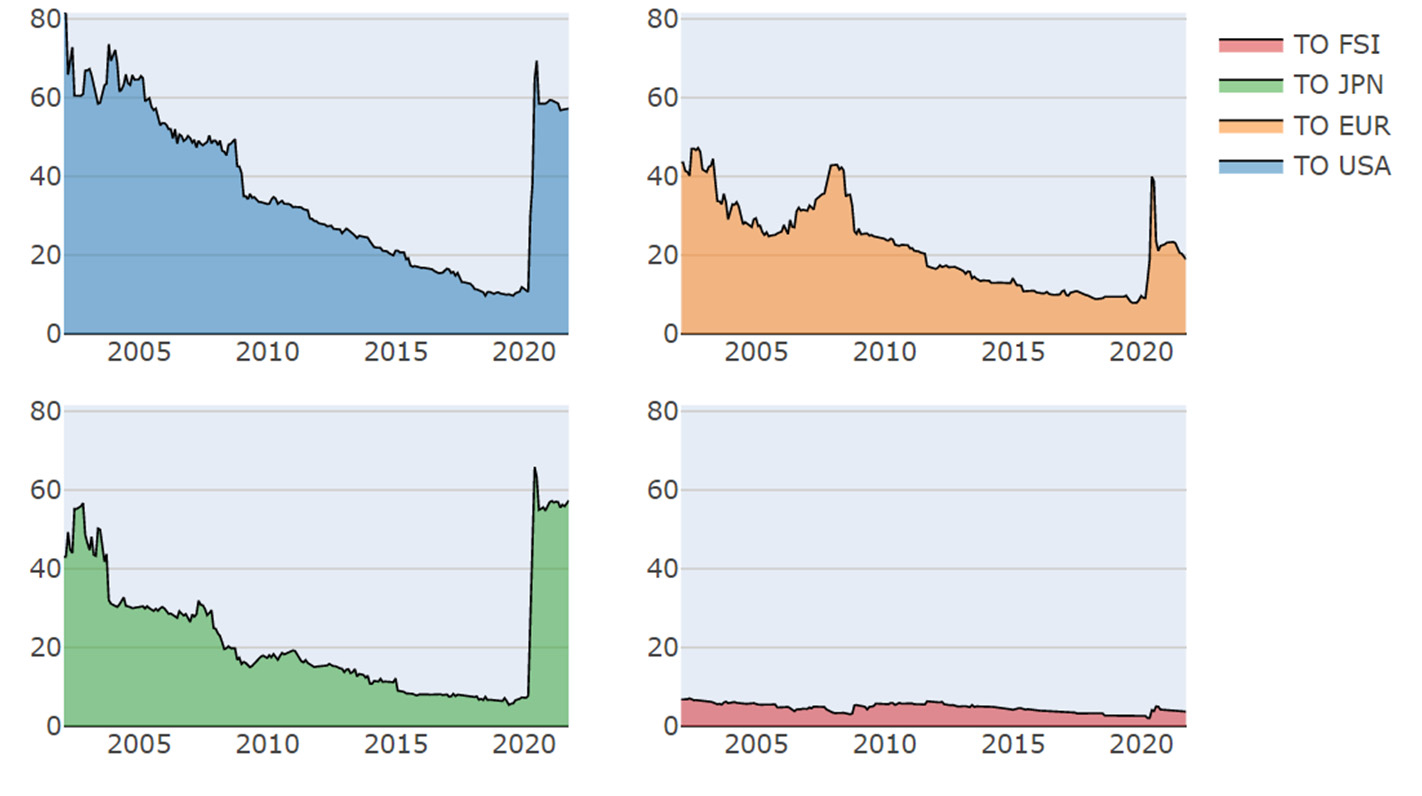
According to (8) and (9), the average dynamic spillover index is computed, facilitating an exploration of the relative magnitude of inter-variable spillovers. Table 2 showcases the average dynamic relationships, wherein "TO others" signifies the aggregate spillover effect of a particular variable on all other variables except itself. "Inc.own" signifies the comprehensive spillover effect of a variable on all variables, including itself; "NET" is derived by calculating "Inc.own-100;" "From others" indicates the aggregate spillover effect from all other variables and "TCI" denotes the total connectivity index, which is the mean of "From others." It is discernible that the Federal Reserve's benchmark interest rate has an aggregate spillover index of 65.51% on other developed economies and China's FSI, the European Central Bank's stands at 72.26% and the Bank of Japan's is at 35.76%, with China's FSI exerting a meager 1.58% spillover effect on other developed economies. In summation, the United States and the Eurozone, owing to their substantial monetary mass, exert a monumental impact on other economies, while Japan's influence is relatively diminutive. Conversely, China's FSI exerts a negligible influence on the monetary policies of developed economies, underscoring China's considerable but isolated sway in global trade markets. In other words, developed economies largely maintain their monetary policy autonomy, impervious to fluctuations in China's financial markets.
According to Table 2, we can also compare the magnitudes of influence that the Federal Reserve, European Central Bank and Bank of Japan's benchmark rates exert on China's FSI. As per the table, the Federal Reserve's spillover index on China's financial markets stands at 15.88%, the European Central Bank's is at 13.73% and the Bank of Japan's is at 5.85%. Evidently, the greater the economic heft of an entity, the more considerable is its spillover effect on China's financial markets.
The foregoing analysis only serves to elucidate the average spillover effects between the years 2002 and 2021 without providing insights into their specific temporal variations. Consequently, this paper employs dynamic trend graphs of the spillover index for further scrutiny (Figures 10 and 11).
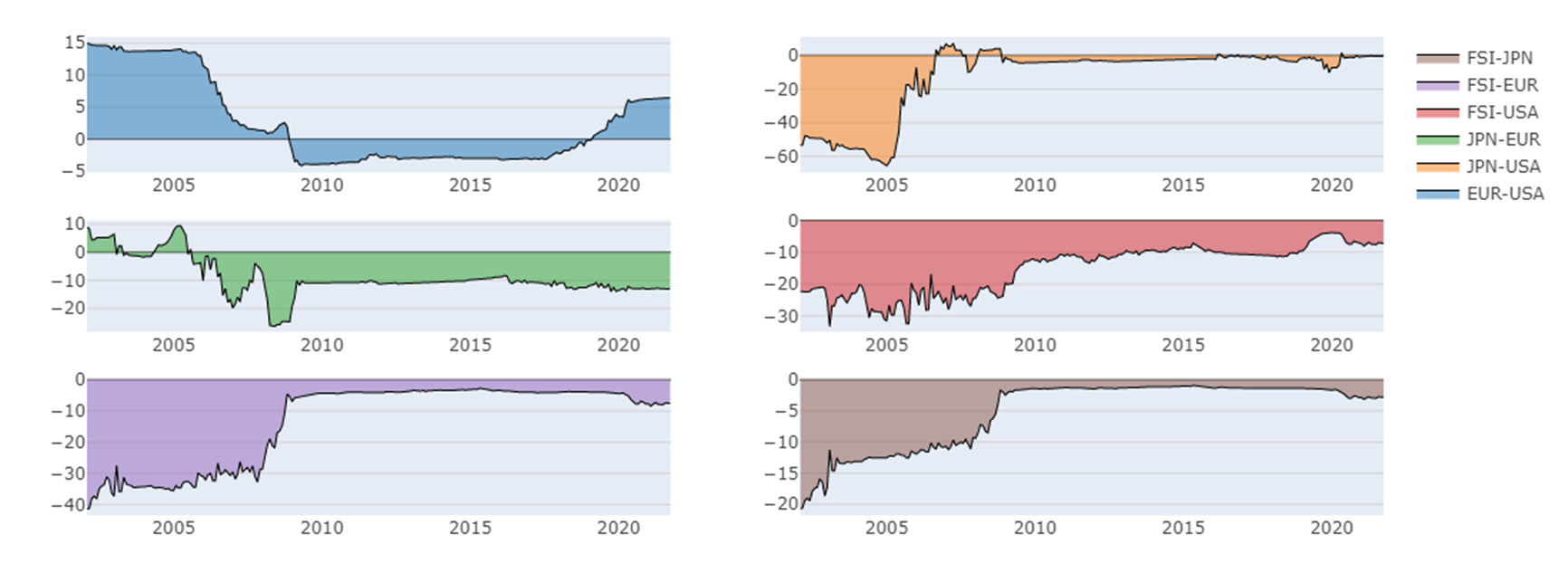
Figure 10 serves as an instrument to delineate the multidirectional correlations among variables, such as the time-varying spillover effects from other variables onto the FSI. Upon comparative examination of the graphical data, this section discerns a palpable spillover effect from the monetary policies of developed economies. This effect has generally weakened around the year 2008. Notably, the FSI in China exhibits negligible outward spillover effects.
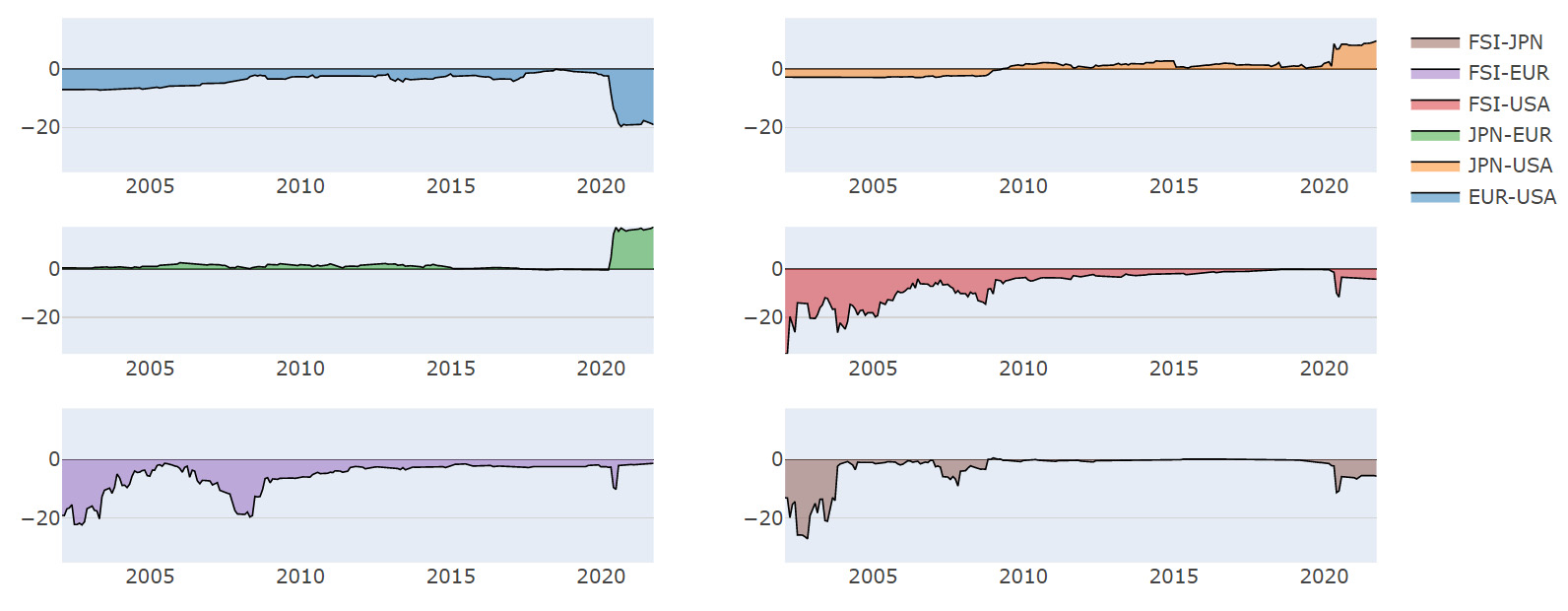
Figure 11 can be utilized to explicate the bidirectional network correlations among variables. For instance, the term "FSI-JPN" encapsulates the time-varying interactive spillover intensity between China's FSI and the Bank of Japan's monetary policy. What becomes evident is that the spillovers between the Federal Reserve's benchmark interest rate and that of the European Central Bank manifest periods of alternation—namely, the former predominates from 2002–2009 and 2019–2021, while the latter assumes a leading role from 2009–2019. The Bank of Japan's benchmark interest rate remains continually influenced by the spillovers from both the Federal Reserve and the European Central Bank. Overall, the spillover effect on China's FSI from the monetary policies of developed economies has been on a decline but experienced a slight uptick following the onset of the pandemic in early 2020.
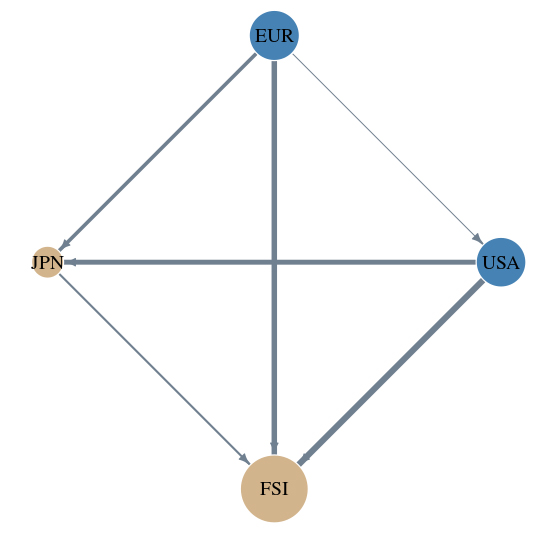
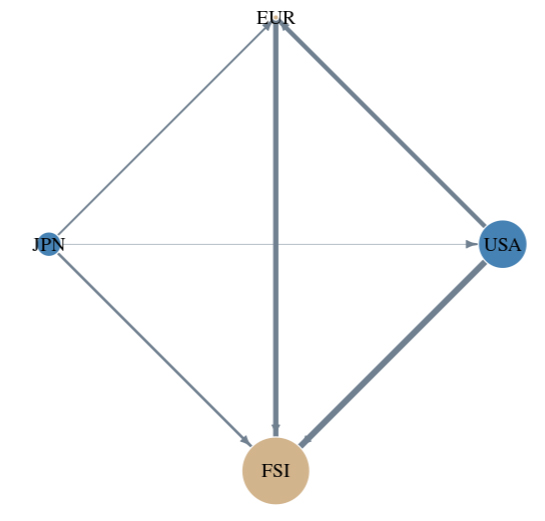
Figure 12, based on the DY index, delineates the specific pathways of interactive spillovers between variables. The blue (yellow) nodes represent the net transmitters (receivers) of shock. Vertex weights are determined through the average network pairwise directional connectivity, with node sizes indicating the weighted average of total network directional connectivity, and arrow thickness denoting the intensity of influence. Observations reveal that: (a) China's FSI is subject to significant spillover effects from global major developed economies' monetary policies, with spillover arrows from both the Federal Reserve and the European Central Bank manifesting greater thickness (intensity), while those emanating from the Bank of Japan display lesser thickness (intensity) and (b) the direction of interactive spillovers between major economies' monetary policies correlates directly with their economic size. Specifically, the Bank of Japan's monetary policy is significantly influenced by spillovers from the European Central Bank and the Federal Reserve, while the latter two, as the dual pillars of western economies, exhibit weaker interactive spillovers between them, reflecting their relative monetary policy independence.
5.
Robustness tests: Substituting monetary policy variables
5.1. Description of substituted variables
In the realm of monetary policy execution, central banks often employ a nuanced blend of interest rates and money supply as intermediate objectives for economic fine-tuning (Albaity, 2011; He & Wang, 2012; Maynard, 2016; Ge, 2019). Scholarly consensus remains elusive regarding the comparative efficacy of these divergent intermediate objectives. A preponderance of studies posits that interest rates outperform money supply as an effective intermediate variable. Quantity-based rules, rooted in Fisher's quantity theory of money, postulate that the velocity of money remains relatively stable in the short-term. However, Mishkin contends that such velocity is too volatile to be considered constant. Keynesian theorists argue that interest rates significantly influence aggregate demand. Through the prism of price mechanisms, which impact individual demand, market expectations and asset prices, balance sheet and wealth effects consequently influence corporate investment and household consumption. In comparison to quantity-based rules, price-based rules demonstrate superior efficacy in providing policy transparency and controlling inflation. Empirical evidence suggests that in mature and developed financial markets, interest rates aptly reflect the price of capital, establishing a nexus between macroeconomic and microeconomic phenomena (Rousseau & Wachtel, 2011). With the evolution of financial markets, the correlation between the money supply and the ultimate objectives of monetary policy wanes. Utilizing money supply as an intermediate variable thus begets complex theoretical and identification challenges. Therefore, compared to money supply, interest rates are more apt as intermediate objectives in monetary policy (Gul et al., 2012).
Contrastingly, a cohort of academics have advanced antithetical or even conflicting arguments, contending that money supply should persist as the intermediate objective of monetary policy. This stance is particularly salient in the context of China, where the interest rate markets are inadequately liberalized. For example, Mehrotra (2007) studied the role of interest rate channels in China's deflationary episodes, concluding that interest rates are not pivotal monetary policy instruments, and neither exchange rates nor interest rate shocks significantly influence price developments. Koivu (2009) employed a vector error-correction model within the credit demand framework to scrutinize whether the influence of past interest rates has intensified. He found that although loan demand is increasingly interest-rate dependent, the channel from interest rates to the real economy remains feeble. Utilizing the FAVAR methodology, He et al. (2013) investigated the efficacy of monetary policy instruments in stabilizing the Chinese economy and found the impact of repo rates, benchmark loan rates and market-based monetary stances on the Chinese economy to be negligible. Li & Liu (2017) posited that, thus far, the objectives for money supply have been more stringent than those for nominal interest rates.
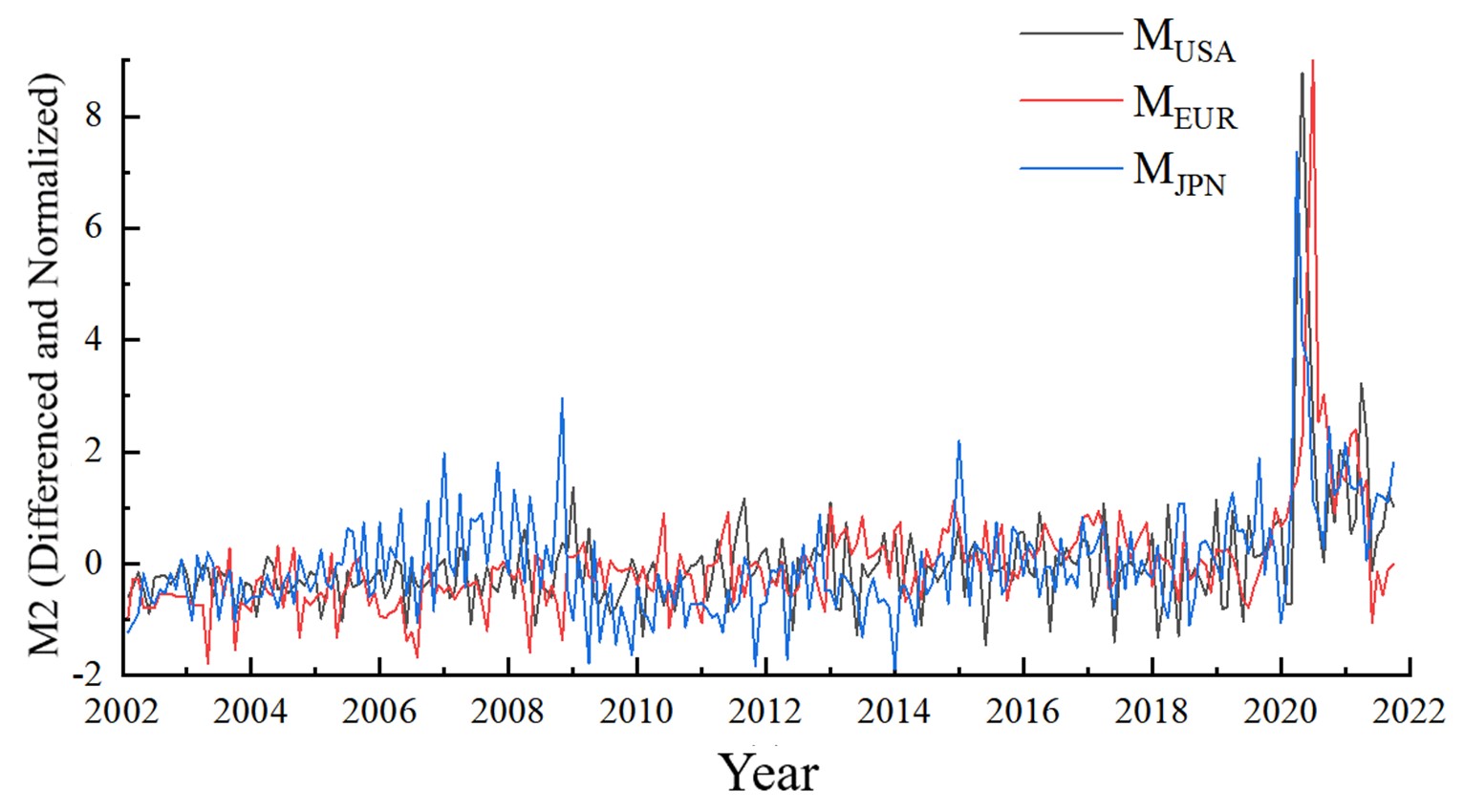
Given that money supply still occupies a significant position in contemporary monetary policy, and in adherence to empirical research rigor, this paper introduces money supply M2 as a secondary proxy variable for the monetary policies of major developed economies for robustness tests. Specifically, data on the money supply M2 for the United States, Eurozone and Japan has been harvested from the BvD-EIU CountryData database, measured respectively in billions of U.S. dollars, billions of Euros and trillions of Yen and has undergone seasonal adjustments and differencing normalization procedures. Figure 13 illustrates the trend changes in the money supply of these major developed economies, revealing a relatively stable trajectory but marked by a pronounced and highly synchronized monetary over-supply in the early stages of the 2020 pandemic outbreak.
5.2. Robustness test of spillover effects based on agent heterogeneity
In this section, we delve into the spillover effects of changes in the money supply of major developed economies on China's financial markets. The focus is on the United States, the Eurozone and Japan. For statistical reliability, all variables underwent unit root tests with a lag of two periods. Moreover, during the MCMC simulation, 20,000 effective samples were generated.
Figure 14 illustrates the impacts of variations in the money supplies of these developed economies on the stress index of China's financial market. Using equidistant impulse responses, the analysis observes effects over short-term (three periods), medium-term (six periods) and long-term (12 periods) durations. For the United States' impact: The graph associated with the Federal Reserve's money supply suggests that changes in the U.S. money supply has a profound short to medium-term effect on China's financial market. Specifically, a one standard deviation increased results in a short-term drop of about 0.006 to 0.015 in China's stress index. Yet, over the long term, this influence appears to wane. For the Eurozone's impact: The graph representing the European Central Bank's money supply indicates that fluctuations in the Eurozone's money supply also has notable short to medium-term repercussions on China's financial market. This results in a decline in the stress index by approximately 0.003 to 0.015. However, post-2020, this effect seems to fade. For Japan's impact: The Bank of Japan's money supply graph shows that Japan's monetary changes shock China's financial market in the short-to-medium-term. A one standard deviation increased leads to a stress index reduction of about 0.002 to 0.018. Over time, the influence of Japan's money supply changes has become more pronounced, possibly influenced by recent Sino-Japanese monetary agreements.
Comparing the results with those from interest rate-based policies suggests a similarity: Contractionary policies (often marked by rising interest rates) from developed economies tend to increase China's FSI. These effects differ based on the country and timeframe.
Further insights from Figure 14 are as follows: (a) Lag effects: There's a discernible lag in the impacts of monetary policies. The U.S. shows the smallest lag (around 0.05 in the short-term), the European Central Bank has a more pronounced lag (close to 0.15) and Japan's falls in between at roughly 0.09. (b) counter-spillover effect: China's market FSI seems to have a counter-reaction to money supply changes in developed economies. This effect is most pronounced in the short-to-medium-term and varies among the three major economies and (c) interactions between economies: Monetary policies of these developed economies reciprocally affect each other. Preliminary findings suggest significant positive short-to-medium-term interactions among the U.S., Eurozone and Japan, proportional to their economic sizes.
5.3. Robustness test of spillover effects based on temporal variations
This study examines the segmented temporal effects of monetary policies from major economies on China's financial market during three significant "crisis epochs": The GFC in October 2008, the EDC in December 2009 and the outbreak of the COVID-19 pandemic in February 2020.
Figure 15 presents the varying impacts of the monetary policies of the United States, the Eurozone and Japan across these epochs. The top left graph pertaining to the Federal Reserve suggests that in the aftermath of an economic downturn, an increase in the monetary supply doesn't immediately reduce China's FSI. Instead, there's a brief positive shock followed by a standard reduction in the stress index, which peaks within three to four periods before fading. However, during the pandemic, a quick boost in the U.S. monetary supply immediately benefits China's financial market with the effect peaking in the second period. This suggests that while the Federal Reserve's policies consistently influence China's market, their effectiveness has grown over time. On the other hand, the top right graph, which depicts the European Central Bank's influence, shows that after an economic crisis, a rise in the monetary supply swiftly reduces China's stress index, peaking between the first and second periods and fading within a year. However, during the pandemic, the European monetary supply's effect on China is muted, possibly due to the extreme negative interest rates in Europe, which make monetary policy effects weaker, both domestically and abroad. Lastly, the bottom graph highlighting the Bank of Japan's monetary policy reveals that its influence is instant across all periods. Yet, during the COVID-19 pandemic, its impact significantly overshadows the effects seen during general economic crises. Specifically, following the pandemic's outbreak, the Bank of Japan's policy creates a reduction in China's FSI, peaking in the second period before waning. In contrast, during typical economic crises, the impact peaks immediately and fades out after twelve periods.
In conclusion, the monetary policies of major developed economies have varied spillover effects on China's FSI depending on the specific epoch in question. These insights further support the assertions made in Section 4.2.
5.4. Robustness examination of dynamic spillover indices
The recent investigations in sections 5.2 and 5.3 have thoroughly examined the enduring effects of the monetary policies of major developed economies on China's market FSI. Through this, a convergence of influences, from both quantitative monetary policies represented by monetary supply and price-based policy and signified by benchmark interest rates, on China's market has emerged.
In alignment with our methodology from section 4.4, Table 3 reveals distinct spillover indices. The Federal Reserve dominates, wielding a composite spillover index of 37.16% on other developed economies' monetary policies and China's market. This is followed by the European Central Bank at 22.12% and the Bank of Japan at 22.56%. Interestingly, China's own FSI only has a 4.67% spillover onto these developed economies' policies. This underscores the prominent influence of the U.S. due to its vast monetary size, whereas Japan, even with its smaller economic stature, still holds considerable sway. The European Central Bank's influence, however, has receded, now closely mirroring that of Japan. China, for its part, exerts negligible influence on the world's leading economies' monetary policies, suggesting that, despite its advances in internationalizing the Renminbi, China's domestic financial clout remains relatively circumscribed.
Table 3 also provides clarity on how these economies impact China's market FSI. The Federal Reserve's policy has an 8.29% spillover, the European Central Bank is at 6.48% and the Bank of Japan is at 4.71%. The inference drawn here is that a country's economic size is directly proportional to the impact its monetary policy has on China's financial arena.
The subsequent graphic representations in Figures 16 and 17 further clarify these relationships. Figure 16 depicts the multidirectional connections among the variables studied. The monetary policies of the developed economies have displayed significant spillover effects with a noticeable dip around 2008. China's FSI remains largely insular, but it's worth noting an upsurge in external influences from the three central economies post the COVID-19 outbreak.
Figure 17, meanwhile, offers insights into the give-and-take dynamics among these entities. Up until 2009, the Federal Reserve's monetary supply overshadowed that of its Japanese counterpart. Post that period, Japan took the reins. Simultaneously, the European Central Bank has consistently felt the ripples from both these major players. China's FSI, on the other hand, while historically influenced by these global monetary policies, particularly felt the heat before 2010, saw a relative lull, and then experienced a spike in response to the pandemic's onset in 2020.
Figure 18 unravels the complex web of spillover interactions among the studied variables using the DY index. From this, it's evident that China's FSI is significantly shaped by the ripple effects from dominant global economies. The Federal Reserve and the European Central Bank play particularly pivotal roles in influencing this index, while the Bank of Japan exerts a more moderate impact. Interestingly, when juxtaposed with Figure 12, we observe stark variations in the interactive spillover dynamics among these major economies. The European Central Bank, previously portrayed as a potent force in Figure 12, now appears to have diminished spillover strength when focusing on quantitative monetary policies. Instead, it is predominantly under the sway of the Federal Reserve and, to a lesser extent, the Bank of Japan. This hints at the European Central Bank's reduced autonomy in monetary policy, a stark contrast to the greater independence showcased by both the U.S. and Japan.
Conclusively, by using monetary supply as a representative measure for monetary policy, our findings on the interactive spillover dynamics between the monetary policies of these major economies and China's FSI align well with the insights of section 4.4, thereby reiterating their validity.
6.
Conclusions
This study meticulously examines the spillover effects of major developed economies' monetary policies on China's financial markets, utilizing advanced data sets and methodologies. One of the key findings is the undeniable spillover effect from the monetary policies of developed nations onto China's financial stress indices. For instance, the Federal Reserve's immediate influence on China's markets is notably strong, surpassing that of the Eurozone and Japan. However, this impact diminishes swiftly, becoming negligible in the long run. In contrast, the European Central Bank has had a consistent, albeit diminishing, influence since the adoption of negative interest rates in 2014. The Bank of Japan's influence, interestingly, exhibits a slight contrary effect in the short to medium term.
Two major channels have been pinpointed through which these spillover effects propagate. The first, described as the "monetary policy → short-term capital flows → financial markets" pathway, showcases how monetary policies from developed nations drive short-term capital inflows, thus influencing China's markets. Owing to its stable economic environment, China has become a magnet for global investments, leading to heightened activity in its capital markets. The second channel, the "monetary policy → changes in foreign exchange reserves → financial markets" route, reveals how fluctuations in foreign exchange reserves, influenced by global monetary policies, play a pivotal role in China's financial markets. When developed economies experience currency appreciation against a declining yuan, China witnesses a surge in foreign investments. These findings highlight the importance of robust oversight policies tailored to managing short-term capital inflows and foreign exchange reserves.
Over the years, a remarkable observation has been the diminished susceptibility of China's financial markets to the monetary policies of developed nations. This resilience has been notable, even though there was a transient spike in vulnerability following the onset of the COVID-19 pandemic. While many speculated that China's financial liberalization efforts would increase its vulnerability to external shocks, the empirical evidence suggests otherwise. While the general impact from developed nations' monetary policies remains consistent across various events, including economic crises or global pandemics, there was a discernible amplification in spillover effects post-COVID-19. Such findings emphasize the need for flexible and adaptive financial policies, especially to tackle challenges presented by volatile short-term capital inflows.
This research, while rooted in meticulous empirical observations, does exhibit certain limitations. First, its foundation on historical data and mathematical logic comes without the underpinning of a broad economic theory. This potentially narrows its long-term relevance. Future studies should prioritize forming universally applicable economic theories. Second, the study's decision to use benchmark interest rates of prominent central banks to anticipate the repercussions of monetary policy normalization in developed economies is rich in data. However, this might sacrifice some statistical robustness, and such an approach isn't without contention. Third, when creating China's FSI using the TVP-FAVAR model, the extended timeframe may have inadvertently excluded significant variables, thereby missing essential insights. Lastly, in determining dynamic spillover effects using the TVP-VAR-DY model, the study limits its focus to monetary policy variables from only three developed economies. Introducing more macroeconomic variables and diverse monetary policy metrics could add depth, even if it brings a risk of overlapping definitions.
Emerging economies, especially China, need to gird themselves for the potential aftershocks that might arise from the normalization of monetary policies in western-developed economies. Historically, during major crises such as the global COVID-19 pandemic, these developed economies have rolled out aggressive monetary policies, which had indirect benefits for emerging markets. However, the sustained use of such extreme measures isn't feasible. As the global economy inches towards recovery, these economies will likely enforce stringent interest rate hikes to stabilize their currencies, potentially rocking the financial stability of emerging markets like China.
Yet, China stands in a unique position. With its vast economic prowess and substantial foreign exchange reserves, it possesses both the ability and determination to maintain its monetary policy autonomy. The evidence from this study suggests that, as China has been liberalizing its financial market, it has simultaneously fortified its resilience against external financial perturbations. This strength underscores the importance of China's independent policy trajectory.
Lastly, China's macro-prudential regulations demand strengthening. The primary conduits of monetary policy spillover, notably short-term capital flows and foreign exchange reserve fluctuations, need meticulous oversight. Policies should be in place to counteract any significant capital outflows proactively. Additionally, China's vast foreign exchange reserves aren't just buffers against external shocks, but potent tools. China can strategically utilize these reserves to further its international trade goals, draw in more foreign investments and effectively navigate and neutralize international financial risks.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgements
This research is supported by the National Natural Science Foundation of China (No. 71721001 & 72371256), the Excellent Young Team Project Natural Science Foundation of Guangdong Province of China (No. 2023B1515040001), Guangdong Social Sciences Co-construction Project of the 13th Five-year Plan, China (NO. GD20XYJ05), and National Natural Science foundation for Young Scholars of China (No. 72101063).
Conflict of interest
All authors declare no conflicts of interest in this paper.










 DownLoad:
DownLoad: