Exponential asymptotic flocking in the Cucker-Smale model with distributed reaction delays

1.   Introduction

Individual-based models of collective behavior attracted the interest of researchers in several scientific disciplines. A particularly interesting aspect of the dynamics of multi-agent systems is the emergence of global self-organizing patterns, while individual agents typically interact only locally. This is observed in various types of systems - physical (e.g., spontaneous magnetization and crystal growth in classical physics), biological (e.g., flocking and swarming, ^[1,2]) or socio-economical ^[3,4]. The field of collective (swarm) intelligence also found many applications in engineering and robotics ^[5,6]. The newest developments in the mathematical approaches to the field are captured in, e.g., ^{[7,8,9,10,11,12,13,14,15,16,17]}.

The Cucker-Smale model is a prototypical model of consensus seeking, or, in physical context, velocity alignment. Introduced in ^[18,19], it has been extensively studied in many variants, where the main point of interest is the asymptotic convergence of the (generalized) velocities towards a consensus value. In this paper we focus on a variant of the Cucker-Smale model with distributed delay. We consider $N \in\mathbb{N}$ autonomous agents described by their phase-space coordinates $(x_i(t), v_i(t))\in\mathbb{R}^{2d}$, $i = 1, 2, \cdots, N$, $t\geq 0$, where $x_i(t) \in \mathbb{R}^{d}$, resp. $v_i(t) \in \mathbb{R}^{d}$, are time-dependent position, resp. velocity, vectors of the $i$-th agent, and $d\geq 1$ is the physical space dimension. The agents are subject to the following dynamics

for $i = 1, 2, \cdots, N$, where $|\cdot|$ denotes the Euclidean distance in $\mathbb{R}^d$. The parameter $\lambda > 0$ is fixed and ${P}$ is a probability measure on $[0, \infty)$. For simplicity we consider constant initial datum on $(-\infty, 0]$ for the position and velocity trajectories,

with $(x_{i}^0, v_{i}^0) \in \mathbb{R}^{d}\times \mathbb{R}^{d}$ for $i = 1, 2, \cdots, N$. The function $\psi: [0, \infty) \to (0, \infty)$ is a positive nonincreasing differentiable function that models the communication rate between two agents $i$, $j$, in dependence of their metric distance. For notational convenience, we shall denote

In our paper we shall introduce the following three assumptions on $\psi = \psi(r)$. First, we assume

which clearly does not restrict the generality due to the freedom to choose the value of the parameter $\lambda > 0$. Moreover, we assume that there exist some $\gamma < 1$ and $c, R > 0$ such that

and that there exists $\alpha > 0$ such that

The prototype rate considered by Cucker and Smale in ^[18,19] and many subsequent papers is of the form

with the exponent $\beta\geq 0$. The assumption (1.5) is verified for (1.7) if $\beta < 1/2$, while assumption (1.6) is satisfied for all $\beta\geq 0$ by choosing $\alpha: = 2\beta$. Let us point out that the results of our paper are not restricted to the particular form (1.7) of the communication rate.

In real systems of interacting agents - animals, humans or robots, the agents typically react to the information perceived from their surroundings with positive processing (or reaction) delay, which might have a significant effect on their collective behavior. The system (1.1)–(1.2) represents a model for flocking or consensus dynamics where the reaction (or information processing) delay is distributed in time according to the probability distribution ${P}$. Observe that the delay $\tau > 0$ is present in both the $v_i$ and $v_j$, as well as the $x_i$ and $x_j$ variables in the right-hand side of (1.2). In contrast, the modeling assumption that the agents receive information from their surroundings with a non-negligible delay (for instance, due to finite speed of signal propagation) would lead to delay present in the $v_j$ (and $x_j$) variables only (and not in $v_i$ and $x_i$, since these do not involve transmission of information). However, since in typical applications in biology or engineering the agents communicate through light signals, and their distances are small compared to the speed of light, one can assume the signal propagation to be practically instantaneous. Consequently, for this type of applications it is appropriate to assume reaction-type delay as represented by our system (1.1)–(1.2).

The main objective in the study of Cucker-Smale type models is their asymptotic behavior, in particular, the concept of conditional or unconditional flocking. In agreement with ^[18,19] and many subsequent papers, we say that the system exhibits flocking behavior if there is asymptotic alignment of velocities and the particle group stays uniformly bounded in time.

Definition 1. We say that the particle system (1.1)–(1.2) exhibits flocking if its solution $(x(t), v(t))$ satisfies

for all $i, j = 1, 2, \cdots, N$.

The term unconditional flocking refers to the case when flocking behavior takes place for all initial conditions, independently of the value of the parameters $\lambda > 0$ and $N\in\mathbb{N}$. The celebrated result of Cucker and Smale ^[18,19] states that the system (1.1)–(1.2) without delay (this corresponds to the formal choice ${\mathrm{d}} {P}(s): = \delta(s) {\mathrm{d}} s$, with $\delta$ the Dirac delta measure) with the communication rate (1.7) exhibits unconditional flocking if and only if $\beta < 1/2$. For $\beta \geq 1/2$ the asymptotic behavior depends on the initial configuration and the particular value of the parameters $\lambda > 0$ and $N\in\mathbb{N}$. In this case we speak about conditional flocking. The proof of Cucker and Smale (and its subsequent variants, see ^[20,21,22]) is based on a bootstrapping argument, estimating, in turn, the quadratic fluctuations of positions and velocities, and showing that the velocity fluctuations decay monotonically to zero as $t\to\infty$.

The presence of delays in (1.1)–(1.2) introduces a major analytical difficulty. In contrast to the classical Cucker-Smale system (without delay), the quadratic velocity fluctuations are, in general, not decaying in time, and oscillations may appear. In fact, oscillations are a very typical phenomenon exhibited by solutions of differential equations or systems with delay, see, e.g., ^[23]. In ^[24] we developed an analytical approach for the Cucker-Smale model with lumped delay (corresponds to the formal choice ${\mathrm{d}} {P}(s): = \delta(s-\tau) {\mathrm{d}} s$ in (1.1)–(1.2), with a fixed $\tau > 0$). It is based on the following two-step procedure: first, construction of a Lyapunov functional, which provides global boundedness of the quadratic velocity fluctuations. Second, forward-backward estimates on appropriate quantities that give exponential decay of the velocity fluctuations. The main goal of this paper is to generalize the approach to the case of distributed delays with a general probability measure $P$. A demonstration of the approach to the scalar negative feedback equation with distributed delay, which can be seen as a special case of (1.1)–(1.2) with $\psi\equiv 1$ and $N = 2$, was recently given in ^[25].

Flocking in Cucker-Smale type models with fixed lumped delay and renormalized communication weights was recently studied in ^[26,27]. Both these papers consider the case where the delay in the velocity equation for the $i$-th agent is present only in the $v_j$-terms for $j\neq i$, i.e., transmission-type delay. This allows for using convexity arguments to conclude a-priori uniform boundedness of the velocities. Such convexity arguments are not available for our system (1.1)–(1.2). In ^[28] the method is extended to the mean-field limit ($N\to\infty$) of the model. In ^[31] the authors consider heterogeneous delays both in the $x_j$ and $v_j$ terms and they prove asymptotic flocking for small delays and the communication rate (1.7). A system with time-varying delays was studied in ^[15], under the a-priori assumption that the Fiedler number (smallest positive eigenvalue) of the communication matrix $(\psi_{ij})_{i, j = 1}^N$ is uniformly bounded away from zero. The same assumption is made in ^[29] for a Cucker-Smale type system with delay and multiplicative noise. The validity of this relatively strong assumption would typically be guaranteed by making the communication rates $\psi_{ij}$ a-priori bounded away from zero, which excludes the generic choice (1.7) for $\psi$. Our approach does not require such a-priori boundedness.

Cucker-Smale systems with distributed delays were studied in ^[12] and ^[17]. In both works, the delay is present in the expression for $v_j$ only, while $v_i$ in (1.2) is evaluated at the present time $v_i(t)$. The $L^\infty$ analysis in ^[17] is based on a system of dissipative differential inequalities for the position and velocity diameters, leading to a nonexplicit "threshold on the time delay''. The work ^[17] introduces hierarchical leadership to the distributed delay system. For the case of free will ultimate leader (i.e., it can change its velocity freely), a flocking result is given under a smallness condition on the leader's acceleration. To our best knowledge, the Cucker-Smale system of the form (1.1)–(1.2), where distributed delay is present in both the $v_j$ and $v_i$ terms on the right-hand side (1.2), has not been studied before.

This paper is organized as follows. In Section 2 we formulate our assumptions and the main flocking result. In Section 3 we provide its proof divided into three steps - uniform bound on the velocities by a Lyapunov functional, forward-backward estimates, and exponential decay of the velocity fluctuation. Finally, in Section 4 we demonstrate the applicability of our theory to particular delay distributions - exponential, uniform and linear. For the exponential distribution, the flocking conditions can be resolved analytically, leading to an explicit formula. For the other two distributions, the satisfiability of the assumptions is tested numerically.

2.   Main result

Let us first introduce several relevant quantities. For $t\in{\mathbb{R}}$ we define the quadratic fluctuation of the velocities,

and the quantity

Moreover, we introduce the moments of the probability measure ${P}$. The $k$-th order moment for $k\in{\mathbb{N}}$ shall be denoted ${\mathbb{M}}_k$,

the exponential moment (or moment generating function) ${{\mathbb{M}}_\mathrm{exp}}[\kappa]$ for $\kappa\in{\mathbb{R}}$,

Finally, we shall need the moment ${\mathbb{K}}[\kappa]$, defined as

Our main result is the following:

Theorem 1. Let the communication rate $\psi = \psi(r)$ verify the assumptions (1.4)–(1.6). If there exists $\kappa > 0$ such that the conditions

and

are mutually satisfied, with $\alpha > 0$ given by (1.6), then the solution of the system (1.1)–(1.2) subject to constant initial datum (1.1) exhibits flocking behavior in the sense of Definition 1. Moreover, the quadratic velocity fluctuation $V = V(t)$ decays monotonically (i.e., non-oscillatory) and exponentially to zero as $t\to\infty$.

The above theorem deserves several comments. First, the conditions (2.4)–(2.5) relate the value of the parameter $\lambda > 0$, the moments of the probability measure ${P}$ and the fluctuation of the initial datum $V(0)$. For fixed $\lambda > 0$ and ${P}$, (2.5) represents a smallness condition on the fluctuation of the initial datum, which is a very natural requirement in the context of asymptotic flocking. On the other hand, the fact that both (2.4) and (2.5) essentially impose an upper bound on $\lambda$ seems less natural, since one would intuitively expect that increasing the coupling strength should lead to stronger tendency to flocking. This is in general true for small values of $\lambda > 0$, however, increasing its value beyond a certain threshold induces oscillations into the system, which become even unbounded for large values of $\lambda$. This phenomenon is clearly illustrated by considering the simple case $N = 2$ and $\psi\equiv 1$ with lumped delay $\tau > 0$. Then (1.2) reduces to the delay negative feedback equation $\dot u(t) = - \lambda u(t-\tau)$ for $u(t): = v_1(t)-v_2(t)$, subject to constant initial datum. It is well known that if $\lambda\tau < e^{-1}$, solutions tend to zero monotonically as $t\to\infty$. However, if $\lambda\tau$ becomes larger than $e^{-1}$ but smaller than $\pi/2$, all nontrivial solutions oscillate (i.e., change sign infinitely many times as $t\to\infty$), but the oscillations are damped and vanish as $t\to\infty$. For $\lambda\tau > \pi/2$ the nontrivial solutions oscillate with unbounded amplitude as $t\to\infty$; see Chapter 2 of ^[32] and ^[23] for details. Consequently, it is natural that (2.4)–(2.5) impose an upper bound on $\lambda$, in relation to the moments of the probability measure ${P}$, in order to obtain nonoscillatory solutions.

As we shall demonstrate in Section 4, for particular choices of the distribution ${P}$ the conditions (2.4)–(2.5) lead to systems of nonlinear inequalities in terms of the distribution parameters and the fluctuation of the initial datum. These can be sometimes resolved analytically, leading to explicit flocking conditions. This is the case for the exponential distribution, as we shall demonstrate in Section 4.1. However, even if the nonlinear inequalities turn out to be prohibitively complex to be treated analytically, they are well approachable numerically. We show this for the uniform and linear distributions in Sections 4.2 and 4.3. Let us also remark that the formal choice ${\mathrm{d}} P(s) = \delta(s) {\mathrm{d}} s$, i.e., no delay, gives ${\mathbb{K}}[\kappa] = 0$, so that (2.4) is void, while ${{\mathbb{M}}_\mathrm{exp}}[\kappa] = 1$, so that (2.5) is always satisfiable just by choosing a large enough $\kappa > 0$. Theorem 1 then reduces to the classical unconditional flocking result ^[18,19] for the original Cucker-Smale model.

We admit that the assumption about the constantness of the initial datum on $(-\infty, 0]$, or on the interval corresponding to the support of the measure $P$, can be perceived as too restrictive. In fact, the methods we present in this paper can be generalized to the case of nonconstant initial data, as we demonstrated in ^[24]. However, since this would significantly increase the technicality of our exposition, we elect to focus on the essence of the method and thus restrict ourselves to the constant initial datum.

We note that by the rescaling of time $t\mapsto \lambda t$, of the velocities $v_i \mapsto \lambda^{-1} v_i$ and of the probability measure $P$, the parameter $\lambda$ is eliminated from the system (1.1)–(1.2). Nonetheless, for the purpose of compatibility with previous literature, we shall carry out our analysis for the original form (1.1)–(1.2). The scaling invariance shall become evident in Section 4, where we shall formulate the flocking conditions in terms of properly rescaled parameters of the probability distribution $P$ and in terms of $V(0)/\lambda^2$.

Finally, we note that the symmetry of the particle interactions $\psi_{ij} = \psi_{ji}$ implies that the total momentum is conserved along the solutions of (1.2), i.e.,

Consequently, if the solution converges to an asymptotic velocity consensus, then its value is determined by the mean velocity of the initial datum.

3.   Asymptotic flocking

The proof of asymptotic flocking for the system (1.1)–(1.2) will be carried out in three steps: First, in Section 3.1 we shall derive a uniform bound on the quadratic velocity fluctuation $V = V(t)$ by constructing a suitable Lyapunov functional. Then, in Section 3.2 we prove a forward-backward estimate on the quantity $D = D(t)$ defined in (2.2), which states that $D = D(t)$ changes at most exponentially locally in time. Finally, in Section 3.3 we prove the asymptotic decay of the quadratic velocity fluctuation and boundedness of the spatial fluctuation and so conclude the proof of Theorem 1.

3.1.   Lyapunov functional and uniform bound on velocity fluctuations

We first derive an estimate on the dissipation of the quadratic velocity fluctuation in terms of the quantity $D = D(t)$ defined in (2.2).

Lemma 1. For any $\delta > 0$ we have, along the solutions of (1.1)–(1.2),

where $[t-s]^+ : = \max\{0, t-s\}$.

Proof. We have

where the last equality is due to the conservation of momentum (2.6). With (1.2) we have

For the first term of the right-hand side we apply the standard symmetrization trick (exchange of summation indices $i \leftrightarrow j$, noting the symmetry of ${\psi}_{ij} = {\psi}_{ji}$),

Therefore, we arrive at

For the last term we use the Young inequality with $\delta > 0$ and the bound $\psi\leq 1$ by assumption (1.4),

Hence,

Next, we use (1.2) to evaluate the difference $v_{i}(t)-{v}_{i}(t-s)$,

where $[t-s]^+ : = \max\{0, t-s\}$ and we used the fact that the initial datum for the velocity trajectories is constant. Taking the square in (3.3) and summing over $i$ we have

The first inequality in (3.4) is Cauchy-Schwartz for the sum term, i.e., $\left|\sum\limits_{i = 1}^N a_{i}\right|^2 \leq N\sum\limits_{i = 1}^N |a_{i}|^2$, and the second Cauchy-Schwartz inequality for the integral term, together with the bound $\psi\leq 1$ imposed by assumption (1.4). Combining (3.2) and (3.4) directly leads to (3.1).

We now define for $t > 0$ the functional

where $V = V(t)$ is the quadratic velocity fluctuation (2.1) and $D = D(t)$ is defined in (2.2). Note that ${\mathcal{L}}(0) = V(0)$.

Lemma 2. Let the parameter $\lambda > 0$ satisfy

Then along the solutions of (1.1)–(1.2) the functional (3.5) satisfies

Proof. Taking the time derivative of the second term in ${\mathcal{L}}(t)$ yields

Therefore, with the choice $\delta: = \lambda\sqrt{{\mathbb{M}}_2}$ in (3.1) we eliminate the integral term and obtain,

We observe that the right-hand side is nonpositive if (3.6) is satisfied, and therefore, ${\mathcal{L}}(t)\leq {\mathcal{L}}(0) = V(0)$ for $t\geq 0$.

Consequently, if (3.6) holds, then the velocity fluctuation $V(t) \leq {\mathcal{L}}(t)$ is uniformly bounded from above by $V(0)$ for all $t\geq 0$.

Remark 1. Having established the decay estimate (3.7), one might attempt to apply Barbalat's lemma ^[30] to prove the desired asymptotic consensus result, assuming merely the validity of (3.6). Indeed, with the uniform bound on velocities provided by Lemma 2 and the properties of the interaction rate $\psi$, one can prove that the second-order derivative $\frac{{\mathrm{d}}^2}{{\mathrm{d}} t^2} {\mathcal{L}}(t)$ is uniformly bounded in time, which implies that $\frac{{\rm{d}}}{{{\rm{d}}t}} {\mathcal{L}}(t) \to 0$ as $t\to\infty$. This in turn gives $D(t) \to 0$ as $t\to\infty$. However, since $\psi$ is not a priori bounded from below (and the uniform velocity bound allows for a linear in time expansion of the group in space), this does not imply that the velocity fluctuation $V(t)$ decays asymptotically to zero.

3.2.   Forward-backward estimates

Lemma 3. Let the communication rate $\psi = \psi(r)$ satisfy assumption (1.6) and assume that (3.6) holds. Then along the solutions of (1.1)–(1.2), the quantity $D(t)$ defined by (2.2) satisfies for any fixed ${\varepsilon} > 0$ the inequality

for all $t > 0$, with $\alpha > 0$ given in (1.6).

Proof. For better legibility of the proof, let us adopt the notational convention that all quantities marked with a tilde are evaluated at time point $t-s$, i.e., $\widetilde{v}_{i}: = v_i(t-s)$, $\widetilde{x}_{i}: = x_i(t-s)$, $\widetilde{\psi}_{ij} = \psi(|\widetilde x_{i}- \widetilde x_{j}|)$, etc. With this notation, we have

and differentiation in time and triangle inequality gives, for $t > 0$,

where $\psi_{ij}' = \psi'(|\widetilde x_{i}- \widetilde x_{j}|)$. By assumption (1.6), $|\psi'(r)|\leq \alpha \psi(r)$ for $r \geq 0$, we have for the first term of the right-hand side

where in the second inequality we used the bound

provided for $t-s > 0$ by Lemma 2. For $t-s\leq 0$ it holds trivially due to the constantness of the initial datum.

For the second term of the right-hand side of (3.9) we apply the symmetrization trick,

and estimate using the Cauchy-Schwartz inequality with some ${\varepsilon} > 0$ and the bound $\psi\leq 1$ imposed by assumption (1.4),

The first term of the right-hand side is equal to $2{\varepsilon} D(t)$, while for the second term, for $t-s > 0$, we have with (1.2), the Jensen inequality and the bound $\psi\leq 1$,

For $t-s < 0$ we have $\frac{{{\rm{d}}{{\tilde v}_i}}}{{{\rm{d}}t}} \equiv 0$ due to the constant initial datum.

Combining the above estimates in (3.9), we finally arrive at

which is (3.8).

The following lemma constitutes the core of the forward-backward estimate method and was proved in [24, Lemma 3.5]. We present it here for the sake of the reader.

Lemma 4. Let $y\in C({\mathbb{R}})$ be a nonnegative function, continuously differentiable on $(0, \infty)$ and constant on $(-\infty, 0]$. Let the differential inequality

be satisfied with some constants $C_1, C_2 > 0$.

If there exists some $\kappa > 0$ such that

then the following forward-backward estimate holds for all $t > 0$ and $s > 0$

Proof Due to the assumed continuity of $y(t)$ and $\dot y(t)$ on $(0, \infty)$, (3.11) implies that there exists $T > 0$ such that

We claim that (3.13) holds for all $t\in{\mathbb{R}}$, i.e., $T = \infty$. For contradiction, assume that $T < \infty$, then again by continuity we have

Integrating (3.13) on the time interval $(T-s, T)$ with $s > 0$ yields

Using this with (3.10) gives

Assumption (3.11) gives then

which is a contradiction to (3.14). Consequently, (3.13) holds with $T: = \infty$, and an integration on the interval $(t-s, t)$ implies (3.12).

We now apply the result of Lemma 4 to derive a backward-forward estimate on the quantity $D = D(t)$ defined in (2.2).

Lemma 5. Let (3.6) be verified and let $\kappa > 0$ be such that (2.5) holds, i.e.,

Then, along the solution of the system (1.1)–(1.2), we have for all $t > 0$ and $s > 0$,

Proof We shall combine Lemma 3 with Lemma 4 for $y: = D$, where we use formula (3.10) with

Clearly, we want to choose ${\varepsilon} > 0$ to minimize the expression $C_1+C_2 {{\mathbb{M}}_\mathrm{exp}}[\kappa]$ in (3.11), which leads to ${\varepsilon}: = \lambda\sqrt{{\mathbb{M}}_\mathrm{exp}}$ and

Therefore, condition (3.11) reads

To estimate the expression $\frac{|\dot{D}(0+)|}{D(0)}$, we apply Lemma 3 again, this time with $t: = 0$ and the optimal choice ${\varepsilon}: = \lambda$. Using the constantness of the initial datum, we have $D(s)\equiv D(0)$ for all $s < 0$, and (3.8) gives then

Since, by definition, ${{\mathbb{M}}_\mathrm{exp}}[\kappa]\geq 1$ for $\kappa > 0$, condition (3.16) reduces to (2.5), and we conclude.

3.3.   Decay of the velocity fluctuations and flocking

In order to bound $D = D(t)$ from below by the quadratic velocity fluctuation $V = V(t)$, we introduce the minimum interparticle interaction $\varphi = \varphi(t)$,

and the position diameter

We then have the following estimate:

Lemma 6. Let the parameter $\lambda > 0$ satisfy

Then along the solutions of (1.1)–(1.2) we have

Proof. Since, by assumption, $\psi = \psi(r)$ is a nonincreasing function, we have

with $d_X = d_X(t)$ defined in (3.18). Moreover, we have for all $i, j = 1, \cdots, N$,

and Lemma 2 gives

Consequently,

and integrating in time and taking the maximum over all $i, j = 1, \cdots, N$ yields

which combined with (3.20) directly implies (3.19).

We are now in position to provide a proof of Theorem 1.

Proof. Let us recall the estimate (3.1) of Lemma 1,

Moreover, note that for any $\kappa > 0$,

{{Therefore}}, if assumption (2.4) of Theorem 1 is satisfied, i.e., if there exists $\kappa > 0$ such that $2\lambda\sqrt{{\mathbb{K}}[\kappa]}\; < \; 1$, then condition (3.6) holds and we may apply the forward-backward estimate (3.15) of Lemma 5 to the integral term

Consequently, we have

Optimizing in $\delta > 0$ gives $\delta: = \lambda\sqrt{{\mathbb{K}}[\kappa]}$, so that

By the definition (3.17) of the minimal interaction $\varphi = \varphi(t)$ we have the estimate

where for the last inequality we used (3.19) and the monotonicity of $\psi$,

Now, if assumption (2.4) is verified, (3.21) implies that $V = V(t)$ is nonincreasing. Thus we have $V(t-s)\geq V(t)$ for all $s > 0$, and, consequently,

Inserting into (3.21) yields

Denoting $\omega: = -2\lambda\left[2 \lambda\sqrt{{\mathbb{K}}[\kappa]} - 1 \right] > 0$ and integrating in time, we arrive at

Consequently, if $\int^\infty \psi(s) {\mathrm{d}} s = \infty$, we have the asymptotic convergence of the velocity fluctuation to zero, $\lim_{t\to\infty} V(t) = 0$.

By assumption 1.5, namely that $\psi(r) \geq Cr^{-1+\gamma}$ for all $r > R$, we have, asymptotically for large $t > 0$,

Therefore, from (3.23),

A slight modification of the proof of Lemma 6 gives

The integral on the right-hand side is uniformly bounded, implying the uniform boundedness of the position diameter $d_X(t) \leq \bar d_X < +\infty$ for all $t > 0$, with some $\bar d_X > 0$. This in turn implies $\varphi(t) \geq \psi(d_X(t)) \geq \psi(\bar d_X)$, so that (3.21) is replaced by the sharper estimate

Thus we finally have, for all $t > 0$,

and conclude the exponential decay of the velocity fluctuations.

4.   Examples of delay distributions

In this section we demonstrate how the flocking conditions (2.4)–(2.5) of Theorem 1 are resolved for particular delay distributions - exponential, uniform on a compact interval and linear. The conditions (2.4)–(2.5) lead to systems of nonlinear inequalities in terms of the distribution parameters. For the exponential distribution they can be resolved analytically, leading to an explicit flocking condition. For the uniform and linear distributions they can be recast as nonlinear minimization problems and easily resolved numerically, using standard matlab procedures.

4.1.   Exponential distribution

We first consider the exponential distribution ${\mathrm{d}} P(s) = \mu^{-1} e^{-s/\mu} {\mathrm{d}} s$ with mean $\mu > 0$. We have for $\kappa < \mu^{-1}$,

Therefore, conditions (2.4) and (2.5) are satisfied if there exists $\kappa > 0$ such that

Due to scaling properties, it is more convenient to investigate the flocking conditions in terms of the product $\lambda\mu$ and rescale $V(0)$ by $\lambda^2$. In this form the flocking conditions read

The first condition in (4.1) is easily resolved for $\kappa\mu$,

and gives the necessary condition $\lambda\mu < (2\sqrt{2})^{-1}$. The second condition in (4.1) we reformulate as

Maximization of the right-hand side in $\kappa\mu > 0$ leads to $\kappa\mu = 1- (2\lambda\mu)^\frac23$ and

which gives the necessary condition $\lambda\mu < \frac12 \left(\frac13\right)^\frac32$ for positivity of the right-hand side. It is easily checked that for this range of $\lambda\mu$, the choice $\kappa\mu : = 1- (2\lambda\mu)^\frac23$ verifies (4.2). Therefore, we conclude that the flocking condition imposed by Theorem 1 is equivalent to the explicit formula

4.2.   Uniform distribution

Our second example is the uniform distribution on the interval $[A, B]$ with $0\leq A < B$, i.e., ${\mathrm{d}} P(s) = \frac{1}{B-A}\chi_{[A, B]}(s) \, {\mathrm{d}} s$. The relevant moments are

Due to the scaling relations, it is convenient to express the flocking conditions in terms of $a: = \lambda A$, $b: = \lambda B$ and $\bar\kappa: = \kappa\lambda^{-1}$. Condition (2.4) reads then

and condition (2.5) reads

Deciding satisfiability (in terms of $\bar\kappa > 0$) of the above conditions seems to be prohibitively complex for the analytical approach. However, the problem is well approachable numerically. For each pair $(a, b)$ the conditions (4.4)–(4.5) can be recast as a minimization problem in $\bar\kappa$, and deciding satisfiability accounts to checking if the minimum is negative. The minimization problem can be efficiently solved using the matlab procedure ${\tt fminbnd}$ if we provide lower and upper bounds on $\bar\kappa$. These can be obtained analytically. Indeed, carrying our Taylor expansion of the exponentials in (4.4) we see that

Combining this estimate with (4.4) gives a necessary condition for its satisfiability in terms of explicit (in $a$ and $b$) lower and upper bounds on $\bar\kappa$, which are roots of the corresponding quadratic polynomial. We do not print the rather lengthy algebraic expressions here; let us just mention that an immediate rough lower bound is $\bar\kappa \geq 2(a+b)$.

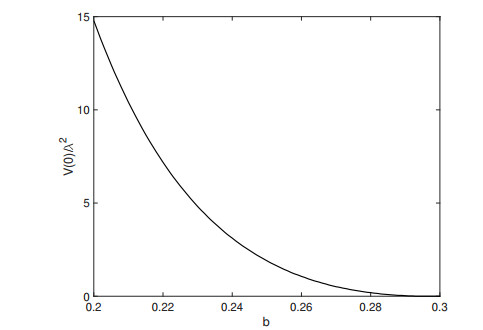
We carried out two numerical studies. First, we fixed the values of $\alpha: = 1$ and $\frac{V(0)}{\lambda^2}: = 1$ and plotted the critical value of the interval length $(b-a)$ in dependence of the value of $a > 0$, see Figure 1. We see that the flocking conditions (4.4)–(4.5) are satisfiable for $a$ at most approx. $0.16$, while for $a$ approaching zero, the interval length can go up to approx. $0.26$. In the second study, we fixed $a: = 0$ and plotted critical value of the initial fluctuation ${V(0)}/{\lambda^2}$ in dependence on the interval length $b > 0$, Figure 2.

4.3.   Linear distribution

Our third example is the linear distribution on the interval $[0, A]$ with $A > 0$, i.e., ${\mathrm{d}}{P}(s) = \frac{2}{A^2} [A-s]^+ {\mathrm{d}} s$, where $[A-s]^+ = \max\{0, A-s\}$. We have

Due to the scaling relations, it is again convenient to express the flocking conditions in terms of $a: = \lambda A$, $\bar\kappa: = \kappa\lambda^{-1}$ and $V(0)/\lambda^2$. Conditions (2.4) and (2.5) take then the form

A necessary condition for satisfiability of (2.4) is $a < \sqrt{3/2}$. We are interested in the dependence of the critical value of the rescaled initial fluctuation $V(0)/\lambda^2$ on the parameter value $a$. We approach the above satisfiability problem numerically, in two steps. First, we observe that for any fixed $a\in (0, \sqrt{3/2})$, the function

is an increasing function of $\kappa > 0$; this is easily seen carrying out the Taylor expansion of the exponentials. Moreover, $\lim_{\bar\kappa\to 0+} f_a(\bar\kappa) = 2a^2/3 < 1$. Consequently, there exists $\bar{\bar\kappa}_a > 0$ such that the first condition of (4.6) is equivalent to $\bar\kappa\in (0, \bar{\bar\kappa}_a)$. The value of $\bar{\bar\kappa}_a$ is conveniently calculable using the matlab procedure ${\tt fminsearch}$, profiting from the monotonicity of the function $f_a$. In the second step, we numerically solve the maximization problem

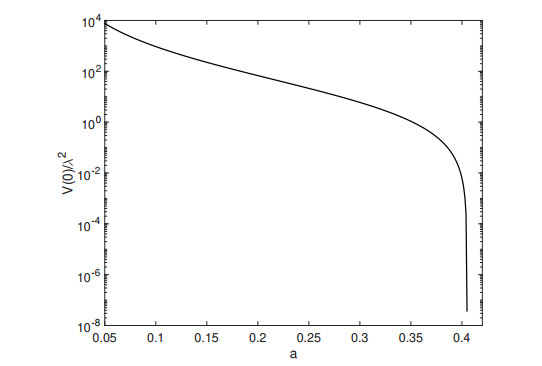
employing the matlab procedure ${\tt fminbnd}$. This gives the critical value of $V(0)/\lambda^2$ for validity of the second condition in (4.6). The outcome of this procedure for $\alpha: = 1$ is plotted in Figure (3).

5.   Conclusion

In this paper we derived sufficient conditions for asymptotic flocking in a Cucker-Smale-type system with distributed reaction delays. The conditions are formulated in terms of moments of the delay distribution and the proof of flocking relies on novel backward-forward and stability estimates on the quadratic velocity fluctuations. A significant feature of our approach is that it guarantees exponential decay of velocity fluctuations, i.e., non-oscillatory flocking regime. Moreover, the sufficient conditions are amenable to either analytic or numerical resolution, as we demonstrated for particular delay distributions (exponential, uniform and linear). An interesting and important question is how far our sufficient conditions are from being optimal. We leave this topic, best approached by systematic numerical simulations, for a future work.

Acknowledgments

JH acknowledges the support of the KAUST baseline funds. IM was funded by the project ARCHERS - Stavros Niarchos Foundation - IACM.

Conflict of interest

The authors declare no conflicts of interest in this paper.