Dynamics and implications of models for intermittent androgen suppression therapy

Tin Phan; Changhan He; Alejandro Martinez; Yang Kuang; Tin Phan; Changhan He; Alejandro Martinez; Yang Kuang

doi:10.3934/mbe.2019010

Mathematical Biosciences and Engineering

2019, Volume 16, Issue 1: 187-204. doi: 10.3934/mbe.2019010

Previous Article Next Article

Research article Special Issues

Dynamics and implications of models for intermittent androgen suppression therapy

1.
School of Mathematical and Statistical Sciences, Arizona State University, Tempe, AZ 85287, USA
2.
Department of Physics,Department of Physics, Tempe, AZ 85287, USA

Received: 11 October 2018 Accepted: 12 October 2018 Published: 11 December 2018

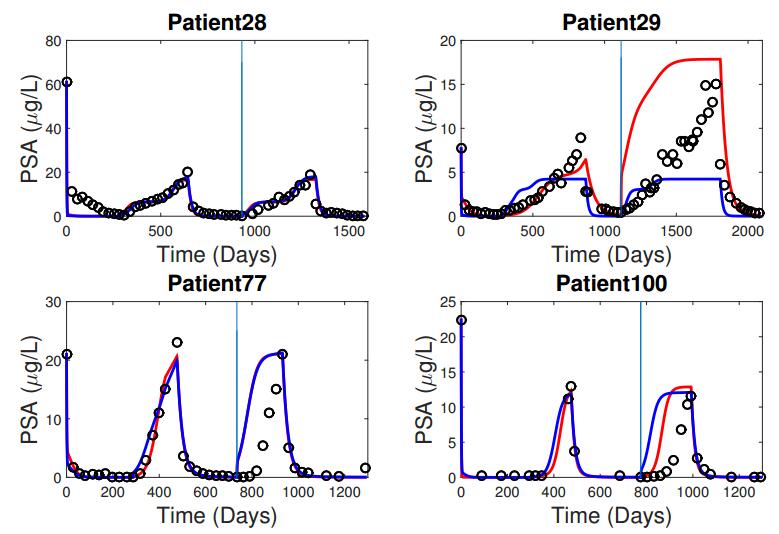
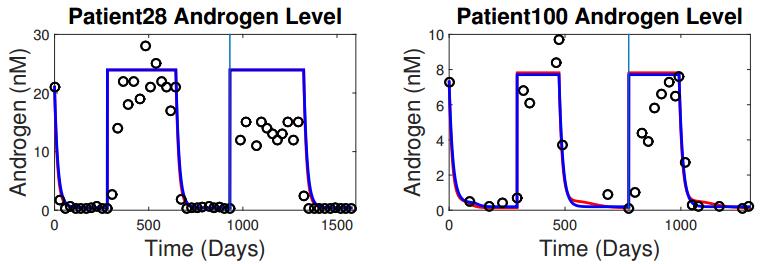
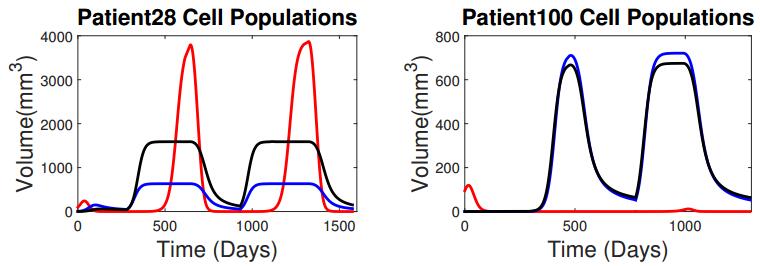
In this paper, we formulate a three cell population model of intermittent androgen suppression therapy for cancer patients to study the treatment resistance development. We compare it with other models that have different underlying cell population structure using patient prostate specific antigen (PSA) and androgen data sets. Our results show that in the absence of extensive data, a two cell population structure performs slightly better in replicating and forecasting the dynamics observed in clinical PSA data. We also observe that at least one absorbing state should be present in the cell population structure of a plausible model for it to produce treatment resistance under continuous hormonal therapy. This suggests that the heterogeneity of prostate cancer cell population can be represented by two types of cells differentiated by their level of dependence on androgen, where the two types are linked via an irreversible transformation.

Keywords:

Citation: Tin Phan, Changhan He, Alejandro Martinez, Yang Kuang. Dynamics and implications of models for intermittent androgen suppression therapy[J]. Mathematical Biosciences and Engineering, 2019, 16(1): 187-204. doi: 10.3934/mbe.2019010

Related Papers:

[1]	Leon J. van Ruiten, Thomas Hartmann . The spatial turn and the scenario approach in flood risk management—Implementing the European Floods Directive in the Netherlands. AIMS Environmental Science, 2016, 3(4): 697-713. doi: 10.3934/environsci.2016.4.697
[2]	Gene T. Señeris . Nabaoy River Watershed potential impact to flooding using Geographic Information System remote sensing. AIMS Environmental Science, 2022, 9(3): 381-402. doi: 10.3934/environsci.2022024
[3]	G Mathias Kondolf, Pilar Lopez-Llompart . National-local land-use conflicts in floodways of the Mississippi River system. AIMS Environmental Science, 2018, 5(1): 47-63. doi: 10.3934/environsci.2018.1.47
[4]	Vijendra Kumar, Kul Vaibhav Sharma, Nikunj K. Mangukiya, Deepak Kumar Tiwari, Preeti Vijay Ramkar, Upaka Rathnayake . Machine learning applications in flood forecasting and predictions, challenges, and way-out in the perspective of changing environment. AIMS Environmental Science, 2025, 12(1): 72-105. doi: 10.3934/environsci.2025004
[5]	Said Qasim, Mohammad Qasim, Rajendra Prasad Shrestha, Amir Nawaz Khan . An assessment of flood vulnerability in Khyber Pukhtunkhwa province of Pakistan. AIMS Environmental Science, 2017, 4(2): 206-216. doi: 10.3934/environsci.2017.2.206
[6]	Ronak P. Chaudhari, Shantanu R. Thorat, Darshan J. Mehta, Sahita I. Waikhom, Vipinkumar G. Yadav, Vijendra Kumar . Comparison of soft-computing techniques: Data-driven models for flood forecasting. AIMS Environmental Science, 2024, 11(5): 741-758. doi: 10.3934/environsci.2024037
[7]	RAHMOUN Ibrahim, BENMAMAR Saâdia, RABEHI Mohamed . Comparison between different Intensities of Rainfall to identify overflow points in a combined sewer system using Storm Water Management Model. AIMS Environmental Science, 2022, 9(5): 573-592. doi: 10.3934/environsci.2022034
[8]	Kitipol Nualtong, Ronnason Chinram, Piyawan Khwanmuang, Sukrit Kirtsaeng, Thammarat Panityakul . An efficiency dynamic seasonal regression forecasting technique for high variation of water level in Yom River Basin of Thailand. AIMS Environmental Science, 2021, 8(4): 283-303. doi: 10.3934/environsci.2021019
[9]	Finu Shrestha, Kabir Uddin, Sudan Bikash Maharjan, Samjwal Ratna Bajracharya . Application of remote sensing and GIS in environmental monitoring in the Hindu Kush Himalayan region. AIMS Environmental Science, 2016, 3(4): 646-662. doi: 10.3934/environsci.2016.4.646
[10]	Ignazio Mongelli, Michalis Vousdoukas, Luc Feyen, Antonio Soria, Juan-Carlos Ciscar . Long-term economic impacts of coastal floods in Europe: a probabilistic analysis. AIMS Environmental Science, 2023, 10(5): 593-608. doi: 10.3934/environsci.2023033

Abstract

1. Introduction

In 1960, Opial ^[12] established the following inequality:

Theorem A Suppose $f\in C^{1}[0, h]$ satisfies $f(0) = f(h) = 0$ and $f(x) > 0$ for all $x\in (0, h).$ Then the inequality holds

$\begin{align} \int_{0}^h\left|f(x)f'(x)\right|dx\leq\frac{h}{4}\int_{0}^{h}(f'(x))^{2}dx, \end{align}$

(1.1)

where this constant $h/4$ is best possible.

Many generalizations and extensions of Opial's inequality were established ^{[2,4,5,6,7,8,9,10,11,15,16,17,18,19]}. For an extensive survey on these inequalities, see ^[13]. Opial's inequality and its generalizations and extensions play a fundamental role in the ordinary and partial differential equations as well as difference equation ^{[2,3,4,6,7,9,10,11,17]}. In particular, Agarwal and Pang ^[3] proved the following Opial-Wirtinger's type inequalities.

Theorem B Let $\lambda\geq 1$ be a given real number, and let $p(t)$ be a nonnegative and continuous function on $[0, a]$ . Further, let $x(t)$ be an absolutely continuous function on $[0, a]$ , with $x(0) = x(a) = 0$ . Then

$\begin{align} \int_{0}^{a}p(t)|x(t)|^{\lambda}dt\leq\frac{1}{2}\int_{0}^{a}[t(a-t)]^{(\lambda-1)/2}p(t)dt\int_{0}^{a}\left|x'(t) \right|^{\lambda}dt. \end{align}$

(1.2)

The first aim of the present paper is to establish Opial-Wirtinger's type inequalities involving Katugampola conformable partial derivatives and $\alpha$ -conformable integrals (see Section 2). Our result is given in the following theorem, which is a generalization of (1.2).

Theorem 1.1 Let $\lambda\geq 1$ be a real number and $\alpha\in (0, 1]$ , and let $p(s, t)$ be a nonnegative and continuous functions on $[0, a]\times [0, b]$ . Further, let $x(s, t)$ be an absolutely continuous function and Katugampola partial derivable on $[0, a]\times [0, b]$ , with $x(s, 0) = x(0, t) = x(0, 0) = 0$ and $x(a, b) = x(a, t) = x(s, b) = 0$ . If $p > 1,$ $\frac{1}{p}+\frac{1}{q} = 1$ Then

$\int_{0}^{a}\int_{0}^{b}p(s, t)|x(s, t)|^{\lambda}d_{\alpha}sd_{\alpha}t\leq\frac{p+q}{pq} \left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\left(\int_{0}^{a}\int_{0}^{b}\Gamma_{abpq\lambda\alpha}(s, t)\cdot p(s, t)d_{\alpha}sd_{\alpha}t\right)$

$\begin{align} \times\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t, \end{align}$

(1.3)

where

$\Gamma_{abpq\lambda\alpha}(s, t) = \left\{(st)^{1/p}[(a-s)(b-t)]^{1/q}\right\}^{\alpha(\lambda-1)}.$

Remark 1.1 Let $x(s, t)$ reduce to $s(t)$ and with suitable modifications, and $p = q = 2$ and $\alpha = 1$ , (1.3) become (1.2).

Theorem C Let $\lambda\geq 1$ be a given real number, and let $p(t)$ be a nonnegative and continuous function on $[0, a]$ . Further, let $x(t)$ be an absolutely continuous function on $[0, a]$ , with $x(0) = x(a) = 0$ . Then

$\begin{align} \int_{0}^{a}p(t)|x(t)|^{\lambda}dt\leq\frac{1}{2}\left(\frac{a}{2} \right)^{\lambda-1}\left(\int_{0}^{a}p(t)dt\right)\int_{0}^{a}\left|x'(t)\right|^{\lambda}dt. \end{align}$

(1.4)

Another aim of this paper is to establish the following inequality involving Katugampola conformable partial derivatives and $\alpha$ -conformable integrals. Our result is given in the following theorem.

Theorem 1.2 Let $j = 1, 2$ and $\lambda\geq 1$ be a real number, and let $p_{j}(s, t)$ be a nonnegative and continuous functions on $[0, a]\times [0, b]$ . Further, let $x_{j}(s, t)$ be an absolutely continuous function and Katugampola partial derivable on $[0, a]\times [0, b]$ , with $x_{j}(s, 0) = x_{j}(0, t) = x_{j}(0, 0) = 0$ and $x_{j}(a, b) = x_{j}(a, t) = x_{j}(s, b) = 0$ . Then for $\alpha\in(0, 1]$

$\int_{0}^{a}\int_{0}^{b}\left(p_{1}(s, t)|x_{1}(s, t)|^{\lambda}+p_{2}(s, t)|x_{2}(s, t)|^{\lambda}\right)d_{\alpha}sd_{\alpha}t\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\leq\frac{1}{2^{\lambda}}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\Bigg[\left(\int_{0}^{a}\int_{0}^{b}(st)^{\alpha(\lambda-1)}p_{1}(s, t) d_{\alpha}sd_{\alpha}t\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)\right|^{\lambda} d_{\alpha}sd_{\alpha}t$

$\begin{align} +\left(\int_{0}^{a}\int_{0}^{b}(st)^{\alpha(\lambda-1)}p_{2}(s, t)d_{\alpha}sd_{\alpha}t\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{2})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\Bigg]. \end{align}$

(1.5)

2. Katugampola conformable partial derivatives

Here, let's recall the well-known Katugampola derivative formulation of conformable derivative of order for $\alpha\in(0, 1]$ and $t\in[0, \infty)$ , given by

$\begin{align} {\cal D}_{\alpha}(f)(t) = \lim\limits_{\varepsilon\rightarrow 0}\frac{f(te^{\varepsilon t^{-\alpha}})-f(t)}{\varepsilon}, \end{align}$

(2.1)

and

$\begin{align} {\cal D}_{\alpha}(f)(0) = \lim\limits_{t\rightarrow 0}D_{\alpha}(f)(t), \end{align}$

(2.2)

provided the limits exist. If $f$ is fully differentiable at $t$ , then

${\cal D}_{\alpha}(f)(t) = t^{1-\alpha}\frac{df}{dt}(t).$

A function $f$ is $\alpha$ -differentiable at a point $t\geq 0$ , if the limits in (2.1) and (2.2) exist and are finite. Inspired by this, we propose a new concept of $\alpha$ -conformable partial derivative. In the way of (1.4), $\alpha$ -conformable partial derivative is defined in as follows:

Definition 2.1 ^[20] ( $\alpha$ -conformable partial derivative) Let $\alpha\in (0, 1]$ and $s, t\in[0, \infty)$ . Suppose $f(s, t)$ is a continuous function and partial derivable, the $\alpha$ -conformable partial derivative at a point $s\geq 0$ , denoted by $\frac{\partial}{\partial s}(f)_{\alpha}(s, t)$ , defined by

$\begin{align} \frac{\partial}{\partial s}(f)_{\alpha}(s, t) = \lim\limits_{\varepsilon\rightarrow 0}\frac{f(se^{\varepsilon s^{-\alpha}}, t)-f(s, t)} {\varepsilon}, \end{align}$

(2.3)

provided the limits exist, and call $\alpha$ -conformable partial derivable.

Recently, Katugampola conformable partial derivative is defined in as follows:

Definition 2.2 ^[20] (Katugampola conformable partial derivatives) Let $\alpha\in (0, 1]$ and $s, t\in[0, \infty)$ . Suppose $f(s, t)$ and $\frac{\partial}{\partial s}(f)_{\alpha}(s, t)$ are continuous functions and partial derivable, the Katugampola conformable partial derivative, denoted by $\frac{\partial^{2}}{\partial s\partial t}(f)_{\alpha^{2}}(s, t)$ , defined by

$\begin{align} \frac{\partial^{2}}{\partial s\partial t}(f)_{\alpha^{2}}(s, t) = \lim\limits_{\varepsilon\rightarrow 0}\frac{\frac{\partial}{\partial s}(f)_{\alpha}(s, te^{\varepsilon t^{-\alpha}})-\frac{\partial}{\partial s}(f)_{\alpha}(s, t)}{\varepsilon}, \end{align}$

(2.4)

provided the limits exist, and call Katugampola conformable partial derivable.

Definition 2.3 ^[20] ( $\alpha$ -conformable integral) Let $\alpha\in (0, 1]$ , $0\leq a < b$ and $0\leq c < d$ . A function $f(x, y):[a, b]\times[c, d]\rightarrow {\Bbb R}$ is $\alpha$ -conformable integrable, if the integral

$\begin{align} \int_{a}^{b}\int_{c}^{d}f(x, y)d_{\alpha}xd_{\alpha}y: = \int_{a}^{b}\int_{c}^{d} (xy)^{\alpha-1}f(x, y)dxdy \end{align}$

(2.5)

exists and is finite.

3. Main results

Theorem 3.1 Let $\lambda\geq 1$ be a real number and $\alpha\in (0, 1]$ , and let $p(s, t)$ be a nonnegative and continuous functions on $[0, a]\times [0, b]$ . Further, let $x(s, t)$ be an absolutely continuous function and Katugampola partial derivable on $[0, a]\times [0, b]$ , with $x(s, 0) = x(0, t) = x(0, 0) = 0$ and $x(a, b) = x(a, t) = x(s, b) = 0$ . If $p > 1,$ $\frac{1}{p}+\frac{1}{q} = 1$ Then

$\begin{align} \times\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t, \end{align}$

(3.1)

where

$\Gamma_{abpq\lambda\alpha}(s, t) = \left\{(st)^{1/p}[(a-s)(b-t)]^{1/q}\right\}^{\alpha(\lambda-1)}.$

Proof From (2.4) and (2.5), we have

$x(s, t) = \int_{0}^{s}\int_{0}^{t}\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)d_{\alpha}sd_{\alpha}t.$

By using Hölder's inequality with indices $\lambda$ and $\lambda/(\lambda-1)$ , we have

$|x(s, t)|^{\lambda/p}\leq \left[\left(\int_{0}^{s}\int_{0}^{t}\left|\frac{\partial^{2}}{\partial s\partial t} (x)_{\alpha^{2}}(s, t)\right|d_{\alpha}sd_{\alpha}t\right)^{\lambda}\right]^{1/p}\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\begin{align} \leq\left(\frac{1}{\alpha^{2}}(st)^{\alpha}\right)^{(\lambda-1)/p}\left(\int_{0}^{s}\int_{0}^{t}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)^{1/p}. \end{align}$

(3.2)

Similarly, from

$x(s, t) = \int_{s}^{a}\int_{t}^{b}\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)d_{\alpha}sd_{\alpha}t,$

we obtain

$\begin{align} |x(s, t)|^{\lambda/q}\leq \left(\frac{1}{\alpha^{2}}[(a-s)(b-t)]^{\alpha}\right)^{(\lambda-1)/q} \left(\int_{s}^{a}\int_{t}^{b}\left|\frac{\partial^{q}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)^{1/q}. \end{align}$

(3.3)

Now a multiplication of (3.2) and (3.3), and by using the well-known Young inequality gives

$\begin{eqnarray*} |x(s, t)|^{\lambda}&\leq &\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\Gamma_{abpq\lambda\alpha}(s, t)\cdot\left(\int_{0}^{s}\int_{0}^{t} \left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)^{1/p}\\ &\times&\left(\int_{s}^{a}\int_{t}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)^{1/q}\\ &\leq &\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\Gamma_{abpq\lambda\alpha}(s, t)\cdot\Bigg(\frac{1}{p}\int_{0}^{s}\int_{0}^{t}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\\ &+&\frac{1}{q}\int_{s}^{a}\int_{t}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\Bigg) \end{eqnarray*}$

$\begin{align} \; \; \; \; \; \; \; \; \; \; = \frac{p+q}{pq}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\Gamma_{abpq\lambda\alpha}(s, t)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t, \end{align}$

(3.4)

where

$\Gamma_{abpq\lambda\alpha}(s, t) = \Big\{(st)^{1/p}[(a-s)(b-t)]^{1/q}\Big\} ^{\alpha(\lambda-1)}.$

Multiplying the both sides of (3.4) by $p(s, t)$ and $\alpha$ –conformable integrating both sides over $t$ from $0$ to $b$ first and then integrating the resulting inequality over $s$ from $0$ to $a$ , we obtain

$\int_{0}^{a}\int_{0}^{b}p(s, t)|x(s, t)|^{\lambda}d_{\alpha}sd_{\alpha}t\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\leq\frac{p+q}{pq}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\int_{0}^{a}\int_{0}^{b}\Gamma_{abpq\lambda\alpha}(s, t)\cdot p(s, t)\left(\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)d_{\alpha}sd_{\alpha}t$

$= \frac{p+q}{pq}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\left(\int_{0}^{a}\int_{0}^{b}\Gamma_{abpq\lambda\alpha}(s, t)\cdot p(s, t)d_{\alpha}sd_{\alpha}t\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t.$

This completes the proof.

Remark 3.1 Let $x(s, t)$ reduce to $s(t)$ and with suitable modifications, (3.1) becomes the following result.

$\begin{align} \int_{0}^{a}p(t)|x(t)|^{\lambda}d_{\alpha}t\leq\frac{p+q}{pq}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\cdot\int_{0}^{a} \Gamma_{apq\lambda\alpha}(t)p(t)d_{\alpha}t\int_{0}^{a}\left|{\cal D}_{\alpha}(x)(t) \right|^{\lambda}d_{\alpha}t, \end{align}$

(3.5)

where ${\cal D}_{\alpha}(x)(t)$ is Katugampola derivative (2.1) stated in the introduction, and

$\Gamma_{apq\lambda\alpha}(t) = \Big\{t^{1/p}(a-t)^{1/q}\Big\} ^{\alpha(\lambda-1)}.$

Putting $p = q = 2$ and $\alpha = 1$ in (3.5), (3.5) becomes inequality (1.2) established by Agarwal and Pang ^[3] stated in the introduction.

Taking for $\alpha = 1$ , $p = q = 2$ and $p(s, t) = {\rm constant}$ in (3.1), we have the following interesting result.

$\int_{0}^{a}\int_{0}^{b}|x(s, t)|^{\lambda}dsdt\leq \frac{1}{2}(ab)^{\lambda}\left[B\left(\frac{\lambda+1}{2}, \frac{\lambda+1}{2}\right)\right] ^{2}\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}x(s, t)\right|^{\lambda}dsdt,$

where $B$ is the Beta function.

Theorem 3.2 Let $j = 1, 2$ and $\lambda\geq 1$ be a real number, and let $p_{j}(s, t)$ be a nonnegative and continuous functions on $[0, a]\times [0, b]$ . Further, let $x_{j}(s, t)$ be an absolutely continuous function and Katugampola partial derivable on $[0, a]\times [0, b]$ , with $x_{j}(s, 0) = x_{j}(0, t) = x_{j}(0, 0) = 0$ and $x_{j}(a, b) = x_{j}(a, t) = x_{j}(s, b) = 0$ . Then for $\alpha\in(0, 1]$

(3.6)

Proof Because

$x_{1}(s, t) = \int_{0}^{s}\int_{0}^{t}\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)d_{\alpha}sd_{\alpha}t = \int_{s}^{a}\int_{t}^{b}\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)d_{\alpha}sd_{\alpha}t.$

Hence

$|x_{1}(s, t)|\leq\frac{1}{2}\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)\right|d_{\alpha}sd_{\alpha}t.$

By Hölder's inequality with indices $\lambda$ and $\lambda/(\lambda-1)$ , it follows that

$p_{1}(s, t)|x_{1}(s, t)|^{\lambda}\leq\frac{1}{2^{\lambda}}p_{1}(s, t)\left(\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t} (x_{1})_{\alpha^{2}}(s, t)\right|d_{\alpha}sd_{\alpha}t \right)^{\lambda}\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\begin{align} \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \leq\frac{1}{2^{\lambda}}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}(st)^{\alpha(\lambda-1)}p_{1}(s, t)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t, \end{align}$

(3.7)

Similarly

$\begin{align} p_{2}(s, t)|x_{2}(s, t)|^{\lambda}\leq\frac{1}{2^{\lambda}}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}(st)^{\alpha(\lambda-1)}p_{2}(s, t)\int_{0}^{a}\int_{0}^{b} \left|\frac{\partial^{2}}{\partial s\partial t}(x_{2})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t, \end{align}$

(3.8)

Taking the sum of (3.7) and (3.8) and $\alpha$ -integrating the resulting inequalities over $t$ from $0$ to $b$ first and then over $s$ from $0$ to $a$ , we obtain

$\int_{0}^{a}\int_{0}^{b}\left(p_{1}(s, t)|x_{1}(s, t)|^{\lambda}+p_{2}(s, t)|x_{2}(s, t)|^{\lambda}\right)d_{\alpha}s d_{\alpha}t\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\begin{eqnarray*} &\leq&\frac{1}{2^{\lambda}}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\Bigg\{\int_{0}^{a}\int_{0}^{b}\left((st)^{\alpha(\lambda-1)}p_{1}(s, t) \int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)d_{\alpha}sd_{\alpha}t\\ &+&\int_{0}^{a}\int_{0}^{b}\left((st)^{\alpha(\lambda-1)}p_{2}(s, t)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{2})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\right)d_{\alpha}sd_{\alpha}t\Bigg\}\\ & = &\frac{1}{2^{\lambda}}\left(\frac{1}{\alpha^{2}}\right)^{\lambda-1}\Bigg[\left(\int_{0}^{a}\int_{0}^{b}(st)^{\alpha(\lambda-1)}p_{1}(s, t) d_{\alpha}sd_{\alpha}t\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{1})_{\alpha^{2}}(s, t)\right|^{\lambda} d_{\alpha}sd_{\alpha}t\\ &+&\left(\int_{0}^{a}\int_{0}^{b}(st)^{\alpha(\lambda-1)}p_{2}(s, t)d_{\alpha}sd_{\alpha}t\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t}(x_{2})_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\Bigg]. \end{eqnarray*}$

Remark 3.2 Taking for $x_{1}(s, t) = x_{2}(s, t) = x(s, t)$ and $p_{1}(s, t) = p_{2}(s, t) = p(s, t)$ in (3.6), (3.6) changes to the following inequality.

$\int_{0}^{a}\int_{0}^{b}p(s, t)|x(s, t)|^{\lambda}d_{\alpha}sd_{\alpha}t\leq\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\begin{align} \times\left(\int_{0}^{a}\int_{0}^{b}(st)^{\alpha(\lambda-1)}p(s, t) d_{\alpha}sd_{\alpha}t\right) \int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t} (x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t. \end{align}$

(3.9)

Putting $\alpha = 1$ in (3.9), we have

$\begin{align} \int_{0}^{a}\int_{0}^{b}p(s, t)|x(s, t)|^{\lambda}dsdt\leq\frac{1}{2^{\lambda}}\left(\int_{0}^{a}\int_{0}^{b}(st)^{\lambda-1}p(s, t) dsdt\right)\int_{0}^{a}\int_{0}^{b}\left|\frac{\partial^{2}}{\partial s\partial t} x(s, t)\right|^{\lambda}dsdt. \end{align}$

(3.10)

Let $x(s, t)$ reduce to $s(t)$ and with suitable modifications, and $\lambda = 1$ , (2.10) becomes the following result.

$\begin{align} \int_{0}^{a}p(t)|x(t)|dt\leq\frac{1}{2}\left(\int_{0}^{a}p(t)dt\right)\int_{0}^{a}\left|x'(t)\right|dt. \end{align}$

(3.11)

This is just a new inequality established by Agarwal and Pang ^[4]. For $\lambda = 2$ the inequality (3.11) has appear in the work of Traple ^[14], Pachpatte ^[13] proved it for $\lambda = 2m$ ( $m\geq 1$ an integer).

Remark 3.3 Let $x_{j}(s, t)$ reduce to $x_{j}(t)$ ( $j = 1, 2$ ) and $p_{j}(s, t)$ reduce to $p_{j}(t)$ ( $j = 1, 2$ ) with suitable modifications, (3.6) becomes the following interesting result.

$\int_{0}^{a}\left(p_{1}(t)|x_{1}(t)|^{\lambda}+p_{2}(t)|x_{2}(t)|^{\lambda}\right)d_{\alpha}t\leq\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\Bigg[\left(\int_{0}^{a}t^{\alpha(\lambda-1)}p_{1}(t)d_{\alpha}t\right)\int_{0}^{a}\left|{\cal D }_{\alpha}(x'_{1})(t)\right|^{\lambda} d_{\alpha}t$

$\begin{align} \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; +\left(\int_{0}^{a}t^{\alpha(\lambda-1)}p_{2}(t)d_{\alpha}t\right)\int_{0}^{a}\left|{\cal D}_{\alpha}(x'_{2})(t)\right|^{\lambda}d_{\alpha}t\Bigg]. \end{align}$

(3.12)

Putting $\lambda = 1$ and $\alpha = 1$ in (3.12), we have the following interesting result.

$\int_{0}^{a}\left(p_{1}(t)|x_{1}(t)|+p_{2}(t)|x_{2}(t)|\right)dt\leq\frac{1}{2}\Bigg(\int_{0}^{a}p_{1}(t)dt\int_{0}^{a}\left|x'_{1}(t)\right| dt+\int_{0}^{a}p_{2}(t)dt\int_{0}^{a}\left|x'_{2}(t)\right|dt\Bigg).$

Finally, we give an example to verify the effectiveness of the new inequalities. Estimate the following double integrals:

$\int_{0}^{1}\int_{0}^{1}\Big[st(s-1)(t-1)\Big]^{\lambda}dsdt,$

where $\lambda\geq 1$ .

Let $x_{1}(s,t)=x_{2}(s,t)= x(s, t) = st(s-1)(t-1)$ , $p_{1}(s,t)=p_{2}(s,t)= p(s, t) = (st)^{1-\alpha}$ , $a = b = 1$ and $0 < \alpha\leq 1$ , and by using Theorem 3.2, we obtain

$\int_{0}^{1}\int_{0}^{1}\Big[st(s-1)(t-1)\Big]^{\lambda}dsdt\; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \; \;$

$\begin{eqnarray*} & = &\int_{0}^{1}\int_{0}^{1}p(s, t) \left|x(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\\ &\leq&\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\left(\int_{0}^{1}\int_{0}^{1}(st)^{\alpha(\lambda-1)}p(s, t) d_{\alpha}sd_{\alpha}t\right) \int_{0}^{1}\int_{0}^{1}\left|\frac{\partial^{2}}{\partial s\partial t} (x)_{\alpha^{2}}(s, t)\right|^{\lambda}d_{\alpha}sd_{\alpha}t\\ & = &\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\left(\frac{1}{\alpha(\lambda-1)+1}\right)^{2} \int_{0}^{1}\int_{0}^{1}\Big[(2s-1)(2t-1)\Big]^{\lambda}(st)^{\alpha-1}dsdt\\ & = &\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\left(\frac{1}{\alpha(\lambda-1)+1}\right)^{2} \left(\frac{1}{2^{\alpha-1}}\int_{-1}^{1}t^{\lambda}\frac{1}{(t+1)^{1-\alpha}}dt\right)^{2}\\ &\leq&\frac{1}{2^{\lambda}}\left(\frac{1} {\alpha^{2}}\right)^{\lambda-1}\left(\frac{1}{\alpha(\lambda-1)+1}\right)^{2} \left(\frac{1}{2^{\alpha-1}}\frac{2^{\alpha}}{\alpha}\right)^{2}\\ & = &\frac{2^{2-\lambda}}{\alpha^{2\lambda}\left(\alpha(\lambda-1)+1\right)^{2}}. \end{eqnarray*}$

4. Conclusions

We have introduced a general version of Opial-Wirtinger's type integral inequality for the Katugampola partial derivatives. The established results are generalization of some existing Opial type integral inequalities in the previous published studies. For further investigations we propose to consider the Opial-Wirtinger's type inequalities for other partial derivatives.

Acknowledgments

I would like to thank that research is supported by National Natural Science Foundation of China(11471334, 10971205).

Conflict of interest

The author declares no conflicts of interest.

References

[1]	Prostate cancer, Am. Cancer Soc., 4–18, 28–29, 56–57.0
[2]	J. Baez and Y. Kuang, Mathematical models of androgen resistance in prostate cancer patients under intermittent androgen suppression therapy, Appl. Sci., 6 (2016), 352.
[3]	N. Bruchovsky, L. Klotz, J. Crook, S. Malone, C. Ludgate, W. J. Morris, M. E. Gleave and S. L. Goldenberg, Final results of the canadian prospective phase ii trial of intermittent androgen suppression for men in biochemical recurrence after radiotherapy for locally advanced prostate cancer, Cancer, 107 (2006), 389–395.
[4]	N. Buchan and S. Goldenberg, Intermittent versus continuous androgen suppression therapy: do we have consensus yet?, Cur. Oncol., 17 (2010), S45.
[5]	L. M. Cook, A. Araujo, J. M. Pow-Sang, M. M. Budzevich, D. Basanta and C. C. Lynch, Predictive computational modeling to define effective treatment strategies for bone metastatic prostate cancer, Sci. Rep., 6 (2016), 29384.
[6]	M. Droop, Some thoughts on nutrient limitation in algae, J. Phycol., 9 (1973), 264–272.
[7]	S. E. Eikenberry, J. D. Nagy and Y. Kuang, The evolutionary impact of androgen levels on prostate cancer in a multi-scale mathematical model, Biol. Direct, 5 (2010), 24.
[8]	M. C. Eisenberg and H. V. Jain, A confidence building exercise in data and identifiability: Modeling cancer chemotherapy as a case study, J. Theor. Biol., 431 (2017), 63–78.
[9]	M. Elishmereni, Y. Kheifetz, I. Shukrun, G. H. Bevan, D. Nandy, K. M. McKenzie, M. Kohli and Z. Agur, Predicting time to castration resistance in hormone sensitive prostate cancer by a personalization algorithm based on a mechanistic model integrating patient data, The Prostate, 76 (2016), 48–57.
[10]	R. Everett, A. Packer and Y. Kuang, Can mathematical models predict the outcomes of prostate cancer patients undergoing intermittent androgen deprivation therapy?, Biophys. Rev. Letters, 9 (2014), 173–191.
[11]	B. J. Feldman and D. Feldman, The development of androgen-independent prostate cancer, Nat. Rev. Cancer, 1 (2001), 34–45.
[12]	J. L. Fleck and C. G. Cassandras, Optimal design of personalized prostate cancer therapy using infinitesimal perturbation analysis, Nonlin. Analysis Hybrid System., 25 (2017), 246–262.
[13]	Q. Guo, Y. Tao and K. Aihara, Mathematical modeling of prostate tumor growth under intermittent androgen suppression with partial differential equations, lnt. J. Bifurcat. Chaos, 18 (2008), 3789– 3797.
[14]	T. Hatano, Y. Hirata, H. Suzuki and K. Aihara, Comparison between mathematical models of intermittent androgen suppression for prostate cancer, J. Theor. Biol., 366 (2015), 33–45.
[15]	Y. Hirata, N. Bruchovsky and K. Aihara, Development of a mathematical model that predicts the outcome of hormone therapy for prostate cancer, J. Theor. Biol., 264 (2010), 517–527.
[16]	Y. Hirata, K. Morino, K. Akakura, C. S. Higano and K. Aihara, Personalizing androgen suppression for prostate cancer using mathematical modeling, Sci. Rep., 8 (2018), 2673.
[17]	A. M. Ideta, G. Tanaka, T. Takeuchi and K. Aihara, A mathematical model of intermittent androgen suppression for prostate cancer, J. Nonlin. Sci., 18 (2008), 593–614.
[18]	T. L. Jackson, A mathematical model of prostate tumor growth and androgen-independent relapse, Discrete Cont. Dyn. B, 4 (2004), 187–202.
[19]	Y. Kuang, Basic properties of mathematical population models, J. Biomath., 17 (2002), 129–142.
[20]	Y. Kuang, J. D. Nagy and S. E. Eikenberry, Introduction to mathematical oncology, vol. 59, CRC Press, 2016.
[21]	J. D. Morken, A. Packer, R. A. Everett, J. D. Nagy and Y. Kuang, Mechanisms of resistance to intermittent androgen deprivation in patients with prostate cancer identified by a novel computational method, Cancer Res., 74 (2014), 3673–3683.
[22]	T. Portz, Y. Kuang and J. D. Nagy, A clinical data validated mathematical model of prostate cancer growth under intermittent androgen suppression therapy, Aip. Advances, 2 (2012), 011002.
[23]	A. Saltelli, K. Chan and E. M. Scott, Sensitivity analysis, Wiley New York, 2000.
[24]	T. Shimada and K. Aihara, A nonlinear model with competition between prostate tumor cells and its application to intermittent androgen suppression therapy of prostate cancer, Math. Biosci., 214 (2008), 134–139.
[25]	R. L. Siegel, K. D. Miller and A. Jemal, Cancer statistics, 2016, CA Cancer J. Clinic., 66 (2016), 7–30.
[26]	H. Vardhan Jain and A. Friedman, Modeling prostate cancer response to continuous versus intermittent androgen ablation therapy, Discrete Cont. Dyn. B, 18.
[27]	A. Wolf, R. C. Wender, R. B. Etzioni, I. M. Thompson, A. V. D'Amico, R. J. Volk, D. D. Brooks, C. Dash, I. Guessous, K. Andrews et al., American cancer society guideline for the early detection of prostate cancer: update 2010, CA Cancer J. Clinic., 60 (2010), 70–98.
[28]	J. Yang, T.J. Zhao, C.Q. Yuan, J.H. Xie and F.F. Hao, A nonlinear competitive model of the prostate tumor growth under intermittent androgen suppression, J. Theor. Biol., 404 (2016), 66– 72.

This article has been cited by:

1.	Jana Cox, Marcel van der Perk, Tatjana Edler, Hans Middelkoop, The effects of changing channel retention: Unravelling the mechanisms behind the spatial and temporal trends of suspended sediment in the Rhine basin, 2024, 0197-9337, 10.1002/esp.5929
2.	Daniele Fabrizio Bignami, Christian Ambrosi, Manuel Bertulessi, Giovanni Menduni, Maurizio Pozzoni, Federica Zambrini, Governance Strategies and Tools Towards the Improvement of Emergency Management of Natural Disasters in Transboundary Areas, 2024, 22124209, 104704, 10.1016/j.ijdrr.2024.104704

Reader Comments

Your name:*

Email:*
© 2019 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)