Non-singular solutions of $p$-Laplace problems, allowing multiple changes of sign in the nonlinearity

We consider positive solutions of

where $\varphi (t) = t|t|^{p-2}$, $p > 1$, so that $\varphi' (t) = (p-1)|t|^{p-2}$. The linearized problem is

Recall that any positive solution of (1) is an even function $u(-x) = u(x)$, satisfying $xu'(x) < 0$ for $x \ne 0$ so that $\max _{(-1, 1)} u(x) = u(0)$, and that any non-trivial solution of (2) is of one sign, so that we may assume that $w(x) > 0$ for $x \in (-1, 1)$, see e.g., P. Korman ^[5], ^[6].

If $f'(u) > (p-1)\frac{f(u)}{u} > 0$ for $u > 0$, it is well known that any positive solution of (1) is non-singular, i.e., the problem (2) admits only the trivial solution $w(x) \equiv 0$. Now suppose that $f'(u) > (p-1)\frac{f(u)}{u} > 0$ holds only for $u > \gamma$, for some $\gamma > 0$. It turns out that positive solutions of (1), with maximum value greater than $\gamma$ are still non-singular, provided that $\int _u^{\gamma} f(t) \, dt < 0$ for all $u \in (0, \gamma)$. The main result is stated next. It is customary to denote $F(u) = \int_0^u f(t) \, dt$.

Theorem 1. Assume that $f(u) \in C^1(\bar R_+)$, and for some $\gamma > 0$ it satisfies

Then any positive solution of (1), satisfying

is non-singular, which means that the linearized problem (2) admits only the trivial solution.

In case $p = 2$ this result was proved in P. Korman ^[7], while for general $p > 1$ a weaker result, requiring that $f(u) < 0$ on $(0, \gamma)$, was given in J. Cheng ^[3] (and before that by R. Schaaf ^[10] for $p = 2$ case), see also P. Korman ^[5], ^[6] for a different proof, and a more detailed description of the solution curve. Other multiplicity results on $p$-Laplace equations include ^[1], ^[2], ^[4] and ^[9].

Proof: $\; \;$ Assume, on the contrary, that the problem (2) admits a non-trivial solution $w(x) > 0$. Let $x_0 \in (0, 1)$ denote the point where $u(x_0) = \gamma$. Define

We claim that

Rewrite (using that $(p-1) \varphi(t) = t\varphi'(t)$)

Since $\varphi'(t) > 0$ for all $t \ne 0$, it suffices to show that the function $z(x) \equiv (1-x)u'(x)+u(x) < 0$ satisfies $z(x_0) < 0$. Indeed,

which implies the desired inequality (7), provided we can prove that

The "energy" function $E(x) = \frac{p-1}{p} {|u'(x)|}^p+F(u(x))$ is seen by differentiation to be a constant, so that $E(x) = E(x_0)$, or

By the assumption (5), it follows that

justifying (8), and then giving (7).

Next, we claim that

which implies, in particular, that

Indeed, by a direct computation, using (1) and (2),

The quantity on the right is positive on $(0, x_0)$, in view of our condition (4). Integration over $(0, x_0)$, gives (9).

We have for all $x \in [-1, 1]$

as follows by differentiation, and using the assumption $u'(1) < 0$. Hence

Since $f(u(x_0)) = 0$, it follows from Eq (1) that $u''(x_0) = 0$. Then (11) implies

We need the following function, motivated by M. Tang ^[11] (which was introduced in P. Korman ^[5], and used in Y. An et al. ^[2])

One verifies that

Integrating (14) over $(x_0, 1)$, and using (5) and (12), obtain

which implies that

On the other hand, using (13), then (9), followed by (10) and (7), we estimate the same quantity as follows

a contradiction.

We remark that in case $f(0) < 0$ it is possible to have a singular positive solution with $u'(1) = 0$, so that the assumption $u'(1) < 0$ is necessary.

We now consider the problem (where $\varphi (t) = t|t|^{p-2}$, $p > 1$)

depending on a positive parameter $\lambda$. The following result follows the same way as the Theorem $3.1$ in ^[5].

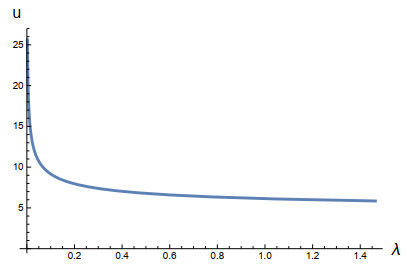
Theorem 2. Assume that $f(u) \in C^1(\bar R_+)$, and the conditions (3), (4) and (5) hold. Then there exists $0 < \lambda _0 \leq \infty$ so that the problem (15) has a unique positive solution for $0 < \lambda < \lambda _0$. All positive solutions, satisfying $u(0) > \gamma$, lie on a continuous solution curve that is decreasing in the $(\lambda, u(0))$ plane (see Figure 1). In case $f(0) < 0$, one has $\lambda _0 < \infty$, and at $\lambda = \lambda _0$ a positive solution with $u'(\pm 1) = 0$ exists, and no positive solutions exist for $\lambda > \lambda _0$. In case $f(0) = 0$ and $f'(0) < 0$, we have $\lambda _0 = \infty$.

Example $\; \;$ In Figure 1 we present the solution curve of the problem (15) in case $p = 3$ and $f(u) = u (u - 1) (u - 2) (u - 4)$. Here $\gamma = 4$, and one verifies that the Theorem 2 applies. The Mathematica program to perform numerical computations for this problem is explained in detail in ^[8] (it uses the shoot-and-scale method). The solution curve in Figure 1 exhausts the set of all positive solutions (since $\int _0^2 f(u) \, du < 0$, there are no solutions with $u(0) = \max _{(-1, 1)} u(x) \in (1, 2)$).

Conflict of interest

The author declares there is no conflicts of interest.