The effect of feedstock densification on the process and product properties of sugarcane leaves pyrolysis

1.   Introduction

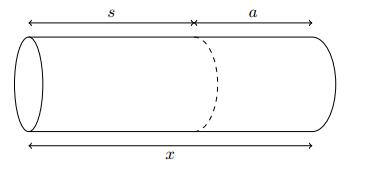
In structured population dynamics, finding the structuring variable(s) which best describes a phenomenon is a crucial question. For a population of proliferating cells or bacteria the variables usually considered are age, size (see [27,12,18]) or a combination of both (see [1,24,10] for modeling and [26,12,10,6] for mathematical analysis). Recent experimental work highlighted the limits of these models to describe bacteria, and a new variable to trigger division emerged: the size-increment, namely the size gained since the birth of the cell (see [22] and references therein for a review of the genesis of the related model). This so called 'adder principle ensures homeostasis with no feedback from the bacteria and explains many experimental data. In this model, bacteria are described by two parameters: their size-increment and their size, respectively denoted by $a$ and $x$ in the following (the choice of letter $a$ is reminiscent from the age variable, since as for the age, the size increment is reset to zero after division). This choice of variables is motivated by the main assumption of the model, which is that the control of the cellular reproduction is provided by the division rate $B$ which is supposed to depend only on $a,$ and the growth rate $g$ which is assumed to depend only on $x.$ With the variables we introduced, the model formulated in [25] reads

The function $n(t,a,x)$ represents the number of cells at time $t$ of size $x$ that have grown of an increment $a$ since their birth. The boundary term denotes an equal mitosis, meaning that after division, a mother cell gives birth to two daughters of equal size. However, if this special case of equal mitosis is appropriate to describe the division of some bacterium (e.g. E. Coli), it is inadequate for asymetric division (like yeast for instance) or for a fragmentation involving more than two daughters (as in the original model formulated for plant growth in [10]). In the current paper, we propose to consider more general division kernels. We assume that when a cell of size $x$ divides, it gives birth to a daughter of size $zx$ with a certain probability which depends on $z\in (0,1)$ but is independent of $x.$ Such fragmentation process is usually called self-similar. More precisely the number of daughters with a size between $zx$ and $(z+\! \, {\rm{d}} z)x$ is given by $\mu([z,z+\! \, {\rm{d}} z]),$ where $\mu$ is a positive measure on $[0,1].$ The model we consider is then formulated as

It appears that this model is a particular case of the one proposed in the pioneer work [10] for plants growing in a single dimension, mixing age and size control. Indeed, in this paper the authors noticed that in the case of a deterministic and positive growth rate, a size/age model is equivalent to a size/birth-size through the relation $a = x-s,$ where $s$ denotes the birth-size (see Figure 1). They preferred working with the size/birth-size description since in this framework the transport term acts only in the $x$ direction. In the case when $g$ is independent of $x$ and $B$ is bounded from above and below by positive constants, it is proved in [26] for $\mu$ a uniform measure on $[0,1],$ and in [12,Chapter V] for the equal mitosis, that the solutions to the system (1) converge to a stable distribution as time goes to infinity. In the present paper we propose to study the model (1) in the case of a linear growth rate (see [1] for a discussion on this hypothesis). More precisely we are interested in populations which evolve with a stable size and size-increment distribution, i.e. solutions of the form $n(t,a,x) = h(t)N(a,x).$ The existence of such separable solutions when $g$ is linear was already the topic of [10], but their proof required the equation to be set on a bounded domain and they had to impose a priori the existence of a maximal size for the population. In our case no maximal size is prescribed and it brings additional difficulties due to a lack of compactness. To address this problem, we will make the following assumptions.

First, we want the sum of the daughters sizes to be equal to the size of the mother. This rule, called mass conservation, prescribes

We also assume that the division does not produce any arbitrarily small daughter by imposing that the support of $\mu$ is a compact subset of $(0,1),$ which ensures that

In particular, these assumptions imply that the mean number of daughters $\mu([0,1])$ is finite. The division rate $B$ is assumed to be a nonnegative and locally integrable function on $\mathbb{R} _+$ such that

see [8] for instance. It will be useful in our study to define the associated survivor function $\Psi$ by

For a given increment $a$, $\Psi(a)$ represents the probability that a cell did not divide before having grown of at least $a$ since its birth. We assume that the function $B$ is chosen in such a way that $\Psi$ tends to zero at infinity, meaning that all the cells divide at some time. More precisely we make the following quantitative assumption

This assumption on the decay at infinity of the survivor function enables a wide variety of division rates. For instance, it is satisfied if there exists $A>0$ such that

The function $B$ being locally integrable, the function $\Psi$ belongs to $W_{loc}^{1,1}( \mathbb{R} _+)$ and (5) ensures that its derivative belongs to ${\rm{L}} ^1( \mathbb{R} _+).$ We can introduce the useful function $\Phi$ defined by

which is the probability distribution that a cell divides at increment $a.$ Recall that, as in [10], we consider the special case of a linear growth rate, namely $g(x) = x.$ In this case, multiplying by the size $x$ and integrating, we obtain $\frac{\! \, {\rm{d}} }{\! \, {\rm{d}} t} \iint xn(t,a,x) \, {\rm{d}} a \, {\rm{d}} x = \iint xn(t,a,x) \, {\rm{d}} a \, {\rm{d}} x,$ and so

This implies that if we look for a solution with separated variables $n(t,a,x) = h(t)N(a,x)$, necessarily $h(t) = h(0) {\rm{e}} ^t$. In other words, the Malthus parameter of the population is $1$. This motivates the Perron problem which consists in finding $N = N(a,x),$ which is solution to the system

It is convenient to define the set $\Omega_0 : = \{(a,x)\in \mathbb{R} ^2,\ 0\leq a \leq x \},$ and we are now ready to state the main result of the paper.

Theorem 1.1. Let $\mu$ be a positive measure on $[0,1]$ satisfying (2) and (3), and $B$ be a nonnegative and locally integrable function on $\mathbb{R} _+$ satisfying (4) such that the associated survivor function $\Psi$ satisfies (5). Then, there exists a unique solution $N \in {\rm{L}} ^1(X,(1+(x-a)^2) \, {\rm{d}} a \, {\rm{d}} x)$ to the eigenproblem (8). This solution is expressed as

where $f$ is a nonnegative function which satisfies

for all $l< k_0$, $k_0$ being the positive number given in hypothesis (5), and

with $b_\theta = \frac{\theta}{1-\theta}b,$ where $\theta$ and $b$ are defined in (3) and (4) respectively.

The fast decay of the function $f$ near zero is a consequence of the form of the support of the fragmentation kernel $\mu.$ Furthermore, this decay is consistent with the decay near zero of the eigenvector for the size equation (see [8]). Remark that for any nonnegative and appropriately normalized function $f\in {\rm{L}} ^1( \mathbb{R} _+),$ the expression given in (9) satisfies (8a), (8c), and (8d). The proof of Theorem 1.1 consists in finding the appropriate function $f$ such that (8b) is also satisfied. This function is obtained as the fixed point of a conservative operator, and this allows us to compute it numerically by using the power iteration (see [20]). We obtain the function on the left on Figure 2. On the right is the related density $N(a,x).$

Notice also that for the function $N$ given by (9), the function $s \mapsto N(a+s,x+s)$ is continuous for any $a\leq x$. It corresponds to the trajectories along the characteristics.

The article is organised as follows. In Section 2 we reduce the Perron eigenvalue problem with two variables to a fixed point problem for an integral operator in dimension one. Section 3 is dedicated to proving the existence and uniqueness of the fixed point by using functional analysis and Laplace transform methods. In Section 4 we go through the usefulness of knowing $N$ to develop entropy methods. Finally in Section 5 we discuss some interesting perspectives.

2.   Transformation into an integral equation

Our study consists in constructing a solution to the eigenproblem (8) from the solution of a fixed point problem. First, we notice that the size $x$ of a cell and its size increment $a$ grow at the same speed $g(x)$, so the quantity $x-a$ remains constant: it corresponds to the birth-size of the cell, denoted by $s$. To simplify the equation and obtain horizontal straight lines as characteristics (see Figure 3), we give a description of the population with size increment $a$ and birth-size $s$, namely we set

Thanks to this relation, it is equivalent to prove the existence of an eigenvector for the increment-size system or for the increment/birth-size system. To determine the equation verified by $M$, we compute the partial derivatives of $xN(a,x) = (a+s)M(a,s)$, which leads to the equation

Writing the non-local boundary condition (8b) with the new variables takes less calculation and more interpretation. In (8b) the number of cells born at size $s$ resulted of the division of cells at size $\frac{s}{z}.$ Then the equivalent of (8b) in the new variables with a linear growth rate is given by

since there is no mass for $a\geq \frac{s}{z}.$ With the relation (10), it is equivalent to solve (8) and to solve

Considering the variable $s$ as a parameter in (11a), we see this equation as an ODE in the variable $a.$ A formal solution is given by

Having this expression in mind, we note that for any nonnegative function $f\in {\rm{L}} ^1( \mathbb{R} _+, \, {\rm{d}} s)$, the function $M_f$ defined on $\mathbb{R} _+^2$ by

is a solution of (11a) and satisfies (11c). Then it remains to choose the appropriate function $f$ and normalize the related function $M_f$ to solve the whole system (11). It turns out that this appropriate function $f$ is a fixed point of the operator $T : {\rm{L}} ^1( \mathbb{R} _+) \to {\rm{L}} ^1( \mathbb{R} _+)$ defined by

where $\Phi = B\Psi,$ as stated in the following lemma.

Lemma 2.1. The function $M_f$ satisfies (11b) if and only if $f$ is a fixed point of the operator $T$.

Proof.

The operator $T$ can be seen as some kind of transition operator: it links the laws of birth size of two successive generations. If $f$ is the law of the parents, then $Tf$ is the law of the birth size of the newborn cells. Indeed, Equation (12) can be understood in words as 'the number of cells born at size $s$ come from the ones that were born at size $a\in[b_\theta,\frac{s}{z}]$ and elongated of $\frac{s}{z}-a,$ for all $z\in [\theta,\eta]$ and all $a,$ before dividing into new cells'. See [7] for a probabilistic viewpoint on the conservative size equation. It is easy to check that $T$ is a continuous linear operator on ${\rm{L}} ^1( \mathbb{R} _+)$ and that $\|T\|_{\mathcal{L}( {\rm{L}} ^1( \mathbb{R} _+))}\leq \|\Phi\|_{ {\rm{L}} ^1( \mathbb{R} _+)} = 1$ using (2) and (6). The following lemma provides a slightly stronger result.

Lemma 2.2. For all $l\leq 0$, the operator $T$ maps continuously ${\rm{L}} ^1( \mathbb{R} _+,s^l \, {\rm{d}} s)$ into itself. Additionally, if (5) holds true, then $T$ maps continuously ${\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)$ into itself for any $l\leq0$ and $k\in[0,k_0).$

Proof. We start with ${\rm{L}} ^1( \mathbb{R} _+,s^l \, {\rm{d}} s)$ where $l\leq0.$ For $f\in {\rm{L}} ^1( \mathbb{R} _+,s^l \, {\rm{d}} s)$ and $\beta>\alpha>0$ one has

which gives the conclusion by passing to the limits $\alpha\to0$ and $\beta\to+\infty.$

For the second part we begin with the proof that under condition (5), for any $k\in[0,k_0)$ one has

First, recall that $\int_0^\infty \Phi(a) \, {\rm{d}} a = 1$ and $\Phi = -\Psi'$. Integrating by parts for $\beta\geq 1$, one has

and the last integral converges when $\beta\to+\infty$ under Assumption (5) because $k<k_0.$ Now let $l\leq0$ and $k\in[0,k_0),$ and let $f\in {\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)$. Due to the first part of the proof, we only have to estimate $\int_0^\beta |Tf(s)|s^k \, {\rm{d}} s$ for $\beta>0.$ Since the function $x\mapsto \frac{(1+x)^k}{1+x^k}$ is uniformly bounded on $\mathbb{R} _+,$ there exists of a constant $C>0$ such that $(a+\sigma)^k\leq C(a^k+\sigma^k)$ for all $a,\sigma\geq0,$ and it allows us to write for any $\beta>0$

3.   The fixed point problem

In this section we prove the existence of a unique nonnegative and normalized fixed point of the operator $T.$

Let us first recall some definitions from the Banach lattices theory (for more details, see [9,23]). Let $\Omega$ be a subset of $\mathbb{R} _+$ and $\nu$ be a positive measure on $\Omega.$ The space ${\rm{L}} ^1(\Omega,\nu)$ is an ordered set with the partial order defined by

Furthermore, endowed with its standard norm, the space ${\rm{L}} ^1(\Omega,\nu)$ is a Banach lattice, i.e. a real Banach space endowed with an ordering $\geq$ compatible with the vector structure such that, if $f, g \in {\rm{L}} ^1(\Omega,\nu)$ and $|f|\geq |g|,$ then $\|f\|_{ {\rm{L}} ^1(\Omega,\nu)} \geq \|g\|_{ {\rm{L}} ^1(\Omega,\nu)}.$ A vector subspace $I\subset {\rm{L}} ^1(\Omega,\nu)$ is called an ideal if $f\in I, g\in {\rm{L}} ^1(\Omega,\nu)$ and $|g|\leq |f|$ implies $g\in I.$ For a given operator $A$ defined on ${\rm{L}} ^1(\Omega,\nu)$, a closed ideal $I$ is $A$-invariant if $A(I)\subset I,$ and $A$ is irreducible if the only $A$-invariant ideals are $\{0\}$ and ${\rm{L}} ^1(\Omega,\nu)$. To each closed ideal $I$ in the Banach lattice ${\rm{L}} ^1(\Omega,\nu)$ corresponds a subset $\omega \subset \Omega$ such that $I = \{f\in {\rm{L}} ^1(\Omega,\nu), {\rm{supp}} \, f \subset \omega\}$. We also define the positive cone ${\rm{L}} ^1_+(\Omega,\nu): = \{f\in {\rm{L}} ^1(\Omega,\nu)\ |\ f\geq 0\ \nu\text{-a.e. on }\Omega\}$. An operator $A: {\rm{L}} ^1(\Omega,\nu)\rightarrow {\rm{L}} ^1(\Omega,\nu)$ is said to be positive if $A( {\rm{L}} ^1_+(\Omega,\nu))\subset {\rm{L}} ^1_+(\Omega,\nu).$

To prove the existence of an eigenvector associated to the eigenvalue $1$, we will use the following theorem, easily deduced from Krein-Rutman's theorem (see [9] for instance) and De Pagter's [5].

Theorem 3.1. Let $A : {\rm{L}} ^1(\Omega,\nu)\rightarrow {\rm{L}} ^1(\Omega,\nu)$ be a non-zero positive compact irreducible operator. Then its spectral radius $\rho(A)$ is a nonnegative eigenvalue associated to a nonzero eigenvector belonging to the positive cone ${\rm{L}} ^1_+(\Omega,\nu).$

Due to a lack of compactness of the operator $T$, which is due to the lack of compactness of $\mathbb{R} _+$, we truncate the operator $T$ into a family of operators $(T_\Sigma)_\Sigma$. Let $b_\theta = \frac{\theta}{1-\theta}b$ and for $\Sigma>b_\theta$ define the operator $T_\Sigma$ on ${\rm{L}} ^1((b_\theta,\Sigma))$ by

Defining the lower bound of the domain as $b_\theta$ will ensure the irreducibility of $T_\Sigma.$ We will apply Theorem 3.1 to the operator $T_\Sigma$ for $\Sigma$ large enough to prove the existence of a pair $(\rho_\Sigma,f_\Sigma)$ such that $T_\Sigma f_\Sigma = \rho_\Sigma f_\Sigma.$ Then, we will prove that there exists a unique $f$ in a suitable space such that $\rho_\Sigma \to 1$ and $f_\Sigma \to f$ as $\Sigma \to \infty,$ with $f$ satisfying $Tf = f.$

The following lemma ensures that the truncated operator $T_\Sigma$ is well defined.

Lemma 3.2. If $f \in {\rm{L}} ^1( \mathbb{R} _+)$ and ${\rm{supp}} \, f \subset [b_\theta,\Sigma]$, then

Lemma 3.2 is a straightforward consequence of the definition of operator $T_\Sigma$ by (13). From Lemmas 2.2 and 3.2, we deduce that $T_\Sigma$ has the same stability mapping properties as $T.$ To prove the compactness of the operator $T_\Sigma$ for a fixed $\Sigma$ and later on that the family $(T_\Sigma)_\Sigma$ is also compact, we use a particular case of a corollary of the Riesz-Fréchet-Kolmogorov theorem. First, we define two properties for a bounded subset $\mathcal{F}$ of ${\rm{L}} ^1(\Omega,\nu)$ with $\Omega$ an open subset of $\mathbb{R} _+,$ and $\nu$ a positive measure, the first on translations and the second on the absence of mass on the boundary of the domain

where $\!^c\Omega$ is understood as the complement of this set in $\mathbb{R} _+.$

Theorem 3.3 (from [3], corollary 4.27). If $\mathcal{F}$ is a bounded set of ${\rm{L}} ^1(\Omega,\nu)$ such that (14) and (15) hold true, then $\mathcal{F}$ is relatively compact in ${\rm{L}} ^1(\Omega, \nu).$

3.1.   Existence of a principal eigenfunction for $T_\Sigma$

Using Theorem 3.1, we prove the existence of an eigenpair $(\rho_\Sigma, f_\Sigma)$ for the operator $T_\Sigma.$

Proposition 1. Let $l$ be a nonpositive number. Under the hypotheses (2), (3) and (4), there exists a unique normalized eigenvector $f_\Sigma \in {\rm{L}} ^1_+((b_\theta,\Sigma),s^l \, {\rm{d}} s)$ of the operator $T_\Sigma$ in ${\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)$ associated to the spectral radius $\rho_\Sigma$ for every $\Sigma>\max(\frac{1}{1-\theta}b,1)$.

Applying Theorem 3.3, to $\Omega = (b_\theta,\Sigma)$ and the family

which is bounded in ${\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s),$ as already shown in the proof of Lemma 2.2, we prove the following Lemma.

Lemma 3.4. Let $l$ be a nonpositive number. Under the hypotheses of Proposition 1, for all

the set $\mathcal{F}$ is relatively compact.

Proof of Lemma 3.4. The set $\mathcal{F}$ is bounded due to the continuity of $T$ proven in Lemma 2.2. First, we show that (14) is satisfied. Any compact set in $(b_\theta, \Sigma)$ is included in a segment $[\alpha, \beta].$ Without loss of generality, we take $b_\theta<\alpha<\theta\Sigma,\ \eta\Sigma<\beta<\Sigma.$ It is sufficient to treat the case $h$ positive, so let $0\leq h<\min(\theta\Sigma-\alpha, \Sigma-\beta, \Sigma(\eta-\theta)).$ Since $T_\Sigma f$ is piecewise defined, we have to separate the integral on $[\alpha,\beta]$ into several parts, depending on the interval $s$ and $s+h$ belong to, and we obtain

since for $(A),\ (C)$ and $(E),$ $T_\Sigma f$ and $\tau_h T_\Sigma f$ have the same expression, the same kind of calculations apply, so we only treat $(C),$ which has the most complicated expression.

The integrals $(C1)$ and $(C3)$ are dealt with in the same way, and we have the following estimate

These integrals are as small as needed when $h$ is small enough, due to the continuity of the translation in ${\rm{L}} ^1( \mathbb{R} _+).$ For $(C2)$ one has

which is small when $h$ is small since $\Phi$ is a probability density. To deal with $(C4),$ we use Fubini's theorem and some changes of variables to obtain

and the continuity of $\Psi$ at $0$ provides the wanted property. Finally, noticing that $(C5)\leq (C2)$ because the integrand are nonnegative, we obtain the desired control on the integral $(C).$ Now for the integral $(B),$ which is dealt with as would be $(D),$ we write

and again the last term vanishes as $h$ vanishes. We now show that there is no mass accumulation at the boundary of the domain $(b_\theta,\Sigma),$ i.e. that (15) holds true. For $\Sigma > \frac{1}{1-\theta}b,$ we haves $b_\theta < \theta \Sigma$ and we can choose $\alpha < \theta \Sigma,$ so that for all $s\in (b_\theta,\alpha),\ \frac{s}{\Sigma} < \theta.$ With the expression of $T_\Sigma f(s),$ we have

since for $b_\theta < s \leq \frac{b_\theta}{z}$ we have $\frac{b_\theta}{z}-a\leq b$ and so $\Psi(\frac{b_\theta}{z}-a) = 1.$ Taking $\alpha$ as closed to $b_\theta$ as needed, we obtain the first estimate of (15).

As done before, we choose a $\beta$ to obtain a simpler expression of $T_\Sigma,$ namely $\beta > \eta\Sigma.$ Then, one has

which is small when $\Sigma-\beta$ is small.

We have checked the assumptions of Theorem 3.3 for the family $\mathcal{F},$ so it is relatively compact.

To prove the irreducibility of the operator $T_\Sigma,$ it is useful to notice that $T_\Sigma$ can be expressed differently after switching the two integrals. One has

Lemma 3.5. Let $l$ be a nonpositive number. Under the hypotheses of Proposition 1, for all $\Sigma>\frac{1}{1-\theta}b$, the operator $T_\Sigma : {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s) \rightarrow {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)$ is irreducible.

Proof. Let $J\neq \{0\}$ be a $T_\Sigma$-invariant ideal in ${\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)$. There exists a subset $\omega \subset (b_\theta,\Sigma)$ such that $J = \{f \in {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)\ |\ {\rm{supp}} \, f \subset \omega \}.$ Let $f_\omega: = s^{-l}{1}_\omega$ and $s_0: = \inf {\rm{supp}} \, f_\omega \geq b_\theta.$ Since $J\neq \{0\}$ (so $s_0<\Sigma)$ and $\theta<\eta,$ one can find $\zeta$ and $\xi$ both positive such that

For $s\geq s_0,$ the functions $z\mapsto \frac{s}{z}-a$ and $a\mapsto \frac{s}{z}-a$ are continuous decreasing functions. So, if $s$ is such that $\frac{s}{\theta}-s_0>b,$ then one can choose $\zeta$ and $\xi$ such that for all $(s,a)\in [s_0,s_0+\zeta]\times [\theta, \theta + \xi],\ \frac{s}{z}-a \in {\rm{supp}} \, \Phi.$ Additionally, for each $\zeta>0,$ the integral $\int_{s_0}^{s_0+\zeta}f_\omega(a) \, {\rm{d}} a$ is positive. We deduce that $[\theta(b+s_0),\Sigma]\subset {\rm{supp}} \, T_\Sigma f_\omega \subset [s_0,\Sigma],$ so $s_0 \leq \theta(b+s_0),$ which is equivalent to $s_0 \leq b_\theta.$ Finally $b_\theta = s_0,$ so $J = [b_\theta,\Sigma]$ and $T_\Sigma$ is irreducible.

Proof of Proposition 1. Lemma 3.4 shows that the set $\mathcal{F}$ is relatively compact in ${\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)$, which is exactly saying that $T_\Sigma$ is a compact operator of the Lebesgue space ${\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s).$ With Lemma 3.5 in addition, we can apply Theorem 3.1 to the operator $T_\Sigma$ for $\Sigma>\frac{1}{1-\theta}b$ to obtain the existence of a nonnegative function $f_\Sigma \in {\rm{L}} ^1((b_\theta,\Sigma),s^l \, {\rm{d}} s)$ which is an eigenvector of $T_\Sigma$ associated to the eigenvalue $\rho_\Sigma.$ Since this function is defined on a compact subset of $\mathbb{R} _+,$ it also belongs to ${\rm{L}} ^1((b_\theta,\Sigma),(s^k+s^l) \, {\rm{d}} s)$ for $k<k_0.$

3.2.   Passing to the limit $\Sigma\to \infty$

We now want to show that up to a subsequence, $(f_\Sigma)_\Sigma$ converges to a fixed point of $T$. To that end, in the rest of the article we extend the functions defined on $(b_\theta,\Sigma)$ to $\mathbb{R} _+$ by $0$ out of $(b_\theta,\Sigma)$. Then we obtain the following proposition

Proposition 2. Under hypotheses (2)- (5) there exists a nonnegative and normalized fixed point

for all $l\leq0$ and $k<k_0,$ of the operator $T.$ Additionally, $f$ is unique in ${\rm{L}} ^1( \mathbb{R} _+)$ and its support is $[b_\theta, \infty).$

First, we will show that the sequence $(\rho_\Sigma)_\Sigma$ converges to $1$ as $\Sigma \to \infty$.

Lemma 3.6. If $(\rho_\Sigma,f_\Sigma)$ is an eigenpair of the operator $T_\Sigma,$ then the following inequality holds true

Proof. Integrating the equality $\rho_\Sigma f_\Sigma = T_\Sigma f_\Sigma$ over $(b_\theta,\Sigma)$, one has

Then notice that for $a\in(b_\theta,\Sigma)$ and $z\in (\theta,\eta)$ one has

so as in the computations leading to (16), $\Psi(\frac{b_\theta}{z}-a) = 1.$ Combining these different expressions, we deduce

Using the fact that the function $\Psi$ is nonincreasing, we obtain the wanted inequality.

Now we show that up to a subsequence, $(f_\Sigma)_\Sigma$ converges to a fixed point of $T$ denoted by $f$. Thanks to (18) and the properties of $\Psi,$ we can define

Lemma 3.7. Under hypotheses (2), (3), (4) and (5), the set of eigenfunctions $\{f_\Sigma, \Sigma \geq \Sigma_0, \|f_\Sigma\|_{ {\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)} = 1\}$ has a compact closure in ${\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)$, for any $l\leq0$ and $0\leq k<k_0, k_0$ being the real number given in (5).

Proof. Let $l\leq0$ and $k\in[0,k_0).$ Once again, we apply Theorem 3.3 to show the desired result. First, we show that (14) hold true with $\Omega = (b_\theta, \infty)$ and $\mathcal{F} = \{f_\Sigma, \|f_\Sigma\|_{ {\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)} = 1\}.$ Let $\omega$ be a compact subset of $(b_\theta,\infty)$ and $b_\theta<\alpha<\beta$ such that $\omega \subset [\alpha,\beta].$ We use the following inequality

The last quantity is small when $h$ is small uniformly with respect to $\Sigma$ since in the proof of Lemma 3.4, the estimates do not depend on the value of $\Sigma.$ To prove that (15) holds true, we use the estimate (16) twice to write

which is again independent of $\Sigma.$ The estimate (17) though depends on $\Sigma,$ so we write for $\Sigma$ larger than $\beta$

For the first integral, we compute

The two other integrals correspond to the integrals $(B),\ (C)$ and $(D)$ from the previous proof. Combining the integrals, we obtain

We deal with the last integral using (19) and obtain after interverting integrals

Since $0<\theta < \eta < 1,$ we can choose $\beta > \frac{\eta}{1-\eta}b \geq b_\theta.$ In that case, $\frac{1}{\eta}-\frac{b}{\beta} > 1,$ and we can pick $r\in ]1,\frac{1}{\eta}-\frac{b}{\beta}[$ such that $\left(\frac{1}{\eta}-r\right)\beta > b.$ Noticing that $1-\Psi(\frac{\beta}{z}-a)$ and $1-\rho_\Sigma$ are nonnegative, we obtain

then

and finally

for $\beta$ large enough. We use this estimate to get

due to hypothesis (5), for $\beta$ large enough.

We are now ready to prove the existence and uniqueness of a fixed point for the operator $T.$

Proof of Proposition 2. We have proved in Lemma 3.7 that the set of eigenfunctions $\{f_\Sigma,\ \|f_\Sigma\|_{ {\rm{L}} ^1( \mathbb{R} _+,(s^l+s^k) \, {\rm{d}} s)}\}$ has a compact closure in ${\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s).$ We deduce the existence of $f\in {\rm{L}} ^1( \mathbb{R} _+,(s^k+s^l) \, {\rm{d}} s)$ such that, up to a subsequence still denoted by $(f_\Sigma)_\Sigma$, $f_\Sigma \to f$ strongly as $\Sigma \to+ \infty$. Now we prove that the function $f$ is a fixed point of the operator $T.$ We use the following inequality

The first term of the right-hand side tends to zero as $\Sigma$ tends to $\infty$ by definition of $f,$ and the second one is smaller than $\Psi\big( (\frac{1}{\eta}-1)\Sigma\big)$ according to (18). For the last one, we write

due to Lemma 3.2 and to the continuity of $T,$ which is proved in Lemma 2.2.

To prove uniqueness of the fixed point, we consider $f_1$ another nonnegative fixed point of $T$ in ${\rm{L}} ^1( \mathbb{R} _+)$ satisfying $\int_0^\infty f_1(s) \, {\rm{d}} s = \int_0^\infty f(s) \, {\rm{d}} s.$ Recalling the definition (12) of the operator $T$, the functions $f$ and $f_1$ satisfy the integral convolution equation

Since $f,\ f_1$ and $\Phi$ are in ${\rm{L}} ^1( \mathbb{R} _+)$, their Laplace transforms exist on $\mathbb{R} _+$ and are continuous decreasing functions. Taking the Laplace transform of $f-f_1$ and switching integrals thanks to Fubini's theorem, one has for every $y\geq0$

The Laplace transform $\mathcal{L}[f-f_1]$ is continuous on $\mathbb{R} _+$ and vanishes at the origin

We now define the functions

By continuity in $0$ of $\mathcal{L}[f-f_1]$ and because $\mathcal{L}[f-f_1](0) = 0,$ one has

From (21), we obtain the inequality

since $\Phi$ is a probability measure. $\overline{\mathrm{L}}$ is a continuous increasing function, so for all $x\leq y,$ one has

from which we deduce

Iterating (22), we obtain for all $y\geq 0$ and all positive integer $j$

Letting $j\to\infty$ in this inequality and using the continuity of the function $\overline{{\rm{L}}}$ we obtain $\overline{{\rm{L}}}(y) = 0$ for all nonnegative $y.$ With the same method, we show that $\underline{{\rm{L}}}(y) = 0$ for all nonnegative $y,$ and finally $\mathcal{L}[f-f_1]$ is the null function. By the injectivity of the Laplace transform (Lerch's theorem [11]), one has $f = f_1.$

It remains to prove that ${\rm{supp}} \, f = [b_\theta,\infty).$ With the same kind of proof than the one we used for $T_\Sigma$, we can prove that $T$ is irreducible on ${\rm{L}} ^1(b_\theta,\infty)$, and since $f$ is not the zero function we get the result.

3.3.   Proof of the main theorem

We are now ready to prove the main theorem of the paper.

Proof of Theorem 1.1. Combining Lemma 2.1 and Proposition 2, we construct a solution to (11) using

It remains to prove uniqueness of the solution in the appropriate space. This solution belongs to ${\rm{L}} ^1( \mathbb{R} _+^2, (1+s^2) \, {\rm{d}} a \, {\rm{d}} s)$ thanks to the following calculation

because $f\in {\rm{L}} ^1((b_\theta,\infty),s^l \, {\rm{d}} s)$ for all nonpositive number $l.$ To prove the uniqueness of the solution $M\in {\rm{L}} ^1( \mathbb{R} _+^2, (1+s^2) \, {\rm{d}} a \, {\rm{d}} s)$, consider another solution $M_1\in {\rm{L}} ^1( \mathbb{R} _+^2, (1+s^2) \, {\rm{d}} a \, {\rm{d}} s)$. Necessarily, as for $M,$ there exists a measurable function $f_1$ such that for almost all $s\geq a\geq0$

For $0 < \alpha < \beta < \infty,$ we can write

and this ensures that $f_1 \in {\rm{L}} ^1( \mathbb{R} _+).$ Additionally we easily check as in Lemma 2.1 that $f_1$ has to be a fixed point of $T.$ Then the uniqueness result in Proposition 2 ensures that $f_1 = f,$ and so $M_1 = M.$ The existence and uniqueness of a solution to the initial problem (8) follows from the relation (10).

4.   Entropy and long time behaviour

Now that we have solved the eigenvalue problem, we would like to characterize the asymptotic behaviour of a solution $n$ of (1), as in [18]. The General Relative Entropy principle provides informations about the evolution of the distance in ${\rm{L}} ^1$ norm between a solution $n(t,\cdot,\cdot)$ and ${\rm{e}} ^tN.$ To establish such useful inequalities, we use the formalism introduced in [13] and [14]. Strictly speaking, to use this method, we should prove some properties on a time-dependent solution $n,$ in particular its existence and uniqueness for any reasonable initial condition. Let us here assume the existence of such a solution, which moreover satisfies the common estimate (see [18])

It is usually ensured by the hypothesis $|n^0(a,x)|\leq CN(a,x)$ and a maximum principle. For $H$ a function defined on all $\mathbb{R} ,$ we define, for $n\in {\rm{L}} ^1( \mathbb{R} _+^2)$

which satisfies the following entropy property.

Proposition 3. If $n$ is a solution of (1) satisfying (23), then

with

where $\, {\rm{d}} \nu_x(a,z) = \frac{B(a)N(a,\frac{x}{z})}{N(0,x)z^2} \, {\rm{d}} a {\rm{d}} \mu (z)$ is a probability measure. Furthermore if $H$ is convex, then $\mathcal{D}\geq 0.$

Before proving this proposition, we make a remark about the conservative problem (i.e. when only one daughter out of two is kept after division). In this case, the dominant eigenvalue is $0$ instead of $1$, and $xN(a,x)$ is an eigenvector associated with the eigenvalue $0,$ since the total mass is preserved. Then we obtain the equation

which might also be obtained multiplying (8a) by $x.$

Proof. Easy computations lead to

where $N(a,x)>0$, i.e. on the domain $\Omega_\theta: = \{x-a>b_\theta\}$. From this equality and (25), we deduce

and integrating (26) over $\Omega_\theta,$ we obtain

since for $x\in [zb_\theta,b_\theta]$ and $z\in [\theta,\eta], \frac{x}{z}-b_\theta\leq b,$ and we conclude using Jensen's inequality.

Appropriate choices of the function $H$ in (24) lead to interesting results. With $H(x) = x$, we recover the conservation law (7). Then taking $H(x) = |1-x|$, we obtain the decay of $\|N-ne^{-t}\|_{ {\rm{L}} ^1( \Omega_\theta, x \, {\rm{d}} x \, {\rm{d}} a)}$ as $t$ tends to infinity. In the case where the fragmentation kernel $\mu$ has a density with respect to the Lebesgue measure on $[0,1],$ we expect that this quantity will vanish, as in [14,18]. In contrast, in the case of the equal mitosis, there is not hope for this distance to vanish. Indeed, one has an infinite number of eigentriplets $(\lambda_j,N_j,\phi_j)$ with $j\in \mathbb{Z}$ defined by

so we expect a behavior as in [2], i.e. the convergence of $n(t,a,x) {\rm{e}} ^{-t}$ to the periodic solution

where $\langle n,\phi\rangle = \int\int n(a,x)\phi(a,x) \, {\rm{d}} a \, {\rm{d}} x.$

5.   Discussion and perspectives

We have proved the existence and uniqueness of a solution of the eigenproblem (8) in the special yet biologically relevant case of linear growth rate with a self-similar fragmentation kernel. Hypotheses on both this kernel and the division rate are fairly general.

As possible future work we can imagine to extend the result to general growth rates. In this case the Perron eigenvalue is not explicit and it has to be determined in the same time as the eigenfunction, as in [26,12,6]. If we denote by $\lambda$ the eigenvalue, the equivalent of Equation (20) is

with $P_\lambda(y) = e^{\lambda \int_1^s\frac{ \, {\rm{d}} u}{g(u)}}M(0,s)$ and the equivalent of the solution given in (9) is

Additionally for nonlinear growth rates, the function $(a,x)\mapsto x$ does not provide a conservation law as in (7), and it has to be replaced by a solution to the dual Perron eigenproblem. Such a dual eigenfunction appears in the definition of the General Relative Entropy [13,14], and for proving its existence one could follow the method in [19,8] for the size-structured model. Another possible generalization of the growth rate is adding variability, in the spirit of [21,15,17]. One might also consider a more general fragmentation kernel than in the case of self-similar fragmentation, or/and with a support which is not a compact subset of $(0,1).$

The other natural continuation of the present work is the proof of the well-posedness and the long-time behavior of the evolution equation, as in [26,12]. To do so one can take advantage of the General Relative Entropy as in [14,4,2] or use general spectral methods [28,16].

Acknowledgments

The authors are very grateful to Marie Doumic for having suggested them the problem treated in this paper, and for the many fruitful discussions.