Metastable capillary filaments in rectangular cross-section open microchannels

1.   Introduction

In biotechnology, biology and medicine, point of care (POC) and home care systems are progressively gaining momentum 1, 2, 3, 4, 5. These systems allow for self-testing and telemedicine. Three main types of test are targeted: first, the search for metabolites—such as cholesterol, glucose, thyroid hormones; second, the search for viral load—such as virus and bacteria; third blood monitoring—such as the measure of INR (prothrombin, thromboplastin and coagulation times) or blood counts. Monitoring at home or at the doctor's office is an important improvement for the patient: the possibility of frequent testing, immediate response, no displacement to the hospital, and monitoring allowed by telemedicine or directly at the doctor's office. Such systems must be low-cost, easily portable, sensitive, and robust.

Let us recall that conventional microfluidic systems use fluids moved by pumps, syringes or electric means, and are not the best solutions when portability and low-cost are an issue. Such systems are bulky and/or require costly, external appliances.

On the other hand, capillary-based systems fulfill the requirements for POC and home-

care 2, 3, 4, 5. In these systems, fluids are moved by capillary and surface tension forces. The self-motion of the fluid is called spontaneous capillary flow (SCF). In fact, in such systems the energy source for the fluid motion is the capillary force exerted by the triple line at the front end of the flow. This contrasts with pump or syringe-driven flows, where the motor of the motion is a pump or a syringe usually placed at the back end of the flow.

Capillary systems can be either confined or open, i.e. the fluid moves inside a closed channel 4, 5, 6 or in a channel open to the air 7, 8, 9, 10, 11, 12.Although capillary-driven microflows have already been the subject of investigations for space applications 13 and microelectronics 14, 15, 16, their main application today is biology and biotechnology. Note that open-surface microfluidic systems are of increasing interest because of their easy and inexpensive fabrication, absence of air bubbles, and their great accessibility.

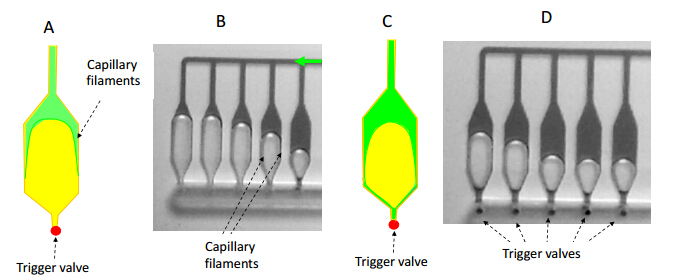
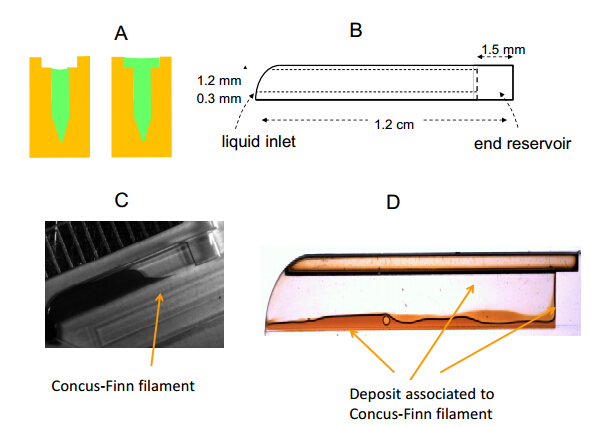
It has been shown that the aspect ratio (width divided by height) of such channels is an important parameter for the establishment of a spontaneous capillary flow (SCF) 17, 18, and it has been shown that capillary precursor filaments may form in sharp corners, depending on the corner angle and on the contact angle 19, 20, 21. These filaments can theoretically extend indefinitely, as long as there is sufficient liquid available, and favorable geometrical conditions 22. They are frequently called Concus-Finn (CF) filaments, from the name of the first researchers who discovered the phenomenon. Figure 1 shows how CF filaments progressing ahead of the bulk capillary flow may trap undesirable bubbles in confined capillary networks.

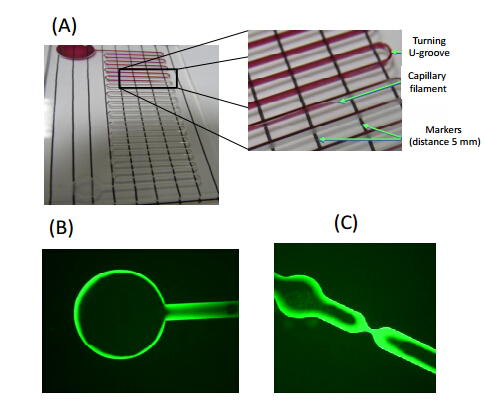
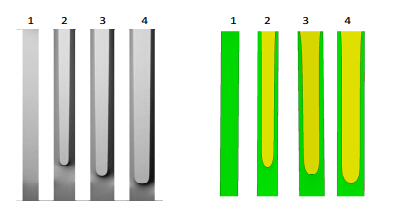
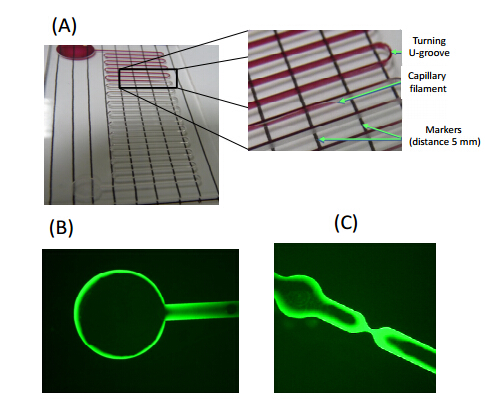
CF filaments are also observed in open channels with sharp corners, as shown in Figure 2 for rectangular U-grooves.

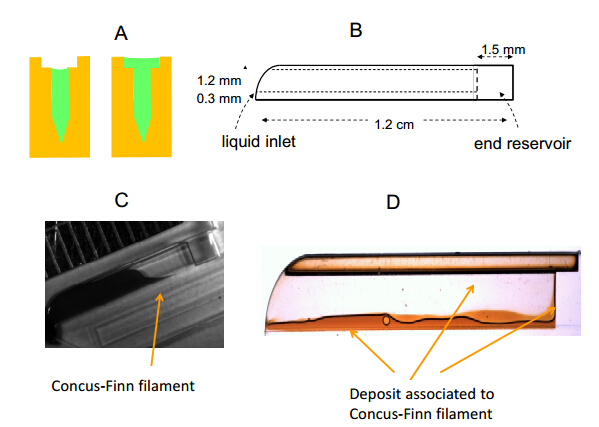
Let us remark that CF filaments are also of great importance when reagents are present in the liquid: it is observed that there is a spatial correlation between the dry reagent deposition regions and the regions where CF precursor filaments are flowing (figure 3) 9, 23.

In this work, we restrict our investigations to the different flow regimes that occur in a U-groove of uniform cross-section, depending on the geometrical aspect ratio and the liquid-solid contact angle. A diagram of the flow regimes is theoretically determined and numerically checked with the Surface Evolver numerical program 24. It is shown that no SCF, bulk SCF (i.e. full channel capillary flow), just capillary filaments in corners, or both SCF and capillary filaments may occur depending on the geometry and contact angle. Moreover, it is shown that the capillary CF filament regime may be metastable. Our approach differs from that of Seemann and colleagues, which only considered droplets in open channels, not SCF 25, but we shall see that there are common features. We finally illustrate the approach by experimental views of the flow regimes.

2.   Theoretical approach

We consider here open, rectangular, U-shaped channels of uniform cross-section, and we first present the theoretical approach in the case of a bulk SCF (contact angle above 45°) with no capillary filaments. Then we investigate the case of contact angles smaller than 45°, for which capillary filaments develop.

2.1.   SCF in uniform cross-section channels (contact angle > 45°)

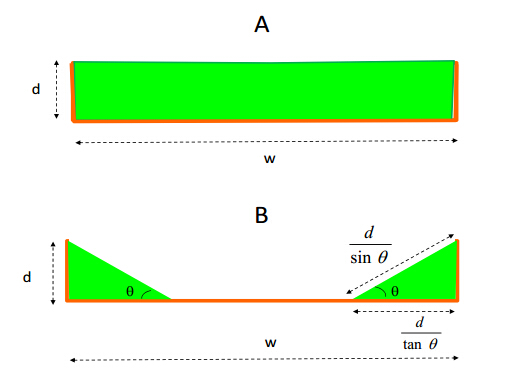
Let us first recall the condition that allows for SCF in uniform cross-section channels. Spontaneous capillary flow results when the energy reduction from wetting walls outweighs the energy increase from extending the free surface. In the case of a contact angle larger than 45°—we will give later the justification of this value—the whole width of the bottom plate is wetted or not, depending on a geometrical condition. Using Gibbs' thermodynamic equation 26, it has been shown that the general condition for SCF in composite wall-and-air systems is that the generalized Cassie angle must be less than 90° 17. Let us recall that the generalized Cassie angle θ* is the average contact angle defined in the appropriate way, i.e

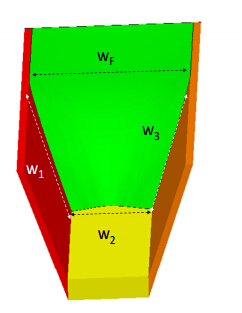
where the θ_k are the Young contact angles with each component k (including air) and the f_k are the areal fractions of each component k in a cross section of the flow (Figure 4). Note that the effective contact angle with air is 180°.

In the case where the walls are composed of a single material (with the same functionalization), relation (1) reduces to

where p_F and p_W are respectively the free—in contact with air—and wetted—in contact with wall— perimeters in a cross section of the channel 21. Note that the contact angle θ is the static contact angle, with hysteresis if the solid surface is such that there is a contact hysteresis. The dynamic contact angle is not a concern here since the SCF limit occurs at zero—or near zero—velocity.

Using (2), one check that the SCF condition in rectangular cross-section open U-channel is

or

Relation (4) indicates whether the liquid advances in the channel, filling the entire cross section.

2.2.   SCF in uniform cross-section channels (contact angle < 45°)

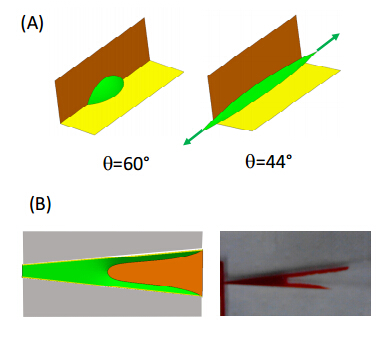
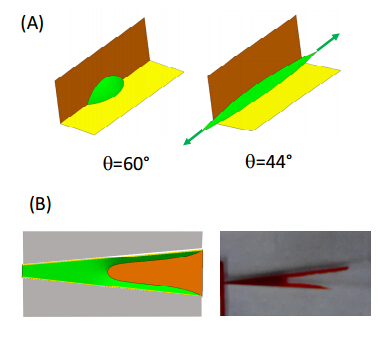
Second, let us recall the condition for filaments to form in dihedrals and corners 19, 20, 21. It has been observed that liquid interfaces in contact with highly wetting solid walls forming a wedge tend to spread in the corner. This behavior results from the fact that the energy decrease from wetting the walls is greater than the energy of the liquid-air interface, so the contact area wants to expand indefinitely, which it can do at low volume by making an ever thinner filament (Figure 5).

Concus and Finn 19 have investigated this phenomenon and they have derived a criterion for capillary motion in the corner of the wedge. If θ is the Young contact angle on both planes and α the wedge half-angle, the condition for capillary self-motion is

In the case of a rectangular cross-section, α = 45°, and the contact angle θ should be smaller than 45° to observe filaments.

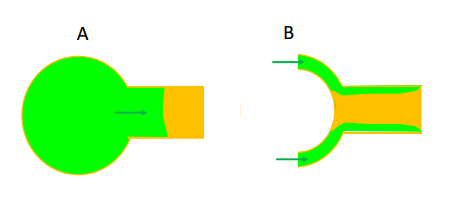
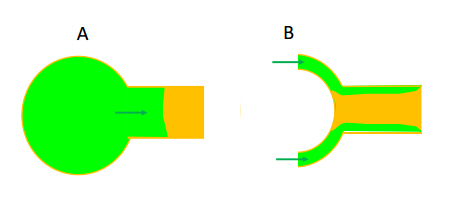
We now consider the case an open channel with a rectangular cross-section and a contact angle of the liquid with the walls θ < 45°. Two inlet conditions are investigated as shown in Figure 6: first, a large reservoir full of liquid at zero pressure (flat interface); second, a double inlet with separated flows.

2.3.   SCF self-dividing into filaments

Let us analyze the case of a straight, rectangular U-groove of large aspect ratio, i.e. q = w/d > > 1, where w is the channel width and d is the channel depth. What qualifies as large aspect ratio will be shown to depend on the contact angle, with smaller contact angles leading to higher critical aspect ratios ("critical" meaning the largest aspect ratio compatible with SCF).

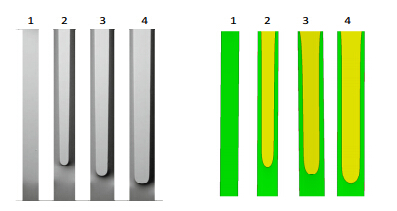
This morphology has been experimentally investigated and reported in the literature 27. In that publication, the rectangular cross-section channel has a height of 8.8 µm and four different widths: 160, 180, 200 and 220 µm (Figure 6). For all these, the aspect ratio is higher than 18. The inlet pressure is supposed to be zero (large reservoir with flat liquid surface).

Analyzing the experimental results, two morphologies of the flow are observed, depending on the value of the aspect ratio (Figure 7). In the first case (1) of the smallest aspect ratio, the channel is completely filled by the fluid, and two filaments advance ahead of the bulk flow. In the second case (2-4), the bulk flow is stopped but two filaments spread in the corners. Figure 8 represent this situation in a cross section.

From a theoretical standpoint, the variation of the surface energy over a distance Δx is different in the two cases: The variation of surface energy in the case of the non-wetted channel center is

where γ_SG, γ_SL and γ_LG are respectively the surface tensions between the solid and gas, solid and liquid, and liquid and gas.

In the case of a totally wetted center,

The sign of ΔE₁-ΔE₂ indicates whether the center of the channel will be wetted or not. Subtracting (7) from (6) and using the Young relation one finds

The center of the channel is not wetted if ΔE₁-ΔE₂ < 0, i.e.

If relation (9) is not satisfied, then the smaller surface energy morphology is that of the fully wetted channel. Note that in this case, there are two CF filaments ahead of the bulk flow. Else, if relation (9) is satisfied, the smaller surface energy morphology is that of two separate CF filaments. The bulk flow is stopped just after the outlet of the reservoir but two CF filaments flow separately alongside the walls.

Note that relation (9) involves the contact angle. The capillary filaments are advancing in the channel, and the question is whether a static or dynamic contact angle be considered here, Due to the geometry of the filaments, the advancing front should present a dynamic contact angle, while the side of the filament, in contact with the bottom, is not advancing (nor receding), and a static value of the contact angle is likely. As our concern is the contact area on the bottom plate in a cross section it seems justified using the static contact angle.

Moreover, considering the advancing tip of the filaments, and remarking that the liquid in the middle of the channel is at nearly zero velocity, the filaments advance slowly, due to the effect of the surface tension. Hence their capillary number is very small. Using the empirical correlations of Bracke and colleagues 28, or Seebergh and colleagues 29, based on the capillary number, we find that the difference between the static and dynamic contact angle is less than 1 or 2°. Hence we estimate that the error associated to a constant value of the contact angle is very small.

2.4.   Initially separated Concus-Finn filaments

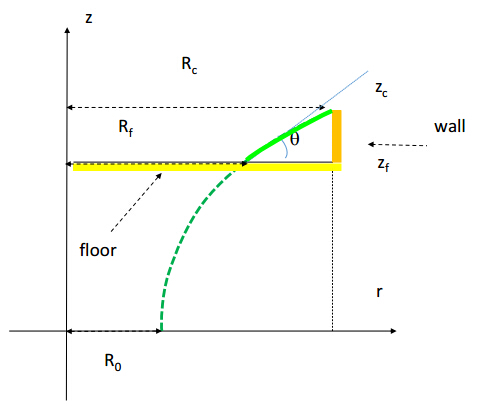
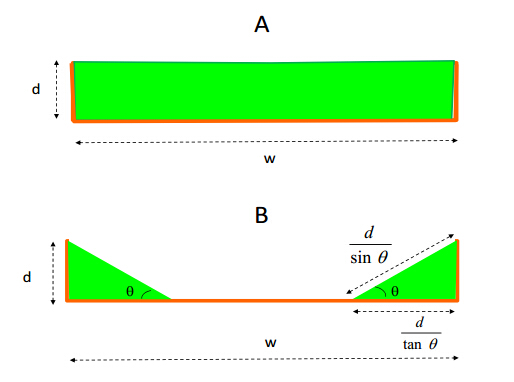
Now consider case B of Figure 8: the fluid inlet is constituted by two separated flows. Two separated Concus-Finn filaments advance in parallel in the open-top channel. In this case, because the pressure is zero, the cross-section of the flow is triangular, and the condition for a filament to continue its motion is given by relation (2).

Using a trigonometric formulation for the free perimeter p_F and the wetted perimeter p_W (Figure 8), relation (2) can be expressed by

Finally, we obtain

and the condition for motion is θ < π/4, which is simply the Concus-Finn condition for perpendicular corners.

On the other hand, using again the triangular shape of the profile in a cross-section, the two filaments stay separated as long as

2.5.   Metastability of CF filaments

An interesting observation stems from the comparison of relations (9) and (12). It can easily be shown that the inequality

is always true whatever the value of θ. If the value of the aspect ratio q is between the two limits of inequality (13)

then the channel can sustain either a bulk SCF flow filling the channel or two CF filaments.

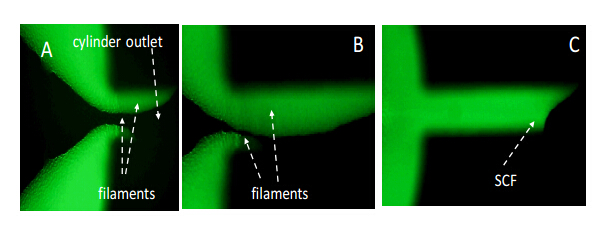
The full and filament modes can change in a single channel. This property is illustrated in Figure 9. When the liquid exits the large reservoir, the channel completely fills, and the channel middle is covered with liquid. When the SCF enters a cylindrical enlargement, the flow separates if the cylinder radius is sufficiently large. The condition for observing a dry disc in the middle is derived in the Appendix, based on the catenoidal shape of the liquid surface 21. The flow is separated into two subflows, and if the cylinder outlet condition satisfies (12), two independent CF filaments continue to flow in the straight outlet channel.

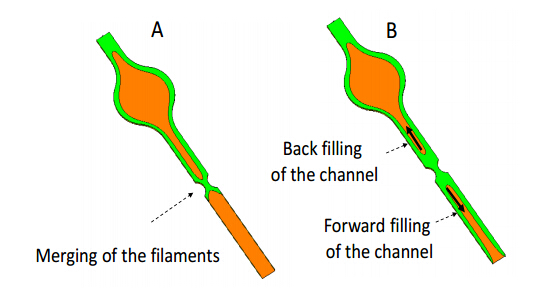
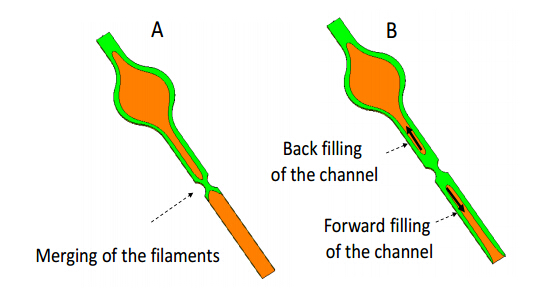
An experimental view of the capillary flow of the liquid in the cylinder is shown in Figure 10, using water marked with a fluorescent dye. The aspect ratio of the channel is w/d=1/4. In this particular case, the outlet channel aspect ratio does not satisfy (12) and the two filaments merge (Figure 11).

In the case of our study (Figure 9), relation (14) is satisfied because downstream of the cylinder the geometrical dimensions are the same as upstream of the cylinder. The analysis of section 2.4 stands and two separate CF filaments flow in the channel. The two filaments are independent, they never merge and the middle of the channel is never wetted.

However, this morphology is metastable: if the two filaments are forced to contact each other, the fully wetted situation is restored, as shown in Figure 12. Note that the restoration of the fully wetted morphology also progresses backward towards the large cylinder.

The corresponding experimental result is shown in figure 13: A neck in the channel forces the separated subflows to merge. The merged part then extends in the two directions, upstream and downstream.

3.   Results and Discussion

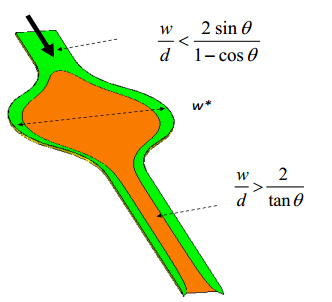
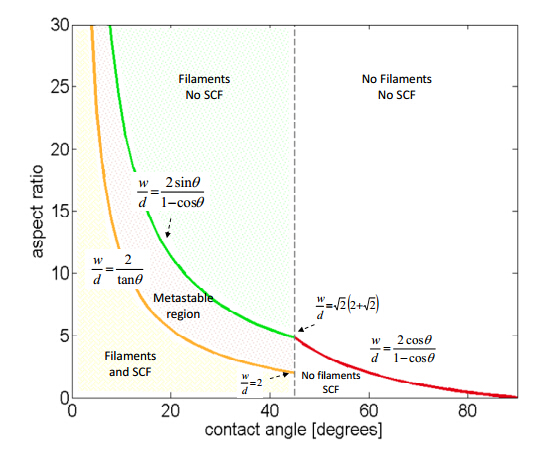
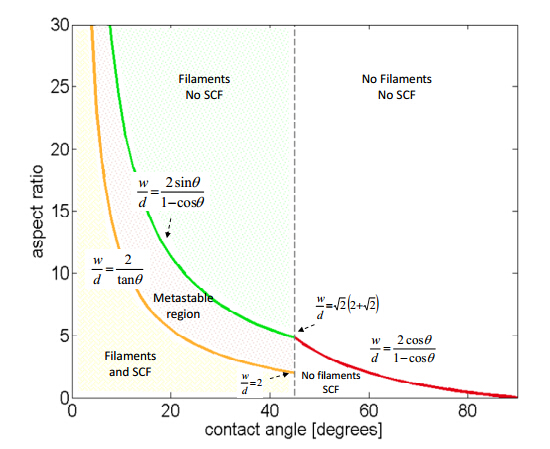
We have seen in section 2.1 that the SCF conditions in rectangular cross-section open U-channel, when the contact angle is larger than 45°, is q = w/d < 2cosθ / (1-cosθ). In such a case, there is no formation of capillary filaments because the CF limit (5) is not respected.

For an aspect ratio not respecting (14), neither bulk SCF nor capillary filaments are observed.

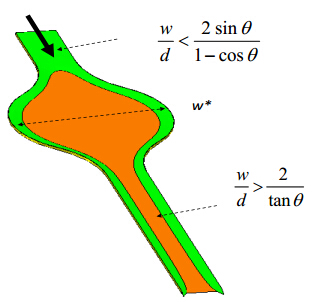
When the contact angle is smaller than 45°, the situation is more complicated: (1) above the limit q > 2sinθ / (1-cosθ), no bulk SCF is observed, but two CF filaments spread in the corners; (2) below the limit q < 2 / tanθ bulk SCF is observed preceded by CF filaments; (3) for an aspect ratio between 2 / tanθ≤ q ≤ 2 sinθ / (1-cosθ), the situation is metastable and depends on the history of the flow: either separated CF filaments progress in the channel, or a full SCF occurs preceded by two CF filaments.

All the above conditions are shown in Figure 14. It is interesting to compare Figure 13 with the experimental and numerical results obtained by Seemann and colleagues for a liquid drop placed in a rectangular open U-groove 25. In their representation, the axes (aspect ratio vs. contact angle) are inverted and the aspect ratio is also inverted. But the two theoretical curves (green and red) of Figure 13 corresponding to the relations q = 2 sinθ / (1-cosθ) for θ < 45° and q = 2 sinθ / (1-cosθ) for θ for θ > 45° are identical to the experimental curves of 25.

4.   Conclusion

Capillary filaments—or Concus-Finn filaments—often appear in microsystems treated hydrophilically. These filaments can have a very large extension, as long as there is liquid available and the geometry of the corners continues. Sometimes they are a drawback because of an unwanted loss of liquid, or an undesirable propagation of liquid. Sometimes they are useful, because they boost the velocity of the capillary flow 9. Moreover, they can flow alone while the bulk of the liquid is at rest, or precede the bulk of the liquid.

In this work, the appearance of such filaments and their stability has been studied for a rectangular cross-section, open channel. The conditions under which they appear have been determined, together with their stability and their association to the capillary motion of the bulk flow.

Appendix: capillary flow in a cylindrical cavity

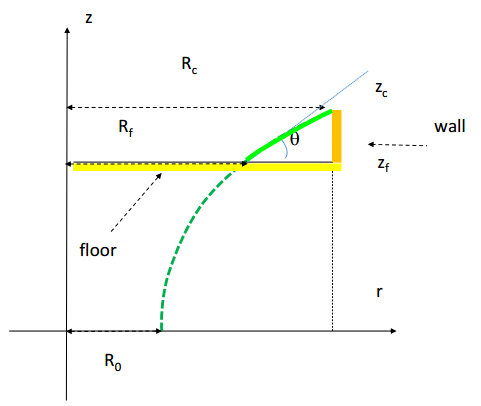
When a SCF arrives in a cylindrical cavity, three flow configurations are possible: (1) the SCF stops, (2) the SCF entirely fills the cavity, (3) filaments fill the bottom corners and the liquid surface has approximately the shape of a catenoid, with the center of the cylinder staying dry 20. These configurations depend on the radius of the cylinder and the contact angle. In the case of a contact angle under 45°, only the two last cases are possible. Let us analyze the condition for observing a catenoid (Figure 15).

A catenoid is a zero-pressure surface shape with cylindrical symmetry. The liquid surface shape in the cylindrical enlargement of a channel is not exactly a catenoid, due to the entry and exit openings, but because the liquid in the openings is below the wall height, a catenoid with the height of the wall and the same contact angle on the floor will act as a barrier beyond which the liquid will not pass.

The equation of a catenoid with a neck defined by z = 0, R = R₀, is

The first condition to determine R₀ is the slope at the contact with the floor: at the floor level (z = z_f) the tangent to the catenoid has a slope dz/dr = tanθ, so

hence

The second condition is the contact at the top, on the edge of the cylinder,

Substitution of (17) in (18) yields

which is an implicit solution for R₀. There is a critical value for R_c below which there are no solutions to (19), and above which there are two solutions for R₀, the larger of which is the stable catenoid of interest. We denote that stable solution R₀=f(θ, h). The floor level is given by (17) using the value of R₀

and using the relation cosh²(z)−sinh²(z)=1, the radius of the dry disc in the middle of the cylindrical cavity is then

Hence, if R_c is greater than the critical value and the liquid enters the cylinder through its ingress opening, a dry disc remains in the middle of the cylinder. This is the case of Figure 9. If the outlet of the cylinder is wider than condition (10), the liquid remains separated in two parts on egress, and two CF filaments continue to advance in the outlet channel.

Acknowledgements

We thank Melanie Flaender, Noémie Villard, Catherine Pudda, and François Boizot from the CEA-Leti, for their help achieving the microflabrication of the capillary devices. We also acknowledge theBiotechnology Department of the CEA-Leti for the funding of this work.