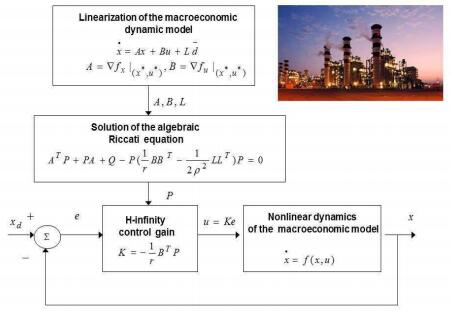
A nonlinear optimal control approach to stabilization of a macroeconomic development model

1.   Introduction

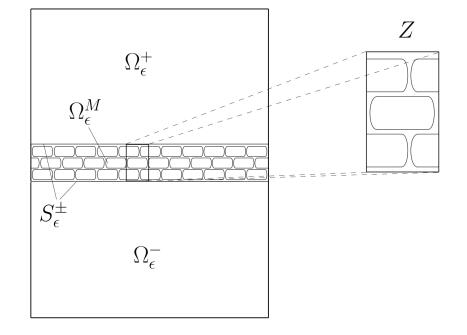
We consider a system of reaction-diffusion equations in a domain $\Omega$ consisting of two bulk regions $\Omega_\epsilon^+$ and $\Omega_\epsilon^-$, which are separated by a thin layer $\Omega_\epsilon^M$ with periodic heterogeneous structure. The thickness of the thin layer as well as the period and the size of its heterogeneities are of order $\epsilon>0$. Here, the parameter $\epsilon$ is much smaller than the length scale of the domain $\Omega$. The equations in the layer depend on $\epsilon$, the diffusion coefficients having the size $\epsilon^\gamma$ with $\gamma \in [-1, 1]$. On the interface $S_\epsilon^\pm$ between the bulk region $\Omega_\epsilon^\pm$ and the thin layer $\Omega_\epsilon^M$, we consider nonlinear transmission conditions formulated in terms of normal fluxes which are given by nonlinear functions of the solutions on both sides of $S_\epsilon^\pm$. Our aim is to derive effective models in the limit $\epsilon \to 0$.

In the limit, the thin layer reduces to an interface $\Sigma$ between the bulk domains $\Omega^\pm$. The processes in the bulk are again described by a system of nonlinear reaction-diffusion equations, and the main challenges of the paper are related to the derivation of effective interface conditions across $\Sigma$. To a certain extent, the effective interface conditions preserve the form of the microscopic ones in the sense that they involve the normal fluxes of the macroscopic solutions at $\Sigma$. These fluxes are given by nonlinear functions of the solutions in the respective bulk domain and the homogenized limit of the microscopic solutions in the thin layer. It turns out that the equations satisfied by the latter depend on the values of the parameter $\gamma$. More precisely, for $\gamma = 1$ the homogenized problem in the layer is formulated on the standard periodicity cell, for $\gamma \in (-1, 1)$, we obtain a nonlinear system of ordinary differential equations on $\Sigma$, whereas for $\gamma = -1$ a system of nonlinear reaction-diffusion equations on $\Sigma$ arises. In all three cases, the homogenized problem in the layer is coupled to the macroscopic solutions in the bulk domains $\Omega^\pm$.

The rigorous derivation of interface conditions for multi-scale and multi-physics problems is a field that is just at the beginning. More and better analytical multi-scale tools are required to treat the arising nonlinear systems in many applications (e.g. biology, material sciences or geosciences, to mention just few of them). Our paper is one of the first steps in this process. The effective model derived here is a non-standard micro-macro strongly coupled system of nonlinear equations, involving the bulk-regions, the separating interface and the so called cell problem which takes care of the microscopic processes in the thin layer.

The derivation of effective interface conditions is based on two-scale convergence for thin heterogeneous layers, and a Kolmogorov-type compactness result for Banach valued functions, applied to the unfolded sequence in the layer. Compared to previous contributions (see [14,15] for $\gamma = 1$ and [10] for $\gamma \in [-1, 1)$), where continuous transmission conditions for the microscopic solutions and their normal fluxes were considered at the interfaces $S_\epsilon^\pm$, we deal here with additional difficulties induced by nonlinear transmission conditions at $S_\epsilon^\pm$. More precisely, we have nonlinear terms on the interfaces $S_\epsilon^\pm$, and less regularity in time for the solutions. To cope with these problems, additionally to the techniques developed in [14,15,10], we make use of the auxiliary function given by the average with respect to the $n$-th spatial variable in the layer, and the averaging operator for thin domains, which is the adjoint of the unfolding operator in the layer. The averaged function is used in case $\gamma \in [-1, 1)$ as an approximation for the solution in the layer. Its properties allow the application of classical compactness results (Aubin-Lions-lemma for $\gamma = -1$, and classical Kolmogorov-criterion for $\gamma \in (-1, 1)$). However, to apply the latter, we have to introduce equivalent norms on the Sobolev space $H^1(\Omega_\epsilon^M)$, which are well adapted to the thin layer and to different choices of $\gamma$. The averaging operator for thin domains is used in case $\gamma = 1$ to prove that the time derivative of the unfolded sequence in the layer exists in a weak sense, and that it can be controlled by the time derivative of the solution itself. This allows to show that the assumptions of the Kolmogorov-type compactness result for Banach valued functions, see [8], are fulfilled, which gives strong convergence of the unfolded sequence. We emphasize that also in this case, the scaled Sobolev spaces mentioned above are needed.

The investigation of processes in domains separated by thin layers with periodic microstructure can also be found in elasticity problems, see e.g., [11,13] where the heterogeneous structure is described by periodically varying constitutive properties, or [12], where two domains separated by a thin layer made of periodic vertical beams are considered. Further applications can be encountered in fluid flow through thin filters, built up by an array of obstacles, see e.g., [3]. In [17], a reactive transport model with an additional convective contribution in the thin layer and a nonlinear transmission condition of Dirichlet type at the bulk-layer interface was considered. In the latter, however, a thin homogeneous layer was considered. In [4], long and horizontally arranged inclusions, only connected in one direction, were considered for a linear reaction-diffusion problem, and a concept of two-scale convergence adapted to this special structure was introduced.

This paper is organized as follows: In Section 2, we introduce the microscopic model, and establish existence and uniqueness of a weak solution. In Section 4, we derive estimates for the microscopic solutions necessary for the derivation of strong compactness results. The averaged function is defined in Section 5 and some basic properties are established. In Section 6 the averaging operator for thin domains is defined and the commuting property of the generalized time derivative and the unfolding operator is proved. Section 7 contains our main results. First, we prove compactness results for the microscopic solutions, especially the strong convergences in the thin layer. These are then used for the derivation of macroscopic models, including the effective interface conditions. In the A, we briefly recapitulate the concepts of two-scale convergence and unfolding operator for thin domains, together with related basic results.

2.   The microscopic model

We consider the domain $\Omega : = \Sigma \times (-H, H) \subset \mathbb{R}^n$ with fixed $H>0$, $n\geq 2$, and $\Sigma$ is a bounded and connected Lipschitz domain in $\mathbb{R}^{n-1}$. Further, let $\epsilon >0$ be a sequence with $\epsilon^{-1} \in \mathbb{N}$. The set $\Omega$ consists of three subdomains given by

see Figure 1. The domains $\Omega_\epsilon^\pm$ and $\Omega_\epsilon^M$ are separated by an interface $S_\epsilon^\pm$i.e.,

hence, we have $\Omega = \Omega_\epsilon^+ \cup \Omega_\epsilon^- \cup \Omega_\epsilon^M \cup S_\epsilon^+ \cup S_\epsilon^-$. As mentioned above, for $\epsilon \to 0$ the membrane $\Omega_\epsilon^M$ reduces to an interface $\Sigma \times \{0\}$, which we also denote by $\Sigma$ suppressing the $n$-th component, and we define

To describe the microscopic structure of $\Omega_\epsilon^M$, we introduce the standard cell $Z$ given by

and denote the upper and lower boundary of $Z$ by

The vector valued functions $u_\epsilon^\pm : (0, T)\times \Omega_\epsilon^\pm \rightarrow \mathbb{R}^m$ and $u_\epsilon^M : (0, T) \times \Omega_\epsilon^M \rightarrow \mathbb{R}^m$ are the solutions of

for $i = 1, \ldots, m$, and

for $\gamma \in [-1, 1]$ and $i = 1, \ldots, m$.

Thin heterogeneous layers occur in many applications, e.g., biology and engineering, and for every specific situation appropriate transmission conditions between the layer domain and the bulk regions are required. In previous contributions (see [14,15] for $\gamma = 1$ and [10] for $\gamma \in [-1, 1)$), the authors considered continuous transmission conditions for the microscopic solutions and their normal fluxes at the interfaces $S_\epsilon^\pm$. However, in many applications the concentrations are discontinuous at the interfaces between different regions and the normal fluxes across these interfaces are controlled by traces of the concentrations in the neighboring regions. In the present paper, this situation is modelled, with the help of the nonlinear transmission conditions at $S_\epsilon^\pm$. Furthermore, as can be seen from the methods used in our paper, we now have the opportunity to mix up the nonlinear Neumann transmission conditions and the continuous transmission conditions on $S_\epsilon^\pm$ for different species, and thus to extend the range of applications which can be investigated using multiscale techniques, see also Remark 5 (ⅱ). Finally, let us also mention that the scaling of the equations in the layer allows for a wide range of diffusion coefficients, and is motivated by the different roles played by the layers in different applications.

Assumptions on the data:

A1) For $i = 1, \ldots, m$ it holds that $D_i^{\pm}>0$ and $D_i^M \in L_{\rm{per}}^{\infty}\big(Y, L^{\infty}(-1, 1)\big)$. Further, we set $D_{i, \epsilon}^M(x): = D_i^M\left(\frac{x}{\epsilon}\right)$ and $D_i^M$ is strictly positive almost everywhere.

A2) The function $f^{\pm} = (f_1^{\pm}, \ldots, f_m^{\pm}): \mathbb{R}^m \rightarrow \mathbb{R}^m$ is Lipschitz continuous. This ensures the existence of a constant $C>0$ such that

A3) The function $g = (g_1, \ldots, g_m): \overline{Y} \times [-1, 1] \times \mathbb{R}^m \rightarrow \mathbb{R}^m$ is continuous, uniformly Lipschitz continuous with respect to the last variable, and $Y$-periodic with respect to the first variable. Especially,

We shortly write $y = ({\bar{y}}, y_n)$ and define $g_{\epsilon}(t, x, z) : = g\left(t, \frac{x}{\epsilon}, z\right)$.

A4) The function $h^{\pm} = (h_1^{\pm}, \ldots, h_m^{\pm}): \mathbb{R}^m \times \mathbb{R}^m \rightarrow \mathbb{R}^m$ is Lipschitz continuous, especially, there exists a constant $C>0$ such that

A5) The function $h^{M, \pm } = (h_1^{M, \pm}, \ldots, h_m^{M, \pm}): \overline{Y} \times \mathbb{R}^m \times \mathbb{R}^m \rightarrow \mathbb{R}^m$ is continuous, $Y$-periodic with respect to the first variable, and uniformly Lipschitz continuous with respect to the second and the third variablei.e., for a constant $C>0$ it holds that

A6) For the initial functions we assume $U_0^{\pm} \in L^2(\Omega^{\pm})^m$ and $U_0^M\in L^2(\Sigma \times (-1, 1))^m$.

In the following, we denote for an arbitrary open set $U\subset \mathbb{R}^n$ the duality pairing $\langle \cdot , \cdot \rangle_{H^1(U)^{\prime}, H^1(U)}$ by $\langle \cdot, \cdot \rangle_U$, and the inner product $(\cdot, \cdot)_{L^2(U)}$ by $(\cdot, \cdot)_U$.

Definition 2.1. We call $u_\epsilon: = (u_\epsilon^+, u_\epsilon^M, u_\epsilon^-)$ with $u_\epsilon^\pm : (0, T)\times \Omega_\epsilon^\pm \rightarrow \mathbb{R}^m$ and $u_\epsilon^M : (0, T) \times \Omega_\epsilon^M \rightarrow \mathbb{R}^m$ a weak solution of the Problem (1), if

and for all test-functions $\phi^{\pm} \in H^1(\Omega_\epsilon^\pm)$ and $\phi^M \in H^1(\Omega_\epsilon^M)$, and almost every $t \in (0, T)$ it holds that

together with the initial conditions (1d) and (1h).

First of all, we establish the existence of a unique solution using a fix-point argument. The idea is standard, therefore we only give a short sketch of the proof.

Proposition 1. For every $\gamma \in [-1, 1]$ there exists a unique weak solution $u_\epsilon$ of the Problem (1).

Proof. Uniqueness follows by standard energy estimates. For the existence, we use Schäfer's fixed point theorem on the space

with $X^{\ast}: = L^2((0, T), H^{\beta}(\Omega_{\epsilon}^{\ast}))^m$ for $\ast \in \{+, -, M\}$ and $\beta \in \left(\frac{1}{2} , 1\right)$ fixed, where on $X$ we define the operator $\mathcal{F}:X \rightarrow X$ by $\mathcal{F}(\bar{u}) = u$ and $u$ is the unique weak solution of the linearized problem of (1), where we replace the functions in the arguments of the nonlinearities on the right-hand side by the function $\bar{u}$. The existence of $u$ is ensured by the Galerkin-method. The continuity and compactness of the operator $\mathcal{F}$ is based on the compactness of the embedding

and similar estimates as in Lemma 4.2 below.

3.   Main results

Our aim is to derive macroscopic approximations for the microscopic solutions $u_\epsilon$ by passing to the limit $\epsilon \to 0$ in the variational equation (2). Hereby, we use multi-scale techniques adapted to the thin layers with microscopic structure, like the two scale-convergence and the unfolding operator for thin domains. The definitions and a brief overview on results related to this concepts are given in Appendix A.

In this section, we point out main steps in this process, and indicate the challenging aspects together with our original contributions. Eventually, we present the macroscopic models (which differ for different values of the parameter $\gamma$) obtained in the limit $\epsilon \to 0.$

3.1.   Estimates of the microscopic solutions

To pass to the asymptotic limit for $\epsilon \to 0,$ we use compactness results based on estimates for $u_\epsilon$. Firstly, we prove energy estimates, see Lemma 4.2, by using rather standard techniques. More challenging are, however, the estimates for the difference between the solutions and their shifts, which are used to prove strong convergence results for the solution $u_\epsilon^M$ in the thin layer and its traces on $S_\epsilon^\pm$, by means of Kolmogorov-type theorems. The estimates for shifts with respect to the spatial variable $x$ are given in Lemma 4.3. Similar estimates can be found in previous works of the authors, e.g., [15,10]. To estimate the shifts with respect to the time variable, see Theorem 7.3, we introduce the equivalent norm

on $H^1(\Omega_\epsilon^M)$, and estimate the solution in the layer and its time deriative with respect to this norm, see Lemma 4.4.

3.2.   The averaged function in thin domains

Our next step is to prove compactness results for the microscopic solutions, especially in the thin layer. We treat differently the cases $\gamma \in [-1, 1)$ and $\gamma = 1$. For $\gamma \in [-1, 1)$, we approximate the solution in the layer by its average with respect to the variable $x_n$, i.e., we define for $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$ the function $\bar{u}_\epsilon \in L^2((0, T)\times \Sigma)$ via

In Section 5, we provide estimates for the averaged function in thin domains and estimate the difference between the averaged function and the microscopic solution $u_\epsilon^M$ on the domain $\Omega_\epsilon^M$ and on the boundaries $S_\epsilon^\pm$. Based on these results, we establish the relation between the weak limit of $\bar{u}_\epsilon$ and the two-scale limit of $u_\epsilon^M$ in the layer, see (13) and show a regularity result with respect to time for the two-scale limit $u_0$, see Proposition 3. Eventually, we prove a strong compactness result for $\gamma = 1$, see Proposition 4. In conclusion, we can say that the averaged function provides an elegant and powerful tool for showing compactness results with respect to strong two-scale convergence in the thin layer. We emphasize however, that a main ingredient in the proof of the compactness result was the fact that for $\gamma \in [-1, 1)$, the two-scale limit of $u_\epsilon^M$ is independent of the microscopic variable $y$.

3.3.   The averaging operator in thin domains and the time derivative of the unfolded sequence

In the critical case $\gamma = 1$, the two-scale limit of $u_\epsilon^M$ in general depends on $y$, and the averaged function in the thin layer is not any longer a good approximation for $u_\epsilon^M$. To show strong compactness results in this case, we use the unfolded sequence in the layer together with a Kolmogorov-typ compactness result for Banach valued functions, see [8]. This requires some regularity of the time derivative of the unfolded sequence $\mathcal{T}_\epsilon^M u_\epsilon^M$. To get rid with this challenging task, in Section 6 we introduce the so called averaging operator for thin domains $U_\epsilon^M$, see also [6] for general domains. It turns out that $\frac{1}{\epsilon} U_\epsilon^M$ is the formal adjoint of the unfolding operator $\mathcal{T}_\epsilon^M.$ Based on the properties of the averaging operator, we are able to show a regularity result with respect to time of the unfolded sequence in the layer, see Proposition 7, and then to estimate $\partial_t \mathcal{T}_\epsilon^M u_\epsilon^M$ by the time derivative of the function $u_\epsilon^M$. Here again the equivalent norm (3) is required, see Proposition 8.

3.4.   Derivation of the macroscopic problems

Using the results from the previous sections, in Section 7 we give the proofs for our main results: The convergences of the microscopic solutions, see Theorem 7.1, 7.3, and 7.5, as well as the macroscopic models. The latter consist of equations in the bulk regions $\Omega^{\pm}$ and effective interface laws at $\Sigma$, and can be found in Theorem 3.1 (for the case $\gamma = -1$), Theorem 3.2 (for the case $\gamma \in (-1, 1)$) and Theorem 3.3 (for the case $\gamma = 1$), which we formulate in the following:

Theorem 3.1. Let $\gamma = -1$ and let $u_\epsilon$ be the solution of Problem (1). Let $u_0^{\pm}$ and $u_0^M$ be the limit functions from Proposition 9 and Theorem 7.1. Then

and $(u_0^{\pm}, u_0^M)$ is the unique weak solution of

for $i = 1, \ldots, m$, and

where the homogenized diffusion matrix $D_i^{M, \ast} \in \mathbb{R}^{(n-1)\times (n-1)}$ is given by

and the $w_{i, j}$ are the solutions of the cell-problems

Theorem 3.2. For $\gamma \in (-1, 1)$, let $u_\epsilon$ be the solution of Problem (1), $u_0^{\pm}$ and $u_0^M$ are the limit functions from Proposition 9 and Theorem 7.3. Then

and $(u_0^{\pm}, u_0^M)$ is the unique weak solution of

for $i = 1, \ldots, m$, and

Theorem 3.3. Let $u_\epsilon$ be the solution of Problem (1) for $\gamma = 1$, $u_0^{\pm}$ and $u_0^M$ are the limit functions from Proposition 9 and Theorem 7.5. Then

and $(u_0^{\pm}, u_0^M)$ is the unique weak solution of

for $i = 1, \ldots, m$, and $(\partial^{\pm} Z: = \partial Z \setminus (S^+ \cup S^-))$

4.   Estimates of the microscopic solutions

Our aim is to derive macroscopic approximations for the microscopic solutions $u_\epsilon$ by passing to the limit $\epsilon \to 0$ in the variational equation (2). For this purpose, we use compactness results based on estimates for $u_\epsilon$. A main issue in the proof of these estimates is to exhibit the precise dependence on the parameters $\epsilon$ and $\delta$.

Throughout this paper, we will frequently use the following trace estimate for thin domains.

Lemma 4.1. For $u_\epsilon \in H^1(\Omega_\epsilon^M)$ and every $\theta > 0$ it holds that

with a constant $C(\theta)>0$.

Proof. There exists an extension $\tilde{u}_\epsilon \in H^1(\mathbb{R}^{n-1} \times (-\epsilon, \epsilon))$ of $u_\epsilon$, such that

with a constant $C^{\ast}>0$ independent of $\epsilon$. This follows from the fact that we extend the function $u_\epsilon$ only with respect to the first $(n-1)$ components and fix the last one. Now, define $R_{\epsilon}: = R\times (-\epsilon, \epsilon)$, such that $R\subset \mathbb{R}^{n-1}$ is a rectangle with integer corner points and $\Sigma \subset R$. By a decomposition argument for $R_{\epsilon}$, we obtain for arbitrary $\theta >0$

In a first step, we obtain the following estimates:

Lemma 4.2. The solution $u_\epsilon$ of Problem (1) fulfills the following a priori estimates for a constant $C>0$ independent of $\epsilon$

for $i = 1, \ldots, m$.

Proof. Test the variational equation (2) for $u_{i, \epsilon}^\pm$ with $u_{i, \epsilon}^\pm$ and use the growth conditions for $f_i^{\pm}$ and $h_i^\pm$ from our assumptions to obtain almost everywhere in $(0, T)$

for an arbitrary $\delta >0$, where we have used the scaled trace estimate from Lemma 4.1. Now, testing the equation (2) for $u_{i, \epsilon}^M$ with $u_{i, \epsilon}^M$ and using similar arguments as above we get

for an arbitrary $\delta>0$. Using the identity $\langle \partial_t u_{i, \epsilon}^\pm, u_{i, \epsilon}^\pm\rangle_{\Omega_\epsilon^\pm} = \frac{1}{2}\frac{d}{dt} \|u_{i, \epsilon}^\pm\|^2_{L^2(\Omega_\epsilon^\pm)}$ and a similar result for the function $u_{i, \epsilon}^M$, we obtain from the both inequalities above by summing over $i = 1, \ldots, m$, choosing $\delta$ small enough (so the terms including $\delta$ can be absorbed by the left-hand side), the positivity of $D_i^M$, and $\epsilon \le \epsilon^{\gamma}$ for small $\epsilon$, the inequality

Integrating with respect to time from $0$ to $t \in (0, T)$ gives us

The assumptions for the initial conditions and Gronwall's inequality imply

and together with the inequality above, we obtain the estimates for the gradients in (5a) and (5b). It remains to prove the estimates for the time derivative. Therefore we test the variational equation (2) for $u_{i, \epsilon}^\pm$ with $v^{\pm} \in H^1(\Omega_\epsilon^\pm)$, such that $\|v^{\pm}\|_{H^1(\Omega_\epsilon^\pm)}\le 1$ and obtain with similar arguments as above

Using again the trace estimate from Lemma 4.1, the boundedness of $v^{\pm}$, and the definition of the operator norm in $H^1(\Omega_\epsilon^\pm)^{\prime}$, we get for almost every $t \in (0, T)$

Integration with respect to time and the inequalities (5a) and (5b) imply the second inequality in (5c). For the first inequality, we choose $v_\epsilon \in H^1(\Omega_\epsilon^M)$ with $\|v_\epsilon\|_{H^1(\Omega_\epsilon^M)} \le 1$ as a test function for the variational equation in the membrane and obtain

Then, using the trace inequality and the boundedness of $v_\epsilon$, we get

Integration with respect to time and the a priori estimates (5a) and (5b) give

This gives us the last inequality and the proof is complete.

The above estimates are not sufficient for the derivation of appropriate strong convergence results for the solution $u_\epsilon^M$ in the thin layer and its traces on $S_\epsilon^\pm$. Such results are, however, needed to pass to the limit $\epsilon \to 0$ in the nonlinear terms in the variational equation (2). We will show strong convergence by means of Kolmogorov-type theorems. These rely on the estimates for the difference between the solutions and their shifts given in the next lemma.

Since we are operating in bounded domains, we have to make sure that the shifts are well-defined. For an arbitrary domain $U \subset \mathbb{R}^d$, we define for $h>0$ the set

Further, we write

In the same way as in (21) (see Appendix A), we define the sets $K_{\epsilon, h}$, $\hat{\Sigma}_{\epsilon, h}$, $\Lambda_{\epsilon, h}$, $\hat{\Omega}_{\epsilon, h}^M$, and $\Lambda_{\epsilon, h}^M$, by replacing $\Sigma$ with $\Sigma_h$. For an arbitrary function $v \in L^2(\Omega)$ we define for $l \in \mathbb{Z}^{n-1}$ small and almost every $x \in \Sigma_h \times (-H, H)$

In the following, we suppress the index $l$ on the left-hand side for an easier notation, but we should keep in mind the dependence on this parameter. The following estimate for the shifts $\delta u_\epsilon^M$ hold:

Lemma 4.3. Let $u_\epsilon$ be the solution of the Problem (1) with $\gamma \in [-1, 1]$. Then for every $0 < h \ll 1$, $l \in \mathbb{Z}^{n-1}$ with $\epsilon|l|<h$ there exists a constant $C = C(h)$, such that for almost every $t \in (0, T)$ the following estimate is valid:

Proof. Let $\eta \in C_0^{\infty}(\Sigma_h)$ be a cut-off function with $0 \le \eta \le 1$ and $\eta = 1$ in $\Sigma_{2h}$. For all $\phi \in H^1(\Omega_\epsilon^M)$ and almost everywhere in $(0, T)$, we have the following variational equality for $\delta u_{i, \epsilon}^M$:

with

where $u_{\epsilon, l}^{\ast}(t, x) : = u_{\epsilon}^{\ast}(t, x+ \epsilon(l, 0))$ for $\ast \in \{+, -, M\}$. Here, we extended $u_\epsilon^M$ by zero outside $\Omega_\epsilon^M$, but this has no influence due to the cut-off function $\eta$. Now, choose $\phi = \delta u_{i, \epsilon}^M$ as a test function, to obtain for a constant $c_0>0$

Now, we integrate with respect to time and estimate the terms on the right-hand side. From the Lipschitz continuity of $g$ we obtain for the first term

For the second term, our a priori estimates imply

Using the Lipschitz continuity of $h_i^{M, \pm}$, the scaled trace estimates for thin domains from Lemma 4.1, and the a priori estimates, we obtain for arbitrary $\theta > 0$

Altogether, we obtain for arbitrary $\theta >0$ and almost every $t\in (0, T)$ the estimate

In a similar way, we get

Adding up all these inequalities, choosing $\theta$ small enough, such that the terms on the right-hand side containing $\theta$ can be absorbed by the left-hand side, and using Gronwall's inequality, we get the desired result.

Finally, we give further estimates for the solution in the thin layer and its time derivative with respect to equivalent norms, which are introduced on $H^1(\Omega_\epsilon^M)$. These norms are well adapted to the thin layer structure and the special choice of $\gamma$, and the estimates in these norms are used especially to to control the time derivative of the unfolded sequence, see Proposition 8, and to estimate the difference of solutions and their shifts with respect to time, see Theorem 7.3. Thus, let us define

together with the inner product

i.e., the norm $\|v_\epsilon\|_{H_{\epsilon, \gamma}}^2 = \frac{1}{\epsilon}\|v_\epsilon\|^2_{L^2(\Omega_\epsilon^M)} + \epsilon^{\gamma} \big\|\nabla v_\epsilon\big\|^2_{L^2(\Omega_\epsilon^M)}$. Of course, the norm $\|\cdot\|_{H_{\epsilon, \gamma}}$ is equivalent to the usual norm $\|\cdot\|_{H^1(\Omega_\epsilon^M)}$ with equivalent-constants depending on $\epsilon$.

Remark 1. We consider the Gelfand-triple $H_{\epsilon, \gamma} \subset L^2(\Omega_\epsilon^M) \subset H_{\epsilon, \gamma}^{\prime}$ under the natural embedding of $H_{\epsilon, \gamma}$ in $L^2(\Omega_\epsilon^M)$. Then for the microscopic solution in the layer, we have $u_\epsilon^M \in H^1((0, T), H_{\epsilon, \gamma}^{\prime})$ with $\langle \partial_t u_\epsilon^M , \phi_{\epsilon} \rangle_{H_{\epsilon, \gamma}^{\prime}, H_{\epsilon, \gamma}} = \langle \partial_t u_\epsilon^M , \phi_{\epsilon} \rangle_{\Omega_\epsilon^M}$ for all $\phi_{\epsilon} \in H_{\epsilon, \gamma}$.

For the solution $u_\epsilon^M$ as a function in $L^2((0, T), H_{\epsilon, \gamma})\cap H^1((0, T), H_{\epsilon, \gamma}^{\prime})$, we obtain the following a priori estimates which are independent of $\gamma$.

Lemma 4.4. Let $u_\epsilon$ be the solution of Problem (1) for $\gamma \in [-1, 1]$. Then, it holds that

Proof. The first inequality follows directly from Lemma 4.2. For the second inequality, we choose $v_\epsilon \in H_{\epsilon, \gamma}$ with $\|v_\epsilon \|_{H_{\epsilon, \gamma}} = 1$i.e., we have

Inequality (6) from the proof of Lemma 4.2 is still valid for this $v_\epsilon$. Using the inequalities above, we obtain

Squaring, integration with respect to time, and Lemma 4.2 give us the desired result.

5.   The averaged function in thin domains

The aim of this section is to provide general results for the averaged function in thin domains, obtained by taking the average over the $n$-th component. This function will be used as an auxiliary function to obtain appropriate strong convergence results for the solution $u_\epsilon^M$ in the case $\gamma \in [-1, 1)$. We define for $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$ the function $\bar{u}_\epsilon \in L^2((0, T)\times \Sigma)$ via

Here, we consider a sequence $u_\epsilon\in L^2((0, T), H^1(\Omega_\epsilon^M))\cap H^1((0, T), H^1(\Omega_\epsilon^M)^{\prime})$, such that

The following estimates hold for the averaged sequence $\bar{u}_\epsilon$ and its derivatives.

Lemma 5.1. It holds that $\bar{u}_\epsilon \in L^2((0, T), H^1(\Sigma)) \cap H^1((0, T), H^1(\Sigma)^{\prime})$, such that

Further, we have

Proof. It is clear that $\bar{u}_\epsilon \in L^2((0, T), H^1(\Sigma))$, so we have to check inequalities (12a) and (12b). We have

In a similar way, we obtain

Now, we consider the time derivative of $\bar{u}_\epsilon$. First of all, we have for all $\psi \in \mathcal{D}(0, T)$, and $\phi \in H^1(\Sigma)$ with constant extension in $x_n$-direction:

i.e., we have $\partial_t \bar{u}_\epsilon \in L^2((0, T), H^1(\Sigma)^{\prime})$ with

Additionally, since $\sqrt{2\epsilon} \|\phi\|_{H^1(\Sigma)} = \|\phi\|_{H^1(\Omega_\epsilon^M)}$, we immediately obtain the following estimates almost everywhere in $(0, T)$

Squaring, integration with respect to time, and (11) gives inequality (12c).

It remains to prove estimate (12d). We obtain with the fundamental theorem of calculus

In the following proposition, we compare the $L^2$-norm on $\Sigma$ of the traces of $u_\epsilon$ on $S_\epsilon^\pm$ and the averaged function $\bar{u}_\epsilon$.

Lemma 5.2. It holds that

Proof. We only consider the trace on $S_\epsilon^+$. Again from the fundamental theorem of calculus, we obtain

Remark 2. From Lemma 5.1, we immediately obtain the existence of a function $\bar{u}o \in L^2((0, T)\times \Sigma)\cap H^1((0, T), H^1(\Sigma)^{\prime})$, such that up to a subsequence it holds that

In Section 7, we will use the averaged function to prove convergence results for the solution $u_\epsilon^M$ in the layer. For this purpose, the following two propositions will be helpful.

Let $u_0 \in L^2((0, T)\times \Sigma \times Z)$ be the two-scale limit of $u_\epsilon$, which exists (up to a subsequence) due to (11) and Proposition 10.

Proposition 2. The following relation holds between the weak limit $\bar{u}o$ of $\bar{u}_\epsilon$ and the two-scale limit $u_0$ of $u_\epsilon$: For almost every $(t, \bar{x}) \in (0, T)\times \Sigma$, we have

Proof. For all $\phi \in L^2(\Sigma)$ and $\psi\in \mathcal{D}(0, T)$, we have

From $\bar{u}o \in H^1((0, T), H^1(\Sigma)^{\prime})$, we get the differentiability respect to time of the mean of $u_0$ over $Z$:

Proposition 3. We have $\frac{1}{|Z|} \int_Z u_0(\cdot_t, \cdot_{\bar{x}}, y) dy \in H^1((0, T), H^1(\Sigma)^{\prime})$ with

Proof. This follows easily from partial integration with respect to time. In fact, for all $\phi \in H^1(\Sigma)$, $\psi \in \mathcal{D}(0, T)$, we get

A straightforward consequence of Proposition 2 and Proposition 3 is given in the following

Corollary 1. If the two-scale limit $u_0$ does not depend on $y$, we have

For $\gamma = -1$, we obtain the following strong compactness result:

Proposition 4. Let $u_\epsilon\in L^2((0, T), H^1(\Omega_\epsilon^M))\cap H^1((0, T), H^1(\Omega_\epsilon^M)^{\prime})$, such that

Then, there exist $u_0 \in L^2((0, T), H^1(\Sigma))\cap H^1((0, T), H^1(\Sigma)^{\prime})$ and $u_1 \in L^2((0, T)\times \Sigma , \mathcal{H}_{\rm{per}}o)$, such that up to a subsequence it holds that

Proof. Proposition 10 implies that, up to a subsequence, $u_\epsilon$ converges in two-scale sense to a limit $u_0$, which is independent of the microscopic variable $y$. On the other hand, due to Lemma 5.1, the sequence $\bar{u}_\epsilon$ is bounded in $L^2((0, T), H^1(\Sigma))\cap H^1((0, T), H^1(\Sigma)^{\prime})$. The Aubin-Lions Lemma implies the strong convergence of $\bar{u}_\epsilon$ and the limit coincides with the two-scale limit $u_0$, see Corollary 1. Using now estimate (12d), it follows that $u_\epsilon$ converges even strongly in the two-scale sense to the limit $u_0$. The convergence of $\nabla u_\epsilon$ follows from Proposition 10(ⅲ). The strong convergence of the traces is a direct consequence of Lemma 5.2, and the weak convergence of $\partial_t \bar{u}_\epsilon$ is obtained from the boundedness of $\bar{u}_\epsilon$ in $H^1((0, T), H^1(\Sigma)^{\prime})$.

6.   The averaging operator in thin domains and the time derivative of the unfolded sequence

Lemma A.2 shows that the unfolded sequence $\mathcal{T}_\epsilon^M u_\epsilon$ inherits the regularity with respect to the microscopic variable $y\in Z$ from the function $u_\epsilon$. Now, we want to analyze if this is also true for the regularity with respect to the time variable. If we have $u_\epsilon \in H^1((0, T), L^2(\Omega_\epsilon^M))$, then an easy integration by substitution shows $\mathcal{T}_\epsilon^M u_\epsilon \in H^1((0, T), L^2(\Sigma \times Z))$ and we have almost everywhere in $(0, T)\times \Sigma \times Z$ the identity $\partial_t \mathcal{T}_\epsilon^M u_\epsilon(t, \bar{x}, y) = \mathcal{T}_\epsilon^M (\partial_t u_\epsilon) (t, \bar{x}, y)$. In our case, however, the microscopic solution $u_\epsilon^M \in H^1((0, T), H^1(\Omega_\epsilon^M)^{\prime})$ and it is not clear, whether the time derivative of the unfolded sequence $\mathcal{T}_\epsilon^M u_\epsilon^M$ exists in a weak sense, since a pointwise definition with respect to the spatial variable is not possible. In this section, we show that also in this case a time derivative exists in some weaker sense and it can be controlled by the time derivative of the function $u_\epsilon^M$ itself. Therefore, we introduce the so called averaging operator for thin domains $U_\epsilon^M$, see also [6] for general domains, and show that under a suitable restriction of the domain of definition, the averaging operator $U_\epsilon^M$ preserves the spatial regularity.

For $\hat{\Omega}_\epsilon^M$ and $\Lambda_{\epsilon}^M$ given in (21), see Appendix, and $\{x\}: = x - [x]$, we define

The following Lemma shows that $\frac{1}{\epsilon} U_\epsilon^M$ is the formal adjoint of the unfolding operator $\mathcal{T}_\epsilon^M$:

Proposition 5. Let $u_\epsilon \in L^2((0, T) \times \Omega_\epsilon^M)$ and $\phi \in L^2((0, T) \times \Sigma \times Z)$, then we have

Further, we have the identity $U_\epsilon^M\big(\mathcal{T}_\epsilon^M u_\epsilon\big) = u_\epsilon$ for all $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$.

Proof. The proof uses the same arguments as in [6,Proposition 4.3] and is skipped here.

As a corollary we immediately obtain the following inequality:

Corollary 2. For $\phi \in L^2((0, T)\times \Sigma \times Z)$ we have

From the definition of the operator $U_\epsilon^M$, we see that for a function $\phi \in L^2((0, T)\times \Sigma, H^1(Z))$, the function $U_\epsilon^M(\phi)$ is not an element of the space $L^2((0, T), H^1(\Omega_\epsilon^M))$. Already for $n = 2$ it is easy to find a counterexample. If we want more regularity of $U_\epsilon^M(\phi)$ with respect to the spatial variable, we have to restrict the domain of definition. We define the space

together with the usual $H^1(Z)$-normi.e., $\mathcal{H}_0$ is the space of functions from $H^1(Z)$ with trace equal to $0$ on the lateral boundary $\partial Z \setminus (S^+ \cup S^-)$.

Proposition 6. It holds $U_\epsilon^M : L^2((0, T)\times \Sigma, \mathcal{H}_0) \rightarrow L^2((0, T), H^1(\Omega_\epsilon^M))$, and for $\phi \in L^2((0, T)\times \Sigma, \mathcal{H}_0)$, we have almost everywhere in $(0, T)\times \Omega_\epsilon^M$

Proof. Let $\phi \in L^2((0, T)\times \Sigma, \mathcal{H}_0)$ and $u_\epsilon \in \mathcal{D}((0, T)\times \Omega_\epsilon^M)$. Then, with Proposition 5 and integration by parts, we obtain for $i = 1, \ldots, n$

The last term is equal to $0$, since $\phi$ vanishes on the lateral boundary of $Z$ and since $u_\epsilon$ has compact support in $\Omega_\epsilon^M$i.e., $\mathcal{T}_\epsilon^M u_\epsilon = 0$ on $S^{\pm}$. Hence, we obtain using again Proposition 5

which gives us the desired result.

We are now able to state our regularity result with respect to time for the unfolding operator.

Proposition 7. Let $u_\epsilon \in L^2((0, T) \times \Omega_\epsilon^M) \cap H^1((0, T), H^1(\Omega_\epsilon^M)^{\prime})$. Then we have

and it holds that

for almost every $t \in (0, T)$ and all $\phi \in L^2(\Sigma, \mathcal{H}_0)$.

Proof. Let $\phi \in L^2(\Sigma, \mathcal{H}_0)$ and $\psi \in \mathcal{D}(0, T)$. Due to Proposition 6, we have $U_\epsilon^M(\phi) \in H^1(\Omega_\epsilon^M)$. We obtain

what gives us the claim.

Our aim now is to estimate the norm of $\partial_t \mathcal{T}_\epsilon^M u_\epsilon$ by a suitable norm of $\partial_t u_\epsilon$. Due to the properties of $U_\epsilon^M$ from Corollary 2 and Proposition 6, we can see that it is appropriate to work with the scaled norm on $H_{\epsilon, 1}$. For this space, the averaging operator $U_\epsilon^M$ is a linear operator between the space $L^2((0, T)\times \Sigma, \mathcal{H}_0)$ and $L^2((0, T), H_{\epsilon, 1})$, such that its operator norm is independent of $\epsilon$:

Lemma 6.1. Let $\phi \in L^2((0, T)\times \Sigma, \mathcal{H}_0)$, then it holds

Proof. The claim follows immediately from Corollary 2 and Proposition 6.

As an easy consequence, we obtain the following estimate for the time derivative $\partial_t \mathcal{T}_\epsilon^M u_\epsilon$.

Proposition 8. Let $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M) \cap H^1((0, T), H^1(\Omega_\epsilon^M)^{\prime})$, then we have

Proof. Due to Lemma 6.1, we have $\big\|U_\epsilon^M(\phi)\big\|_{H_{\epsilon, 1}} \le 1$ for all $\phi \in L^2(\Sigma, \mathcal{H}_0)$ with $\|\phi\|_{L^2(\Sigma, \mathcal{H}_0)} = 1$. Hence, for almost every $t \in (0, T)$ we obtain for all $\phi \in L^2(\Sigma, \mathcal{H}_0)$ with $\|\phi\|_{L^2(\Sigma, \mathcal{H}_0)} = 1$

i.e., $\big\| \partial_t \mathcal{T}_\epsilon^M u_\epsilon(t) \big\|_{L^2(\Sigma, \mathcal{H}_0)^{\prime}} \le \frac{1}{\epsilon} \|\partial_t u_\epsilon(t)\|_{H_{\epsilon, 1}^{\prime}}$. Squaring and intgration with respect to time gives us the desired result.

7.   Derivation of the macroscopic problems

In this section, we derive convergence results for the sequences $u_\epsilon^\pm$ and $u_\epsilon^M$, which we then use for the derivation of the macroscopic problems. Compared with the results in [10,15], new challenges appear concerning the convergence of the solutions in the thin layer. Firstly, the time derivative of the solutions is no more bounded in the $L^2$-norm, but is only a functional pointwise in time. This causes difficulties especially for the derivation of strong compactness results, which are needed for passing to the limit in the nonlinear terms. Concerning the nonlinear terms, other than in [10,15], here, we have nonlinear terms at the interfaces $S_\epsilon^\pm$, which require the strong two-scale convergence of the traces $u_\epsilon^M|_{ S_\epsilon^\pm }$.

For the derivation of the convergence results for $u_\epsilon^M$ in the layer, we will use different approaches: For $\gamma = -1$ and $\gamma \in (-1, 1)$, when the limit function $u_0^M$ is independent of the microscopic variable $y$, we approximate the solution in the layer by its average $\bar{u}_\epsilon^M$, see (15) below. In the first case, the latter is bounded in $L^2((0, T), H^1(\Sigma))\cap H^1((0, T), H^1(\Sigma)^{\prime})$, and thus converges strongly, due to the Aubin-Lions Lemma. In the second case, the gradient $\nabla_\bar{x} \bar{u}_\epsilon^M$ is no longer bounded uniformly with respect to $\epsilon$. Here, we use the Kolmogorov compactness result, based on estimates for the shifts with respect to $t$ and $\bar x$. In the critical case $\gamma = 1$, when the two-scale limit in general depends on $y$, we use the unfolded sequence together with a Kolmogorov-type compactness result for Banach valued functions. To pass to the limit in the microscopic problem (2), we use the two-scale convergence for thin domains with heterogeneous structure, see Definition A.1 in A.

Let us start with the convergence results in the bulk domains. These are similar to those in [10,15], except for the time derivative, which is in this case only a functional pointwise in time. For the convergence of the time derivative, we transform the fixed domain $\Omega^{\pm}$ to the $\epsilon$-dependent set $\Omega_\epsilon^\pm$ via the transformation

and define $\tilde{u}_\epsilon^\pm(t, x): = u_\epsilon^\pm\big(t, \Phi_\epsilon^\pm(x)\big)$ for almost every $(t, x) \in (0, T)\times \Omega^{\pm}$. Integration by substitution gives us $\tilde{u}_\epsilon^\pm \in L^2((0, T), H^1(\Omega^{\pm}))^m\cap H^1((0, T), H^1(\Omega^{\pm})^{\prime})^m$ with

and especially we obtain with our a priori estimates from Lemma 4.2

Proposition 9. Let $u_\epsilon$ be the solution of Problem (1) for $\gamma \in [-1, 1]$. Then there exists $u_0^{\pm} \in L^2((0, T), H^1(\Omega^{\pm}))^m \cap H^1((0, T), H^1(\Omega^{\pm})^{\prime})^m$, such that up to a subsequence

Further, for all $\phi \in L^2((0, T), H^1(\Omega^{\pm}))$ it holds that

Proof. The convergences of $\chi_{\Omega_\epsilon^\pm} u_{i, \epsilon}^\pm$, $\chi_{\Omega_\epsilon^\pm} \nabla u_{i, \epsilon}^\pm$, the trace $\tilde{u}_{i, \epsilon}^\pm$, and the time derivative $\partial_t \tilde{u}_{i, \epsilon}^\pm$ follow the same lines as in [15]. The last convergence follows easily from the representation of $\partial_t \tilde{u}_{i, \epsilon}^\pm$ above.

After having established the convergence results for the sequences $u_\epsilon^\pm$, we concentrate on the sequence $u_\epsilon^M$ in the layer. Since different approaches are used for different values of the parameter $\gamma$, we consider each of these cases in a separate subsection, where after establishing the convergence results, we also derive the corresponding macroscopic problem.

Remark 3. In the following, we consider the traces $u_\epsilon^M|_{ S_\epsilon^\pm }$ and $u_\epsilon^\pm|_{ S_\epsilon^\pm }$ as functions in $L^2((0, T)\times \Sigma)$ instead of the $\epsilon$-dependent space $L^2((0, T)\times S_\epsilon^\pm )$, especially, we have $u_\epsilon^\pm|_{ S_\epsilon^\pm } = \tilde{u}_{i, \epsilon}^\pm|_{\Sigma}$.

7.1.   The case $\gamma = -1$

To prove the convergence results for the sequence $u_\epsilon^M$ and to pass to the limit in the microscopic equations, we will use as an auxiliary function the averaged function

introduced in Section 5.

Theorem 7. For $\gamma = -1$, there exist functions $u_0^M \in L^2((0, T), H^1(\Sigma))^m\cap H^1((0, T), H^1(\Sigma)^{\prime})^m$ and $u_1^M \in L^2((0, T)\times \Sigma , \mathcal{H}_{\rm{per}}o)^m$, such that up to a subsequence it holds that

Proof. This follows directly from Lemma 4.2 and Proposition 4.

From the strong convergences above, we immediately obtain the two-scale convergences of the nonlinear terms:

Corollary 3. For $\gamma = -1$, it holds up to a subsequence

Proof. The first convergence follows from Proposition 9. To show the second convergence, we start from

with $\phi \in C^0\big([0, T]\times \overline{\Sigma}, C_{\rm{per}}^0\big([0, 1]^{n-1}, C^0([-1, 1])\big)\big)$. Using the Lipschitz-continuity of the function $g$ and inequality (12d) corresponding to $u_\epsilon^M$, we obtain $\lim_{\epsilon \to 0}I_1^{\epsilon} = 0$. To estimate $I_2^{\epsilon}$, we proceed as follows

Thus, the strong convergence of $\bar{u}_\epsilon^M$ to $u_0^M$ in $L^2((0, T)\times \Sigma)$ implies $\lim_{\epsilon \to 0}I_2^{\epsilon} = 0$. Finally, the two-scale convergence of $g\left(\frac{\cdot}{\epsilon}, u_0^M\right)$ to $g\left(\cdot, u_0^M\right)$ yields the desired result. In a similar way the third and the last convergence follow, by using additionally the strong convergence of $u_\epsilon^\pm|_{ S_\epsilon^\pm }$ in $L^2((0, T)\times \Sigma)$ from Proposition 9.

Now, based on the above convergence results, we derive the macroscopic model from Theorem 3.1.

Proof of Theorem 3.1. To obtain the equations in the bulk domains, we test the variational equation (2) in the bulk with $\phi(x) \psi(t)$, where $\phi \in \mathcal{D}\big(\overline{\Omega^{\pm}}\big)$ and $\psi\in \mathcal{D}(0, T)$. Integration with respect to time, using Proposition 9, Theorem 7.1, Corollary 3, and a density argument, we can go to the limit $\epsilon \to 0$, and obtain the weak formulation for $u_0^{\pm}$.

Testing the variational equation (2) of $u_{i, \epsilon}^M$ with $\phi_{\epsilon}(t, \bar{x}): = \epsilon\psi(t)\phi(\bar{x}) \theta\left(\frac{x}{\epsilon}\right)$ and $\psi \in \mathcal{D}(0, T)$, $\phi \in \mathcal{D}\big(\overline{\Sigma}\big)$, and $\theta \in \mathcal{H}_{\rm{per}}$, we get after integrating with respect to time

Due to our a priori estimates, all the terms except the diffusion therm involving $\nabla_y \theta$ are of order $\epsilon$. Hence, from the two-scale convergence of $\nabla u_{i, \epsilon}^M$ in Theorem 7.1, we obtain for $\epsilon \to 0$

This implies almost everywhere in $(0, T)\times \Sigma \times Z$

where $w_{i, j}$ is the unique weak solution of the cell problem (4).

Now, we choose as a test function $\psi(t)\phi(\bar{x})$, with $\psi$ and $\phi$ as above. After integration with respect to time and using the properties of $\bar{u}_\epsilon$ from Section 5, we obtain

From Theorem 7.1, Corollary 3, and the identity (17), we get for $\epsilon \to 0$

Since $\mathcal{D}\big(\overline{\Sigma}\big)$ is dense in $H^1(\Sigma)$, this gives us the weak formulation for the problem of $u_0^M$. The initial condition is obtained by similar arguments, where we have to choose test function $\psi \in \mathcal{D}\big([0, T)\big)$ and use integration by parts in the term involving the time derivative. Uniqueness is standard.

7.2.   The case $\gamma \in (-1, 1)$

Again, we use the averaged function $\bar{u}_\epsilon^M$. However, now, the gradient $\nabla_\bar{x} \bar{u}_\epsilon^M$ is no longer bounded uniformly with respect to $\epsilon$i.e., we are not able to apply the Aubin-Lions Lemma to obtain the strong convergence of $\bar{u}_\epsilon^M$. Instead, we apply the Kolmogorov compactness theorem [5,Theorem 4.26]. To cope with the shifts with respect to time, we make use of the following embedding of Nikolskii-type, applied to the Hilbert space $H_{\epsilon, \gamma}$ with its corresponding norm.

Lemma 7.2. Let $V$ and $H$ be Hilbert spaces and we assume that $(V, H, V^{\prime})$ is a Gelfand triple. Let $v \in L^2((0, T), V) \cap H^1((0, T), V^{\prime})$. Then, for every $\phi \in V$ and almost every $t \in (0, T)$, $h\in(-T, T)$, such that $t + h \in (0, T)$, we have

Especially, it holds that

Proof. The proof follows the same lines as the proof for the special case $V = H^1(\Omega)$ and $H = L^2(\Omega)$, see [9,Lemma 9].

Theorem 7.3. For $\gamma \in (-1, 1)$, let $u_\epsilon$ be the solution of Problem (1). Then, there exists $u_0^M \in L^2((0, T)\times \Sigma)^m\cap H^1((0, T), H^1(\Sigma)^{\prime})^m$, such that up to a subsequence it holds for all $p \in [1, 2)$, that

Proof. As in Proposition 7.1, Lemma 4.2 and Proposition 10 imply that, up to a subsequence, $u_\epsilon^M$ converges in two-scale sense to a limit $u_0^M$, which is independent of the microscopic variable $y$. Concerning the averaged sequence $\bar{u}_\epsilon^M$, we have that due to Lemma 5.1, it is bounded in $L^2((0, T) \times \Sigma)$. Thus, up to a subsequence, $\bar{u}_\epsilon^M$ converges weakly and the limit coincides with the two-scale limit $u_0^M$. The next step in the proof is to show the strong convergence of $\bar{u}_\epsilon^M$ in $L^p((0, T)\times \Sigma)$ by using the Kolmogorov compactness criterion.

We extend all functions outside their domain of definition by zero. Since $\bar{u}_\epsilon^M$ is bounded in $L^2((0, T)\times \Sigma)\subset L^p((0, T)\times \Sigma)$, to apply the Kolmogorov compactness result, see [5,Theorem 4.26], it is enough to show

uniformly with respect to $\epsilon$. Therefore, we show the following two statements:

(ⅰ) For all $h>0$ fixed, we have

(ⅱ) It holds that

For the definition of the domains $(0, T)_h, \Sigma_h$ and $\Omega_\epsilon^Mh$, see (7) and (8). Condition (ⅱ) is an easy consequence of the Hölder-inequality and the boundedness of $\bar{u}_\epsilon^M$ in $L^2((0, T)\times \Sigma)$. In fact, we have

For condition (ⅰ), due to the triangle inequality, it is enough to consider shifts separately with respect to the variable $t$ and $\bar{x}$. We fix $h>0$, and obtain for $|\bar{\xi}| <h$

Due to the mean-value theorem and the a priori estimates for $u_\epsilon^M$, the first term is of order $\epsilon^{\frac{1-\gamma}{2}}$ and therefore tends to zero for $\epsilon \to 0$. The second term goes to zero, due to Lemma 4.3 for $\epsilon, \bar{\xi} \to 0$. The uniform convergence with respect to $\epsilon$ is then obtained from the convergence of the difference of the shifts to $0$ for $\bar{\xi} \to 0$ for every fixed $\epsilon$.

Now, we have to consider shifts with respect to time. From Lemma 4.4, we have

Hence, Lemma 7.2 with $V = H_{\epsilon, \gamma}$ and $H = L^2(\Omega_\epsilon^M)$ implies for $|s| < h$

This gives us the strong convergence of $\bar{u}_\epsilon^M$ in $L^p((0, T)\times \Sigma)$. Then, the strong converges of the traces $u_\epsilon^M|_{ S_\epsilon^\pm }$ follow by Lemma 5.2 and the triangle inequality. The convergence of the time derivative follows since the sequence is bounded in $L^2((0, T), H^1(\Sigma)^{\prime})$.

Again, we obtain the convergences for the nonlinearities.

Corollary 4. For $\gamma \in (-1, 1)$, it holds up to a subsequence

Proof. We use the same arguments as in Corollary 3. The only difference is that terms like (16) are estimated by the $L^p$-norm, with $p < 2$, of $\bar{u}_\epsilon^M - u_0^M$ instead of the $L^2$-norm, and then the strong convergence of $\bar{u}_\epsilon^M$ to $u_0^M$ in $L^p((0, T)\times \Sigma)$ is used.

Passing to the limit $\epsilon \to 0$, we obtain Theorem 3.2:

Proof of Theorem 3.2. The arguments are quite similar to those in Theorem 3.1 for the case $\gamma = -1$, so we only point out the main differences. The variational equation (18) is still valid for all $\phi \in \mathcal{D}\big(\overline{\Sigma}\big)$ and $\psi \in \mathcal{D}(0, T)$ if we replace $\frac{1}{\epsilon}$ by $\epsilon^{\gamma}$ in front of the diffusion term. In the limit $\epsilon \to 0$ this terms vanishes, since

Finally, the $L^2$-regularity of the time derivative $\partial_t u_0^M$ follows from the regularity of the right-hand side of the differential equation of $u_0^M$.

7.3.   The case $\gamma = 1$

Here, we treat the critical case $\gamma = 1$. Since in this case the two-scale limit $u_0^M$ depends on the macroscopic and the microscopic variable, it is no longer sufficient to work with the averaged function $\bar{u}_\epsilon$. To prove the convergences of the nonlinear terms, we use a Kolmogorov type compactness result for Banach valued functions, see [8], applied to the unfolded sequence $\mathcal{T}_\epsilon^Mu_\epsilon^M \in L^p(\Sigma, L^2((0, T), H^{\beta}(Z)))$. A main ingredient in this proof is the following lemma.

Lemma 7.4. For all $\phi_{\epsilon}^M \in L^2((0, T)\times \Omega_\epsilon^M)$, $h>0$, and $\bar{\xi} \in \mathbb{R}^{n-1}$ with $|\bar{\xi}| < h$, it holds for $\epsilon$ small enough that

with $\delta_l \phi_{\epsilon}^M$ defined in (9) with $l = l(\epsilon, \bar{\xi}, j) = j + \left[\frac{\bar{\xi}}{\epsilon}\right]$.

Proof. The proof is based on a special decomposition of $Z$ and can be found in [15,page 709], where here, we additionally use the fact that $\Omega_{\epsilon, 2h}^M \subset \hat{\Omega}_{\epsilon, h}^M \subset \Omega_\epsilon^Mh$ for $\epsilon$ small enough.

Theorem 7.5. For $\gamma = 1$, let $u_\epsilon$ be the solution of Problem (1). Then, there exists $u_0^M \in L^2((0, T)\times \Sigma, \mathcal{H}_{\rm{per}})^m\cap H^1((0, T), L^2(\Sigma, \mathcal{H}_0)^{\prime})^m$, such that for $p\in [1, 2)$ and $\beta \in \left( \frac12, 1 \right)$ up to a subsequence it holds that

Remark 4. Due to the boundedness of the sequence $\mathcal{T}_\epsilon^M u_\epsilon^M$ in the space $L^2((0, T)\times \Sigma, H^1(Z)) \cap H^1((0, T), L^2(\Sigma, \mathcal{H}_0^{\prime}))$, see Lemma 4.2, 4.4, and Proposition 8, we additionally obtain the weak convergence of $\mathcal{T}_\epsilon^M u_\epsilon^M$ in $L^2((0, T)\times \Sigma, H^1(Z))$ and $\partial_t \mathcal{T}_\epsilon^M u_\epsilon^M$ in $L^2((0, T), L^2(\Sigma, \mathcal{H}_0^{\prime}))$. However, the convergence of the time derivative will not be used in the derivation of the macroscopic problem, since the structure of the limit problem gives us even more regularity with respect to time.

Proof of Theorem 7.5. The two-scale convergences of $u_\epsilon^M$ and $\epsilon \nabla u_\epsilon^M$ follow from the a priori estimates for $u_\epsilon^M$ and Proposition 10.

We prove the strong convergence of $\mathcal{T}_\epsilon^M u_\epsilon^M$. We make use of the Kolmogorov-type compactness result from [8,Theorem 2.2]. We have to show (all functions are extended by zero)

(ⅰ) For every $A\subset \Sigma$ measurable, the sequence

is relatively compact in $L^2((0, T), H^{\beta}(Z))$.

(ⅱ) It holds that

First of all, we have $v_A^{\epsilon} \in L^2((0, T), H^1(Z))\cap H^1((0, T), \mathcal{H}_0^{\prime})$ with time derivative

since for every $\psi \in \mathcal{D}(0, T)$ and $\phi \in \mathcal{H}_0$, we have

Hence, Lemma 4.2, 4.4, and Proposition 8 imply the boundedness of $v_A^{\epsilon}$ in the space $L^2((0, T), H^1(Z))\cap H^1((0, T), \mathcal{H}_0^{\prime})$. For $\beta \in \left(\frac{1}{2}, 1\right)$ the embedding $H^1(Z) \hookrightarrow H^{\beta}(Z)$ is compact and the embedding $H^{\beta}(Z) \hookrightarrow \mathcal{H}_0^{\prime}$ is continuous. Therefore, we can apply the Aubin-Lions Lemma and obtain that the sequence $v_A^{\epsilon}$ is relatively compact in $L^2((0, T), H^{\beta}(Z))$, what proves (ⅰ). Since for all $h>0$ and $|\bar{\xi}|\ll h$, we have

by the same arguments as in the proof of Theorem 7.3, it is enough to show that for all $h>0$, it holds that

So, we fix $h>0$ and obtain with Lemma 7.4

Due to Lemma 4.3, the right-hand side converges to zero for $\epsilon, \bar{\xi} \to 0$. The uniform convergence is again obtained by the convergences of the shifts to $0$ for every fixed $\epsilon$.

The last statement of the theorem follows from the strong convergence of $\mathcal{T}_\epsilon^M u_\epsilon^M$, together with the continuity of the trace operator from $H^{\beta}(Z)$ into $H^{\beta - \frac12}(\partial Z)$ for $\beta \in \left(\frac12, 1\right)$ and the fact that $\mathcal{T}_\epsilon^\Sigma \big(u_\epsilon^M|_{ S_\epsilon^\pm }\big) = \big(\mathcal{T}_\epsilon^M u_\epsilon^M\big)|_{S^{\pm}}$.

Corollary 5. For $\gamma = 1$, it holds up to a subsequence

Proof. We only show the last convergence. From the strong convergence of $u_\epsilon^\pm|_{ S_\epsilon^\pm }$ to $u_0^{\pm}|_{\Sigma}$ in $L^2((0, T)\times \Sigma)$, see Proposition 9, it follows that $\mathcal{T}_\epsilon^\Sigma \big( u_\epsilon^\pm|_{ S_\epsilon^\pm }\big)$ converges strongly to $u_0^{\pm}|_{\Sigma}$ in $L^2((0, T)\times \Sigma \times Y)$. We have

The strong convergences of $\mathcal{T}_\epsilon^\Sigma \big( u_\epsilon^\pm|_{ S_\epsilon^\pm }\big)$ and $\mathcal{T}_\epsilon^\Sigma \big(u_\epsilon^M|_{ S_\epsilon^\pm }\big)$ imply, up to a subsequence, the pointwise convergence of the right-hand side almost everywhere in $(0, T)\times \Sigma \times Y$ to $h^{M, \pm}\big(\cdot , u_0^M|_{S^{\pm}}, u_0^{\pm}|_{\Sigma}\big)$. Further, this sequence is bounded in $L^2((0, T)\times \Sigma \times Y)$i.e., it converges weakly in $L^2((0, T)\times \Sigma \times Y)$ to the same limit. Now, Lemma A.3 gives us the result.

Finally, the compactness results from Theorem 7.5 and Corollary 5 allow us to pass to the limit $\epsilon \to 0$ in the microscopic problem and to obtain the macroscopic model in Theorem 3.3.

Proof of Theorem 3.3. The equations for the bulk-domains $\Omega^{\pm}$ can be obtained by similar arguments as for the cases $\gamma = -1$ and $\gamma \in (-1, 1)$.

To derive the limit equation for the membrane, we test the variational equation of $u_\epsilon^M$ in (2) with $\phi\left(\bar{x}, \frac{x}{\epsilon}\right) \psi(t)$, where $\phi \in \mathcal{D}\big(\overline{\Sigma}, C_{\rm{per}}^{\infty}\big(\overline{Y}, C^1([-1, 1])\big)\big)$, $\psi \in \mathcal{D}((0, T))$, integrate over time and use integration by parts in the term involving the time derivative to obtain

For $\epsilon \to 0$, we obtain from Theorem 7.5 and Corollary 5

By a density argument the equation holds for all $\phi \in L^2(\Sigma, \mathcal{H}_{\rm{per}})$. The regularity of the terms on the right-hand side imply $\partial_t u_0^M \in L^2((0, T), L^2(\Sigma, \mathcal{H}_{\rm{per}})^{\prime})$. The initial condition can be established in the usual way, where we have to use the two-scale convergence of $U_0^M\left(\cdot_{\bar{x}}, \frac{\cdot_{x_n}}{\epsilon }\right)$ to $U_0(\bar{x}, y_n)$. Uniqueness is again standard.

Remark 5.

(ⅰ) Due to the uniqueness of the solutions of the effective models in Theorems 3.1, 3.2, and 3.3, it follows that the whole sequence converges.

(ⅱ) The results also hold, if the parameter $\gamma$ is different for every speciesi.e., we have to replace $\gamma$ by $\gamma_i$ in the microscopic problem. Further, the results from this paper and [10] remain valid if we mix up the nonlinear Neumann-transmission conditions and the continuous transmission conditions on $S_\epsilon^\pm$ for different species.

8.   Summary and discussion

In this paper, we derived effective models for reaction-diffusion processes through thin layers with nonlinear transmission conditions at the bulk-layer interface, and diffusivities of order $\epsilon^\gamma, \gamma \in [-1, 1]$ in the layer region. It turns out, that for all $\gamma \in [-1, 1]$, the effective bulk solution $u_0^{\pm}$ is described by the same system of reaction-diffusion equations in $\Omega^\pm$, with parameters inherited from the microscopic system. At the interface $\Sigma$, we obtain interface laws, which strongly depend on the choice of the parameter $\gamma$.

In the critical case $\gamma = 1$, the effective solution $u^0$ and its normal flux may exhibit jumps across $\Sigma$. The normal flux of $u_0^{\pm}$ on $\Sigma$ is given by a nonlinear Neumann boundary condition which involves the homogenized solution $u_0^M$ in the layer. The evolution of $u_0^M$ is described by a reaction-diffusion system on the standard cell $Z$, where $x\in \Sigma$ plays the role of a parameter. This system is strongly coupled to the effective equations for $u_0^{\pm}$ in $\Omega^{\pm}$ via a nonlinear Neumann boundary condition on $S^{\pm}$.

For $\gamma \in [-1, 1)$, the solution $u^0$ is continuous across $\Sigma$, and the common trace is equal to $u_0^M$, which in this case is independent of the microscopic variable $y$. The normal flux of $u_0^{\pm}$ on $\Sigma$ is again given by a nonlinear Neumann boundary condition which depends on $u_0^M$. The interface laws satisfied by $u_0^M$ are, however, different for $\gamma \in (-1, 1)$ and $\gamma = -1$. For $\gamma \in (-1, 1)$, the function $u_0^M$ is the solution of an ordinary differential equation which also depends on the parameter $x \in \Sigma$. In the case $\gamma = -1$, we obtain an additional surface diffusion on $\Sigma$i.e., $u_0^M$ is the solution of a reaction-diffusion equation on $\Sigma$. In both cases, the jump in the normal flux of $u_0$ across $\Sigma$ enters the equation for $u_0^M$ as a sink/source term.

The methods developed in this paper can also be used for more complex multi-physics processes. Furthermore, the effective models obtained here rise new challenges also to numerical approaches, which have to take into account the special micro-macro features of the model.

Appendix A.   Two-scale convergence and the unfolding operator in thin domains

We repeat the definition of the two-scale convergence and the unfolding operator for thin domains, and briefly summarize some results related to these approaches, which are needed frequently throughout the paper. The two-scale convergences for domains was introduced in [1,16], and extended to thin layers with heterogeneous structure in [15].

Definition A.1. We say that a sequence $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$ converges (weakly) in the two-scale sense to the limit function $u_0 \in L^2((0, T)\times \Sigma \times Z)$, if for all $\phi \in C^0\big([0, T] \times \overline{\Sigma}, C_{\rm{per}}^0\big([0, 1]^{n-1}, C^0([-1, 1])\big)\big)$ it holds that

Further, we say that a (weakly) two-scale convergent sequence $u_\epsilon$ converges strongly in the two-scale sense to the limit $u_0$, if we additionally have

We remark that in [4] a definition of the two-scale convergence was given for a thin domain with a more particular geometry.

In the following, we consider functions $u_\epsilon \in L^2((0, T), H^1(\Omega_\epsilon^M))$, such that

with $\gamma \in [-1, 1]$. The behavior in the limit $\epsilon \to 0$ depends on the choice of $\gamma$, and we have to distinguish the three cases $\gamma = 1$, $\gamma \in (-1, 1)$, and $\gamma = -1$. To state the compactness results for $u_\epsilon$ and $\nabla u_\epsilon$ for different $\gamma$, we make use of the following spaces:

equipped with the $\|\cdot\|_{H^1(Z)}$-norm.

Proposition 10. Let $u_\epsilon$ be a sequence in $L^2((0, T), H^1(\Omega_\epsilon^M))$ such that

with a constant $C>0$ independent of $\epsilon$. Then, we have the following convergence results:

(i) For $\gamma = 1$, there exists a subsequence and a limit function $u_0 \in L^2((0, T)\times \Sigma, \mathcal{H}_{\rm{per}})$, such that

(ii) For $\gamma \in (-1, 1)$, there exist $u_0 \in L^2((0, T) \times \Sigma)$ and $u_1 \in L^2\big((0, T) \times\Sigma, \mathcal{H}_{\rm{per}}o\big)$, such that up to a subsequence

(iii) For $\gamma = -1$, there exist $u_0\in L^2((0, T), H^1(\Sigma))$ and $u_1\in L^2((0, T) \times \Sigma, \mathcal{H}_{\rm{per}}o)$, such that up to a subsequence

where $\nabla_{\bar{x}} u_0: = (\partial_1 u_0, \ldots, \partial_{n-1} u_0, 0)$.

Proof. The proof of (ⅰ) can be found in [15], and the proof of (ⅱ) and (ⅲ) in [10].

Next, we define the unfolding operator $\mathcal{T}_\epsilon^M$ for thin domains introduced in [15], which is an extension of the unfolding operator in fixed or perforated domains, see e.g., [2,6,18]. Here, we consider thin domains with a general lateral boundary $\partial \Sigma \times (-\epsilon, \epsilon)$, see also [7]. For the definition, we need the following notations:

Then, we define the unfolding operator as follows:

The unfolding operator $\mathcal{T}_\epsilon^M$ has the following basic properties:

Lemma A.2. [15] For $u_\epsilon, v_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$, we have

If additionally it holds that $u_\epsilon \in L^2((0, T), H^1(\Omega_\epsilon^M))$, then $\mathcal{T}_\epsilon^M u_\epsilon \in L^2((0, T)\times \Sigma, H^1(Z))$ and almost everywhere in $(0, T)\times \Sigma \times Z$ it holds that

Finally, we have the following relation between the unfolding operator $\mathcal{T}_\epsilon^M$ and the two-scale convergence in thin domains.

Lemma A.3. [15] Let $u_\epsilon \in L^2((0, T)\times \Omega_\epsilon^M)$ be a sequence with

with a constant $C>0$ independent of $\epsilon$. Then the following statements are equivalent:

(i) $u_\epsilon \rightarrow u_0$ weakly/strongly in the two-scale sense,

(ii) $\mathcal{T}_\epsilon^M u_\epsilon \rightarrow u_0$ weakly/strongly in $L^2((0, T)\times \Sigma \times Z)$.