1. Introduction

---

1.1. Background

In global financial markets, the pricing of a bond becomes more complicated due to different credit rating and different interest rate. A change of credit rating or a change of interest rate may cause drastic swing of bond prices. To manage the complication, one needs to understand how the credit risk impacts on the bond markets and how it can be measured. There are two types of credit risks: defaults and credit rating migrations. In the previous researches, the defaults are paid more attentions. However, in the financial crisis in 2008 and European debt crisis in 2010, the credit rating migrations played a much more important role in the finance and economics than what people expected. Therefore, the study of credit rating migrations becomes significant. Another observed factor in a financial market is that bond prices strongly depend on the interest rate. This leads to a crucial question of how to price a corporate bond with credit rating migration risks and interest rate change.

---

1.2. Literature review

There are many existing research papers on bond pricing models, see examples, Briys and de Varenne (Briys and Varenne, 1997), Tsiveriotis and Fernandes (Tsiveriotis and Fernandes, 1998).To measure default risks, there are two basic frameworks: a structure model, and a reduced form one. It is well-known that the first structure model for credit default proposed by Merton (Merton, 1974) is under the assumption that the firm's value follows a geometric Brownian motion process and the corporate bonds are considered as contingent claims. The default event may only occur at the maturity of the claim. In 1976, Black and Cox extended Merton's model to a first-passage-time model by introducing safety covenants. This extends the original model which gives bondholders the right to recognize a firm's value if its asset falls below some exogenously given threshold. In the modified model(Black and Cox, 1976), the default is allowed to occur any time up to the debt's maturity. By the reduced form framework, the credit events are considered to be governed by exogenous reason stochastically, see (Duffie and Singleton, 1999; Jarrow and Turnbull, 1995; Lando, 1998; Leland and Toft, 1996).

In study of credit rating migration, a major tool is the Markov chain. Most researchers mainly adopted a transfer intensity matrix. By this approach, the reduced form framework is naturally developed for the dynamic process of credit rating migrations, see Jarrow et al. (1997), Das and Tufano (1996), Hurd and Kuznetsov (2007), Lando (1998), Thomas et al. (2002) and so forth. The transfer intensity matrix usually comes from general statistical data, which do not include any particulary firm's information. However, a firm's own value plays a key role of its credit rating migration. In this situation, the Markov chain alone cannot fully capture the credit-rating mitigation for a firm. From the existing literatures, few research took this into account. Liang et al. (2015) in 2015 used a structure model for pricing bonds with credit rating migrations at first time. They gave a predetermined migration threshold where a firm's value is divided into high and low rating regions, under the assumption that the value of the firm follows a stochastic process. By using Feynman-Kac theorem, these models can be reduced to certain boundary value problems of partial differential equations. This PDE method is very different from the traditional approaches in the research field of corporative bond modelling. However, the rating migration boundary is usually not predetermined in the real financial world. It depends on the proportion of the debt and the value of the firm. From this point of view, the migration boundary should be a free boundary. Recently, Hu et al. (2015) and liang et al. (2016) proposed a new model to reflect this fact under the fixed-interest rate. They obtained the existence and uniqueness for the free boundary problem. Also, they proved that there is a travelling wave in the problem.

For pricing a bond, interest rate is a sensitive factor. Many stochastic models are used for the model, see Longstarff and Schwartz (1995) as an example. However, when a stochastic interest rate is considered, the bond model becomes two dimensions, which is much harder to analyze.

---

1.3. Result description

So far, all valuations for credit rating migration via pricing a corporate bond by structure model are assumed a constant interest rate. However, the stochastic interest rate for a bond is essential. So the extension for the model to stochastic interest rate is necessary. However, the extension meets a great difficult that the original free boundary model from one dimension to a higher one. That is the main contribution and solved problem in this paper.

In the present paper, we follow the ideas from (Hu et al., 2015) to derive a new model for a bond price in which credit-rating may change and the interest rate follows a stochastic process. To be more precise, we assume that the interest rate follows a Vasicek's stochastic process and the bond price follows a geometric Brownian motion with a different volatility in different credit-rating region. A coupled system of PDEs is derived. By employing a dimension-reduction technique we are able to simplify the coupled system to a single equation with a nonlinear jump coefficient in the leading term of the equation. Under certain conditions, the existence and uniqueness are established for the new model. We would like to indicate that our approach is different from that in (Hu et al., 2015; Liang et al., 2016). Moreover, the uniqueness proof in Section 4 is new to our knowledge. The method is also suitable for n-dimensional problems. Furthermore, the regularity of the free boundary is also considered. By transforming the problem to a Stefan-like problem, we prove that the free boundary is smooth. Some numerical results are also illustrated in the present paper. Those numerical results are well matching the theoretical analysis.

---

1.4. Paper structure

The paper is organized as follows: In Section 2, a new model with credit-rating migration and stochastic interest rate is rigorously derived under usual assumptions. In Section 3, we use the dimension reduction technique to simplify the model to a free boundary problem. In Section 4, we first use various PDE techniques to derive uniform estimates for the approximate problem. The existence and uniqueness are then established by using a compactness argument. Uniqueness is proved via an energy method. In Section 5, further properties of the solution are shown. In Section 6, some numerical results are presented. Some concluding remarks are given in section 7.

---

2. The Derivation of the Model

---

2.1. Basic assumptions

Let $(\Omega, \cal{F}, P)$ be a complete probability space. We assume that the firm issues a corporate bond, which is a contingent claim of its value on the space $\cal{F}$.

Assumption 2.1. Interest rate $r_t$ follows Vasicek process:

$dr_t=a(t)(\theta(t)-r_t)dt+\sigma_r(t)dW_t^r,$

where $a(t)$, $\theta(t)$ and $\sigma_r(t)$ are given positive functions, which are supposed to be positive constants $a, \theta, \sigma _r$ for the simplification in this paper. $W_t^r$ is the Brownian motion which generates the filtration $\{{\cal F}_t\}$.

We would like to point out that our model is a big improvement from the assumption of a constant interest rate to the stochastic one. There are many stochastic models for the interest rate, Vesicek model is popular one. The advantage point of this model is simply and easily calculable, while the weak point is the possibility of negative interest rate, which is proved to be very low. We assume that the interest rate follows Vesicek model also because that in our dimension reducing technique, only Vesicek model works.

Assumption 2.2 (the firm asset with credit rating migration). Let $S_t$ denote the firm's value in the risk neutral world. It satisfies

$\begin{equation*} dS_t=\left\{\begin{array}{l}r_tS_tdt+\sigma_H(t)S_tdW_t, \quad in\; high \;rating\; region, \\ r_tS_tdt+\sigma_L(t)S_tdW_t, \quad in\;low\; rating \;region, \end{array}\right. \end{equation*}$

where $r_t$ is the risk free interest rate, and

$\sigma_{H}(t)<\sigma_{L}(t)$

(1)

represent volatilities of the firm under the high and low credit grades, respectively. They are assumed to be deterministic and differentiable on $[0, T]$ and have a positive lower bound:

$0<\tilde\sigma_0\leq \sigma_{H}(t)<\sigma_{L}(t)\leq \tilde\sigma_1<\infty, 0\leq t\leq T.$

(2)

$W_t$ is the Brownian motion which generates the filtration $\{{\cal F}_t\}$, and

$Cov(dW_t, dW_t^r)=\rho dt,$

where $-1 < \rho < 1$ is a constant.

It is reasonable to assume (1), namely, that the volatility in high rating region is lower than the one in the low rating region. This implies that there is a jump for the volatility when the rating changes.

Assumption 2.3 (the corporate bond). The firm issues only one corporate zero-coupon bond with face value $F$. Denote $\Phi_t$ the discount value of the bond at time $t$, which is also the function of $r_t$. Therefore, on the maturity time $T$, an investor can get $\Phi_T=\min\{S_T, F\}$.

Assumption 2.4 (the credit rating migration time). High and low rating regions are determined by the proportion of the debt and value. The credit rating migration time $\tau_1$ and $\tau_2$ are the first moments when the firm's grade is downgraded and upgraded, respectively, as follows:

$\tau_1=\inf\{t>0|\Phi_0/S_0<\gamma, \Phi_t/S_t\geq \gamma\}, \tau_2=\inf\{t>0|\Phi_0/S_0>\gamma, \Phi_t/S_t\leq \gamma\},$

where $\Phi_t=\Phi_t(S_t, r, t)$ is a contingent claim with respect to $S_t$ and

$0<\gamma<1$

(3)

is a positive constant representing the threshold proportion of the debt and value of the firm's rating.

---

2.2. Cash flow

Once the credit rating migrates before the maturity $T$, a virtual substitute termination happens, i.e., the bond is virtually terminated and substituted by a new one with a new credit rating. There is a virtual cash flow of the bond. Denoted by $\Phi_H(y, r, t)$ and $\Phi_L(y, r, t)$ the values of the bond in high and low grades respectively. Then, they are the conditional expectations as follows:

$\begin{align} \nonumber \Phi_H(y, r, t)&=E_{y, t}\Big[e^{-\int_t^Tr_sds} \min(S_{T}, F)\cdot {\bf 1}_{\tau_1\ge T}\\ & +e^{-\int_t^{\tau_1}r_sds}\Phi_L(S_{\tau_1}, r_t, \tau_1)\cdot {\bf 1}_{t<\tau_1< T}\Big|S_t=y>\frac 1\gamma\Phi_H(y, t), r_t=r\Big], \end{align}$

(4)

$\begin{align} \label{lowholding} \nonumber \Phi_L(y, r, t)&=E_{y, t}[e^{-\int_t^Tr_sds} \min(S_{T}, F)\cdot {\bf 1}_{\tau_2\ge T}\\ &+e^{-\int_t^{\tau_2}r_sds}\Phi_H(S_{\tau_2}, r_t, \tau_2)\cdot {\bf 1}_{t<\tau_2< T}\Big|S_t=y<\frac 1\gamma\Phi_L(y, t), r_t=r\Big], \end{align}$

(5)

where ${\bf 1}_{event}=\left\{\begin{array}{ll}1, & \mbox { if "event" happens }, \\ 0, & \mbox{ otherwise. }\end{array}\right.$

---

2.3. Mathematical model

By Feynman-Kac formula (Dixit and Pindyck, 1994), it is not difficult to drive that $\Phi_H$ and $\Phi_L$ are the function of the firm value $S$, interest rate $r$ and time $t$. They satisfy the following partial differential equations in their regions:

$\begin{eqnarray*} &&\frac{\partial \Phi_H}{\partial t} +\frac12 \sigma ^2_HS^2\frac{\partial ^2\Phi_H}{\partial S^2} + \sigma _r\sigma _H\rho S\frac {\partial ^2\Phi_H}{\partial S\partial r} +\frac 12 \sigma _r^2 \frac{\partial ^2\Phi_H}{\partial r^2} +rS\frac{\partial \Phi_H}{\partial S}\\ &&\qquad+a(\theta-r)\frac{\partial \Phi_H}{\partial r}-r\Phi_H = 0, \qquad S>\frac 1\gamma\Phi_H, \; 0<t<T, \end{eqnarray*}$

(6)

$\begin{eqnarray*} &&\frac{\partial \Phi_L}{\partial t} +\frac12 \sigma ^2_LS^2\frac{\partial ^2\Phi_L}{\partial S^2} + \sigma _r\sigma _L\rho S\frac {\partial ^2\Phi_L}{\partial S\partial r} +\frac 12 \sigma _r^2 \frac{\partial ^2\Phi_L}{\partial r^2} +rS\frac{\partial \Phi_L}{\partial S}\\ &&\qquad+a(\theta-r)\frac{\partial \Phi_L}{\partial r}-r\Phi_L = 0, \qquad S<\frac 1\gamma\Phi_L, \; 0<t<T, \end{eqnarray*}$

(7)

with the terminal condition:

$\Phi_H(S, T)=\Phi_L(S, T)=\min\{S, F\}.$

(8)

(4) and (5) imply that the value of the bond is continuous when it passes the rating threshold, i.e.,

$\Phi_H=\Phi_L =\gamma S\quad \mbox{on the rating migration boundary}.$

(9)

Also, as in (Hu et al., 2015; Jin et al., 2016), we have

$\frac {\partial \Phi_H}{\partial S} =\frac{\partial \Phi_L}{\partial S} \quad \mbox{on the rating migration boundary}.$

(10)

This is a two-dimensional free boundary problem of an terminate value parabolic problem with discontinuous leading coefficient.

---

3. A PDE Problem

---

3.1. Reduction of dimension

The equations (6) and (7) are two-dimensional. For the purpose of simplifying the problem, we could use a technique to reduce the dimension.

Denote $P(r_t, t:T)$ to be the value of a zero-coupon bond faced 1 at time $T$. So that, it satisfies

$\frac{\partial P}{\partial t} +\frac 12 \sigma _r^2 \frac{\partial ^2 P}{\partial r^2} +a(\theta-r)\frac{\partial P}{\partial r}-rP = 0,$

(11)

which admits a unique solution (Hall, 1989)

$P(r, t:T)= A(t)e^{-rB(t)},$

(12)

where

$A(t)=e^{\{\frac 1{a^2}[B^2(t)-(T-t)](a^2\theta-\frac {\sigma _r^2}2)-\frac{\sigma ^2}{4a}B^2(t)\}}, \quad B(t)=\frac 1a(1-e^{-a(T-t)}).$

Now make a transform by

$y=\frac S{P(r, t;T)}, \quad V_H(y, t)=\frac{\Phi_H(S, r, t)}{P(r, t;T)}, \quad V_L(y, t)=\frac{\Phi_L(S, r, t)}{P(r, t;T)}.$

Then $V(y, t)$ satisfies

$\frac{\partial V_H}{\partial t} +\frac 12\hat{\sigma }_H^2y^2 \frac{\partial ^2 V_H}{\partial y^2} = 0, \quad y>\frac 1\gamma V_H, \ 0<t<T,$

(13)

$\frac{\partial V_L}{\partial t} +\frac 12\hat{\sigma }_L^2y^2 \frac{\partial ^2 V_L}{\partial y^2} = 0, \quad y<\frac 1\gamma V_L, \ 0<t<T,$

(14)

$V_H(y, T)=V_L(y, T)=\min\{y, F\},$

(15)

where

$\hat{\sigma }_i=\sqrt{\sigma _i^2+2\rho\sigma _i\sigma _rB(t)+\sigma _r^2B^2(t)}, \quad i=H, L.$

(16)

And on credit rating migration boundary, there holds

$V_H(y, t)=V_L(y, t)=\gamma y, \qquad \frac{\partial V_H}{\partial y}= \frac{\partial V_L}{\partial y}.$

(17)

---

3.2. A free boundary problem

Using the standard change of variables $x=\log y$ and renaming $T-t$ as $t$, and defining

$\phi(x, t)=\left\{\begin{array}{l}V_H(e^x, T-t) \mbox{ in high rating region}, \\ V_L(e^x, T-t) \mbox{ in low rating region}, \end{array}\right.$

using also (6) and (7), we then derive the following equation from (6), (7):

$\frac{\partial \phi}{\partial t} -\frac12 \sigma ^2\frac{\partial ^2\phi}{\partial x^2} +\frac12\sigma ^2\frac{\partial \phi}{\partial x} = 0, \quad (x, t)\in Q_T\backslash \Gamma_T$

(18)

where

$Q_T=(-\infty, +\infty)\times(0, T], \quad \Gamma_T=\{(x, t)|\phi(x, t)=\gamma e^{x}\}$

(19)

and $\sigma$ is a function of $\phi$ and $(x, t)$, i.e.,

$\sigma = \sigma (\phi, x, t) = \left\{\begin{array}{ll}\hat{\sigma }_H & \text{if} \;\phi<\gamma e^{x}, \\ \hat{\sigma }_L & \text{if} \;\phi\ge \gamma e^{x}. \end{array}\right.$

(20)

The constant $\gamma$ is defined in (3), and $\hat\sigma _H, \hat\sigma _L$ are defined in (16). There exist constants $\sigma _0, \sigma _1$, such that

$0<\sigma_0\le\hat{\sigma }_H, \hat{\sigma }_L\le\sigma _1<\infty.$

(21)

Without loss of generality, we assume $F=1$. Equation (18) is supplemented with the initial condition (derived from (8))

$\phi(x, 0) = \phi_0(x)=\min\{e^x, 1\}, \quad -\infty<x<\infty.$

(22)

The domain will be divided into the high rating region $Q_{T}^H$ where $\phi < \gamma e^{x}$ and a low rating region $Q_{T}^L$ where $\phi > \gamma e^{x}$. We shall prove that these two domains will be separated by a free boundary $x=s(t)$, and

$Q_T^H = \{x>s(t), 0<t<T\}, \qquad Q_T^L = \{x<s(t), 0<t<T\}.$

(23)

In another word, $s(t)$ is apriorily unknown since it should be the solved by the equation

$\phi(s(t), t)=\gamma e^{s(t)},$

where the solution $\phi$ is apriorily unknown.

---

4. Existence and Uniqueness

---

4.1. Approximation

Let $H(\xi)$ be the Heaviside function, i.e., $H(\xi)=0$ for $\xi < 0$ and $H(\xi)=1$ for $\xi>0$. Then we can rewrite (20) as

$\sigma (t, \phi) = \hat\sigma _H + (\hat\sigma _L-\hat\sigma _H) H(\phi - \gamma e^{x}).$

We use a standard approximation for $H(\xi)$ and $\phi_0(x)$ such that

$H_\varepsilon (\xi)\in C^{\infty}(\mathbb{R}^1)\quad \mbox{and}\quad H_\varepsilon (\xi)=H(\xi) \;\;\text{for}\;\; |\xi|\ge\varepsilon , \\ \phi_{0\varepsilon }(x)\in C^3(\mathbb{R}^1)\cap W^{1, \infty}(\mathbb{R}^1), \\ \phi'_{0\varepsilon }(x)-\gamma e^x<0, \quad \;\;\text{for}\;\;0<x<\ln\frac1\gamma .$

Now we consider the following approximated problem

$\frac{\partial \phi_\varepsilon }{\partial t} -\frac12 \sigma ^2_\varepsilon \Big(\frac{\partial ^2\phi_\varepsilon }{\partial x^2} -\frac{\partial \phi_\varepsilon }{\partial x}\Big) = 0, \quad (x, t)\in Q_T,$

(24)

$\phi_\varepsilon (x, 0) = \phi_{0\varepsilon }(x), \quad -\infty<x<\infty,$

(25)

where

$\sigma _\varepsilon = \hat\sigma _H + (\hat\sigma _L-\hat\sigma _H) H_\varepsilon (\phi_\varepsilon - \gamma e^{x}).$

(26)

---

4.2. Estimates

Lemma 4.1. The problem (24)-(25) admits a unique solution

$\phi_\varepsilon (x, t)\in C^{2, 1}(Q_T)\cap C^{\alpha, \frac\alpha2}(\overline{Q}_T), \quad \;\;\text{for}\;\; \;\;\text{for}\;\;all\alpha\in(0, 1)$

Moreover, the following estimates hold uniformly with respect to $\varepsilon$:

a) $0\le\phi_\varepsilon (x, t)\le 1$, $0\le\phi_{\varepsilon x}(x, t)\le C_1$

b) $\sup\limits_{0\le t\le T}\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2dx+\iint\limits_{Q_T}(\phi_{\varepsilon xx}^2+\phi_{\varepsilon t}^2) dxdt\le C_2$

where $C_1$ and $C_2$ depend only on known data.

Proof. By the theory of parabolic equation, we know that the problem (24)-(25) admits a unique classic solution $\phi_\varepsilon \in C^{2, 1}(Q_T)\cap C^{\alpha, \frac\alpha2}(\overline{Q}_T)$.

Now we derive the uniform estimates. First of all, by the maximum principle for Cauchy problem, we see

$0\le\phi_\varepsilon (x, t)\le 1, \quad (x, t)\in\overline{Q}_T.$

Let $\psi_\varepsilon (x, t)=\phi_{\varepsilon x}(x, t)$, then

$\psi_{\varepsilon t}-\frac{\partial }{\partial x}\big[\frac12\sigma _\varepsilon ^2\big(\psi_{\varepsilon x}-\psi_\varepsilon \big)\big], \quad (x, t)\in\overline{Q}_T, \\ \psi_\varepsilon (x, 0)=\phi'_{0\varepsilon }(x)\ge 0, \quad -\infty<x<+\infty$

Again, since $\phi'_{0\varepsilon }(x)\ge 0$, by maximum principle, we obtain

$\psi_\varepsilon (x, t)\ge 0.$

On the other hand,

$\psi_{\varepsilon t}-\frac{\partial }{\partial x}\big(\frac12\sigma _\varepsilon ^2 \psi_{\varepsilon x}\big)=-\frac{\partial }{\partial x}\big(\frac12\sigma _\varepsilon ^2 \psi_{\varepsilon }\big)$

(27)

We multiply equation (27) $\psi_\varepsilon ^p$ for any $p\ge 1$, to obtain

$\frac{d}{dt}\frac{1}{p+1}\int_{-\infty}^{+\infty}\psi_\varepsilon ^{p+1}dx+p\int_{-\infty}^{+\infty}\frac12\sigma _\varepsilon ^2 \psi^2_{\varepsilon x}\psi_\varepsilon ^{p-1}dx\\ =p\int_{-\infty}^{+\infty}\frac12\sigma _\varepsilon ^2 \psi_\varepsilon ^p\psi_{\varepsilon x}dx\\ \le\delta \int_{-\infty}^{+\infty}p\cdot\frac12\sigma _\varepsilon ^2\psi_\varepsilon ^{p-1}\psi_{\varepsilon x}^2dx+C(\delta )p\int_{-\infty}^{+\infty}\frac12\sigma _\varepsilon ^2\psi_\varepsilon ^{p+1}dx.$

If we choose $\delta$ sufficiently small, we have

$\frac{d}{dt}\frac{1}{p+1}\int_{-\infty}^{+\infty}\psi_\varepsilon ^{p+1}dx+\frac12 p\int_{-\infty}^{+\infty}\frac12\sigma _\varepsilon ^2\psi^2_{\varepsilon x}\psi_\varepsilon ^{p-1}dx \le C\int_{-\infty}^{+\infty}\frac12\sigma _\varepsilon ^2 \psi_\varepsilon ^{p+1}dx$

Since $0 < \sigma _0\le\sigma _\varepsilon \le\sigma _1 < +\infty$, we have

$\int_{-\infty}^{+\infty}\psi_\varepsilon ^{p+1}dx+\int_{-\infty}^{+\infty}\int_{0}^{t}\psi^2_{\varepsilon x}\psi_\varepsilon ^{p-1}dxdt\\ \le\int_{-\infty}^{+\infty}\phi'_{0\varepsilon }(x)^2dx+C\int_{0}^{t}\int_{-\infty}^{+\infty}\psi_\varepsilon ^{p+1}dxdt$

Gronwall's inequality yields,

$\int_{-\infty}^{+\infty}\psi_\varepsilon ^{p+1}dx+\int_{-\infty}^{+\infty}\int_{0}^{T}\psi^2_{\varepsilon x}\psi_\varepsilon ^{p-1}dxdt\le C,$

where $C$ depends only on known data and $p$, but not on $\varepsilon$.

Now from equation (27),

$\psi_{\varepsilon t}-\frac{\partial }{\partial x}\big(\frac12\sigma _\varepsilon ^2 \psi_{\varepsilon x}\big)=-\frac{\partial }{\partial x}\big(\frac12\sigma _\varepsilon ^2 \psi_{\varepsilon }\big), \quad (x, t)\in Q_T\\ \psi_\varepsilon (x, 0)=\phi'_{0\varepsilon }(x), \quad \quad -\infty<x<+\infty$

where $\sigma _\varepsilon ^2 \psi_{\varepsilon }\in L^p(\mathbb{R}^1)$ for any $p>1$. Then $|| \psi_\varepsilon || _{L^{\infty}(Q_T)}\le C$. To derive the $W^{2, 1}_2(Q_T)-$estimate, we multiply equation (24) by $\phi_{\varepsilon xx}$ and integrate over $Q_t$ to obtain

$\sigma _0\iint\limits_{Q_t}\phi_{\varepsilon xx}^2dxdt+\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2dx-\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2(x, 0)dx\\ =\iint\limits_{Q_t}\frac12\sigma _\varepsilon ^2\phi_{\varepsilon x}\phi_{\varepsilon xx}dxdt\\ \le \delta \iint\limits_{Q_t}\phi_{\varepsilon xx}^2dxdt+C(\delta )\iint\limits_{Q_t}\phi_{\varepsilon x}^2dxdt$

By choosing $\delta$ sufficiently small, we have

$\sigma _0\iint\limits_{Q_t}\phi_{\varepsilon xx}^2dxdt+\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2(x, t)dx \le\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2(x, 0)dx+C\iint\limits_{Q_t}\phi_{\varepsilon x}^2dxdt$

Gronwall's inequality yields

$\sup\limits_{0\le t\le T}\int_{-\infty}^{+\infty}\phi_{\varepsilon x}^2dx+\iint\limits_{Q_t}\phi_{\varepsilon xx}^2dxdt\le C.$

From the equation (24), we see

$\iint\limits_{Q_t}\phi_{\varepsilon t}^2dxdt\le C$

Lemma 4.2. The solution $\phi_\varepsilon (x, t)\in C^{1+\alpha, \frac{1+\alpha}{2}}({Q}_{T})$ uniformly with respect to $\varepsilon$, for some $\alpha\in (0, 1)$. Moreover, for any $\rho>0$ there exists a constant $C(\rho)$ such that

$||\psi_{\varepsilon }||_{C^{\alpha, \frac{\alpha}{2}}(\bar{Q}_{T}\backslash B_{\rho}(0, 0))}\leq C(\rho),$

where $B_{\rho}(0, 0)=\{(x, t): -\rho < x < \rho, 0 < t < \rho\}$ and $C(\rho)$ depends only on known data and $\rho$.

Proof. Indeed, we see $\psi_\varepsilon (x, t)$ is a solution of the following problem:

$\psi_{\varepsilon t}-\frac{\partial }{\partial x}\big[\frac12\sigma ^2\big(\psi_{\varepsilon x}-\psi_{\varepsilon }\big)\big]=0, \quad (x, t)\in {Q}_T, \\ \psi_{\varepsilon }(x, 0)=\phi'_{\varepsilon 0}(x)\ge 0, \quad -\infty<x<+\infty.$

By the standard theory for parabolic equations, we see that $\psi_{\varepsilon }(x, t)$ is Hölder continuous in $Q_{T}$. Moreover, the Hölder continuity of $\psi_\varepsilon$ holds up to the initial time $t=0$ except a neighborhood of the singular point $(0, 0)$. Hence, for any $\rho>0$, we have

$||\psi_{\varepsilon }||_{C^{\alpha, \frac{\alpha}{2}}(\bar{Q}_{T}\backslash B_{\rho}(0, 0))}\leq C(\rho),$

where $B_{\rho}(0, 0)$ defined above and $C(\rho)$ depends only on known data and $\rho$, but not on $\varepsilon$.

---

4.3. Existence

Theorem 4.1. The problem (17), (21) admits a solution $\phi(x, t)\in W^{1, 0}_\infty(Q_T)\cap W^{2, 1}_2(Q_T)\cap C^{\alpha, \frac{\alpha}{2}}(\overline{Q}_T)$, for any $\alpha\in(0, 1)$. Furthermore, the solution satisfies

a) $0\le\phi(x, t)\le 1$, $0\le\phi_{x}(x, t)\le C_1$,

b) $\int_{-\infty}^{+\infty}\phi_{x}^2dx+\iint\limits_{Q_T}(\phi_{xx}^2+\phi_{t}^2) dxdt\le C_2$,

where $C_1$ and $C_2$ are constants which depend only on known data.

Proof. By the standard compactness argument, we see that there exists a function $\phi(x, t)\in C^{\alpha, \frac\alpha2}(\overline{Q}_T)\cap W^{2, 1}_2(Q_T)$ such that

$\phi_{\varepsilon }(x, t)\to \phi(x, t) \quad \mbox{uniformly in } C^{\alpha, \frac\alpha2}(\overline{Q}_T), \\ \phi_{\varepsilon }(x, t)\to \phi(x, t) \quad \mbox{weakly in } W^{2, 1}_2(Q_T).$

Moreover, $0\le\phi(x, t)\le 1$, $0\le\phi_{x}(x, t)\le C_1$. For the limit function $\phi$, and $(x_0, t_0)$ with $(x_0, t_0)\notin\Gamma_T$ and $t_0>0$, where $\Gamma_T$ is defined in (19), there exists a small neighborhood $P_\delta (x_0, t_0)=\{(x, t)|(x-x_0)^2+(t-t_0)^2 < \delta \}$ such that $P_\delta (x_0, t_0)\cap\Gamma_T=\emptyset$. In $P_\delta (x_0, t_0)$, $\sigma _\varepsilon (x, t, \phi)=\sigma (x, t, \phi).$ By regularity theory of parabolic equation, $\phi(x, t)\in C^{2, 1}(P_\delta (x_0, t_0))$, and

$\phi_t-\frac12\sigma ^2(\phi_{xx}-\phi_{x})=0.$

It follows that for any $(x, t)\notin\Gamma_T$

$\phi_t-\frac12\sigma ^2(\phi_{xx}-\phi_{x})=0, \quad \mbox{in the classical sense in $Q_T\backslash\Gamma_T$}, \\ \phi(x, 0)=\phi_0(x), \quad -\infty<x<+\infty.$

Notice also by Lemma 4.2, $\phi, \phi_x$ are continuous across $\Gamma_T.$ That is, $\phi(x, t)$ is a solution in $Q_T$.

We can obtain a better regularity for the strong solution obtained in Theorem 4.1.

Theorem 4.2. The solution $\phi(x, t)\in C^{1+\alpha, \frac{1+\alpha}{2}}({Q}_{T})$ for some $\alpha\in (0, 1)$. Moreover, $\phi_x(x, t)$ is Hölder continuous up to the initial time $t=0$ except a neighborhood of $(0, 0)$.

Proof. Indeed, we see $\psi(x, t)=\phi_x(x, t)$ is a weak solution of the following problem:

$\psi_{ t}=\frac{\partial }{\partial x}\big[\frac12\sigma ^2\big(\psi_{ x}-\psi\big)\big], \quad (x, t)\in {Q}_T, \\ \psi(x, 0)=\phi'_{0}(x)\ge 0, \quad -\infty<x<+\infty.$

By the standard regularity theory of parabolic equations, we see that $\psi(x, t)$ is Hölder continuous in $Q_{T}$. Moreover, the Hölder continuity of $\psi$ holds up to the initial time $t=0$ except a neighborhood of the singular point $(0, 0)$.

---

4.4. Uniqueness

In this section we use a novel approach to prove the uniqueness. This method is also suitable for a general n-dimensional problem.

Theorem 4.3. The solution obtained in Theorem 4.1 is unique.

Proof. We rewrite Eq.(18) as follows:

$k(\phi, x, t)\phi_t=\phi_{xx}-\phi_x,$

(28)

where

$k(\phi, x, t)=\Big\{\begin{array}{ll}2/\hat\sigma _L^2(t), \quad&\mbox{ if }\phi>\gamma e^x, \\2/\hat\sigma _H^2(t), \quad&\mbox{ if }\phi<\gamma e^x.\end{array}$

Define

$\begin{eqnarray*} e(\phi, x, t)\nonumber&=&\Big(1/\hat\sigma _L^2(t)+1/\hat\sigma _H^2(t)\Big)(\phi-\gamma e^x)\\ \nonumber&&+\Big(1/\hat\sigma _L^2(t)-1/\hat\sigma _H^2(t)\Big)|\phi-\gamma e^x|\\ &=&\Big\{\begin{array}{ll}2(\phi-\gamma e^x)/{\hat \sigma _L^2(t)}, \quad &\mbox{ if } \phi>\gamma e^x, \\ 2(\phi-\gamma e^x)/{\hat \sigma _H^2(t)}, &\mbox{ if } \phi<\gamma e^x.\label{em} \end{array} \end{eqnarray*}$

(29)

which satisfies that

$k(\phi, x, t)= \frac{\partial e}{\partial \phi}=\Big\{\begin{array}{ll} 2/\hat\sigma _L^2(t), \quad &\mbox{ if } \phi>\gamma e^x, \\ 2/\hat\sigma _H^2(t), &\mbox{ if } \phi<\gamma e^x. \end{array}$

(30)

Therefore,

$\frac{\partial }{\partial t}[e(\phi, x, t)]-[\phi_{xx}-\phi_x]=\frac{\partial e}{\partial t}\equiv f(\phi, x, t),$

(31)

where

$\begin{eqnarray*} f(\phi, x, t)&:=&\Big(1/\hat\sigma _L^2(t)+1/\hat\sigma _H^2(t)\Big)'(\phi-\gamma e^x)\\ &&+\Big(1/\hat\sigma _L^2(t)-1/\hat\sigma _H^2(t)\Big)'|\phi-\gamma e^x|. \end{eqnarray*}$

We also have

$e(\phi, x, t)|_{t=0}=e_0(x),$

(32)

where

$\begin{eqnarray*} e_0(x)&=&\Big(1/\hat\sigma _L^2(0)+1/\hat\sigma _H^2(0)\Big)(\phi_0(x)-\gamma e^x)\\ &&+\Big(1/\hat\sigma _L^2(0)-1/\hat\sigma _H^2(0)\Big)|\phi_0(x)-\gamma e^x|, \end{eqnarray*}$

To prove the uniqueness, we assume that there are two solutions $\phi_i(x, t), \ i=1, 2$ of the problem (17), (21), which satisfy the estimates in Theorem 4.1. Set

$\phi(x, t)=\phi_1(x, t)-\phi_2(x, t), \hspace{1cm}(x, t)\in Q_T.$

Now we multiply $\int_t^\tau\phi(x, s) ds$ in the both sides of (31), where $\tau\in [0, T]$, and then integrate it in $\mathbb{R}$, we have

$\begin{eqnarray*} && \frac{\partial }{\partial t}\int_{\mathbb{R}}\Big[( e(\phi_1, x, t)-e(\phi_2, x, t))\int_t^\tau\phi ds\Big]dx\\ &&+\int_{\mathbb{R}}\Big[( e(\phi_1, x, t)-e(\phi_2, x, t))\phi \Big]dx\\ &=&\int_{\mathbb{R}}\Big[(\phi_{xx}-\phi_x)\int_t^\tau\phi ds\Big]dx +\int_{\mathbb{R}}\Big[\Big(f(\phi_1, x, t)-f(\phi_2, x, t)\Big)\int_t^\tau\phi ds\Big]dx\\ &:=& A_1(x, t, \tau)+A_2(x, t, \tau), \end{eqnarray*}$

where

$\begin{eqnarray*} A_1(t, \tau) & = & \int_{\mathbb{R}}\Big[(\phi_{xx}-\phi_x)\int_t^\tau\!\phi ds\Big]dx, \\ A_2(t, \tau) & = &\int_{\mathbb{R}}\!\!\Big[\Big(f(\phi_1, x, t)-f(\phi_2, x, t)\Big)\!\!\int_t^\tau\!\phi ds\Big]dx. \end{eqnarray*}$

Now,

$\begin{eqnarray*} A_1(t, \tau) & = &- \int_{\mathbb{R}}\Big[(\phi_{x}-\phi)\int_t^\tau\phi_x ds\Big]dx\\ & = &\frac 12\frac{\partial }{\partial t}\int_{\mathbb{R}}\Big[\int_t^\tau \phi _xds\Big]^2dx +\int_{\mathbb{R}}\Big[\phi\int_t^\tau\phi_x ds\Big]dx. \end{eqnarray*}$

$A_2(t, \tau) = \int_{\mathbb{R}}\Big[\Big(f_\phi(\xi, t)\phi\Big)\int_t^\tau\phi ds\Big]dx,$

where $\xi$ is the mean-value between $\phi_1$ and $\phi_2$.

We take integration to the above equation with respect to $t$ from $0$ to $\tau$ to obtain:

$\begin{eqnarray*} && \int_0^\tau \int_{\mathbb{R}}[e(\phi_1, x, t)-e(\phi_2, x, t)]\phi dxdt+\frac 12\int_{\mathbb{R}}\Big[\int_{0}^{\tau}\phi _xds\Big]^2 dx\\ & = & \int_{0}^{\tau}\int_{\mathbb{R}^+} \Big[\phi\int_t^\tau\phi_x ds\Big] dxdt +\int_0^\tau\int_{\mathbb{R}}\Big(f_{\phi}(\xi, x, t)\phi\Big)\int_t^\tau\phi dsdxdt\\ &\le &2\varepsilon \int_0^\tau \int_{\mathbb{R}}\phi^2dxdt+C(\varepsilon)\int_{0}^{\tau}\int_{\mathbb{R}} \Big(\Big[\int_0^\tau\phi ds\Big]^2+\Big[\int_0^\tau\phi_x ds\Big]^2\Big) dxdt. \end{eqnarray*}$

Here at the final step we have used the uniform boundedness of $f_{u}(u, t)$ and Cauchy-Schwarz's inequality with small parameter $\varepsilon$.

Notice (29), we have

$[e(\phi_1, x, t)-e(\phi_2, x, t)]\ge c_0(\phi_1-\phi_2)=(2/\sigma _1^2)\phi,$

where $\sigma _1$ defined in (21) has a positive lower bound. We choose $\varepsilon$ sufficiently small to obtain

$\int_0^\tau \int_{\mathbb{R}}\phi^2 dxdt+\int_{\mathbb{R}}\Big[\int_{0}^{\tau}\phi _xds\Big]^2 dx\\ \leq C\Big[\int_0^\tau [\int_0^t \int_{\mathbb{R}}\phi^2 dxds]dt+\int_{0}^{\tau}\int_{\mathbb{R}} \Big[\int_0^\tau\phi_x ds\Big]^2 dxdt\Big].$

Consequently, Gronwall's inequality yields $\phi(x, t)\equiv 0$ on $Q_{\tau}$. As $\tau$ is arbitrary over $[0, T]$, we have $\phi(x, t)\equiv 0$ on $Q_{T}$.

Thin concludes the proof of uniqueness.

---

5. Free Boundary and its Regularity

In this section, we show that $\Gamma_T$ is the graph of a smooth free boundary $x=s(t)$.

Lemma 5.1. There exists a number $T_0>0$, such that $\Gamma_{T_0}$ defined in (19) is the graph of a function $x=s(t), 0\le t\le T_0$ with $s(0)=s_0=\ln \frac{1}{\gamma}$.

Proof. Note that $\phi_0(x)=\min\{e^x, 1\}$, then $s_0:=s(0)=\ln 1/\gamma >0$, it follows that $\phi'_0(x)=0$, for $x>0$; $\phi'_0(x)=e^x$, for $x < 0$. It follows that if $x>0$,

$\phi_{0}^{'}(x)-\gamma e^x=-\gamma e^x<0.$

Since $\phi_x(x, t)$ is Hölder continuous except $(0, 0)$, there exists small numbers $t_0>0$ and $\delta_0>0$ such that

$\phi_x(x, t)-\gamma e^x\le m_0<0, \quad s_0-\delta_0<x<s_0+\delta_0, \quad 0\le t\le t_0,$

where $m_0$ depends on $t_0$ and $\delta_0$.

By the implicit function theorem, there exists a function, denoted by $x=s(t)$ solves $\phi(s(t), t)=\gamma e^{s(t)}$ such that

$\Gamma_{t_0}=\{(x, t)|x=s(t), 0\le t\le t_0\}.$

It will be seen in the next theorem that we can extend $t_0$ as long as $\phi_x^-(s(t), t)-\gamma e^{s(t)} < 0$.

Theorem 5.1. There exists a function $x=s(t) \in C^{\beta}[0, T]$ with $\beta\in (\frac{1}{2}, 1)$ such that

$\Gamma_T = \{(s(t), t): 0\leq t\leq T\}.$

Moreover, there exists a constant $\varepsilon _0>0$ such that

$\phi_{x}(s(t), t)-\gamma e^{s(t)}\leq -\varepsilon _0<0, 0\leq t\leq T.$

Proof. From Lemma 5.1, we know that there exists at least a small internal $[0, T_{0}]$ such that

$\Gamma_{T_{0}}=\{(s(t), t): 0\leq t\leq T_0.\}$

Moreover,

$\phi_x(s(t), t)-\gamma e^{s(t)}<0, t\in [0, T_{0}].$

Since $F(x, t):=\phi(x, t)-\gamma e^x$ is differentiable in $Q_{T}$ with respect to $x$, by the implicit function theorem, the set

$\Gamma_{t_0}=\{(x, t); F(x, t)=0, 0\leq t\leq t_0\}$

can be extended as the graph of a curve $x=s(t)$ as long as

$F_x(x, t)<0, \mbox{for all $(x, t)\in \Gamma_{T}$}.$

Moreover, $x=s(t)$ is decreasing on $[0, T]$.

Define

$T^*=\sup\{ T_{0}: \phi_x(s(t), t)-\gamma e^{s(t)}<0, 0\leq t\leq T_{0}\}.$

We claim $T^*=T$. Assume the contrary with $T^* < T$. It is clear by the definition of $T^*$ and the continuity of $\phi_x(x, t)$ that

$\lim\limits_{t\to T^{*}}(\phi_x^-(s(t), t)-\gamma e^{s(t)})=0 .$

We define $W(x, t)=\phi(x, t)-\gamma e^{x}$, then

$W_t=\phi_t, \quad W_x=\phi_x-\gamma e^{x}, \quad W_{xx}=\phi_{xx}-\gamma e^{x}$

Therefore, $W(x, t)$ satisfies

$W_t-\frac12\sigma _{H}^2(W_{xx}-W_x)= 0, \, -\infty<x<s(t), 0<t<T^*, \\ W(s(t), t)=0, \hspace{1cm} 0<t<T^*, \\ W(x, 0)=W_0(x), \hspace{1cm}-\infty<x<s_0,$

where $W_0(x):=\phi_0(x)-\gamma e^x\geq 0$ on $(-\infty, s_0]$.

Since $s(t)$ is decreasing on $[0, T^*)$, Hopf's lemma holds at $(s(T^*), T^*)$. Hence, by Hopf's lemma, there exists a number $\varepsilon _0>0$ such that $W_x(s(T^*), T^*)\leq -\varepsilon _0 < 0$, which contradicts with the definition of $T^*$. It follows that $T=T^*$. Consequently, there exists a curve, denoted by $x=s(t)$, such that

$\Gamma_{T}=\{(s(t), t): 0\leq t\leq T.\}.$

Moreover, there exists a constant $\varepsilon _0>0$ such that

$\phi_{x}(s(t), t)-\gamma e^{s(t)}\leq -\varepsilon _0<0, 0\leq t\leq T.$

(33)

To prove the Hölder continuity of $s(t)$ on $[0, T]$, we take any $t_1 < t_2$ in $[0, T]$ with $|t_1-t_2|$ sufficiently small,

$\phi(s(t_2), t_2)-\phi(s(t_1), t_1)=\gamma (e^{s(t_{2})}-e^{s(t_{1})}).$

Then,

$\phi(s(t_2), t_1)-\phi(s(t_1), t_1)-\gamma (e^{s(t_{2})}-e^{s(t_{1})})\\ = \phi(s(t_2), t_1)-\phi(s(t_2), t_2).$

We use the mean-value theorem to obtain

$|s(t_2)-s(t_1)|\leq \frac{|\phi(s(t_{2}), t_{2})-\phi(s(t_{2}), t_{1})|}{|F_{x}(\theta, t_{1})|},$

where $\theta$ is the mean-value between $s(t_{1})$ and $s(t_{2})$.

Since $|F_x(x, t)|$ is bounded and $|F_x(x, t)|$ has a positive lower bound in a neighborhood of $x=s(t), 0\leq t\leq T$, we find that there exists a constant $C$ such that

$|s(t_2)-s(t_1)|\leq C|t_1-t_2|^{\beta},$

where $\beta=\frac{1+\alpha}{2}$.

It follows that $s(t)$ is Hölder continuous on $[0, T]$ with a Hölder exponent $\beta\in (\frac{1}{2}, 1)$.

Theorem 5.2. The free boundary $s(t)$ satisfies

$s'(t)=-\frac{ \sigma _{H}(t)(\phi_{xx}(s(t)-, t)-\phi_x(s(t)-, t))}{\phi_{x}(s(t), t)-\gamma e^{s(t)}}, 0\leq t\leq T.$

Moreover, $s(t)\in C^{\infty}[0, T]$, provided that $\sigma_{L}(t)$ and $\sigma_{H}(t)$ are smooth on $[0, T]$.

Proof. By Theorem 5.1, we can define $Q_{T}^{L}$ and $Q_{T}^{H}$ as in Section 3. From the theory of parabolic equations (Ladyženskaja et al., 1968), we know that $\phi(x, t)$ is smooth in $Q_{T}^L$ and $Q_{T}^H$, respectively. Moreover, $\phi_x(x, t)$ is continuous up the boundary $x=s(t)$ from the left-hand side and the right-hand side on $[0, T]$.

To prove the further regularity of $s(t)$, we note that $\psi(x, t)=\phi_x(x, t)$ is a classical solution of the following free boundary problem

$\psi_t=\frac{\partial }{\partial x}[\frac{1}{2}\sigma ^2 (\psi_{xx}-\psi)], \hspace{1cm} (x, t)\in Q_{T}\backslash \Gamma_{T}, \\ \psi(s(t)-, t)=\psi(s(t)+, t), \hspace{1cm} 0<t<T, \\ \sigma _{H}(\psi_x(s(t)-, t)-\psi(s(t), t))=\sigma _{L}(\psi_x(s(t)+, t)-\psi(s(t)+, t)), 0\leq t\leq T, \\ s'(t)=-\frac{\sigma _{H}(t) (\psi_{x}(s(t)-, t)-\psi(s(t), t))}{\psi(s(t), t)-\gamma e^{s(t)}}, \hspace{1cm} 0\leq t\leq T, \\ \psi(x, 0)=\phi_{0}^{'}(x), \hspace{1cm} -\infty<x<\infty.$

We can apply the classical technique for the Musket problem Evans (1978) to obtain $s(t)\in C^{\infty}[0, \infty]$.

Theorem 5.3. $s(t)$ is non-increasing on $[0.T]$.

Proof. We go back the approximate problem (24)-(25). Note that $\phi_{0}^{'}(x)-\phi_{0}(x)=0$ if $x < 0$ and $\phi_{0}^{'}(x)-\phi_{0}(x)=-1$ if $x>0$. We can construct a smooth approximation for $\phi_0(x)$ such that

$\phi_{\varepsilon 0}^{''}-\phi_{0}^{'}(x)<0, -\infty<x<\infty.$

Let $w(x, t)=\phi_{\varepsilon t}(x, t)$. Then $w(x, t)$ satisfies

$w_t=\frac{1}{2}\sigma ^2[w_{xx}-w_{x}]+a(x, t)w, (x, t)\in Q_T.$

where

$a(x, t):=\frac{ (\sigma ^{2}(t, \phi))_{t} }{\sigma ^{2}(t, \phi)}.$

Moreover,

$w(x, 0)=\frac{1}{2}\sigma _{\varepsilon }(0, \phi_0)^2[\phi_{0\varepsilon }^{''}(x)-\phi_{0\varepsilon }^{'}(x)]<0, -\infty<x<\infty.$

By the maximum principle, we see $w(x, t)\leq 0$ on $Q_T$. After passing the limit, we see

$\phi_{xx}(x, t)-\phi_x(x, t)\leq 0, (x, t)\in Q_{T},$

in the $L^2$-sense. On the other hand,

$\phi_x(s(t), t)-\gamma e^{s(t)}<0, 0\leq t\leq T,$

we see from the representation of $s(t)$ that

$s'(t)\leq 0, \hspace{1cm} t\in [0, T].$

---

6. Numerical Example

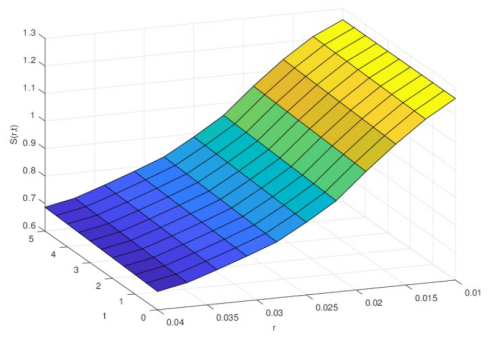
The numerical solution of the Eq.(17), (21) can be calculated by using a standard PDE method. However, we are more interested in the. For convenience, the free boundary is denoted by $s(r, t)$, which depends on the interest rate $r$.

In order to draw the graph $s(r, t)$, we use the explicit difference scheme (Jiang, 2005), which is equivalent to the well-known the Binomial-Tree method. The steps of the computation are as follows:

1. Discrete $t\in(0, T)$ by $t_i=T -i\Delta t$, where $T=t_0, ..., t_I=0$, $I=10,$ $\Delta t =\frac{t_I-t_0}{I};$ $r\in(0.01, 0.04)$ by $r_k=0.01 + k\Delta r$, where $0.01=r_0, ..., r_K=0.04$, $\Delta r =\frac{r_K-r_0}{K};$ in space using a mesh with difference $\Delta x$: $0=x_0, ..., x_j=2$. And at every point $(t_i, x_j, r_k)$, denote the function $\phi(t_i, x_j, r_k)$ by $\phi_{i, j, k}$.

2. For $t_0=T$, $\phi_{0, j, k}=\min(x_j, F)$, then solve $\phi_{i, j, k}$ by finite difference scheme:

$\begin{eqnarray*} \phi_{i, j, k}=&&\phi_{i-1, j, k}+\frac{\sigma ^2\Delta t}{2\Delta x^2}x_j^2[\phi_{i-1, j-1, k}+\phi_{i-1, j+1, k}-2\phi_{i-1, j, k}] \\ &&+\frac{\sigma _r^2\Delta t}{2\Delta r^2}r_k^2[\phi_{i-1, j, k-1}+\phi_{i-1, j, k+1}-2\phi_{i-1, j, k}]\\ &&+r_kx_j\frac{\Delta t}{2\Delta x}[\phi_{i-1, j+1, k}-\phi_{i-1, j-1, k}]\\ &&+a(\theta-r_k)\frac{\Delta t}{2\Delta r}[\phi_{i-1, j, k+1}-\phi_{i-1, j, k-1}]-r_k\phi_{i-1, j, k}\Delta t\\ &&+\frac{\sigma \sigma _r\Delta t\rho x_j}{4\Delta r\Delta x}[\phi_{i-1, j+1, k+1}1-\phi_{i-1, j+1, k-1}-\phi_{i-1, j-1, k+1}\\ &&+\phi_{i-1, j-1, k-1}], \end{eqnarray*}$

where

$\sigma = \left\{\begin{array}{ll}\sigma _L, &\mbox{ if }\phi_{i-1, j, k}>\gamma x_k, \\ \sigma _H, &\mbox{ otherwise, }\end{array}\right.$

Denote the joint points to be $s_{j, k}$, which represents the approximated credit rating migration joints.

3. Repeat the above process.

4. Draw the graph based on $\{s_{j, k}\}$ in space $r.S, t.$

Now we have the 2-d free boundary interface.

The numerical solution of the free boundary is shown in Figure 1, where the parameters and the ranges of the variables are chosen as follows

$a=1, \, \theta = 0.03, \, F=1, \, \gamma=0.8, \, \\ \sigma_L=0.4, \, \sigma_H=0.2, \, \sigma_r=0.3, \, \rho=0.5, T=5, \\ r\in(0.01, 0.04), \quad \ t\in(0, 5).$

From the graphs, we see that a free boundary $y=s(r, t)$ divides the region into two parts: high and low rating regions. Moreover, the free boundary is decreasing with respect to $r$ and increasing with respect to $t$ as expected (see Theorem 4.1 and Theorem 5.2), where $t$ is the original life time of the bond. From the graph Fig. 1, we also see that the change of free boundary is more sensitive with respect to the interest rate than that of time.

---

7. Conclusion

In this paper, we derived a new model for pricing corporate bonds with credit-rating migration and a stochastic interest rate. The new model consists of a coupled system of partial differential equations. By employing a dimension-deduction technique, we obtain a new free boundary problem. By using various analysis techniques for partial differential equations, we established the existence, uniqueness and regularities of the solution under certain assumptions. Moreover, some properties of the solution are derived. A $C^\infty$-regularity of the free boundary is proved. Their financial implications are explained. The model is new to our knowledge and is a big improvement for the current model. The analysis is delicate. Finally, numerical simulations are also presented.

---

Acknowledgement

This work is supported by National Natural Science Foundation of China (No.11671301).The authors would like to thank Professor Bei Hu for some discussions during the preparation of the paper. Many thanks also go to anonymous referees for their comments and suggestions. Chen and Yin would like to thank the hospitalities of Tongji University during their visiting where the part of this work was completed.

---

Conflict of Interest

All authors declare no conflicts of interest in this paper.

---