1. Introduction
The increasing development of land dedicated to viticulture and the growing demand for grapevines has prompted the search for information indicating the best viticultural practices to protect both natural resources and grape quality [1,2,3]. Of the several viticultural practices that wine grape growers need to be concerned about, soil and vineyard canopy management have a particular relevance because of the impact they can have on grapevine vegetative and reproductive development [4,5].
Soil management practices include the amendments used for improving and maintaining the soil's fertility and reducing environmental impact. An important contribution to fertility and physical structure of soil is based on use of peat (PT) and compost (CM), two natural soil amendments with potential beneficial effects on plant growth [6,7]. Peat is an expensive and non-renewable material. Thus, the conservation of peatlands has gained importance and currently the peat used as a growing media is considerably limited. Research for finding low-cost and environmentally friendly alternative products is encouraged [8]. Compost originated from different natural resources such as decayed plant matter is another source of nutrients for improving the soil fertility [9,10].
Biochar (BC), produced through the pyrolysis of feedstocks such as wood chips, manure and pruning residues has attracted the attention in horticultural practices[11]. Much research has been done on the addition of BC to soil systems with contrasting effect on plants [12]. Among BC positive effects of application on agricultural soils, those related to changes of soil physical and chemical parameters leading to increased water-holding capacity, reduction of bulk density, and addition of cation exchange sites are better known [13]. In addition, the positive effects of the interactions between BC and mineral fertilization with nitrogen (N) and phosphorus (P) on plant growth have showed[14]. By this interaction, reduced leaching was observed and hence more efficient use of nutrients [15]. However, the critical effect of biochar amendment is due to alkalinization of soil and reduced nutrient availability for plants [16,17]. The intrinsic heterogeneity of biochars, due to the feedstock sources and temperature conditions, lead to a large variability of effects on soil properties and productivity [18] as a consequence, the biochar effects on crop production are variable. In addition, the presence of specific BC components (e.g. ash content) also influences application rate. High ash can constitute a source of nutrients for crops, and it should be taken into consideration when managing soil fertility at the field level. Positive effects of BC application were observed on crop yields with rates of 5-50 tons of biochar per hectare. Even though this is a large range, the treatment with the higher biochar application rate showed the better results [19].
Although a number of studies have been carried out illustrating the biochar effect on herbaceous crops [20,21], few studies have been performed on vineyards [22,23,24]. In vinestocks a high correlation between the developments of the underground and aboveground parts has been demonstrated [25,26], thus our hypothesis is that the potential benefits of BC for vine growth may be leveraged in the nurseries i.e., working with young bare root cuttings. Pot experiments enable to test plant response to different substrates on the same climatic conditions (i.e., greenhouse) or test different environmental factors (i.e., heat, drought, substrate pH, salinity). Recent research conducted with vine cuttings grown in pots are reported [26,27]. In such concerns, peat is recognized by many authors as typical growing media [11], and biochar could be an additive and or substitute when mixed with soil or compost [11]. Peat and biochar have been used in pots experiments with typical greenhouse plants such as Pelargonium [28], mini-sunflower [11], pepper [29], Calathea [30,31], radish [32] and vines [33].
Plant responses to the addition of BC to substrates can be similar to those found with standard substrates containing peat [30,34] with the benefits of creating a beneficial environment for microbes. Dumroese et al. [35] enhanced hydraulic conductivity and improved water availability by adding 25% biochar to peat. Biochar was also successfully used to replace perlite in growing media [36]. The chlorophyll and anthocyanin contents are a reliable indicator for estimating the impact of various stress factors (abiotic, biotic, xenobiotic) a plant [37]. Therefore, the major goal of the present study was to investigate the short-term effect of biochar and compost, applied at different rates to peat growing media, on soil properties such as pH and electrical conductivity (EC) and on development of rooted grapevine grown in a greenhouse experiment. Moreover, the present study also intends to display how chlorophyll (CHL), flavonoids (FL), anthocyanin (ANT) content, the nitrogen balance index (NBI), and canopy biomass may be useful tools to evaluate the effect of amendments on the plant development at nursery level.
2. Materials and Methods
2.1. Experimental setup
The experimental setup derives from the factorial combination of three types of amendments (biochar, BC, compost, CM, and peat, PT) with four application rates and time, on the growth of one year old bare root grapevine cuttings. The experiment was organized in a complete randomized block with eight replicates Biochar was produced by fast pyrolysis at ≈ 500-750 ℃ from southern yellow pine wood. Biochar was washed with water to reduce the dust content and then was oven-dried at 60 ℃ for 72 h. Biochar was homogenized by crushing and sieving ( < 1 cm) before using in the experiment. Compost (OMRI certified) was produced by a mix of chicken litter, food waste, horse bedding and wood chips. Peat from Sunshine # 4 (Sun Gro Horticulture, Agawarn, MA) was used as soilless potting media. Perlite was added in place of sand and dolomitic limestone was used as supplies of magnesium and calcium.
2.2. Chemical and DRIFT characterization of substrates
For each substrate, the moisture content was determined on an oven dry weight basis at 105 ℃ [38] (Table 1). The pH was measured potentiometrically in a 1: 5 (w/v, dry weight basis) water solution [38] and the electrical conductivity (EC) in saturation paste extraction [38]. Content of total C and N was performed by using CNS Vario Macro elemental analyzer (Elementar, Hanau, Germany). The C value was corrected subtracting the inorganic C content from carbonates. Carbonates were determined by the calcimeter method and by gravimetric loss of CO2 [39]. Total content of P, and metals (Ca, Mg, Zn, Fe, Mn and Cu) were determined after acid digestion with ultrapure nitric acid (Merck, Darmstadt, Germany) [40]. Concentration of these elements was determined by inductively coupled plasma atomic emission spectroscopy (ICPAES) (Spectro CirosCCD, Kleve, Germany).
The spectra of the three substrates were performed by using a Bruker TENSOR series FT-IR spectrophotometer (Bruker, Ettlingen, Germany) equipped with an accessory for diffuse reflectance (DRIFT) (Spectra-Tech. Inc., Stamford, CT, USA). Each spectrum was collected as Kubelka-Munk units using KBr (Aldrich Chemical Co. Milwaukee, WI, USA) as background reference. The measurements were recorded over the range of 4000-400 cm−1 at 4 cm−1 and 64 co-added scans. All samples were ground into powders prior to spectral acquisition. Second derivative was applied by using Grams/386 spectroscopic software (Galactic Industries, Salem, NH, USA) in order to enhance the separation of overlapping peaks.
2.3. Plant material
The experiment was carried out in a polycarbonate-glazed greenhouse at the University of Missouri (Columbia, MO, USA). Temperature ranged between 20-25 ℃ and relative humidity between 70-85%, shaded by plastic black netting during the summer months. As experimental plant, one-year-old bare root cuttings of Valvin Muscat grapevine (NY62.0122.01) grafted on 1103P from Double A nursery (New York) were used. Rooted cuttings were planted in 20 L black plastic pots (∅ 37 cm, height 27.5 cm) with four holes (∅ 0.5 cm) at the bottom and containing BC or CM mixed with PT. The application rates were 0% (= control = 100% PT), 30%, 70%, and 100% w/w on a dry weight basis BC or CM, plus the remaining part with media, and the mixtures (15+15%, 35+35% and 50+50%). These corresponded to an application rate of 90, 110 and 160 t ha−1 in the top 10 cm depth for BC, and 139, 231 and 300 t ha−1 in the top 10 cm depth for CM.
Vines were cultivated for 125 days, pruned at two shoots per vine, irrigated ones a week and fertilized with N, P and K at rate of 250, 108 and 2018 mg kg−1, respectively (Excel Cal Mag fertilizer; Scotts, Marysville, OH, USA). At 70th day after planting (DAP), 5 g of iron sulfate was supplied to the mixtures to reduce the pH. At 60, 75, 90, 105 and 120 DAP, pH and EC of the substrates were measured with the Pour-Through extraction method [41,42]. At 90, 105 and 120 DAP, CHL, FL, ANT content and NBI were determined by using a portable leaf-clip device (Dualex, Force-A, Orsay, France). The plants were harvested and removed from the pots and separated into above-and belowground fractions. Leaves, stems and roots were stored in individual paper bags and dried at 70 ℃ for 72 h and then weighted. Plants growth in pots at two weeks after planting are displayed in Figure 1.
2.4. Statistical analysis
All examined variables were tested for normality and homoscedasticity by Shapiro-Wilk's and Levene's tests, respectively, and log transformed when necessary to satisfy assumptions required by parametric statistics. Three-way ANOVA was carried out with substrate type (biochar, compost, peat, and mixes), application rate (0, 30, 70 and 100%), and time (60, 75, 90, 105, 120 DAP for the soil parameters, and 90, 105, 120 DAP for the plant parameters) as factors. Statistical differences between means were processed with Duncan and Student-Newman-Keuls tests [43]. The level of significance (P) used was 0.05. All statistical analysis was carried out using CoHort Software (Birmingham, UK).
3. Results
3.1. Chemical features of substrates
Table 1 shows the values of pH, EC and elemental analysis for each substrate.As expected, BC showed an alkaline pH (9.7) with respect to CM and PT. It is interesting to note that the EC was instead higher in CM with respect to BC. Both BC and PT differed from CM for the highest C content while BC was very low in N and P. Nutrients such as K as well as Fe, Mn and Zn were much more concentrated in CM with respect to BC and PT.
Table 1. Main chemical parameters of biochar, compost and peat-based media used in the plant growth experiment (mean value, ± SE, n = 3).
| Parameter |
Units |
Biochar |
Compost |
Peat |
| Moisture |
% |
60 ± 0.31a* |
32 ± 4.56b |
32 ± 1.89b |
| [H+] |
pH |
9.73 ± 0.01a |
7.55 ± 0.01b |
6.07 ± 0.01c |
| Electrical conductivity (C) |
dS m−1 |
1.89 ± 0.22b |
2.39 ± 0.83a |
1.21 ± 0.03b |
| Organic carbon (OC) |
g kg−1 |
358.7 ± 2.2a |
83.6 ± 8.3c |
221.7 ± 0.3b |
| Total nitrogen (N) |
g kg−1 |
0.48 ± 0.01b |
5.22 ± 0.04a |
4.78 ± 0.02a |
| C:N ratio |
|
750 ± 37a |
14.80 ± 0.36c |
46 ± 2.1b |
| Phosphorus (P) |
g kg−1 |
0.20 ± 0.01b |
0.84 ± 0.01a |
0.49 ± 0.01b |
| Potassium (K) |
g kg−1 |
0.97 ± 0.03b |
2.45 ± 0.057a |
0.48 ± 0.002c |
| Calcium (Ca) |
g kg−1 |
2.19 ± 0.15b |
11.63 ± 0.35a |
11.35 ± 0.01a |
| Magnesium (Mg) |
g kg−1 |
0.56 ± 0.01c |
1.65 ± 0.11b |
4.09 ± 0.013a |
| Zinc (Zn) |
mg kg−1 |
1.15 ± 0.08c |
37.60 ± 3.85a |
10.35 ± 1.95b |
| Iron (Fe) |
mg kg−1 |
264 ± 45b |
3650 ± 260a |
606 ± 64b |
| Manganese (Mn) |
mg kg−1 |
94.8 ± 16b |
206.4 ± 24a |
38.7 ± 2.9c |
| Copper (Cu) |
mg kg−1 |
3.18 ± 0.33b |
8.02 ± 1.63ab |
9.72 ± 2.71a |
| *In the same row different letter indicates different mean at P ≤ 0.05 as per the Duncan test. |
3.2. Spectroscopic features of substrates
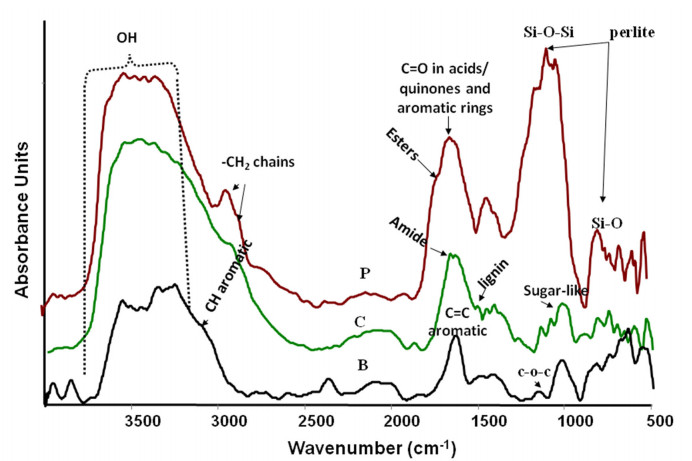
The main functional groups of each substrate were detected by using FT-IR spectroscopy, as displayed in Figure 2. In all spectra there are vibrational bands which are assigned to the same functional groups such as the broad peak appearing between 4000-3000 cm−1 is ascribable to (O-H) vibrations. The position and shape of this region suggest that the OH groups are involved in hydrogen bonding. The residual water of the raw material could take part in the formation of hydrogen bonds. The peaks appearing at 2930 and 2855 cm−1 coupled with those at around 1463 and 1413 cm−1 are due to (C-H) stretching and bending motions in aliphatic substances, respectively.
In more detail, BC spectrum (Figure 2, black line) shows a shoulder at 3055 cm−1 assigned to (=CH) stretching vibration in aromatic rings. The stronger band at 1638 cm−1 is produced by aromatic C=C stretching vibrations. Usually, the C=C bonds in charred materials are not IR-active; however, the substitution of C with N or O in the aromatic bonds breaks the symmetry of rings, promoting bond dipoles and producing IR-active bands [44]. The broad band between 1100 and 1300 cm−1 is caused by C-O stretching vibrations in aliphatic and aromatic ether groups and by C-C stretching vibrations.
In the CM spectrum (Figure 2, green line) the peaks between 1700 and 1600 cm−1 are ascribable to C=O vibration of carboxylic acids, amides, ketones and/or quinones and the (C=C) vibration of aromatic components [45]. The presence of a weak peak at 1507 cm−1 is typical of aromatic skeletal vibration in lignin. The peaks between 1190 and 900 cm−1 are due to polysaccharides and mineral compounds.
Peat spectrum (Figure 2, brown line) is characterized by a shoulder at 1738 cm−1 due to (C=O) stretching in esters while the strong band at 1651 cm−1 is assigned to carbonyl stretching in quinones and carboxylic acids [46]. The shoulder peak at 1224 cm−1 is attributed to (C-O) stretching vibration in aryl ethers, carboxylic acids and esters. Furthermore, the region from 1153 to 865 cm−1 is typical of polysaccharides. However, the bands at 1085 and 800 cm−1 are also ascribable to (Si-O-Si) stretching and (Si-O) bending vibration of perlite a component added to the substrate.
3.3. Influence of different mixtures in potting media on pH and EC
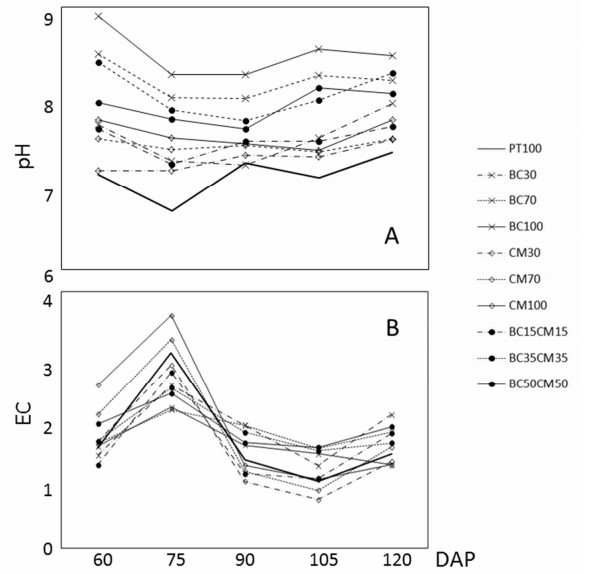
ANOVA analysis revealed that pH and EC were affected by the type of substrate (P ≤ 0.001), application rate (P ≤ 0.001) and days after planting (P ≤ 0.001). Significant were also the first (P ≤ 0.001) and second grade factors interaction (P ≤ 0.01). A considerable alkaline effect was observed in potting mix with BC and CM with respect to the alone PT (P ≤ 0.05) (Table 2). Application rate increased the pH with values ranging from 7.1 in untreated to 8.0 at the highest dose (P ≤ 0.05) (Table 2). Concerning the days after planting, the pH was slightly higher (7.8) both at the initial (60 DAP) and final period (120 DAP) than at intermediate period (75, 90 and 105 DAP, ca 7.5) (P ≤ 0.05) (Table 2). Note, BC at dose 100% kept higher pH values than the other treatments (Figure 3A and Table S1) while PT 100% had the lowest ones (Figure 3A and Table S1) (substrate × dose × DAP interaction significant at P ≤ 0.01).
Table 2. Reaction (pH) and electrical conductivity (EC) of the substrates of the potted experiment. Mean value per substrate, dose and days after planting 1-year-old bare root cuttings of grapevine.
| FACTOR |
|
pH |
EC (dS m−1) |
| Substrate |
Peat (PT) |
7.1d* |
1.59b |
|
Biochar (BC) |
8.0a |
1.78a |
|
Compost (CM) |
7.4c |
1.69ab |
|
BC+CM |
7.8b |
1.78a |
| Rate (%) |
0 |
7.1D |
1.59B |
|
30 |
7.4C |
1.67B |
|
70 |
7.8B |
1.79A |
|
100 |
8.0A |
1.76A |
| DAP |
60 |
7.8 a |
1.69 b |
|
75 |
7.5 c |
2.61 a |
|
90 |
7.5 c |
1.21 d |
|
105 |
7.6 b |
1.48 c |
|
120 |
7.8 a |
1.61 b |
DAP, days after planting.
*In the same column differences among substrate, dose (capital letters) and DAP (italicized letters) were at P ≤ 0.05 as per the Duncan test. |
Concerning EC, BC and BC plus CM mixture gave significantly higher values (1.78 dS m−1) with respect to PT (1.59 dS m−1) (p ≤ 0.05) (Table 2). High applications rates showed higher EC (1.79 and 1.76 dS m−1) than low doses (1.59 and 1.67 dS m−1) (p ≤ 0.05) (Table 2). Concerning the time sequence, EC had the highest and lowest value at 75 DAP and 90 DAP, respectively (2.61 dS m−1 and 1.21 dS m−1) (P ≤ 0.05) (Table 2). As for pH, also for EC the substrate × dose × DAP interaction was significant (P ≤ 0.01). In particular, at 75 DAP EC was in the following order of: CM 70% (3.25 dS m−1) ≥ PT 100% (3.04 dS m−1) ≥ CM 30% (2.85 dS m−1) ≥ BC 100% (2.15-2.54 dS m−1) (Figure 3B and Table S2). CM plus BC had intermediate values with respect to PT 100% and BC 100% (2.40-2.73 dS m−1). Instead the lowest EC was found at 90 DAP by CM 30% (0.75 dS m−1) (Figure 3B and Table S2).
3.4. Influence of different mixtures in potting media on grapevine plant growth
The dry weight of true leaves, lateral leaves, roots, primary shoots, total scion biomass and total biomass were influenced by the different mixtures in potting media (P ≤ 0.001), rate (P ≤ 0.001) and treatment per rate interaction (P ≤ 0.01), whereas the post-hoc tests showed significant differences between the treated and control plants.
No interaction between substrate and application rate were found when plant dry weight was measured, consequently only the main effects are reported in Table 3. In general, BC and CM induced a detrimental effect on the dry weight of true leaves (0.7 and 0.6 fold PT), lateral leaves (0.6 and 0.5 fold PT), roots (0.6 and 0.8 fold PT) and total biomass (0.7 and 0.8 fold PT) (Table 3), whilst BC slightly enhanced the weight in the primary shoot (1.2 fold PT) (Table 3). Intermediate values were instead observed for BC plus CM mixtures with respect to the PT and the single substrates. A broad detrimental effect on the dry weights was also observed ranging from low to high doses (Table 3). Indeed, BC 70% and BC 100% induced the lowest dry weight of true leaves (0.3 fold PT) (P ≤ 0.05) and roots (0.4 fold PT) (P ≤ 0.05) (Table S3) whereas BC 30% was not statistically different from PT. As a consequence the ratio between total scion and root was 3.2 in BC 30%, 2.6 in PT, and 2.4 in CM treated vines (Table S3).
Table 3. Effects of substrates on potted grapevines grown. Dry weight of true leaves, later leaves, roots, primary shoot, total scion biomass and total biomass at the end of trial. Mean value per substrate and dose.
| Factor |
Dry weight (g) |
| True leaves |
Lateral leaves |
Roots |
Primary shoot |
Total scion biomass |
Total biomass |
| Substrate |
|
|
|
|
|
|
| Peat (PT) |
65.3a |
25.2a |
42.0a |
22.4b |
112.8a |
154.9a |
| Biochar (BC) |
38.6c |
15.7c |
26.4c |
25.8a |
83.3c |
111.7c |
| Compost (CM) |
48.2b |
12.5c |
34.4b |
22.6b |
83.3c |
118.6c |
| BC+CM |
54.0b |
19.2b |
37.2ab |
24.7a |
97.0b |
134.0b |
| Rate (%) |
|
|
|
|
|
|
| 0 |
65.3a |
25.2a |
42.0a |
22.4a |
112.8a |
154.9a |
| 30 |
61.2a |
22.5a |
39.7ab |
25.0a |
108.7a |
148.4a |
| 70 |
46.8b |
16.1b |
34.0b |
24.2a |
88.3b |
123.3b |
| 100 |
32.2c |
7.4c |
24.2c |
23.9a |
63.3c |
88.7c |
| *Values with different letters indicate significant differences (P ≤ 0.05) as per the Duncan test. |
3.5. Influence on biochemical parameters
ANOVA revealed that the CHL, FL and ANT content and NBI were affected by substrate (P ≤ 0.001), dose (P ≤ 0.001) and days after planting (P ≤ 0.001), as well significant were the interactions.
BC enhanced ANT (1.9 fold PT) and NBI (1.3 fold PT) (Table 4), while CM slightly increased CHL (1.1 fold PT) and NBI (1.1 fold PT) (Table 4). BC plus CM mixtures reported intermediate effects with respect alone substrates. Concerning doses, high rates in general gave high ANT and NBI (1.5 and 1.2 fold PT, respectively) (Table 4).
Table 4. Chlorophyll (CHL), flavonoids (FL), anthocyanins (ANT) content and nitrogen balance index (NBI) on 1-year-old bare root cuttings of grapevine: mean values per substrate, rate and days after planting.
| Factor |
|
CHL (Dualex Unit) |
FL |
ANT |
NBI |
| Substrate |
Peat (PT) |
28.19b |
0.51a |
0.026c |
57.76d |
|
Biochar (BC) |
24.28d |
0.37c |
0.049a |
74.59a |
|
Compost (CM) |
29.84a |
0.49b |
0.024d |
64.98b |
|
BC+CM |
27.33c |
0.49b |
0.034b |
61.56c |
| Rate (%) |
100 |
25.73C |
0.41C |
0.040A |
70.81A |
|
70 |
27.34B |
0.43B |
0.037B |
72.78A |
|
30 |
28.30A |
0.52A |
0.030C |
58.42B |
|
0 |
28.19A |
0.51A |
0.026D |
57.76B |
| DAP |
90 |
27.44 b |
0.45 b |
0.033 b |
64.23 b |
|
105 |
27.96 a |
0.47 a |
0.039 a |
68.79 a |
|
120 |
26.45 c |
0.46 a |
0.032 c |
64.94 b |
DAP, days after planting.
*In the same column differences among substrate, dose (capital letters) and DAP (italicized letters) were at P ≤ 0.05 as per the Duncan test. |
In more detail, the vines grown with CM 70% had all the time sequence higher values in CHL (30.35, 31.47 and 30.14 Dualex unit) with respect to those treated with PT (28.34, 28.32 and 28.03 Dualex unit) and the other treatments (Table 5) (substrate × dose × DAP interaction significant at P ≤ 0.001). Moreover, BC 70% at 105 DAP and BC 100% all-time along sequence had high amount in ANT (Table 5) (substrate × dose × DAP interaction significant at P ≤ 0.001). NBI higher values were found in the vines treated with BC 70% at 105 DAP with respect with the vines treated with PT (89.45 versus 51.41 Dualex unit) (P ≤ 0.05) (Table 5). Higher values were also induced by BC 100% both at 105 DAP and 120 DAP (81.12 versus 51.41 and 82.79 versus 57.17 Dualex unit) (P ≤ 0.05) and by CM 70% at 105 DAP (86.55 versus 51.41 Dualex unit) compared to PT (Table 5) (P ≤ 0.05).
Table 5. Chlorophyll (CHL), flavonoids (FL), anthocyans (ANT) and nitrogen balance index (NBI) in 1-year-old bare root cuttings of grapevine: mean value (n = 8) of factorial combination of types of substrate (biochar, compost, peat-based media as control, and mixtures) with application rates (peat = control, 30, 70, 100%) along the time sequence (90, 105 and 120 days after planting).
| Substrate |
Rate (%) |
CHL (Dualex unit) |
FL |
ANT |
NBI |
| 90 (DAP) |
105 |
120 |
90 |
105 |
120 |
90 |
105 |
120 |
90 |
105 |
120 |
| Peat (PT) |
100 |
28.34d* |
28.32d |
28.03d |
0.43cd |
0.58ab |
0.51bc |
0.024g |
0.031f |
0.024g |
67.72de |
51.41f |
57.17ef |
| Biochar (BC) |
30 |
27.87de |
29.07c |
26.60f |
0.44cd |
0.49c |
0.41d |
0.033f |
0.037ef |
0.032f |
70.47d |
62.09e |
71.42d |
|
70 |
23.62g |
24.03g |
21.93h |
0.40d |
0.35e |
0.37e |
0.054c |
0.066a |
0.053c |
64.02e |
89.45a |
76.32c |
|
100 |
21.78h |
23.07g |
20.33h |
0.36e |
0.30ef |
0.28f |
0.060b |
0.057bc |
0.056bc |
62.51e |
81.12b |
82.79b |
| Compost (CM) |
30 |
31.00ab |
28.52d |
27.89de |
0.51bc |
0.55b |
0.58ab |
0.014i |
0.037ef |
0.024g |
63.41e |
55.87ef |
52.72f |
|
70 |
30.35b |
31.47a |
30.14b |
0.43cd |
0.40gh |
0.50c |
0.024g |
0.032f |
0.017hi |
73.24d |
86.55a |
69.06d |
|
100 |
29.50bc |
30.48b |
30.11b |
0.47c |
0.48c |
0.50c |
0.020gh |
0.024g |
0.016hi |
62.78e |
64.31e |
62.94e |
| BC+CM |
15+15 |
29.18c |
28.64d |
27.48e |
0.53bc |
0.60a |
0.54b |
0.025g |
0.037ef |
0.026g |
57.47ef |
49.02n |
51.29f |
|
35+35 |
29.17c |
29.66bc |
27.16e |
0.49c |
0.48c |
0.52bc |
0.026g |
0.026g |
0.032f |
60.77e |
72.43d |
52.98f |
|
50+50 |
23.83g |
26.19f |
24.51g |
0.41d |
0.43cd |
0.42d |
0.052c |
0.043d |
0.039de |
59.80e |
78.02c |
72.02d |
DAP, days after planting.
*Values with different letters indicate significant differences (P ≤ 0.05) as per the Duncan test. |
4. Discussion
The effects of biochar in growing media mainly arise from the stable C structures like benzene-type aromatic rings formed during the feedstocks pyrolysis as displayed by IR spectrum and by high alkaline pH [47,40]. It is possible that carbonates and other inorganic alkalis content in BC might be leached by water treatment before its use. We suppose that the high alkalinity of BC is due to the presence of organic functional groups that are capable of accepting protons, including functional groups bonded to condensed aromatic rings containing oxygen or nitrogen atoms. The positive correlation between the fused-ring aromatic structure and aromatic C-O groups and pH and the negative correlation between the aliphatic O-alkylated carbons and anomeric O-C-O carbons and pH found by Li et al. [48] well agree with our results. In addition, the weak absorbance values in the spectrum are indicative of high pyrolysis temperatures used for producing biochar. The spectral features of other substrates differed between them, in particular CM displayed a label band due to the lignin residue while in PT was dominant the presence of perlite. While the lowest C:N ratio in CM with respect PT and BC indicates a good proportion between soil mineralization and the humification process, the differences in functional groups might differently affect the pH and EC values [48]. The agronomic relevance is that a modification of soil pH towards alkaline condition reduce the bioavailability of nutrients such as phosphate and iron. This was not detected for PT because the pH value was close to neutrality and thus, a positive effect on soil nutrient status for some nutrients was expected.
Biochar and CM led to a general detrimental effect on the biomass production of vine plantlets. This result was unequivocally related to the high dosage of BC, probably for alkaline pH, and CM for high EC. The different behavior of CM can be also related to the content and composition of the mineral fraction. Our findings agree with those of other authors who found both increments and decrements in plants aboveground and belowground biomass varying with biochar application [21,23,49]. However, Steiner and Harttung [11] found no significant differences when compared the weights of mini sunflowers grown in potted media with 75% biochar and 25% biochar addition. Nevertheless, one interesting aspect of our results is that the total scion to root biomass ratio was stimulated by BC application with respect to both PT and CM, especially when BC was used at low dosages. This resulted from a decrease in shoot growth lower than root growth, probably because in short term roots were more susceptible than shoot to the new substrate induced conditions. Nonetheless, stimulation of dry mass accumulation in the vegetative parts likely driven by higher N availability [50], might confirm a direct role of BC in the nutrient supply to plants [51]. This suggests the idea that the yield response to biochar and compost is not simply due to a priming effect on soil organic matter decomposition finally leading to faster mineralization and enhanced nutrient availability to the crop. Another mechanism for the enhancement in the total scion biomass respect to root may have been into humic substances. Indeed, soil and compost contain humic like substances that might stimulate plant growth and metabolism having hormone-like influences [7,29,52].
From a biochemical point of view BC and CM led to significant increments in CHL, FL and ANT content and NBI in vine plantlets. The combined use of BC with CM appeared to have intermediate effects thus ameliorating those obtained by pure substrates. Therefore, the combination of biochar with organic fertilizers such as compost is most promising for agronomic performance [53]. The data might support the interaction between N and photosynthesis, as already been seen to be influenced by other C-rich amendments and biostimulants in other specie [54,55]. Chlorophyll, flavonoids and anthocyanin content are practical indicators of both potential photosynthetic productivity and plant vigor, which are related to the N concentration and serve as a measure of the response of crop to N fertilizer application and soil nutrient status [56]. In particular, the production and localization of anthocyanins in root, stem and especially leaf tissues may allow the plant to develop resistance to a number of environmental stresses [57]. Thus, although the amendments rate adopted in this study were not adequate for the shedding in the open field, our findings suggest that BC and CM may be of great relevance in nursery or at transplanting in soil surrounding roots giving greater vigor to plant. Our results corroborate with those of other authors who found positive effects of BC on pepper [29], durum wheat [21], oat [20], Pelargonium [28], tomato [51], and grape [24]. From an environmental concern, it is important to point out that the decay of biochar is extremely slow [58]. Therefore, carbonization decelerates the carbon cycle and sequesters C for relatively long periods of time. In addition, C emissions associated with the decay of peat would be avoided if biochar replaced peat.
5. Conclusions
On the basis of these results, biochar and compost have in the short period contrasting effects on nutrient availability since they increased pH and augmented EC in the potted grapevines. Nevertheless, biochar favored an increment of the total scion biomass to root ratio, especially when used at low dosage along with increments in CHL, FL and ANT content, which are indicators of plant vigor. From a management perspective, it is evident that biochar may turn raw material into plant health promoting compounds and potentially seems to be an efficient and environmental sustainable peat substitute in nursery/potted plant production.
Acknowledgements
This work is supported by the Missouri Wine and Grape Board. We thank Spencer Fiser for kindly improving the English of the manuscript.
Conflicts of Interest
The authors declare no conflict of interest.










 DownLoad:
DownLoad: