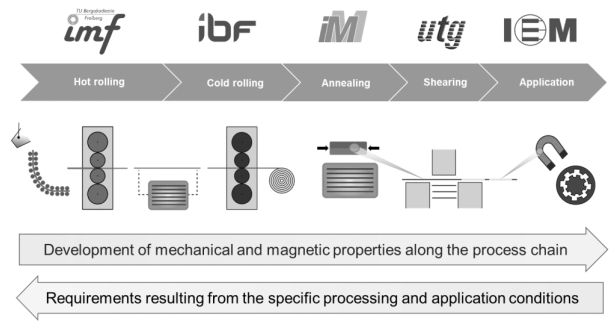
1. Introduction
Today, electrical energy is one of the most important forms of energy. Its relevance is going to increase even more in the lights of a continuing substitution of fossil energy resources, e.g., in electric mobility. With an increasing demand of environmental efficiency for industrial products and economic systems, highly efficient usage of energy resources and the reduction of losses is essential. Therefore, further improvements of the efficiency of electrical drives, which are responsible for the largest part of the total production and consumption of electrical power, is one of the major means in regard to reach the given targets of establishing a more ecofriendly industrial society. Factors limiting the efficiency of electrical machines are the losses caused by mechanical friction, electrical resistance of the conducting parts, eddy currents and magnetization changes. They are depending on the machine design, the usage conditions, and the used materials. As the potential for improvements by constructive measures and machine design is largely utilized, the focus needs to be shifted to the materials, mainly to the non-grain oriented electrical steels. In order to minimize specific magnetic losses and to maximize the efficiency, it is necessary to link the interdependencies of the processing parameters of the individual processing steps with the resulting texture and microstructure. Consequently, linking the material properties to the resulting electromagnetic behavior.
Considering, that the amount of iron losses and the magnetizability is depend on material as well as process based factors, but also on the machine design and operation mode, the task of an optimization gets rather complicated. It involves different fields of knowledge and technology with partly conflicting perspectives and scientific approaches hardly fitting to each other. Facing these challenges, since 2015 a group of researchers, including materials scientists, production engineering scientists and electrical engineering experts, are working in a joint initiative. The DFG (Deutsche Forschungsgemeinschaft) is the founder of this joint initiative, the research unit 1897 "Low-loss FeSi Sheet for Energy-efficient Drive", which aims at bridging the interdisciplinary gaps in order to develop an integrated approach of modeling the production, processing and application of electrical steel sheet.
2. Concept of interdisciplinary approach
Part of the experimental work focuses on the implementation and successful establishment of a continuous experimental process chain, starting from hot rolling to shear cutting of fully processed non-oriented electrical steel and application, see Figure 1. At the moment, the work concentrates on non-oriented electrical steel with 2.4 wt% Si. In the future we will investigate a material with 2.8 wt% Si. This interdisciplinary experimental process chain was and is important to investigate the various influencing factors and complex interactions between the process stages as well as the evolution of material properties within the process chain. Hence, different process variants, for example conventional hot forming after reheating or alternatively thin slap casting with direct hot forming from the casting heat, were realized. By modelling an entire process chain for manufacturing and processing of electrical steel sheets experimentally, unique conditions for the cross-process investigation of the influence of individual process parameters were created.
Based on several test campaigns, in which the entire process chain was realized with several variations of individual process parameters, the dependencies between individual process stages and the entire process chain could be clearly demonstrated. Numerous relationships between parameters of hot and cold rolling and final annealing were identified. Additionally, various cutting parameters and the resulting magnetic properties of the produced electrical sheet are determined and documented.
With regard to the individual processes, the understanding of the existing dependencies between selected process parameters, the microstructure, and the resulting material properties was improved significantly. Thus, a texture model based on the representative volume element (RVE) was developed and tested for cold working and prediction of texture development in the final heat treatment for the material under investigation. Furthermore, for the overall process modeling we defined the interfaces and transfer between the partial models. This also allows the coupling of the various model systems and the simulation of cross-process effects of parameter variations.
3. Research objective and results
3.1. Hot rolling and hot strip annealing
Generally, the electromagnetic and mechanical properties of non-oriented electrical steel sheets are influenced by the alloying elements, the grain size and the texture. The hardening and softening processes occurring during hot forming determine the microstructure of the hot strip, with a defined softening state, (sub-)grain size, texture and their distribution over the hot strip thickness. Since iron-silicon steels with silicon contents of more than 2 wt% are ferritic in the entire temperature range, starting from solidification temperature to room temperature [1], morphology and texture are not homogenized by phase transformations, but inherited through the entire production route. Therefore, it is important to optimize morphology and texture already in the hot rolling process. Additionally, the hot strip modeling and characterization has to be done layered. Overall, the hot strip characteristics influence the mechanisms of microstructural development in the subsequent production steps of cold rolling and heat treatment and have a direct influence on the magnetic properties.
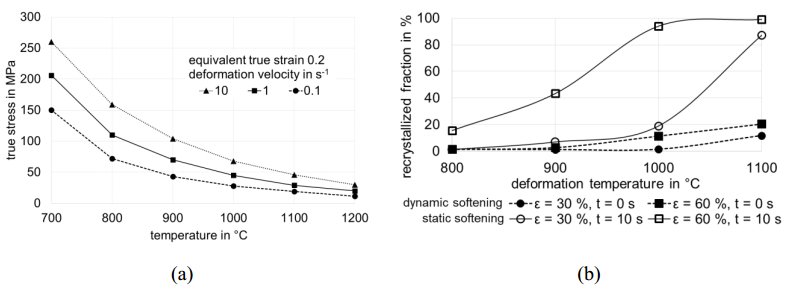
To simulate the hot rolling process, the layer model was used, as described in [2] and [3]. For simulating hot rolling, the knowledge of hardening and softening behavior is necessary. One of the required material parameters is the flow stress, for example at a defined deformation degree and temperature as shown in Figure 2a. Moreover, softening takes place during deformation as dynamic, and between passes as static, recovery and recrystallization. Depending on the material either recovery or recrystallization is more pronounced. In the case of bcc Fe–Si alloys dynamic recovery is the dominant softening mechanism during hot working, because of their high stacking fault energy. Thus, just a small dynamic recrystallized fraction evolves during hot rolling. Only hot rolling at temperatures of at least 1000 ℃ and interpass times of more than 10 s can achieve a fully recrystallized hot strip, see Figure 2b. These parameters are uncommon in the finishing section of a hot rolling mill. Finishing temperatures below 900 ℃ and interpass times below 3 s are typically. Therefore, and by an additional temperature gradient over the strip thickness, a heterogeneous microstructure and texture is the result of hot rolling, see Figure 3.
As known from cold rolling simulation studies (see section cold rolling), a strong cube texture in the hot strip seems to be preferable. For this reason, the hot rolling trials and simulation are aiming for process parameters to produce a hot strip with a strong cube texture over the complete strip thickness.
3.2. Cold rolling
Cold-rolled texture is affected by the initial state of hot strip and cold rolling strategies, at the same time it has significant influence on the final annealed texture. In addition to experimental study, a numerical study has been conducted in order to predict the cold-rolled texture and guide the design of rolling strategies. In previous studies [4,5], a crystal plasticity finite element (CPFE) model was created in ABAQUS/Standard using the Düsseldorf Advanced Material Simulation Kit (DAMASK)
[6] implemented as subroutines. It was then calibrated by applying the deformation gradient and the flow curves within the CPFE model. The model is furtherly used in this study to investigate the influencing parameters on texture evolution, namely the initial texture before cold rolling and the deformation type during cold rolling.
Figure 4 shows the influence of initial texture on the deformed texture. Three initial textures with different dominant components were investigated. After three rolling passes with 50% total height reduction, the deformed textures show two tendencies: The rolling with initial dominant α-/γ-fiber in case A and initial dominant Goss texture in case B result in a typical rolling texture that contains mainly α- and γ-fiber, while the texture containing dominant rotated cube shows a different behavior during rolling. Most of the desired cube texture in the case C remains after deformation and only a small proportion is converted to the undesired α- and γ-fiber. From this result, it can be seen that a strong cube texture as the initial texture before cold rolling might be desirable.
Furthermore, the influence of deformation type is studied. Model A in Figure 5 shows the texture evolution during simulated compression, while model B shows the texture development under simulated simple shear. After the deformation with a total strain of 0.58, the texture is dramatically changed in case B. A texture with dominant Goss texture component evolves as the undesired α- and γ-fiber disappear. Thus, this result shows a possibility to improve the cold-rolled texture by conducting large shear during cold rolling.
From the numerical studies, the cold-rolled texture might be changed and improved by using a hot strip with dominant rotated cube texture or by conducting large shear deformation during cold rolling. These simulated results will be examined with experimental work in the further study, for example, a hot strip with dominant rotated cube texture can be realized by proper hot rolling strategies, and the large shear during cold rolling can be achieved by asymmetrical rolling.
3.3. Heat treatment and crystal plasticity
The Institute of Physical Metallurgy and Metal Physics (IMM) is in charge of the annealing process and most of the texture measurements. In this context, experiments as well as simulations are carried out to analyze the influence of process parameters and initial microstructure/texture on the final microstructure/texture and therefore on the magnetic properties.
In this context, a direct coupling within the simulation chain has been achieved, similar to the continuous experimental process chain. The simulations consequently comprise two separate steps: first, the microstructure is generated with grains and subgrains by the algorithm called MicrostructureGenerator [7], available online [8], considering the experimental data on cold-rolled sheet material and simulations by crystal plasticity finite element modeling (CP-FEM) at the Institute of Metal Forming (IBF). In a second step, recrystallization and grain growth during heat treatment are simulated with the help of the level-set code called GraGleS2D, made available online [9]. The calculated results are in close agreement with the experimental results presented below and therefore form an integral part of the through-process modeling capabilities for electrical sheet developed in this cooperative project.
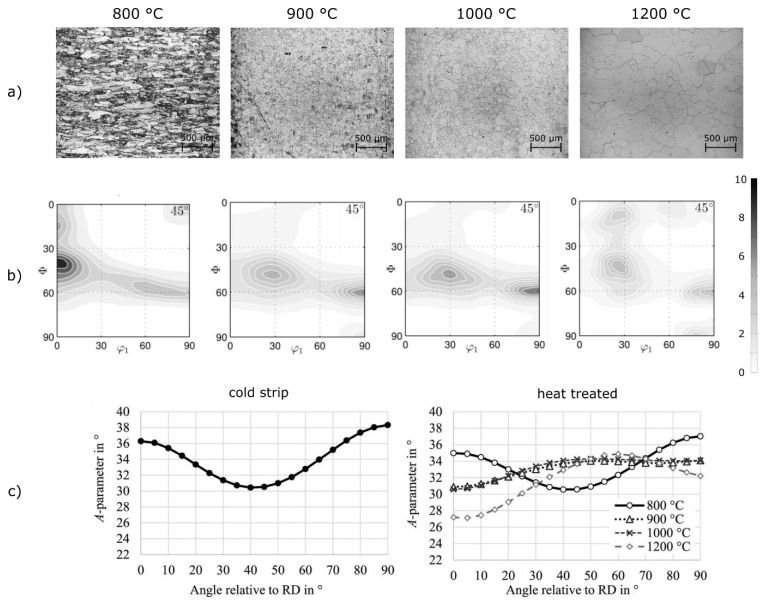
Figure 6 exemplifies the influence of annealing on the microstructure and texture evolution. Initially, a 64 mm continuously cast thin slab was hot rolled in 9 passes to 1 mm followed by cold rolling down to 0.25 mm.
All samples were annealed for 3 minutes. After annealing at 800 ℃ the microstructure still contains elongated grains in rolling direction (RD). Concerning the texture, the γ-fiber remains strong, the α-fiber is widely covered and there is some intensity in the Cube-ND45 component. For a full recrystallization, the annealing time was too short at this temperature and the initial microstructure. Therefore, texture remains close to that of the cold rolled samples. After annealing for 3 min at 900 ℃ and 1000 ℃, full recrystallization takes place. The recrystallized grains are small and equiaxed. The Cube-ND45 component and the γ-fiber disappear upon recrystallization and the α-fiber grew stronger locally within the ODF. Moreover, some intensity evolves in the Goss component. At 1200 ℃, grain growth occurs, the Goss component shows further, if slight, increase in intensity and a new orientation component forms at Φ = ~10°, φ1 = ~25°.
The A-parameter [10,11] may be calculated from these texture results in order to obtain a direct indication of the magnetic properties resulting from the different heat treatments. It describes the mean angle deviation of an individual texture from the easy magnetization axis < 100 > relative to the magnetization direction used in the measurement, e.g., the rolling or transverse direction or any angle in-between. The derived A-parameters for the cold-rolled strip and the sample annealed at 800 ℃ exhibit a similar dependence on angle relative to the rolling direction (Figure 6c). The minimum at an angle of 40° relative to the rolling direction results from the Cube-ND45 component. As this component vanishes at higher temperatures, the A-parameter dip also vanishes. Qualitatively, the curves of the recrystallized samples, i.e., those annealed at 900 ℃ and above, have a similar trend. Resulting from the changes in the α- and γ-fibers, the A-parameter is smallest in rolling direction and reaches its maximum at 45° relative to the rolling direction.
In the ongoing project phase, the research at IMM will expand from experiments and simulations on the microstructure evolution during heat treatment to crystal plasticity research of the given material in order to elucidate the effect of deformation of the recrystallized material during cutting on the final magnetic properties. The objective is to improve the understanding of the defect structure and grain boundary evolution during deformation. This is important since the increase of iron losses during the final shear cutting process cannot be explained based on the currently available continuum models owing to the importance of individual grains and grain boundaries in the fully recrystallized sheets, where the grain size approaches the sheet thickness.
3.4. Shear cutting
As is widely known, cutting electrical steels deteriorates their magnetic properties due to induced residual stress. It emerges because of excessive material deformation during the separation process. The amount of elastic and plastic strain next to the cutting line thereby depends on the mechanical and metallurgical material properties. Thereby, especially tensile strength UTS and maximum elongation Ag as well as grain size dg influence the deformation behavior of the electrical steel. Next to the silicon content these material properties result from the rolling and heat treatment strategy used during the production process. In addition shear cutting parameters, like the cutting clearance and the cutting edge wear state, result in alternating stress state distributions within the cutting affected zone [12].
Our interdisciplinary research concept allowed investigating the effect different rolling and heat treatment strategies have on the induced residual stress due to the shear cutting process. Therefore, outgoing from a 2.4 wt% silicon hot strip having a thickness s0, HS of 1 mm, cold strip having a thickness s0, CS of 0.5 mm and 0.25 mm is rolled and annealed at 1000 ℃ as well as 1200 ℃ for one minute. The resulting material properties of the non-grain oriented electrical steels produced are displayed in Table 1.
Table 1. Material properties.
| Material |
s0, HS (mm) |
s0, CS (mm) |
Heat treatment (℃) |
dg (µm) |
UTS (MPa) |
Ag (%) |
| 1 |
1 |
0.5 |
1000 |
73.3 |
524.4 |
0.192 |
| 2 |
1 |
0.5 |
1200 |
271.3 |
499.3 |
0.088 |
| 3 |
1 |
0.25 |
1000 |
74.0 |
449.1 |
0.149 |
| 4 |
1 |
0.25 |
1200 |
216.7 |
423.9 |
0.085 |
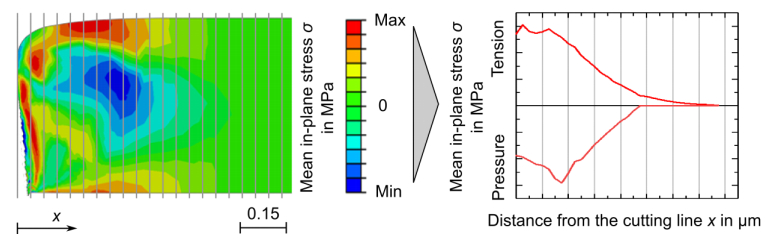
The extent of shear cutting is analyzed by separating the produced electrical steels with an industrial cutting tool presented in [13]. By using two different cutting clearances of 15 µm and 35 µm as well as two different cutting edge wear states on punch and die a correlation of shear cutting process parameters and electrical steel material properties is possible. Cutting force over punch travel curves and cutting surface parameters determined within the experiments are used to calibrate a numerical finite element shear cutting simulation in order to acquire the residual stress distribution next to the cutting line. Calculating mean values of tension and pressure stress over the blank thickness and plotting them against the distance to the cutting line x allows for an investigation of the shear cutting parameters on the resulting residual stress distributions to be carried out. This approach, already presented in [12], is shown in Figure 7.
Since different stress levels affect the magnetic properties up to different extents, deteriorations due to shear cutting get smaller with increasing distance to the cutting line [14]. Nevertheless, varying material thickness and material properties as well as different shear cutting parameters change the amount and distribution of induced residual stress. Therefore, such variations affect the magnetic properties up to different extents.
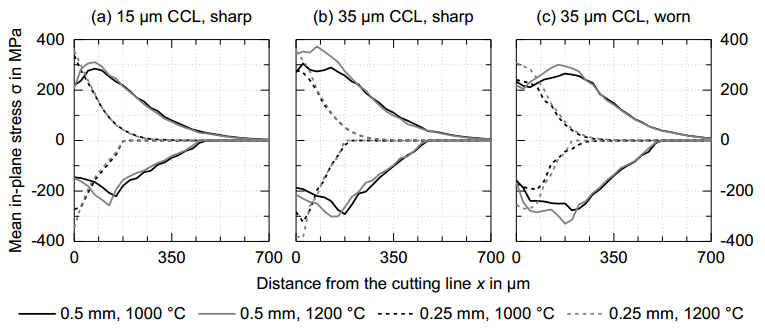
Changing the blank thickness from 0.5 to 0.25 mm significantly reduces the amount of induced residual stress as well as the width of the stress affected zone. This behavior can be observed regardless of the annealing temperature and shear cutting parameter setup used. The three plots in Figure 8 show, that for bigger cutting clearance and worn cutting edges both the amount as well as the penetration depth of the induced stress increase clearly. Independent of the shear cutting parameters used, annealing at a higher temperature negatively affects the amount of induced residual stress. The penetration depth although does not change.
Looking at the effect of shear cutting parameter variations, using small cutting clearances and sharp tools can reduce the amount of residual stress induced. In addition the extent of the stress affected area can also be minimized. As already mentioned above, the effect a shear cutting parameter variation has is particularly more noticeable for bigger sheet thicknesses.
3.5. Application
In order to increase the power density of electrical machines, the soft magnetic material needs to be utilized to its maximum capacity. With an increase of rotor speed, the magnetization frequency in the magnetic circuit increases. The frequency dependence of magnetic losses and magnetization behavior requires particular attention for the design of the magnetic circuit and the design of its magnetic material. A higher speed also contributes to higher requirements on the mechanical strength of the material. Centrifugal forces, respectively mechanical stress, act on the rotor material and therefore vary its magnetic properties in dependence of the rotor speed. Such effects of elementary magneto mechanical interaction have to be considered. Higher losses lead to higher temperature. Other coupling effects, such as the thermal conductivity must therefore be considered. Particular attention must be paid to the occurrence of hot spots, which have to be avoided.
It is well known that the production of electrical steel as well as the processing steps to manufacture an electrical machine's magnetic circuit determine the magnetic properties of the electrical steel laminations [10,15]. Strong interrelations between the manufacturing and the resulting behavior lead to the necessity of more comprehensive considerations [16]. In a first step, the primary material parameters affected during manufacturing and with a strong effect on the magnetic properties need to be identified and understood in order to be able to model the material. Through various test series on the experimental electrical steel processing chain as well as with various experiments on conventional industrial steel grades, the main parameters have been identified as, crystallographic texture, grain size with its distribution, local plastic deformation, global residual stress, local residual stress and homogeneity of the parameters across the sheet thickness. Here, the microstructure and texture on the microscale and the mechanical stress distribution and magnetic properties on the macro scale need to be considered alongside each other.
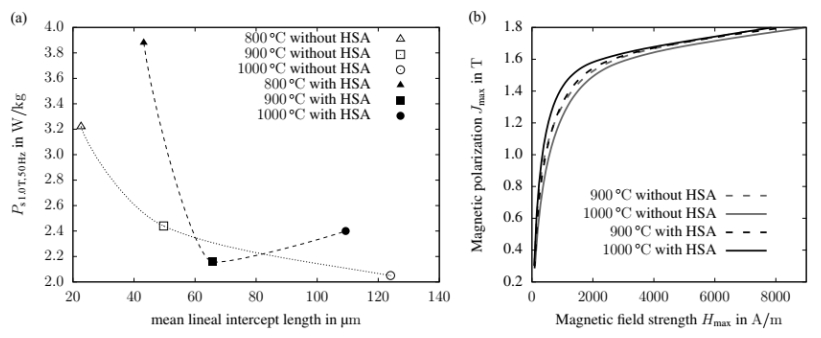
As mentioned before, the magnetic properties are closely related to the grain size and texture of the fully processed material. As can be seen from Figure 9a, the grains become larger with higher final annealing temperature for both, the samples with and without hot strip annealing. For the sample without hot strip annealing the magnetic losses are decreasing with increasing grain size. This has been observed in various research, where the effect of annealing temperature on grain size and texture has been studied in great detail [17,18]. In contrast, the sample with hot strip annealing has a reverse evolution of magnetic losses at a higher annealing temperature of 1000 ℃. One explanation for this behavior can be a distinct difference in the crystallographic texture. Often the variations of crystallographic texture by conventional processed electrical steel are small and of minor impact for the losses compared with the impact of grain size. However, unconventional process variation like cross rolling or HSA can have a greater effect on the texture. The residual stress within the samples can also be affected. A further indication of a different texture can also be deduced from the magnetization curves. The 900 ℃ annealed samples have a very similar magnetization curve, whereas the 1000 ℃ annealed samples deviate from each other. Although exhibiting higher losses the material with hot strip annealing has a distinctly better magnetization curve at 1000 ℃ like the material without hot strip annealing. A further look at the crystallographic texture is needed to deepen the understanding of the impact of HAS. From the experiments conducted, HSA has potential to alter specific magnetic properties, i.e., improve magnetization at the cost of magnetic loss. This highlights the complex interdependence of production parameters, their impact on material properties and the application requirements for non-oriented electrical steels.
Due to the different scales of the parameters it is a challenge to model the material appropriate. The identified parameters have a direct influence on the magnetic properties, which has been broadly studied and discussed in the scientific literature [19,20,21,22,23,24]. However, the interdependencies are still in need of a thorough description. For example, the crystallographic texture does not only affect the magnetic anisotropy but also the mechanical stress dependency [25]. Most material models cannot be directly applied because these interdependencies are not considered. The aim of the research group is to deepen the understanding between the material and magnetic properties of electrical steel. Also the description in applicable models for magnetization and losses with a semi-physical approach for the FE simulations of electrical machines [26,27,28] is an important aim. Having accomplished this aim, the effect of production and processing history can be incorporated and considered in the design process of electrical machines.
The previously presented approach of experimental variations on the laboratory processing chain [5,10] enables a detailed consideration of different material parameters, e.g., change of grain size or integral of mechanical residual stress from different cutting parameters. A crucial conclusion for the correlation between the intrinsic material parameters, e.g., grain size and texture and the magnetic properties is, that standardized characterization routines for general steel applications cannot be directly transferred to electrical steel. Conventionally, the grain size for approximately spherical grains is obtained from micrographs on the cross section. Thereby information on the grain length in different orientations of the sheet plane is lost. A consideration on surface micrographs is therefore better suited [29]. The macrotexture of thin sheets is conventionally obtained by X-ray goniometry on the surface or in the middle plane of a material. However, for electrical steel even small changes across the thickness of the laminations proof to be of interest for the magnetic properties. The same applies to grain size variations across the sheet thickness.
Another interesting interrelation is occurring between the annealing conditions, the grain size and the sheet thickness. When the sheet thickness is smaller than the grain size, grain growth is impeded, i.e., high annealing temperatures that lead to very large grains in thick material lead to smaller grains in thin sheets. The cutting force is also affected by the occurrence of large grains relative to the sheet thickness. The study on this effect is still ongoing. As previously mentioned, the influence of induced stress by cutting is also interdependent with the intrinsic factors [12]. The residual stress is not only induced by the processing, i.e., cutting of the laminations, but occurs also during manufacturing. Therefore, the interrelation of the residual stress from production with induced mechanical stress by processing has to be considered. Studies on the effect of external loading, plastic deformations and stress-relief annealing are conducted in order to deepen the understanding between the stress contributions. The experimental procedures are performed to understand vital relations between the magnetic properties und the manufacturing. In a next step, the relation between the material and its application needs to be deduced.
In [30], it has been shown that the appropriate material choice and subsequently the distribution of losses is affected by the driving cycle. The distribution of operating points and a machine related translation of this information into frequency and polarization combinations is the basis for an advanced material choice. Due to the conducted knowledge on the production and processing parameters and the semi-physical model approach for the relevant influencing factors, optimum material parameters can be concluded. Within the scope of the research group, a deduction of production and processing strategies of FeSi electrical steels, based on specific application requirements, is aimed to be enabled.
4. Conclusion and outlook
In order to create efficient non-oriented electrical steels, it is necessary to consider the individual processing steps and their influence on the final mechanical and electromagnetic properties, as well as the interdependencies of all processing steps. An understanding of the materials and process related potentials is achieved by detailed investigations within the presented interdisciplinary research concept with scientist from the field of material science, process engineers and electrical engineering, as presented in this article.
The relation between the production processes of the electrical steel, the processing of the material and the resulting magnetic properties is a vital step to enable improved material choice and material design to account for different application requirements. Depending on the machine topology (induction machine, permanent magnet machine, or other types), machine design (number of pole pairs, size, etc.), and torque-speed requirements (different driving cycle, operation) a particularly specified material behavior is required to optimize the material's utilization. At high motor speed the frequency dependence and iron losses are especially important, as for high torque the magnetic flux density in the air gap needs to be maximized. These requirements can be used to strategically produce materials, specifically designed and manufactured for defined application profiles which will be part of the experimental activities of the research unit until 2020. The modeling approaches will be used to determine specific processing parameters to produce the required material. This laboratory material will be analyzed in a novel magnetic testing equipment, designed to reproduce real loading conditions.
Acknowledgments
The authors are grateful for the financial support of this work being part of the research unit "FOR 1897 - Low-loss FeSi Sheet for Energy-efficient Electrical Drives" funded by the Deutsche Forschungsgemeinschaft (DFG, German Research Foundation) – 255681924, 255707264, 255711070, 218259799, 255713208, 255715828.
Conflict of interest
All authors declare no conflict of interest in this paper.









 DownLoad:
DownLoad: