1. Introduction

Singular values and the singular value decomposition play an important role in numerical analysis and many other applied fields ^{[3,4,5,6,7,8]}. First, we will use the following notations and definitions. Let $N :=\{1, 2, \ldots, n\}$, and assume $n \geq 2$ throughout. For a given matrix $A=(a_{ij}) \in \mathbb{C}^{n\times n}$, we define $a_i = |a_{ii } |$, $s_i = \max\{r_i, c_i \}$ for any $i \in N$ and $u_+ = \max\{0, u\}$, where

$r_i := \sum\limits_{j = 1, j \ne i}^n {\left| {a_{ij} } \right|}, \ \ c_i := \sum\limits_{j = 1, j \ne i}^n {\left| {a_{ji} } \right|}.$

In terms of $s_i$, the Geršgorin-type, Brauer-type and Ky-Fan type inclusion sets of the matrix singular values are given in ^[2,1,9,10], we list the results as follows.

Theorem 1 If a matrix $A=(a_{ij}) \in \mathbb{C}^{n\times n}$, then

(ⅰ) (Geršgorin-type, see ^[1]) all singular values of $A$ are contained in

$C(A):=\bigcup \limits_{i = 1}^n C_i \ \ with \ \ C_i=[(a_i-s_i)_+, (a_i+s_i)]\in R.$

(1.1)

(ⅱ) (Brauer-type, see ^[2]) all singular values of $A$ are contained in

$D(A):=\bigcup \limits_{i = 1}^n \bigcup \limits_{j = 1, j\neq i}^n \{z\geq 0: |z-a_i||z-a_j|\leq s_is_j\}.$

(1.2)

(ⅲ) (Ky Fan-type, see ^[2]) Let $B=(b_{ij}) \in \mathbb{R}^{n\times n}$ be a nonnegative matrix satisfying $b_{ij} \geq max{|a_{ij}|, |a_{ji}|}$ for any $i \neq j$, then all singular values of $A$ are contained in

$E(A):=\bigcup \limits_{i = 1}^n \{z\geq 0: |z-a_i|\leq \rho(B)-b_{ii}\}.$

We observe that, all the results in Theorem 1 are based on the values of $s_i = \max\{r_i, c_i \}$, if $r_i\ll c_i$ or $r_i\gg c_i$, all these singular values localization sets in Theorem 1 become very crude. In this paper, we give some new singular values localization sets which are based on the values of $r_i$ and $c_i$. The remainder of the paper is organized as follows. In Section 2, we give our main results. In Section 3, some comparisons and illustrative example are given.

---

2. New inclusion sets for singular values.

Based on the idea of Li in ^[2], we give our main results as follows.

Theorem 2 If a matrix $A=(a_{ij}) \in \mathbb{C}^{n\times n}$, then all singular values of $A$ are contained in

$\Gamma(A):=\Gamma_1(A) \bigcup \Gamma_2(A),$

where

$\Gamma_1(A):=\bigcup\limits_{i = 1}^n {\left\{ {\sigma \ge 0:\left| {\sigma ^2 - \left| {a_{ii} } \right|^2 } \right| \le \left| {a_{ii} } \right|r_i (A) + \sigma c_i (A)} \right\}},$

and

$\Gamma_2(A):=\bigcup\limits_{i = 1}^n {\left\{ {\sigma \ge 0:\left| {\sigma ^2 - \left| {a_{ii} } \right|^2 } \right| \le \left| {a_{ii} } \right|c_i (A) + \sigma r_i (A)} \right\}}.$

Proof. Let $\sigma$ be an arbitrary singular value of $A$. Then there exist two nonzero vectors $x = (x_1, x_2, \ldots, x_n)^t$ and $y = (y_1, y_2, \ldots, y_n)^t$ such that

$\sigma x = A^*y \ \ \mathrm{and} \ \ \sigma y = Ax.$

(2.1)

Denote

$|x_p| = \max\{|x_i|, \ \ 1\leq i \leq n \}, \ \ |y_q| = \max\{|y_i|, 1\leq i \leq n\},$

and $x_q$ is the q-th element in the vector $x =(x_1, x_2, \ldots, x_n)^t$.

The $q$-th equations in (2.1) imply

$\sigma x_q-\overline{a}_{qq}y_q=\sum\limits_{j = 1, j \ne q}^n {\overline{a}_{jq} } y_j,$

(2.2)

$\sigma y_q-a_{qq}x_q=\sum\limits_{j = 1, j \ne q}^n {a_{qj} } x_j.$

(2.3)

Solving for $y_q$ we can get

$\left( \sigma^2-a_{qq}\overline{a}_{qq} \right)y_q=a_{qq}\sum\limits_{j = 1, j \ne q}^n {\overline{a}_{jq} } y_j +\sigma \sum\limits_{j = 1, j \ne q}^n {a_{qj} } x_j.$

(2.4)

Taking the absolute value on both sides of the equation and using the triangle inequality yields

$\left| \sigma^2-|a_{qq}|^2 \right||y_q| \leq |a_{qq}|\sum\limits_{j = 1, j \ne q}^n {|\overline{a}_{jq}| } |y_j |+\sigma \sum\limits_{j = 1, j \ne q}^n {|a_{qj}| } |x_j| \notag \\ \;\;\;\;\;\;\;\;\leq |a_{qq}|\sum\limits_{j = 1, j \ne q}^n {|\overline{a}_{jq}| } |y_q|+\sigma \sum\limits_{j = 1, j \ne q}^n {|a_{qj}| } |x_p|.$

(2.5)

If $|x_p|\leq |y_q|$, we can get

$\left| \sigma^2-|a_{qq}|^2 \right| \leq |a_{qq}|c_q (A) + \sigma r_q (A).$

Similarly, if $|y_q|\leq |x_p|$, we can get

$\left| \sigma^2-|a_{pp}|^2 \right| \leq \left| {a_{pp} } \right|r_p (A) + \sigma c_p (A).$

Thus, we complete the proof.

Remark 1 Since

$\left| {a_{ii} } \right|r_i (A) + \sigma c_i (A) \leq \left(\left| {a_{ii} } \right| + \sigma \right)s_i,$

and

$\left| {a_{ii} } \right|c_i (A) + \sigma r_i (A) \leq \left(\left| {a_{ii} } \right| + \sigma \right)s_i.$

Therefore, the inclusion sets in Theorem 2 are always tighter than the inclusion sets in Theorem 1 (ⅰ). That is to say, our results in Theorem 2 are always better than the results in Theorem 1 (ⅰ).

Theorem 3 If a matrix $A=(a_{ij}) \in \mathbb{C}^{n\times n}$, then all singular values of $A$ are contained in

$\Delta(A):=\Delta_1(A) \bigcup \Delta_2(A),$

where

$\Delta_1(A)=\bigcup\limits_{i = 1, j=1}^n \left\{ {\sigma \ge 0: \left(\left| \sigma^2-|a_{ii}|^2 \right|-|a_{ii}|c_i(A) \right)\left| \sigma^2-|a_{jj}|^2 \right|\leq \sigma r_i (A)\left(\sigma c_j (A)+|a_{jj}|r_j(A)\right)} \right\},$

$\Delta_2(A)=\bigcup\limits_{i = 1, j=1}^n \left\{ {\sigma \ge 0: \left| \sigma^2-|a_{ii}|^2 \right| \left(\left| \sigma^2-|a_{jj}|^2 \right|-|a_{jj}|r_j(A) \right)\leq \sigma c_j (A)\left(\sigma r_i (A)+|a_{ii}|c_i(A)\right)} \right\}.$

Proof. Let $\sigma$ be an arbitrary singular value of $A$. Then there exist two nonzero vectors $x = (x_1, x_2, \ldots, x_n)^t$ and $y = (y_1, y_2, \ldots, y_n)^t$ such that

$\sigma x = A^*y \ \ \mathrm{and} \ \ \sigma y = Ax.$

(2.6)

Denote

$|x_p| = \max\{|x_i|, \ \ 1\leq i \leq n \}, \ \ |y_q| = \max\{|y_i|, 1\leq i \leq n\}.$

Similar to the proof of Theorem 2, the $q$-th equations in (2.6) imply

$\left| \sigma^2-|a_{qq}|^2 \right||y_q| \leq |a_{qq}|\sum\limits_{j = 1, j \ne q}^n {|a_{jq}| } |y_j |+\sigma \sum\limits_{j = 1, j \ne q}^n {|a_{qj}| } |x_j| \notag \\ \;\;\;\;\;\;\;\;\leq |a_{qq}|\sum\limits_{j = 1, j \ne q}^n {|a_{jq}| } |y_q|+\sigma \sum\limits_{j = 1, j \ne q}^n {|a_{qj}| } |x_p|.$

(2.7)

That is,

$\left(\left| \sigma^2-|a_{qq}|^2 \right|-|a_{qq}|\sum\limits_{j = 1, j \ne q}^n {|a_{jq}| } \right)|y_q|\leq \sigma \sum\limits_{j = 1, j \ne q}^n {|a_{qj}| } |x_p|.$

(2.8)

If $|x_p|\leq |y_q|$, the $p$-th equations in (2.6) imply

$0\leq \left| \sigma^2-|a_{pp}|^2 \right||x_p|\leq \left(\sigma \sum\limits_{j = 1, j \ne p}^n {|a_{jp}| } +|a_{pp}|\sum\limits_{j = 1, j \ne p}^n {|q_{pj}| }\right)|y_q|.$

(2.9)

Multiplying inequalities (2.8) with (2.9), we have

$\left(\left| \sigma^2-|a_{qq}|^2 \right|-|a_{qq}|c_q(A) \right)\left| \sigma^2-|a_{pp}|^2 \right|\leq \sigma r_q (A)\left(\sigma c_p (A)+|a_{pp}|r_p(A)\right).$

Similarly, if $|x_p|\geq |y_q|$, we can get

$\left| \sigma^2-|a_{qq}|^2 \right| \left(\left| \sigma^2-|a_{pp}|^2 \right|-|a_{pp}|r_p(A) \right)\leq \sigma c_p (A)\left(\sigma r_q (A)+|a_{qq}|c_q(A)\right).$

Thus, we complete the proof.

We now establish comparison results between $\Delta (A)$ and $\Gamma (A)$.

Theorem 4 If a matrix $A=(a_{ij}) \in \mathbb{C}^{n\times n}$, then

$\sigma (A) \in \Delta (A)\subseteq \Gamma (A).$

Proof. Let $z$ be any point of $\Delta_1 (A)$. Then there are $i, j \in N$ such that $z\in \Delta_1(A)$, i.e.,

$\left(\left| z^2-|a_{ii}|^2 \right|-|a_{ii}|c_i(A) \right)\left| z^2-|a_{jj}|^2 \right|\leq z r_i (A)\left(z c_j (A)+|a_{jj}|r_j(A)\right).$

(2.10)

If $z r_i (A)\left (z c_j (A)+|a_{jj}|r_j (A)\right)=0$, then

$\left| {z ^2 - \left| {a_{ii} } \right|^2 }\right| - \left| {a_{ii} } \right|c_i (A)=0,$

or

$\left| z^2-|a_{jj}|^2 \right|=0.$

Therefore, $z \in \Gamma_1 (A)\bigcup \Gamma_2 (A)$. Moreover, If $z r_i (A)\left (z c_j (A)+|a_{jj}|r_j (A)\right)> 0$, then from inequality (2.10), we have

$\frac{{\left| {z^2 - \left| {a_{ii}^2 } \right|} \right| - \left| {a_{ii} } \right|c_i (A)}}{{zr_i (A)}}\frac{{\left| {z^2 - \left| {a_{jj}^2 } \right|} \right|}}{{z c_j (A)+|a_{jj}|r_j(A)}} \le 1.$

(2.11)

Hence, from inequality (2.11), we have that

$\frac{{\left| {z^2 - \left| {a_{ii}^2 } \right|} \right| - \left| {a_{ii} } \right|c_i (A)}}{{zr_i (A)}} \le 1,$

or

$\frac{{\left| {z^2 - \left| {a_{jj}^2 } \right|} \right|}}{{z c_j (A)+|a_{jj}|r_j(A)}} \le 1.$

That is, $z \in \Gamma_1 (A)$ or $z \in \Gamma_2 (A)$, i.e., $z \in \Gamma (A)$. Similarly, if $z$ be any point of $\Delta_2 (A)$, we can get $z \in \Gamma (A)$.

Thus, we complete the proof.

Example 1. Let

$\left[{\begin{array}{*{20}c} 1 & 4 \\ {0.1} & {0.5} \\ \end{array}} \right].$

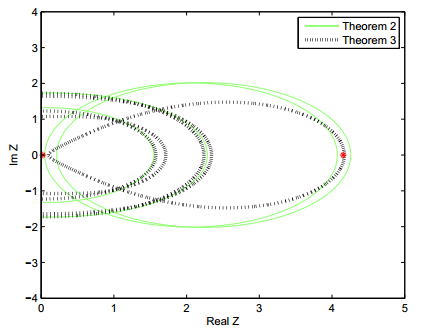
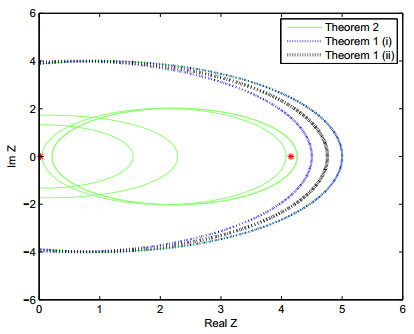
The singular values of $A$ are $\sigma_1 = 4.1544$ and $\sigma_2 = 0.0241$. The singular value inclusion sets $C (A)$, $D (A)$, $\Gamma (A)$ and the exact singular values are drawn in Figure 1. From Figure 1, we can say, all the singular values are contained in the singular value inclusion sets $C (A)$, $D (A)$, $\Gamma (A)$, but the inclusion sets $\Gamma (A)$ are more tighter than the inclusion sets $C (A)$, $D (A)$. That is to say, the results in Theorem 2 are better than the results in Theorem 1 for certain examples.

The singular value inclusion sets $\Gamma (A)$, $\Delta (A)$ and the exact singular values are drawn in Figure 2. From Figure 2, we can say, all the singular values are contained in the singular value inclusion sets $\Gamma (A)$, $\Delta (A)$, but the inclusion sets $\Delta (A)$ are more tighter than the inclusion sets $\Gamma (A)$. That is to say, the results in Theorem 3 are always better than the results in Theorem 2, which are shown in Theorem 4.

---

3. Conclusion

In this paper, some new inclusion sets for singular values are given, theoretical analysis and numerical example show that these estimates are more efficient than recent corresponding results in some cases.

---

Acknowledgements

He is supported by Science and technology Foundation of Guizhou province (Qian ke he Ji Chu [2016]1161); Guizhou province natural science foundation in China (Qian Jiao He KY [2016]255); The doctoral scientific research foundation of Zunyi Normal College (BS[2015]09). Liu is supported by National Natural Science Foundations of China (71461027); Science and technology talent training object of Guizhou province outstanding youth (Qian ke he ren zi [2015]06); Guizhou province natural science foundation in China (Qian Jiao He KY [2014]295); 2013, 2014 and 2015 Zunyi 15851 talents elite project funding; Zhunyi innovative talent team (Zunyi KH (2015)38). Tian is supported by Guizhou province natural science foundation in China (Qian Jiao He KY [2015]451);Scienceand technology Foundation of Guizhou province (Qian ke he J zi [2015]2147). Ren is supported by Science and technology Foundationof Guizhou province (Qian ke he LH zi [2015]7006).

---

Conflict of Interest

All authors declare no conflicts of interest in this paper.

---