Frustration in mathematical problem-solving: A systematic review of research

1.   Introduction

Emotions are present in learning and problem solving [1, 2] and can influence decision-making [3]. Additionally, there is evidence that individuals experience patterned changes of affect during problem-solving [e.g. 4]. Goldin [5] introduces the idea of affective pathways - the changes in local affect in mathematics problem-solving. With this in mind, he expresses interest in "ways that affect is or can be utilized by mathematical problem solvers to guide their steps" (p. 212). Generally, studies have aimed to inform how to cope with or reduce students' experience of negative emotions and promote positive emotions, but little research has sought to address capitalising on emotions at a step-by-step level to stimulate useful affective or cognitive responses in the course of problem solving.

It is notoriously difficult to research student emotions in real-time and many methods for doing so are underused in educational research [6]. In, for example, self-report or follow-up interviews, students can misremember their emotions and assessing emotions during tasks qualitatively or quantitatively can impact student affect [7]. For researchers, it is vital to understand how different methodological approaches influence the boundaries of our possible findings.

One exception to studying emotions at a step-by-step level is research into surprise, which is a powerful and often obvious emotion making it accessible for such an investigation. Studies have explored how it can be utilised during problem solving to generate certain reactions in students. For example, Koichu et al. [8] argue that surprise can be used to stimulate an aesthetic appreciation of a solution to a problem in middle school students. Marmur and Koichu [9] present findings from undergraduate calculus that suggest that experiencing surprise at a solution can promote an aesthetic response in students, which in turn can positively influence student's mathematics-related affect and their retention of the material.

Can emotions traditionally conceived as negative similarly be used or transformed to support problem solving? Perhaps there are opportunities to move beyond simply coping and instead toward rethinking how we treat negative emotions. They have possibly fallen victim to the convenient label "negative, " resulting in an avoid-at-all-costs approach, but negative emotions may not have strictly negative consequences or be compromisingly unpleasant to experience. For example, reasonable levels of anxiety may increase motivation to succeed [6]. Of particular interest is frustration, which is a prominent emotion during problem-solving across all levels of learners [4, 10-11].

Goldin [5] argues that frustration arises from a lack of progress. Frustration can be considered as resulting from being unsuccessful at resolving an impasse [4, 12]. Emotive language such as, "dissatisfaction, " "annoyance, " and "irritated", is often used in definitions for frustration [4, 13]. Additionally, studies have shown that students' perceived control over and value of a mathematical task can negatively predict frustration [12, 14]. Generally, the literature considers frustration as the resulting discontent when efforts do not yield progress or seem pointless.

Sinclair [15] argues that the strategies mathematicians themselves use reflect an acceptance that problem-solving may be frustrating. This suggests that interventions simply aiming to reduce frustration are not necessarily the best solution to tackling the consequential issues [10], though it is not clear whether frustration can be more harmful for certain levels of learners. Regulating the intensity of and normalising frustration is critical so that students do not, for example, panic upon becoming frustrated. However, given the perspective of mathematicians - does frustration also have the potential to play a positive role in problem-solving? This review is structured around the following aims:

1. To clarify the correlates and consequences of frustration in the context of problem solving in order to better understand its role.

2. To investigate the variation in how frustration manifests in problem-solving at different levels of mathematics.

3. To provide an overview of the research methods in publications on frustration in problem-solving and whether these impact the conclusions.

2.   Method

2.1.   Collection

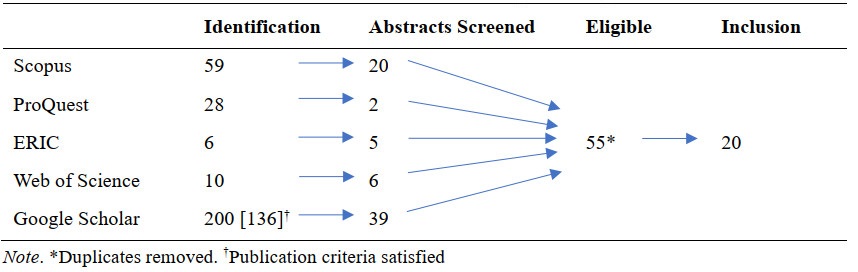
The databases Scopus, ProQuest, ERIC, Web of Science, and Google Scholar were searched in March 2021. As frustration is often researched alongside other affective constructs, I conducted the database search so that the abstract explicitly mentioned frustration, affect, or emotions (affect* OR emotion* OR frustration). Further, since I was specifically interested in frustration during mathematical problem solving, I required an indication the study was about mathematics (math*) to be in the abstract. Finally, I required "problem solving" and "frustration" to appear in the full text. For example, the Scopus database search appeared as, (ABS (affect* OR emotion* OR frustration) AND ABS (math*) AND ALL ("problem solving") AND ALL (frustration)).

Where possible, filters for only fully published, peer-reviewed journal articles and conference proceedings written in English were turned on, otherwise results that did not meet this criterion were manually excluded. The subject area on the Scopus database was limited to maths, social science, and psychology. For the ProQuest database, the results were filtered to include the following databases: Education database, Psychology database, Science database, Social Sciences database, Research Library: Science & Tech, and Research Library: Social Sciences. Only the Core Collection of the Web of Science database was searched. Finally, since searching in the abstract is not available in Google Scholar, I only required "frustration, " "problem solving, " and "math*" to appear in the full text. The first 200 results were manually screened to satisfy the publication criteria, resulting in 136 articles and conference proceedings.

2.2.   Inclusion and exclusion criteria

The PRISMA protocol (Table 1) was used to conduct the screening process, which established whether the article discussed correlates or consequences of frustration in the context of problem solving. In google scholar, abstracts were screened to satisfy the same search criteria as in the other databases. Results were excluded which did not report on frustration specifically in relation to mathematical problem-solving (e.g. teacher frustration; student frustration in an online environment; comparing methods to investigate frustration). Articles that either identified frustration as a component of problem solving or presented methods to mitigate it without investigating its relationship to problem solving were also excluded. In cases where it was unclear whether the article met these criteria from the abstract, it was included for full-text eligibility assessment.

3.   Results

After screening, 20 journal articles and conference proceedings met the inclusion criteria. These are presented in Table 2 with the respective research method, participants, and valency of the discussion on frustration in problem-solving. Valency was determined by 1) whether the paper discussed the findings as informative of mitigating frustration 2) reporting frustration as correlating or causing negative/positive cognitive processes or 3) reporting frustration as correlating or causing affect traditionally understood as negative/positive.

In this review, I found 8 papers that presented frustration as having a negative role in problem solving, 3 that considered the positive role of frustration in problem solving, 7 papers that discussed the potential for frustration to play a positive or negative role, and 2 that were unidentified.

3.1.   The cognitive relationships of frustration

Frustration in mathematical problem solving has been shown to correlate positively with perceived difficulty [16], perceived mathematical incompetence [17], and time taken to solve the problem [18]. It correlates negatively with task control [11, 14], task value [12, 14], mathematical self-concept, and task achievement [17]. Frustration negatively predicts planning, cognitive learning strategies [11], enactment of learning strategies, and metacognitive self-regulation [14]. It positively predicts the use of shallow cognitive strategies [12]. In contrast, Goldin et al. [19] argues that frustration "can signify serious engagement with impasse" (p. 551), so it can be experienced as negative in anticipating failure or positive in heightening interest.

3.2.   The consequences of frustration

Given the largely negative relationships outlined above, it is no surprise that many researchers draw conclusions about the negative consequences of frustration. Frustration is commonly recognised as an antecedent of giving up [e.g. 20, 21]. Weber et al., [22] report that frustration resulted in the student relying on rote-learning strategies or disengaging, in turn contributing to greater future frustration during problem-solving. However, Goldin [23] argues that frustration may evoke "new heuristic processes" (p. 154), which is elaborated in Goldin [5] where he claims, "frustration need not be a negative problem-solving outcome. It can evoke numerous useful heuristic processes: starting over; trying a radically new approach…; trying a simpler problem…; making a new set of assumptions…; and so on" (p. 215). In this vein, Di Leo et al. [11] report, "frustration resulted in students asking for help, monitoring or controlling their progress or understanding, and making plans to continue to solve the problem" (p. 135).

Bjuland [24] relays a similar finding but frames it as coping with frustration rather than frustration cuing the action, "In order to deal with frustration and to stimulate their motivation in the problem-solving process, the students suggest starting to work on an easier problem" (p. 217). Additionally, Voica et al. [25] identify an interaction between frustration and self-efficacy through sharing an excerpt from a student outlining how, although they feel frustrated, try harder with each failure. The authors' interpretation is that the motivation is despite frustration rather than a consequence. Goldin [20] theorises precisely on this scenario - that frustration might motivate a desire to persevere or be more systematic in problem-solving attempts. This is supported by DeBellis and Goldin [26], who share of a student, "frustration at her difficulty in explaining her conclusion appears to have served her well, motivating her to articulate an explanation" (p. 34).

3.3.   The affective relationships of frustration

During problem solving, frustration has been shown to correlate positively with confusion [11-14, 18], boredom [11, 12, 17], anxiety [12, 13], surprise, and hopelessness [13]. Frustration has been shown to correlate negatively with confidence [16], curiosity [12], and joy [12, 17], specifically at the conclusion of a problem [17]. Carlson and Bloom [27] comment that an intimacy with the problems coincided with "strong affective responses" (p.11), including in frustration. Thus, a certain level of frustration may indicate a deep investment in the problem.

3.4.   Frustration in affective pathways

Frustration has been argued to be part of positive or negative affective pathways, depending on the individual or the scenario [e.g. 5, 10]. Commonly, confusion transitions to frustration [13, 14], with the two states often forming a cycle [11, 13] or the pattern of confusion-frustration-disengagement [13]. Di Leo et al. [11] showed that frustration can also follow surprise, anxiety, and hopelessness, and can be followed by anger and hopelessness. In contrast, some authors identify positive patterns of emotions involving frustration. Presmeg and Balderas-Cañas [28] affirm the possibility for different affective pathways as presented in Goldin [5], showing frustration in pathways with both positive and negative problem-solving outcomes. O'Dell [29] claim that frustration was often followed by a moment of joy during problem solving and argues the oscillation between excitement/joy and frustration is a sign of "productive struggle".

3.5.   Participants

This review aimed to understand whether frustration manifested differently for students at different levels of mathematics. Table 3 outlines the 15 non-theoretical studies done on different levels of mathematical learners and whether the discussion on the role of frustration in problem-solving is positive or negative. It is not reasonable to infer any meaningful conclusion about whether frustration is positive or negative at each level due to the small sample of studies. However, it is noteworthy that frustration has been discussed as having the possibility of being positive for primary, secondary, and tertiary students, as well as student-teachers. Additionally, Table 3 illuminates that research into frustration in problem-solving has been largely investigated at a primary level.

3.6.   Methods

The final research aim for this review was to determine whether the research method of a study impacted what was able to be concluded. Table 4 summarises the method of each paper by the determined valency of the discussion on frustration in problem-solving. Again, the small sample size makes it unreasonable to make informed conclusions about this question. What does become obvious from Table 4, however, is the large number of theoretical studies suggesting that frustration can play both a positive and negative role. It appears that quantitative and mixed-methods studies did not as frequently support this possibility than qualitative studies.

Some studies opted to collect data after the participant had engaged in their problem-solving [16, 17, 25]. As mentioned previously, this type of data collection can be problematic regarding students' recollection and remove the influence frustration has on any given moment of the problem-solving process. The remaining 12 non-theoretical studies all attempted to measure frustration during problem solving, using such methods as thinking-aloud or completing a questionnaire at fixed intervals during the process. Of these, 5 reported frustration as only negative.

3.7.   Methodological concerns

As outlined previously, the small number of studies that qualified to be in this review limited the conclusions that can be drawn about participants and research methods. This, however, highlights the need for more research in this area.

As seen in Table 2, of the ten papers that consider the positive role of frustration in problem solving, five are exclusively theoretical and largely by the same author, which skews the findings. However, the arguments that have been made in these papers have almost all not been followed up with empirical investigations. Instead, there is a general acceptance by the community that frustration is negative in mathematical problem solving, demonstrated by the large number of studies focused specifically on mitigating it. For example, Goldin [20] claims that frustration may result in more systematic problem-solving behaviour in discrete mathematics. Positive relationships will not be found if they are not researched. This finding of the systematic review informs the need for researchers to address these questions.

4.   Discussion

Research on affect in mathematics education has been recently accelerating, but the literature regarding frustration during mathematical problem-solving is still not abundant, as evidenced by the small number of papers included in this review. At the beginning of this paper, I attempted to negotiate a definition, but the boundaries of what constitutes and characterises frustration have not been consolidated across the field. While this analysis employs a systematic review approach, it addresses frustration in mathematical problem-solving where this emotion has been identified and labelled as such, and does not claim to systematically address all research into what may, by others, be understood as frustration. The conclusions drawn about the nature of frustration therefore need to be mediated by this knowledge.

It may be that frustration playing a positive role during mathematical problem solving has been observed in other studies under a different name. For example, there have been arguments for the benefits of productive failure or productive struggle [e.g. 30]. The idea that struggle is significant in building new understanding and the value of engaging just outside a learner's comfort zone can be seen in the work of Piaget [31] and Vgotsky [32]. What remains unclear is if frustration is indeed a common or even necessary part of productive struggle. Should productive struggle be accompanied by confusion, frustration, or absent of an emotional component altogether?

This review has not provided conclusive evidence that frustration is experienced differently during problem-solving by different levels of learners. It has instead demonstrated demand for a greater body of research on this issue given its unavoidable presence, particularly for students outside of primary school. The review was also not able to determine whether research methods associate with the types of conclusions drawn. I suggest that qualitative studies in education are not as limited by the variables chosen for investigation and can attend to what is happening in the moment, in turn allowing for more flexibility in identifying the outcomes of frustration during problem-solving.

However, in analysing the papers of the present review, I believe there remains a negative bias on how the experience of frustration is interpreted. Further, the number of participants that can be investigated and the generalisability of the conclusions of such studies remains limited. Thus, future studies that use self-report measures should be informed by this review of the possibility for frustration to play a positive role and incorporate this aspect into their research questions. Additionally, the call remains to incorporate other quantitative approaches (e.g. EEG methods, cortisol testing) for investigating student emotions in real-time [6]. The review has also illuminated the high ratio of theoretical papers to empirical studies. For mathematics education to progress as a field of research, I argue this theorising should be considered and validated with multiple research approaches.

Frustration is often negatively associated with cognitive and affective processes beneficial to problem-solving. Yet, it is an inevitable part of engaging with mathematics and these findings imply that frustration may not be one-dimensionally negative; that there may be opportunities to alter how frustration is experienced by students and how it in turn impacts their local affect and cognitive processes. Goldin [20] comments, "It should not be our goal to remove frustration, but to develop the ability in students to channel it into constructive strategic choices〞and positive meta-affect [thoughts, beliefs, and feelings around affect]" (p. 60). There is a distinction between simply coping with or reducing frustration, to transforming the experience of and, in turn, outcomes of frustration. Though much remains unknown, this synthesis has uncovered some avenues for rethinking frustration for mathematics students.

5.   Practical recommendations

5.1.   Rethinking frustration in practice indirectly through lesson design

For the benefits of frustration to be realised by students, they must first realise it is a possibility for frustration to lead to another outcome besides, for example, boredom or anxiety. In designing lessons aimed at rethinking frustration, the goal would be to stimulate other emotional pathways in students following frustration - exposing them to a different pattern of emotions they may experience when engaging with mathematical problem-solving. Students, for example, could experience frustration in their problem-solving leading to resolution and enlightenment. As a one-off, this provides them with the valuable knowledge that being frustrated does not always result in becoming anxious or bored. Building on this, Pekrun [33] argues that we may assume that the repetition of emotions can cause cognitive appraisals to be bypassed, leading the emotion to become habitualised in recurring scenarios. Therefore, repeated experiences of this nature could theoretically shift students' default patterns of local affect, as well as indirectly altering their meta-affect (that is, what they think and feel about experiencing frustration).

The goal in designing such a lesson is to try and elicit a different pattern of emotions in students. A potential way to introduce this at a tertiary level would be to present an unsolvable problem, which can be disproved. Attempting to find a solution is likely to induce frustration in most students. Rather than resignation, frustration can cue the prospect of discovery - even if it is not what the student initially sets out to do in the problem. Important features of a lesson design such as this would be:

●  The selected problem should be one where efforts to solve provide some evidence that the problem is unsolvable (e.g, the Water Gas Electricity problem through sketching).

●  After some time, many students should begin questioning the possibility of solving the problem. This step may need to be facilitated but it is important that there is minimal prompting and that students are allowed authority over the problem to reduce the risk that students remain frustrated through not understanding how certain logical steps were made. The appropriate amount of time may vary between assisting students to avoid frustration leading to disengagement with the mathematics from boredom.

●  Students should possess the appropriate prerequisite knowledge required to disprove the problem.

Through engaging in this problem-solving process, students are also provided the opportunity to utilise frustration as a motivator for considering a problem differently, asking a simpler question (i.e. Instead of "How do I do this?" asking, "Is this possible?"), or pursuing a different course of action. Their struggle to formulate a solution elicits the question of why, promoting the idea that frustration is associated with curiosity and flexible thinking about a problem. Through engaging in this problem-solving process, students are given the opportunity to experience frustration as a normal part of engaging with mathematical discovery. Finally, following the experience of frustration is resolution and enlightenment, which in many cases should affectively manifest as satisfaction in students.

It would be useful to investigate whether such an intervention is effective as theorised in stimulating different affect and cognition in students experiencing frustration. Would an exercise soliciting frustration over the course of multiple lessons rather than a single lesson be more impactful or lead to greater anxiety and disengagement? How frequently should these problem-solving exercises occur to disrupt default patterns of emotions? Given the variation between how students experience frustration resulting from, for example, self-regulation, how can we design lessons to unlock these potential benefits for all students and avoid further disengagement from certain groups? What would a problem-solving exercise aimed at inducing and resolving frustration look like at a primary or secondary level?

5.2.   Rethinking frustration in practice by directly addressing student mindset

The suggestion that negative emotions can have positive consequences is not new - for example, anxiety and shame in certain scenarios may have a positive relationship with motivation [6]. However, perhaps the consequences of an emotion are mediated by students' beliefs about that emotion. A recent innovation argued the effects of stress depends on an individual's mindset about stress. Crum, et al. [34] challenge the view that stress is inherently negative and propose the construct of stress mindset, defined to be the extent to which an individual believes stress has enhancing or debilitating consequences. Further, Crum et al., [34, 35] demonstrated stress mindset can be changed through using short video interventions. Perhaps the related emotion of frustration behaves similarly, in that the consequences result from whether an individual believes it to be negative (for their health, performance, learning, etc.) or positive. In fact, it could be that the results of many emotions, particularly those that research has identified to have mixed consequences are a product of students' mindset about those emotions. Emotion-mindset may be considered a cognitive subset of DeBellis and Goldin's [10] notion of meta-affect and provides an opportunity to directly address the issues around how negative emotions influence students' problem-solving decision-making. This is an avenue for future research to explore with the view to adapt student beliefs about frustration through direct interventions.

6.   Conclusion

The systematic review provided a synthesis of the current literature on the role of frustration during mathematical problem-solving. The literature has suggested that frustration associates with different affective and cognitive features of problem-solving, which are not all negative. However, empirical studies tend to concentrate on the known negative correlates and consequences of frustration, limiting the exploration of its possible benefits as demonstrated in several studies. Additionally, the literature contains numerous theoretical publications that have yet to be followed up with empirical investigations. This has illuminated the need for more research of varying methodologies and across all levels of mathematics learning. Informed by this synthesis, I have identified directions that can be taken to investigate and unlock the possible benefits to students experiencing frustration, such as stimulating different affective pathways and addressing their mindset around experiencing this emotion. It would be valuable to research whether these proposed methods are viable approaches to rethinking students' experience of frustration during mathematical problem-solving.