1.
Introduction
In this paper, we consider boundary tracing problem of nonlinear fractional diffusion equations with Neumann boundary condition
by iterative learning algorithms, where Dαt is the Caputo fractional derivative of order α, 0<α<1, (x,t)∈ΩT≜[0,1]×[0,T] and F(x,t,φ,φx) is the nonlinear function.
The basic idea of iterative learning control (ILC) [1,4,16] can be traced back to Garden [8] in 1967 and Uchiyama [28] in 1978. ILC is a control method suitable for dealing with iterative systems, which uses information obtained from previous trial to improve the tracking performance of current trial. Owing to simplicity and effectiveness, ILC plays an important role in many fields and applications [9,10,14].
ILC schemes are widely used for ordinary differential equations (ODEs) [23,25,26,29]. However, there are few studies on its application to partial differential equations (PDEs) and fractional partial differential equations (FPDEs) [11,24]. Choi et al. [3] employed the characteristic line method and the Q-ILC method to study the ILC schemes of a first-order hyperbolic PDE system. Huang et al. [12] studied the P-type ILC scheme for boundary tracking of nonlinear hyperbolic parametric systems and evaluated the robustness of the scheme. Kang et al. [15] proposed a Newton-type ILC algorithm for nonlinear parametric equations and provided sufficient conditions for convergence of the Newton descent method using the λ-norm. Different from the convergence in the sense of the λ norm, Dai et al. [5] derived the P-type ILC for linear parabolic parametric equations and proved its convergence in the sense of the L2-norm and the W1,2-norm. Lan et al. [22] presented a second-order ILC method for a class of multi-agent systems (MAS) with time-delay distributed parameters and proved its convergence.
For the diffusion equation, Xu et al. [30] proposed P-type and D-type ILC methods for infinite-dimensional linear systems in Hilbert spaces. Huang et al. [13] extended ILC to solve the boundary tracking problem of inhomogeneous heat equations and provided evidence for the effectiveness of the D-type ILC scheme. Zhang et al. [32] presented a novel intermittent updating PD-type ILC algorithm for semi-linear distributed parameter systems with sensors or actuators, and provided convergence conditions for the output error. For the fractional diffusion equation, Lan et al. [20] discussed the P-type ILC of fractional order parameter exchange systems and demonstrated that the exchange system maintains traceability over two time periods. Lan et al. [21] proposed a second-order P-type ILC scheme for a class of linear fractional order distributed parameter systems and established a sufficient condition for convergence using λ-norm and generalized Gronwall inequality.
Overall, there have been relatively few studies on iterative learning control algorithms for fractional diffusion equations, which can describe a variety of memory materials and genetic processes [6,18]. Applying the ILC algorithm to fractional diffusion equations can improve control of the system for nonlocal transport phenomena and long-range memory effects, leading to faster convergence and improved tracking accuracy [19]. We aim to extend ILC to the nonlinear fractional diffusion equation and study their convergence. However, this work is challenging, as the difficulty lies in proving the convergence of the iterative learning control algorithm for fractional diffusion equations, with added challenges posed by the fractional derivatives and nonlinear source terms. Therefore, we assume that source term is Lipschitz continuous and employ Sobolev imbedding theorem to overcome difficulties in the proof.
In this paper, we consider boundary tracing problem of one dimensional fractional diffusion equation with input, state and output functions at the k-th iteration,
where k denotes the iterative number of the process and uk,φk,yk(t) are the input, state and output of the system at the k-th iteration respectively. The main idea is to adjust the control input uk(t) iteratively in order that system output {yk(t)} can track the predefined target yd(t) when k→∞.
In addition, we make some assumptions about the functions in system (1.2). Suppose c(t) and d(t) are bounded and F(x,t,φk,φkx) satisfies Lipschitz condition.
Assumption 1: The functions c(t) and d(t) satisfy
where c1,d1,d2 are positive constants.
Assumption 2: The nonlinear function Fk≜F(x,t,φk,φkx) is Lipschitz continuous,
where CF is a constant.
This paper is organized as follows. Preliminaries are presented in Section 2. In Section 3, P-type ILC scheme, PIθ-type ILC scheme and corresponding convergence conditions are proposed for the nonlinear system. Numerical examples are given in Section 4 to illustrate the effectiveness of the methods. Finally, conclusions are drawn in Section 5.
2.
Preliminaries
To prepare for our subsequent analysis, it is essential to introduce some definitions, useful lemmas and theorems.
Definition 2.1. [17] Let z(t)∈AC[0,T], the Caputo fractional derivative of order α is defined by
Definition 2.2. [17] Let z(t)∈L(0,T), the Riemann-Liouville fractional integral of order α is defined by
Definition 2.3. [27] The two-parameter Mittag-Leffler function is defined by the series expansion
Lemma 2.1. [27] Suppose 0<α<1. Caputo fractional derivative and fractional integral of order α have the following relationship
Lemma 2.2. [7] Assume x(t) be a differentiable function. The following relationship holds
Lemma 2.3. (Gronwall inequality [31]) Suppose a(t) is a nonnegative, nondecreasing, locally integrable function over 0≤t0≤t≤T and g(t) is a nonnegative, nondecreasing continuous function over 0≤t0≤t≤T, g(t)≤M, where M is a postive constant. If u(t) is nonnegative and locally integrable function over 0≤t0≤t≤T and satisfies
then, we have
Theorem 2.1. (Sobolev imbedding theorem [2]) Let Ω∈Rd be a bounded Lipschitz domain and 1≤p≤∞. If 0≤m<k−dp<m+1, the space Wk,p(Ω) is continuously imbedded in Cm,α(¯Ω) for α=k−dp−m and compactly imbedded in Cm,β(¯Ω) for all 0≤β<α.
Remark 2.1. Using the Sobolbev imbedding theorem 2.1 in the case of d = 1, we can get
where ||φ(x,t)||2H1≜∫Ωφ2+φ2xdx and C1 is a positive constant.
3.
ILC design for nonlinear systems
We need to give some necessary lemmas to obtain the convergence conditions for the ILC scheme.
Lemma 3.1. Suppose e(t)∈AC[0,T) and 0.5<θ≤1, then, we have
Proof. From the Definition 2.2 of fractional integral, we can get
where |e|2λ≜supt∈[0,T]{e−λt|e(t)|2,λ>0} and |e(t)| represents absolute value of e(t). Let t−τ=ω and λω=v, we have
From the definition of the Gamma function, we can get
This completes the proof.
Lemma 3.2. If ψ satisfies the equation
we have
where
|e(t)| represents absolute value of e(t), M=maxt∈[0,T]|Dαtψ(0,t)|2, c1=αααΓ(α)eα, δF=F(x,t,φk+1,φk+1x)−F(x,t,φk,φkx) and ψ=φk+1−φk.
Proof. (i) We firstly prove the formula (3.5). Multiplying both sides of the equation Dαtψ=ψxx+δF by ψ and integrating with respect to x, it yields
Based on Lemma 2.2, formula (1.3) and boundary condition, it is not hard to know
Using Young inequality (weighted form) and taking the positive constant C1 in formula (2.1), it leads to
where c2=C2F+2CF. It follows from Theorem 2.1 that
Integrating both sides of the inequality with respect to t, by Lemma 2.1 we have
Using initial condition, we can get
Applying Lemma 2.3, we can obtain
Taking λ-norm on both sides of inequality, we can derive
(ii) We then prove the formula (3.6). Multiplying both sides of the equation Dαtψ=ψxx+δF by ψxx and integrating with respect to x, it yields
By boundary condition, we get
Based on Lemma 2.2, it is not hard to know
We can conclude from the formula (1.3) that
Using Young inequality (weighted form), it leads to
where M=maxt∈[0,T]|Dαtψ(0,t)|2. Integrating both sides of the inequality about t and using initial condition, according to Lemma 2.1 we get
Applying Lemma 2.3, we obtian
Taking λ-norm on both sides of inequality, we can derive
Since tαe−λt gets the maximum value ααλαeα at t=αλ. Therefore, we can get
where c1=αααΓ(α)eα. Then, taking the maximum value on the left side of the inequality, we have
This completes the proof.
Lemma 3.3. If ψ satisfies the equation
we have
where
|e(t)| represents absolute value of e(t), M=maxt∈[0,T]|Dαtψ(0,t)|2, c1=αααΓ(α)eα, δF=F(x,t,φk+1,φk+1x)−F(x,t,φk,φkx), ψ=φk+1−φk and c3=2Γ(2θ−1)γ2TΓ(θ)2.
Proof. (i) We firstly prove the formula (3.12). Multiplying both sides of the equation Dαtψ=ψxx+δF by ψ and integrating with respect to x, it yields
Based on Lemma 2.2 and boundary condition, it is not hard to know
Using Young inequality (weighted form) and taking the positive constant C1 in formula (2.1), we obtain
Applying Theorem2.1 and Lemma 3.1, it leads to
where c3=2Γ(2θ−1)γ2TΓ(θ)2. Integrating both sides of the inequality about t and using initial condition, by Lemma 2.1 we get
It follows from Lemma 2.3 that
Taking λ-norm on both sides of inequality, we can derive
(ii) We then prove the formula (3.12). Multiplying both sides of the equation Dαtψ=ψxx+δF by ψxx and integrating with respect to x, it yields
Based on boundary condition, it is not hard to know
According to Lemma 2.2, we obtain
Applying Lipschitz condition (1.3), we have
Using Young inequality (weighted form), it leads to
Integrating both sides of the inequality with respect to t and using initial condition, by Lemma 2.1 and Lemma 3.1, we get
where c3=2Γ(2θ−1)γ2TΓ(θ)2. Using Lemma 2.3, we have
Taking λ-norm on both sides of inequality, similar to Lemma (3.2), we obtain
where c1=αααΓ(α)eα. This completes the proof.
3.1. Open-loop P-type ILC
The open-loop P-type ILC scheme for Eq (1.2) is
where ek(t)=yd(t)−yk(t) denotes the output error and the learning gain β is an unknown parameter to be determined later.
Theorem 3.1. For system (1.2) and the open-loop P-type law (3.13), if there exist a learning gain β and a constant l(l>0) satisfying
where ¯ρ1=maxt∈[0,T]|1−βd(t)|, then the output error ek can converge to the ϵ-neighborhood of zero for any constant ϵ>0 in the sense of λ-norm as k→∞.
Proof. From the definition of error, we get
Based on the formula (3.13), it is not hard to know
Squaring both sides of the equation, we get
where ¯ρ1=maxt∈[0,T]|1−βd(t)| and ¯c=maxt∈[0,T]|c(t)|. Using Young inequality (weighted form) to ensure (1+l)¯ρ21≤1 and Theorem 2.1, we have
Taking λ-norm on both sides of inequality, we get
Using Lemma 3.2, we obtain
where
and Mk=maxt∈[0,T]|Dαtφk(0,t)|2. Choosing λ large enough so that q1<1, we get
where ¯μ1,k≜maxm∈{0,1,⋯,k}μ1,m. We select λ large enough so that ¯μ1,k is sufficiently small. Therefore, to ensure |ek+1(t)|2λ≤ϵ2, it is sufficient to make
which means that the output error converges to the ϵ-neighborhood of zero after finite step iteration (k>2(lnϵ−ln|e0|λ)lnq1−1).
Remark 3.1. Due to q1(λ) is a monotonic decreasing function of λ and (1+l)¯ρ21<1, we can see that the inequality q1<1 holds when λ is large enough. From the definition of μ1,k, μ1,k is proportional to λ−α. The number of iterations k is finited, so ¯μ1,k is also proportional to λ−α and ¯μ1,k tends to zero when λ is large enough.
Remark 3.2. In order to satisfy the convergence condition (3.14), the learning gain β should satisfy
To ensure that the above inequality holds, parameter l should satisfy
3.2. Closed-loop P-type ILC
The closed-loop P-type ILC control scheme for (1.2) is
where ek+1(t)=yd(t)−yk+1(t) is the output error and the learning gain β is an unknown parameter to be determined later.
Theorem 3.2. For system (1.2) and the ILC law (3.21), if there exist a learning gain β and a constant l(l>0) satisfying
where ¯ρ2=maxt∈[0,T]1|1+βd(t)|, then the output error ek can converge to the ϵ-neighborhood of zero for any constant ϵ>0 in the sense of λ-norm as k→∞.
Proof. From the definition of error, we get
Based on the formula (3.21), it is not hard to know
Simplifying the above equation, we have
Squaring both sides of the equation, we get
where ¯ρ2=maxt∈[0,T]1|1+βd(t)| and ¯c=maxt∈[0,T]|c(t)|. Using Theorem 2.1 and Young inequality (weighted form) to ensure (1+¯ρ22l)¯ρ22<1, we have
Taking λ-norm on both sides of inequality, we get
According to Lemma 3.2, we obtain
where
and Mk=maxt∈[0,T]|Dαtφk(0,t)|2. Selecting a sufficiently large λ such that N1<1, we can get
where q2=(1+¯ρ22l)¯ρ221−N1 and μ2,k=N2,k1−N1. Using recursion, we get
where ¯μ2,k≜maxm∈{0,1,⋯,k}μ2,m. We select λ large enough such that q2 is less than 1 and ¯μ2,k is sufficiently small. Therefore, to ensure |ek+1(t)|2λ≤ϵ2, it is sufficient to make
which means that the output error converges to the ϵ-neighborhood of zero after finite step iteration (k>2(lnϵ−ln|e0|λ)lnq2−1).
Remark 3.3. In order to satisfy the convergence condition (3.22), the learning gain β should satisfy
3.3. Open-loop PIθ-type ILC
The open-loop P-type ILC scheme for (1.2) is
where ek(t)=yd(t)−yk(t) denotes the output error and the learning gain β and γ are unknown parameters to be determined later.
Theorem 3.3. For system (1.2) and the ILC law (3.30), if the learning gain γ is bounded, and there exist the learning gain β and the constant l(l>0) satisfying
where ¯ρ1=maxt∈[0,T]|1−βd(t)|, then the output error ek can converge to the ϵ-neighborhood of zero for any constant ϵ>0 in the sense of λ-norm as k→∞.
Proof. By the definition of error, we have
Based on the formula (3.30), it is not hard to know
Applying Young inequality (weight form), we get
Using Theorem 2.1, it leads to
where ¯ρ1=maxt∈[0,T]|1−βd(t)| and ¯c=maxt∈[0,T]|c(t)|. Using Lemma 3.1, we obtain
where c3=2Γ(2θ−1)γ2TΓ(θ)2. Taking λ-norm on both sides of inequality, we have
According to Lemma 3.3, we get
where
and
Choosing λ large enough such that q3<1, it leads to
where ¯μ3,k≜maxm∈{0,1,⋯,k}μ3,m. We select λ large enough such that q3 is less than 1 and ¯μ3,k is sufficiently small. Therefore, to ensure |ek+1(t)|2λ≤ϵ2, it is sufficient to make
which means that the output error converges to the ϵ-neighborhood of zero after finite step iteration (k>2(lnϵ−ln|e0|λ)lnq3−1).
3.4. Closed-loop PIθ-type ILC
The closed-loop P-type ILC scheme for (1.2) is
where ek(t)=yd(t)−yk(t) denotes the output error and the learning gain β and γ are unknown parameters to be determined later.
Theorem 3.4. For system (1.2) and the ILC law (3.36), if the learning gain γ is bounded, and there exist the learning gain β and the constant l(l>0) satisfying
where ¯ρ2=maxt∈[0,T]1|1+βd(t)|, then the output error ek can converge to the ϵ-neighborhood of zero for any constant ϵ>0 in the sense of λ-norm as k→∞.
Proof. By the definition of error, we have
Based on the formula (3.36), it is not hard to know
Combining similar items, it leads to
Simplifying the above equation, we have
Applysing Young inequality (weighted form), we get
where ¯ρ2=maxt∈[0,T]1|1+βd(t)| and ¯c=maxt∈[0,T]|c(t)|. Using Theorem 2.1, we obtain
According to Lemma 3.1, we have
where c3=2Γ(2θ−1)γ2TΓ(θ)2. Taking λ-norm on both sides of inequality, it leads to
Using Lemma 3.3, we can get
where
CE=1+C2FEα,1(C2FTα)λα and Mk=maxt∈[0,T]|Dαtφk(0,t)|2. Selecting a sufficiently large λ such that q4<1, we can obtain
where q4=(1+¯ρ22l)¯ρ221−N3 and μ4,k=N4,k1−N3. Using recursion, we get
where ¯μ4,k≜maxm∈{0,1,⋯,k}μ4,m. We select λ large enough such that q4 is less than 1 and ¯μ4,k is sufficiently small. Therefore, to ensure |ek+1(t)|2λ≤ϵ2, it is sufficient to make
which means that the output error converges to the ϵ-neighborhood of zero after finite step iteration (k>2(lnϵ−ln|e0|λ)lnq4−1).
4.
Numerical examples
In this section, we use the following numerical examples to verify convergence conditions of the open-loop P-type ILC, Closed-loop P-type ILC, open-loop PIθ-type ILC and Closed-loop PIθ-type ILC schemes. We can also observe the convergence speed of the four iterative learning algorithms from the numerical results.
Example 4.1. We consider a boundary tracing problem of one dimensional fractional diffusion equation
where
α=0.9 and T=1. In this simulation, the output is determined as yk(t)=tφk(1,t)+(t2−t+1)uk(t), that is c(t)=t, d(t)=(t2−t+1). The output reference trajectory is yd(t)=2(t3−t2+t).
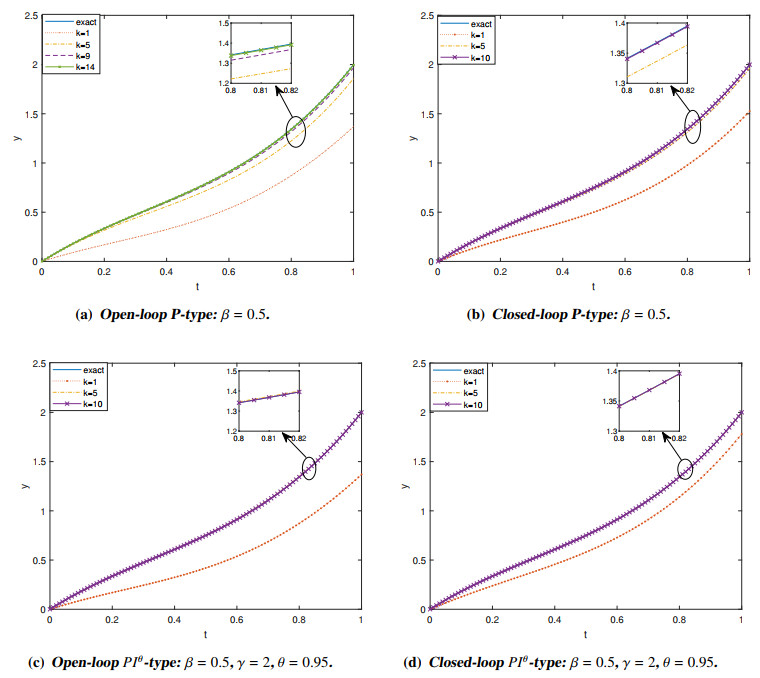
Figure 1a displays the tracking performance of the open-loop P-type ILC, while Figure 1b shows the tracking performance of the closed-loop P-type ILC. Additionally, Figure 1c displays the tracking performance of the open-loop PIθ-type ILC, and Figure 1d shows the tracking performance of the closed-loop PIθ-type ILC.
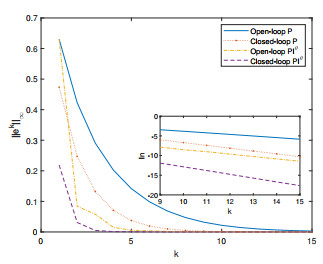
Figure 2 displays the maximum norm of four ILC schemes at different iteration times, including the open-loop P-type, closed-loop P-type, open-loop PIθ-type, and closed-loop PIθ-type ILC schemes. The results demonstrate that the closed-loop-type ILC schemes converge faster than the open-loop-type ILC schemes.
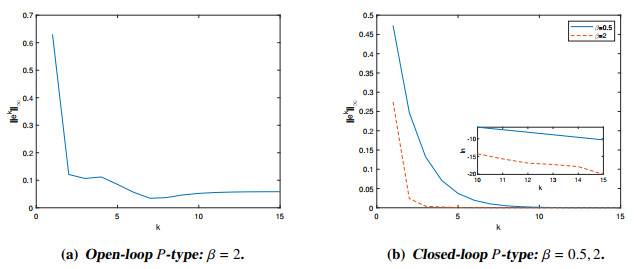
Figure 3a shows the unstable behavior of the open-loop ILC scheme. When β is set to 2, the open-loop P-type ILC scheme fails to meet the convergence conditions. Figure 3b displays that the closed-loop P-type ILC scheme satisfies the convergence conditions and achieves faster convergence speed.
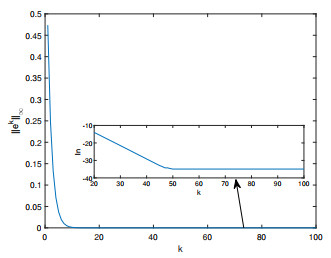
Figure 4 illustrates the convergence behavior of the maximum error ||ek||∞ of the closed-loop P-type ILC scheme over 100 iterations. Although the maximum error does not decrease at iteration k=50, the scheme remains stable and does not diverge.
Tables 1 and 2 respectively provide the maximum error of open-loop PIθ-type and closed-loop PIθ-type schemes. Comparing the data of PIθ-type and P-type schemes in the tables, it can be observed that the PIθ-type ILC scheme converges faster than the P-type ILC scheme. Comparing the data of the PIθ-type (0.5<θ<1) and the PI-type (θ=1) schemes in the tables, it can be observed that the PIθ-type ILC scheme converges faster than the PI-type ILC scheme.
5.
Conclusions
In this paper, we investigate iterative learning algorithms for boundary tracking of nonlinear fractional diffusion equation. We provide convergence conditions for open-loop P-type, closed-loop P-type, open-loop PIθ-type and closed-loop PIθ-type ILC algorithms. Numerical results demonstrate the effectiveness and stability of our proposed ILC schemes. Specifically, the closed-loop ILC schemes converge faster than the open-loop ILC schemes, and the PIθ-type ILC scheme outperforms the P-type and PI-type ILC schemes.
Acknowledgments
This research was supported by National Natural Science Foundation of China (No.11971387) and the fund of Sichuan Gas Turbine Establishment Aero Engine Corporation of China (GJCZ-2020-0018).
Conflict of interest
The authors declare that there is no conflict of interest.









 DownLoad:
DownLoad: