1.
Introduction
Cell death is inevitable, and the terminal event in the life cycle of a cell. The human body intricately regulates the homeostatic balance between the newly produced cells, as a result of cell division and those dying as a result of ageing or any pathological injury. Both, reduced as well as excessive cell death, have been linked to the development of various diseases [1]. Historically, two major forms of cell death have been recognized necrosis and apoptosis, each with its own patho-physiological mechanism and morphological manifestations. While necrosis is an undesired form of cell death induced primarily by an external injury, apoptosis is a more controlled form of cell death involving activation of proteolytic enzymes, and is also known as programmed cell death or “cellular suicide” [2]. Subsequently, other forms of cell death, namely autophagy, pyroptosis, necroptosis, oncosis etc, have also been discovered, differing primarily in their morphologic and biochemical mechanisms [3]. A more recently discovered form of cell death, ferroptosis, does not result in morphological changes, similar to the chromatin condensation seen in apoptosis, the loss of plasma membrane integrity seen in necrosis, or the formation of double membrane bound autophagic vacuoles seen in autophagy; instead, it manifests primarily as mitochondrial shrinkage and increased mitochondrial membrane density [4]. Ferroptosis is an iron dependent form of programmed cell death, characterized by the accumulation of lipid reactive oxygen species (ROS) [5]. Ferroptosis is involved in the patho-physiological mechanism of several diseases; hence, understanding its molecular mechanisms has garnered interest owing to its implications in offering targeted therapy [6].
Iron is an essential element for life that participates in a number of vital life processes. Majority of it is stored in the erythrocytes bound to hemoglobin, and the rest is stored within the splenic/hepatic macrophages and bound to other proteins (transferrin and ferritin). It serves as a medium of transport for oxygen from lungs to tissues, transfer of electrons between molecules and forms an indispensable part of various enzyme systems within body [7]. Though necessary for cellular functions, an excess of iron can prove to be deleterious, causing degradation of nucleic acids, proteins and fatty acids, by generating ROS [8]. Lipid peroxides are one such oxidative product implicated in many pathological processes, like inflammation and cancer. Once generated, lipid peroxides exert their toxic effects by disturbing the integrity, structure and composition of cell membranes, moreover, being highly reactive compounds, they further propagate the generation of ROS capable of cross-linking DNA and proteins [9]. The series of redox reactions involved in the generation of lipid peroxides are catalysed by the intracellular labile iron pool, and is referred to as Fenton chemistry [10]. The increase in free radical production, fatty acid supply and lipid peroxidation by dedicated enzymes is the key for ferroptosis [11].
2.
Discovery of ferroptosis–a form of regulated cell death
Dolma et al. in 2003 interrogated 23,550 compounds, including a novel compound erastin, for their ability to kill genetically engineered tumorigenic cells, but not their isogenic normal cell counterparts. Erastin was found to be selectively lethal to tumour cells carrying Rat sarcoma virus (RAS) mutation. Another important finding was the different morphological pattern of cell death shown by erastin treated cells, as these cells did not show the characteristic apoptotic nuclear changes, leading to the conclusion that cell death induced by erastin was non-apoptotic [12]. It was subsequently seen that another novel compound Ras selective lethal 3 (RSL3) was found to induce a similar pattern of cell death, and that this pattern of cell death could be inhibited by iron chelating agents [13],[14]. This mode of cell death, induced by erastin, RSL3 and related compounds, was previously unrecognized, and it was termed “ferroptosis”, suggesting a critical role for cellular iron in this oxidative cell death [5].
2.1. Molecular mechanisms regulating ferroptosis
Biochemically ferroptosis is characterized by the breakdown of the cellular antioxidant system, together with Fe2+ catalysed generation of a large number of ROS. Iron increases the activity of various enzymes involved in lipid peroxidation like lipoxygenase (ALOX), which causes peroxidation of polyunsaturated fatty acids (PUFA) in membrane phospholipids [15]. PUFAs are the main substrate during ferroptosis, leading to cell death. Recently, some transcription factors have also been found to regulate ferroptosis [16].
2.1.1. Iron metabolism
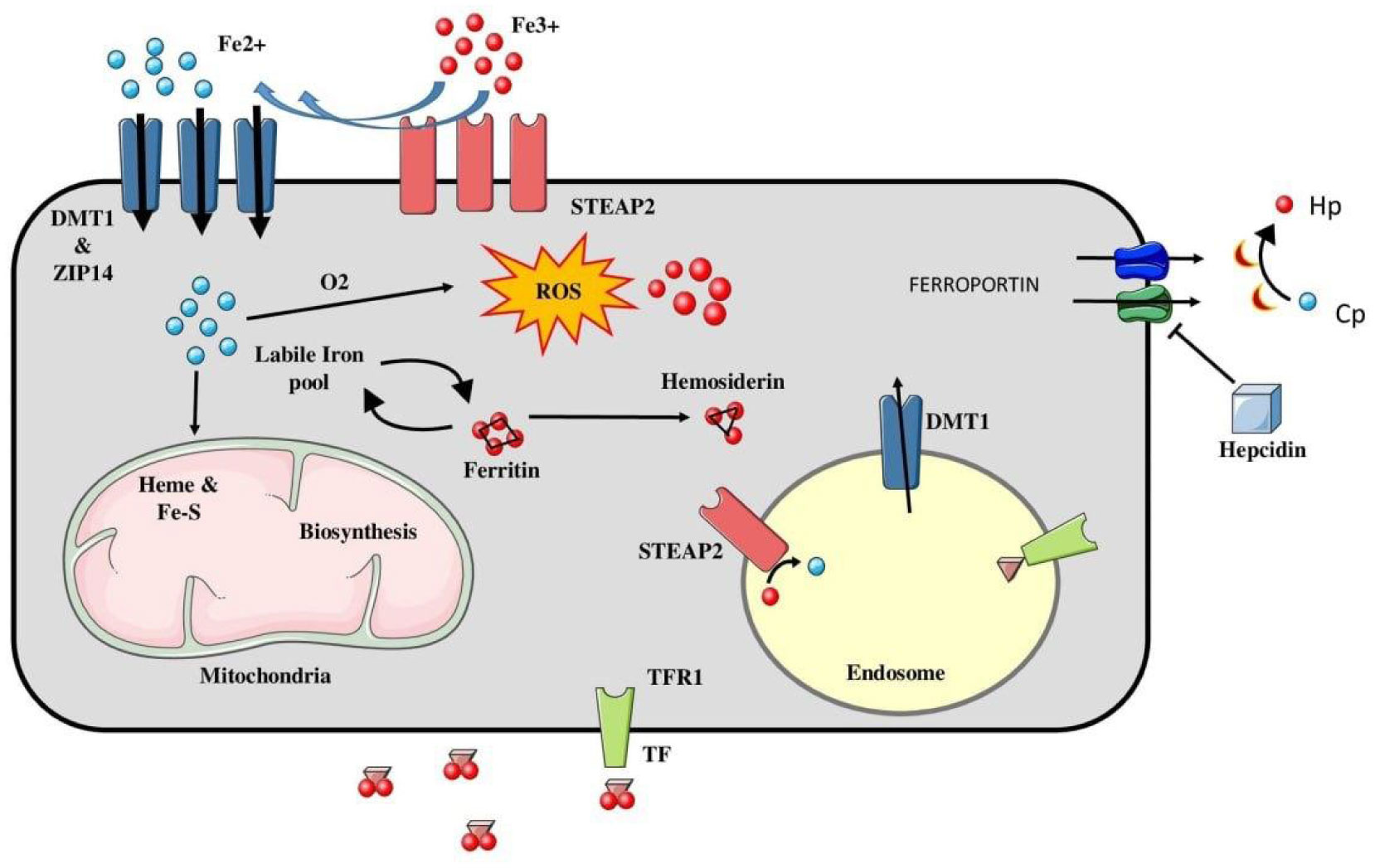
The dietary insoluble non-heme iron Fe3+ is reduced to Fe2+ for absorption. Fe3+ binds to transferrin (TF) on the cell membrane to form a complex that is endocytosed through the membrane protein TF receptor 1 (TFR1). Fe3+ is then reduced to Fe2+ by six trans-membrane epithelial antigens of the prostate 3 (STEAP 3) (Figure 1). Ferrous iron is then released from the endosome into the cytoplasm, and TFR1 is shuttled back to the cell surface to be reused by the cell. In the cytoplasm, ferrous iron is oxidized to its ferric state by cytoplasmic ferritin, and the resulting ferritin-bound iron can be either degraded for use in enzymatic reactions or stored for later use [17]. Free cellular Fe2+ can catalyze the production of ROS through the Fenton reaction, followed by lipid peroxidation and the induction of ferroptosis. Degradation of ferritin, a process termed ferritinophagy, also provides free Fe2+, which contributes to ferroptosis. Furthermore, increased cytoplasmic Fe2+ via ferritinophagy was observed to activate siderofexin (SFXN1) expression on the mitochondrial membrane. SFXN1, in turn, transported Fe2+ from the cytoplasm into the mitochondria, leading to mitochondrial ROS induction and ferroptosis in sepsis-induced cardiac injury. Divalent metal transporter 1 (DMT1) is also expressed in the outer mitochondrial membrane, and induces mitochondrial uptake of iron, thus indicating its potential role in ferroptosis [18]. Inhibition of nuclear receptor coactivator 4 (NCOA 4) mediated the degradation of ferritin through lysosomes, which increases iron storage and limits ferroptosis. Overexpression of iron-efflux protein solute carrier family 40 member 1 (SLC40A1/ ferroportin 1) extrudes iron to extracellular space, which inhibits ferroptosis [19].
2.1.2. Lipid metabolism
Peroxidation of PUFAs is an essential element of ferroptosis (Figure 2). Free PUFAs are esterified into membrane phospholipids, and oxidized to transmit ferroptosis signal. The main phospholipid that induces ferroptosis is phosphatidylethanolamine (PE). Acyl Co A synthetase long chain family member 4 (ACSL4) and lysophosphatidylcholine acyl transferase 3 (LPCAT 3) are involved in the biosynthesis of PE, reducing the expression of ACSL4 and LPCAT3, which reduces the accumulation of lipid peroxide substrates in the cell, thereby inhibiting ferroptosis [20]. Iron is also an important component of certain enzymes involved in ferroptosis, as it serves as a cofactor of lipoxygenases (LOXs) to catalyze PUFA peroxidation [21].
2.1.3. Oxidative stress
Free radicals produced as a result of redox reactions during cellular metabolism, like reactive oxygen species (ROS) and reactive nitrogen species (RNS), serve as important signals of ferroptosis. Reactive oxygen species, including superoxide anion, hydroxyl radical and hydrogen peroxide, mainly produced during mitochondrial metabolism serves as important signals of ferroptosis induction [22]. Glutamate catalyzes the production of alpha ketoglutarate, which acts as a promoter of ferroptosis through two pathways. The first is by increasing citrate production in mitochondria, which is used to produce Acetyl co-A an anabolic precursor for lipid biosynthesis. The second is through dihydrolipoamide dehydrogenase mediated production of mitochondrial reactive oxygen species [23]. Mitochondrial voltage dependent anion channels (VDACs) have been found to play an important role in ferroptosis by regulating the transport of metabolites across mitochondrial membrane during oxidative stress. Erastin acts on VDACs, leading to mitochondrial dysfunction and the release of large amounts of oxides, which leads to iron mediated cell death [14].
2.2. Antioxidant system
Antioxidant proteins act by inhibiting ferroptotic cell death. Some of the major molecular pathways regulating antioxidant system, include Solute carrier family 7 member 11(SLC7A11/xCT/system xcˉ), and the amino acid antiporter involved in the production of Glutathione (GSH), an endogenous antioxidant (Figure 3). Inhibition of SLC7A11 causes GSH depletion and induces ferroptosis [24]. The expression of SLC7A11 is regulated by a number of factors, including TP53, nuclear factor erythroid-derived 2-like 2 (NFE2L2) and BRCA1 associated protein 1 (BAP1), to name a few [25]–[27]. Another important regulator is Glutathione peroxidase (GPX4), which acts by inhibiting production of lipid peroxides by converting GSH into oxidized glutathione (GSSH). Inhibition of GPX4 activity made cells sensitive to ferroptosis and vice-versa. RAS selective lethal small molecule (RSL3) inhibits the activity of GPX4, thus inducing ferroptosis [28]. Apoptosis-inducing factor mitochondria-associated 2 (AIFM2), also known as Ferroptosis suppressor protein 1 (FSP1), is another antioxidant regulator with a potential role in ferroptosis. Its translocation from mitochondria to cell membrane catalyzes the reaction, trapping lipid peroxides in a GPX4 independent manner [29]. A reduced form of Nicotinamide adenine dinucleotide phosphate (NADPH), generated through the pentose phosphate pathway, is a principal compound limiting the peroxidation damage caused by ferroptosis. Silencing the nicotinamide adenine dinucleotide kinase (NADK) enzyme involved in the generation of NADPH, enhances erastin and RSL3 induced ferroptosis. Other ferroptosis regulators, like GPX4 and AIFM2, also utilize NADPH for electron transport, thus conferring an important role to NADPH in reactions involving ferroptosis [30].
3.
Role of p53 and other transcription factors in ferroptosis
p53, an important tumour suppressor gene, is the guardian of the genome, and its mutation results in the development of various cancers. Recently, this gene has also been found to be a regulator of ferroptosis by regulating the activity of various molecular targets [31]. One such target is downregulation of SLC7A11 expression, thereby affecting the activity of GPX4, resulting in reduced antioxidant capacity and promotion of ferroptosis [32]. Another molecular target of p53 is Spermidine/ Spermine N1 acetyltransferase-1 (SAT1), an enzyme of polyamine catabolism. p53 mediated activation of SAT1 induces lipid peroxidation, thus promoting ferroptosis [33]. Nuclear factor erythroid 2–related factor 2 (Nrf 2) plays a crucial role in modulating ferroptotic response, as it induces the expression of SLC7A11 to protect cancer cells from ferroptosis. Wild-type p53 transcriptionally represses SLC7A11 expression to induce ferroptosis, while mutant p53 bind to Nrf 2 and inhibit its transcription activity, reducing the expression of SLC7A11 [34]. Recently, it has been shown that Nrf 2 activation resists against ferroptosis in dissociated human embryonic stem cells (hESCs), and Nrf 2 activation could be a powerful anti-ferroptotic target for the maintenance of the genetic integrity [35]. The BTB and CNC homolog 1 (BACH1) promotes ferroptosis by repressing the transcription of a subset of protective genes, such as GCLM, SLC7A11, FTH1, FTL and SLC40A1. Because these genes are typical Nrf 2 target genes, functional interactions between Nrf 2 and BACH1 may occur in ferroptosis [36]. Other transcription factors with a dual control of ferroptosis include activated transcription factors (ATFs), yes related protein 1 (YAP1) and hypoxia inducible factor 1 (HIF 1) [37]–[39].
4.
Role of ferroptosis in various cancers and disease states
Ferroptosis has been linked to several different types of diseases, including cancer. Many drugs currently employed/under research for the treatment of various cancers act by inducing ferroptosis in the cancer cells.
4.1. Cancers
4.1.1. Hepatocellular carcinoma (HCC)
Low-density Lipoprotein (LDL) – docosahexaenoic acid (DHA) nanoparticles have been seen to selectively kill human hepatocellular carcinoma cells (HCC) through the ferroptotic pathway by inducing lipid peroxidation, GSH depletion and GPX4 inactivation [40]. Another drug widely used in the treatment of HCC, Sorafenib, induces ferroptosis in the retinoblastoma gene negative HCC cells [41]. Studies have also demonstrated hepatocyte Sigma 1 receptor inhibition to promote ferroptosis in HCC cells [42]. Further, some substances negatively regulating ferroptosis in HCC cells, include metallothionein 1G (MT-1G), which is seen to promote development of sorafenib resistance [43].
4.1.2. Colorectal cancer (CRC)
Cisplatin, used in the treatment of CRC, induces ferroptosis in CRC cells through GSH depletion and GPX4 inactivation pathways. p53 is found to inhibit erastin induced ferroptosis in CRC cells by blocking the activity of dipeptidyl-peptidase-4 (DPP4). Thus, loss of p53 in CRC cells promotes DPP4 mediated membrane lipid peroxidation, promoting ferroptosis [44]. The use of combination therapy, comprising cisplatin and erastin, greatly enhances the anti-tumour effect of drugs, indicating the promising role of utilizing the mechanism of ferroptosis for anti-tumour therapy against various cancers [45].
4.1.3. Breast cancer
Targeting the ferroptotic pathway of cell death is found to have a potentially therapeutic role in the treatment of triple negative breast cancers (TNBC). The idea behind it is reducing the activity of system Xc- by reducing cystine intake, which is an important amino acid in TNBC [46],[47]. Another potential target of therapy is MUC1-C transmembrane protein, which is highly expressed in TNBC, and plays role in maintaining GSH levels and redox potential. Targeting this protein can induce ferroptotic killing of tumour cells.
4.1.4. Lung cancer
Ferroptosis enhances the anticancer effect of cisplatin in lung cancer, as combining cisplatin with erastin improved the antitumor activity of both the substances, significantly. Similarly, combining cisplatin with RSL3 led to a reduction in tumor growth more effectively than the use of either cisplatin or RSL3 alone. The potential mechanisms used by cisplatin to synergize with RSL3 was the induction of ferroptosis via ferritinophagy. A recent investigation has suggested that erastin/sorafenib could result in cisplatin-resistant NSCLC cell ferroptosis via inhibiting the Nrf2/xCT pathway. Erastin also results in decreased radioresistance in NSCLC cells, partially through inducing GPX4-mediated ferroptosis [48].
4.1.5. Ovarian cancer
Similar to lung cancer, cisplatin triggers numerous forms of cell death in ovarian cancer as well. Erastin elevated ROS levels can induce ferroptosis to enhance the cytotoxicity of cisplatin. Combination treatment with cisplatin and erastin increases their therapeutic potential, while reducing adverse effects in in vitro and in vivo models of ovarian cancer. In ovarian cancer, superparamagnetic iron oxide nanoparticles (SPIONs) can induce oxidative stress and ferroptosis, causing inhibition of tumor proliferation, invasion and drug resistance [49].
4.1.6. Renal cell carcinoma (RCC)
SLC7A11 was shown to be markedly upregulated in multiple cell subtypes of renal cancer, and is also an unfavorable prognostic factor. SLC7A11 results in metabolic reprogramming of cancer cells to meet the biosynthetic demands of abnormal proliferation. High SLC7A11 expression retards antitumor immunity, and also promotes the proliferation, migration and invasion of renal cancer cells by enhancing GPX4 output, which inhibits ferroptosis. [50].
4.2. Other diseases
4.2.1. Acute kidney injury (AKI)
Studies have demonstrated the role of ferroptosis in causing AKI in mouse models. The incidence of mortality in AKI was seen to increase significantly in GPX4 knockout mice [51]. Ferroptosis is also implicated in acute tubular injury and ischemia reperfusion injury in mice models, a process that could be prevented by a ferroptosis inhibitor, ferrostatin [52]. Another study showed improved renal function in mouse models with nephrotoxic folic acid induced acute kidney injury upon administering ferrostatin [53]. Hence, ferroptosis is the main cell death pathway in acute kidney injury, and the drugs inhibiting this pathway could prove to be an effective therapeutic modality in improving kidney function.
4.2.2. Ischemia and reperfusion injury
Studies have demonstrated the therapeutic aspect of blocking the ferroptotic pathway to limit myocardial injury caused by ischemia and reperfusion. Significant form of morbidity and mortality, following heart transplantation, is attributed to ischemia-reperfusion injury mediated inflammation, where ferroptosis was found to play a significant role by promoting neutrophil adhesion to coronary endothelial cells of inflamed myocardium. Ferrostatin is seen to limit neutrophil recruitment to myocardium and significantly preventing cardiomyocyte death, thus improving clinical outcomes [54]. Blocking the ferroptotic pathway was also seen to reduce infarct size and improve cardiac function in myocardial ischemia-reperfusion injury mediated by coronary artery ligation [55].
4.2.3. Stroke
Blocking the ferroptotic pathway is significantly seen to improve clinical outcomes and prognosis in patients of both ischemic and haemorrhagic stroke. Following hypoxic brain injury, iron deposition increases in thalami, basal ganglia, peri-ventricular and sub-cortical white matter [56]. Cell death through ferroptosis in ischemic brain injury is evidenced by reduced levels of GSH, increased lipid peroxidation and decreased activity of GPX4 [57]. Studies have demonstrated the use of ferroptosis inhibitors in patients of ischemic stroke with improvement in prognosis [58].
4.2.4. Neurodegenerative disorders
Disorders of iron homeostasis and abnormal iron accumulation in various parts of the brain and peripheral nervous system are the principal features of a number of neurodegenerative disorders, like Alzheimer's disease (AD), Parkinson's disease (PD) and Huntington disease (HD). Alzheimer's disease is characterized by cognitive impairment with significantly raised iron levels in the hippocampus [59]. Similarly, in Parkinson's disease, there is degeneration of dopaminergic neurons in pars compacta and substantia nigra, areas that are rich in iron [60]. Excessive iron accumulation leads to ROS production and lipid peroxidation, together with a decrease in GSH and GPX4 levels, preparing the grounds for ferroptosis mediated neuronal cell injury. Application of ferroptosis inhibitors improves clinical outcome in AD. Also, the use of deferoxamine (DFO), an iron chelator, prevents oxidative stress injury, improving motor neurological symptom and preserving motor functions in early PD patients [61]. Huntington disease is characterized by abnormalities of glutamate and GSH levels with reduced GPX activity in erythrocytes, leading to ferroptosis [62]. Again, the use of ferrostatin and iron chelators has a protective effect on neurons [63]. Other neurodegenerative diseases where ferroptosis is implicated in the pathophysiology, include Amyotrophic lateral sclerosis (ALS), Friedreich's ataxia (FRDA) and Periventricular leukomalacia (PVL) [64],[65]. Studies have demonstrated protective roles of ferroptosis inhibitors, thus providing promising treating modalities for the treatment of these neurodegenerative diseases [66].
4.2.5. Type 2 diabetes mellitus and gestational diabetes mellitus
The global prevalence of one of the most debilitating chronic metabolic disorders, diabetes mellitus, was estimated at 10.5% (536.6 million people) in 2021, and projected to rise to 12.2% (783.2 million people) in 2045 [67]. Pathogenesis of Type 2 diabetes mellitus mainly revolves around insulin resistance, leading to progressive secretory insulin defect. Studies have shown pancreatic beta cells to be low on antioxidant enzymes like superoxide dismutase (SOD), GSH peroxidase and catalase, making it prone to oxidative stress because of ROS accumulation, and triggering ferroptosis [68]. Human pancreatic islet beta cells were demonstrated to show reduced glucose-stimulated insulin secretion (GSIS) capacity on treatment with ferroptosis inducer erastin in vitro, which was prevented by pre-treating with ferroptosis inhibitor Fer-1 or DFO [69]. Thus, pro-ferroptotic factors induce pancreatic beta cell dysfunction, showing that monitoring ferroptosis related factors may help in early diagnosis and treatment of type 2 diabetes mellitus. Ferroptosis is also implicated in the pathogenesis of gestational diabetes mellitus by studies demonstrating raised serum ferritin levels and oxidative stress index in pregnant females with high pre-pregnancy body mass index, which leads to the generation of ROS via a fenton reaction, making pancreatic beta cells liable to injury and death by triggering ferroptosis [70],[71]. The roles of ferroptosis and ferritinophagy have also been demonstrated in causation and progression of diabetes related complications, like cardiomyopathy, nephropathy, retinopathy, neuropathy and diabetic foot, thus targeting ferroptosis could also provide potential prevention and treatment options for complications of diabetes [72].
4.2.6. Male fertility
Iron helps in maintaining sperm motility and its energy metabolism, and plays a key role in sperm physiology. However, iron overload can lead to disruption of the hypothalamic/pituitary/gonadal axis, leading to hypogonadism and low testosterone levels. Iron overload also causes ferroptosis-related gene expression and enzyme inactivation, which may result in the dysfunction of the male reproductive system. By inhibiting ferroptosis, busulfan induced mice oligospermatosis can be reversed, and the protein expression of Nrf 2, GPX4 and ferroportin1 (FPN1) could be inhibited. Some male reproductive disorders are directly related to ferroptosis because of the deposition of iron or ROS in the testis. Oxidative stress can negatively affect sperm quantity, quality and physiological functioning by promoting lipid peroxidation, mitochondrial dysfunction, DNA damage and apoptosis. Low levels of ROS can help the sperms to fertilize, whereas high levels can damage sperm and cause infertility. Ferroptosis and ROS have been intricately related to sperm DNA damage, positively linked to oxidative stress, and negatively associated with embryo quality and fertilization rate. Thus, damage to sperm DNA can reduce its ability to fertilize [73].
5.
Ferroptosis for therapy
Induction or inhibition of ferroptosis can be employed as a therapeutic modality in diseases associated with it. For cancer therapy, currently, the main approach is to use anti-cancer drugs to trigger the apoptotic death of cancer cells. However, due to the inherent and acquired resistance of cancer cells to apoptosis, the therapeutic effect is limited. Inducing ferroptosis of cancer cells is one of the best ways to avoid drug resistance. Ferroptosis inducers (FINs) can be classified into four basic catagories: Class I FINs activate ferroptosis via decreasing intracellular GSH and targeting system Xc− (such as Erastin, Erastin analogs, sulfasalazine, Sorafenib and glutamate); class II ferroptosis stimulants (e.g., RSL3 and ML162) induce ferroptosis via inactivating GPX4 directly, leading to lipid peroxidation and ferroptosis [74]; However, class III ferroptosis inducers (e.g FIN56) act by indirectly suppressing and inactivating GPX4 through the squalene–mevalonate pathway [75]; Finally, class IV FINs accelerate ferroptosis through iron overloading [76]. Apart from these, class I-IV FINs, others can also induce ferroptosis, such as zalcitabine, which damages mitochondrial DNA, causing ferroptosis in human pancreatic cancer cells [77]. As far as ferroptosis inhibition is concerned, iron chelation, lipophilic antioxidants and cleaning lipid peroxides are the three main effective methods to inhibit ferroptosis. DFO, deferiprone, ciclopirox and other iron chelators can chelate iron and prevent lipid peroxidation by inhibiting the Fenton reaction [74]. Additionally, lipophilic antioxidants, such as α-tocopherol, Lip-1 and Fer-1, act as the radical scavengers to decrease lipid peroxides and, ultimately, ferroptosis [78]. Ferroptosis inhibition has been shown to be effective in various acute neurological injuries (ischemic stroke, spontaneous intracerebral hemorrhage, subarachnoid hemorrhage, traumatic brain injury and injury to the spinal cord), as well as neuro-degenerative diseases (Alzheimer's disease, Parkinsons disease, Huntington's disease, Amyotrophic lateral sclerosis and Friedreich ataxia) [79]. In addition, inhibiting ferroptosis could prove beneficial in diseases like osteoporosis, osteoarthritis and rheumatoid arthritis [80].
6.
Conclusion
Ferroptosis, a newly discovered type of programmed cell death, is characterized by generation of ROS and iron-dependent lipid peroxidation of membrane phospholipids. Antioxidant proteins act by inhibiting ferroptotic cell death, where the system xcˉ is involved in the production of Glutathione (GSH), an endogenous antioxidant preventing ferroptosis. An enzyme, GPX4, blocks ferroptosis by inhibiting production of lipid peroxides by converting GSH into oxidized glutathione (GSSH). These specific molecular mechanisms, involving iron homeostasis, lipid peroxidation and glutathione status, affects several physiological and pathological processes leading to various diseases. Targeting the multifaceted and intricate molecular pathway of ferroptosis has shown a potential therapeutic role in several types of cancers, including HCC, CRC, TNBC, RCC, lung cancer, ovarian cancer and others. In addition, renal injury, ischemia-reperfusion injury, stroke, several neuro-degenerative diseases, DM (including gestational type) and male fertility have several ramifications by ferroptosis. Finally, induction/inhibition of ferroptosis can be harnessed for therapeutic purposes, as the pro-ferroptotic compounds are proving to be potent anti-cancer candidates, whereas anti-ferroptotic agents can be cyto-protective, alleviating multiple degenerative and injurious diseases.









 DownLoad:
DownLoad:





