Beam bending and Λ-fractional analysis

Abbreviations: PV: photovoltaic; RE: renewable energy; MAS: Multi-Agent System; HEMS: Home Energy Management System; MILP: mixed integer Liner programing; ESS: Energy storage system; ToU: Time-to-use; RTP: Real-time price; PSO: Particle Swarming optimization; EV: Electric vehicle

1.   Introduction

In recent years, energy management in buildings has become crucial to reducing excessive consumption and hence the end-user electricity bill. An energy management system can control lighting, heating, cooling, and home appliances in an optimal manner. The literature contains numerous studies and tools developed to reduce energy consumption and conserve available power. The authors in ^[1] studied the effect of household consumption, mainly the use of water heaters for long periods, and demonstrated the ability to employ alternative energy sources to supply these high loads. The study showed the importance of integrating renewable energy (RE) sources to supply the electrical loads of buildings. The Home Energy Management System (HEMS) is a system that monitors and controls the operation of a considerable number of electrical appliances to save energy and reduce costs. The Single Knapsack approach was designed to effectively manage renewable energy sources and storage systems. Future demand load forecasting was also carried out to determine the needed power generation during periods of high demand ^[2].

An intelligence system called Multi-Agent System (MAS), consisting of several collaborative environmental agents, was developed to improve building power productivity and optimize energy consumption ^[3]. Moreover, MAS is an effective tool in load control, offering optimal use of home appliances. Real-time management of power consumption was developed, taking into account users' desires and the number of electrical home appliances. Through an artificial intelligence algorithm, a group of devices was scheduled in response to demand and electricity prices ^[4]. The authors proposed an advanced technique that provides communication and control between the grid and consumers because the residential sector is regarded as a high electricity consumer. Thus, consumers will take advantage of price variations in electricity to save energy and money ^[5]. In another development, a new energy management algorithm has been proposed for smart homes using energy supplied from multiple energy sources. The management strategy was applied on a real home to reduce its electricity bill utilizing a multi-price tariff. The obtained results demonstrated the effectiveness of the strategy in controlling the amount of power being consumed ^[6].

Demand response programs are used to reduce peak demand by managing large loads on the consumer's side. The authors in ^[7] presented a new approach for scheduling home loads and renewable energy RE units to reduce the use of conventional sources. The algorithm's reliability was verified with a real case study which demonstrated that by using high-efficiency appliances, the amount of power consumed by a building can be reduced. Another study investigated various models to explain the operation of a refrigerator during the cooling and heating phases. The models were built using actual data from a home refrigerator ^[8]. Data processing, modeling and parameter determination were carried out using MATLAB ^[9]. Additionally, the author reviewed previous research on home energy management systems (HEMS), including demand response (DR) programs, smart technologies, and load scheduling controllers. The use of artificial intelligence for load scheduling is also reviewed ^[10]. An up-to-date review along with a comparative analysis of recent innovations related to building energy management systems is provided in ^[11]. In ^[12], an energy distribution system based on UK electricity pricing was developed. Based on data from 30 homes in three price ranges, the model was tested. The model was able to adapt to the price shift and save money by reducing energy consumption.

Recently, as the availability of renewable energy has grown and the performance of small-scale storage systems has improved greatly, a great amount of research has been conducted to solve the home energy scheduling problem while taking into account how well distributed generation and storage can work together. However, the goals of optimizing household energy activities are to use as much locally produced renewable energy as possible and to manage storage charging and discharging schemes in the most efficient manner.

In terms of the literature, this work makes two contributions. First, the study suggests the intelligent operation of home appliances based on a combination of renewable energy sources, such as a rooftop photovoltaic (PV) system, a wind energy system, and batteries based on nonlinear energy pricing profiles. Second, the study suggests the development of appliance operation scheduling based on available power to reduce electricity consumption while maintaining energy sustainability. As a result, unlike previous research, the end-user's goal is to produce and/or store energy at the appropriate times and in the required amounts in order to achieve cost reduction rather than simply relying on renewable energy.

The contribution of this paper is as follows:

● This work analyzes the effect of temperature change and the number of consumers on daily electricity consumption. A renewable energy system consisting of wind, photovoltaic (PV), and batteries was included to satisfy the energy demand all day round.

● The home devices were simulated to analyze the dynamic variation of consumption under the influence of weather and consumer activities.

● Optimal load management was applied to reduce the power consumption considering the available amount of RE at a particular hour.

The remainder of the paper is organized with Section 2 presenting Materials and Methods. The modeling of smart home appliances is presented in Section 3. Data and system simulation is explained in Section 4. The results and discussion are found in Section 5 and finally the conclusion is presented in Section 6.

1.1.   Literature review

A hybrid optimization method that combines Differential Evolution and Grey Wolf algorithms with real-time pricing was proposed in ^[13]. Based on the collected data from the utility, shifting loads to low-price hours was applied. The study presented an operation scheduling for home appliances connected via a wireless system. The wireless receiver acquires real-time energy pricing from a smart meter and based on the price sends a signal to the controller to switch off or on some appliances. Xiong et al. ^[14] adopted a communication system connected to electrical appliances to allow the end-user to manage their power consumption and, hence, keep overall demand below a target level. The authors in ^[15] developed an algorithm that shifts loads to off-peak or low-energy prices hours, with the results indicating that the algorithm worked with high reliability and efficiency. In another study, based on user comfort, a multi-objective optimization technique for the home energy management system (HEMS) was developed to minimize electricity costs while maintaining user satisfaction ^[16]. In ^[17], electrical appliances were simulated in order to monitor their operating times. Priority was given to wind and solar energy in feeding the loads in the building. Each appliance was mathematically modeled in MATLAB/Simulink. The authors in ^[18] use the time-varying queueing method to model end-users and their respective electrical devices. Consumer loads generated by the queueing model are then summed to build the load curve. The method demonstrated its effectiveness in scheduling electrical loads without disturbing customer comfort. An open-source Python script was utilized in ^[19] to generate a home load schedule. The optimization algorithm was proposed as a heuristic technique for energy consumption scheduling. The proposed algorithm schedules the consumption based on two power pricings, namely critical-peak-price and real-time-price, both of which are used to determine the price of electricity at peak times. In reference ^[20], the author proposed an effective multi-objective solution based on the Mixed Integer Linear Programming formulation. To achieve its outcomes, the methodology uses lexicographic optimization and secularizing functions. This feature makes the program ideal for Home Energy Management in terms of energy costs. The results demonstrated the efficacy and efficiency of the method. Another study investigated the optimal use of electrical appliances by using MILP optimization to determine the most appropriate tariff for smart consumers and optimal operation hours. In addition, optimum scheduling of a group of devices on different days can be obtained from aggregation of historical load data. This process is carried out repeatedly for different tariff options, and in the end, the best option is selected ^[21]. Marcos Tostado developed a home energy management model to control thermal appliances. Various results obtained in an isolated house were analyzed using a diesel engine as a backup generator. The results indicated that the power generated from the standby generator can be reduced by 15% by operating heat-based devices in a flexible manner, while the amount of fuel consumed, fuel cost and CO₂ emissions are improved by 12% ^[22]. In his study, the author designed an optimum size for a hybrid PV and battery storage system for a home power supply by considering reliability against grid interruptions. As a result, a new optimization framework was developed that aims to reduce electricity bills with system reliability ^[23]. The author developed an optimization problem based on time-of-use pricing. Using a powerful descriptive algorithm called the Gray Wolf Optimizer, which is compared to the Particle Swarm Optimization algorithm to show efficiency and reliability. The rooftop photovoltaic system is also integrated with the system for lower consumption costs ^[24]. In another study, a deep hierarchical reinforcement learning method was proposed for the scheduling of the energy consumption of smart home appliances and renewable energy resources, including the Energy Storage System and electric vehicle. As a result, two goals were achieved, the first of which is the scheduling of household appliances and the second being charging and discharging scheduling for ESS and EV ^[25]. Based on the combination of the Grey Wolf and Crow Search Optimization algorithms, a new home appliance scheduling system is proposed. The suggested technique is used to examine the cost of electricity, and the peak to average ratio reduction for household appliances in the presence of real-time electricity prices ^[26]. In reference ^[27], the authors analyzed the scheduling of home appliances to show the optimal approach to power consumption reduction. The scheduling system minimizes energy waste and the operational periods of time. The scheduling plan for the appliances was constructed using the C# programming language. The simulation findings demonstrate that energy usage in households may be scheduled, monitored, and managed in an efficient manner. In another study, the author presented a new model for home energy management. The proposed model uses multiple pricing schemes, namely time-to-use (ToU) and real-time (RTP) next-day pricing. The energy cost reduction problem has been solved by multiple optimization techniques, namely Particle Swarming (PSO). The results showed the effectiveness of the model ^[28]. An Energy Management model was created by the author. It balances the amount of energy needed and the amount of energy that is available. Utility prices and customer satisfaction are taken into account when simulating different types of appliances in the home, such as non-shifted, shifted and controlled loads, to observe how they would work. PV energy resources, electric cars, and battery energy storage systems can also be used to produce electricity at certain times. The model successfully reduced power consumption while still maintaining end-user satisfaction ^[29]. A system for scheduling energy supply and load demand is developed wherein rooftop PV panels, diesel generators, and energy storage devices are combined with grid electricity. To reduce daily energy costs and maintain high consumer comfort, the scheduling model utilizes mixed-integer linear programming (MILP) and "min-max" optimization methods. As a result, dynamic energy price signals were used to make day-ahead optimal scheduling decisions ^[30].

The development of sustainable supply chain models is very active at present, which leads to the integration of the concept of energy management with the sustainable development goals and, at the same time, consideration of all economic, environmental, and social factors. The author addresses the design of a sustainable closed-loop supply chain including suppliers, manufacturers, distribution centers, customer areas, and disposal centers with energy consumption in mind. To reach the goal of increasing the total profit, reducing energy consumption, and increasing the number of job opportunities ^[31]. The smart city concept proposes a revolutionary strategy for delivering energy in a city by the use of Artificial Intelligence (AI), renewable energy such as Photovoltaic (PV) technology, and Transformational Participation (TP) based on citizen incentive programs. Based on the experts' information, this study seeks to analyze the overall power consumption in Mashhad, Iran, using machine learning technologies and to propose dynamic solutions for encouraging residents' desire for renewable energy generation ^[32]. Energy shortages are a serious worry for the future growth of modern cities. The rising population increases waste output and energy consumption. This explains why both wealthy and developing countries must work for long-term growth. The author proposed an intelligent approach to connecting green building waste management and energy supply. Response Surface Methodology (RSM) and Artificial Intelligence are combined in the suggested framework ^[33].

In summary, the research gaps discovered by the literature review are as follows:

1. Although there are several scheduling algorithms, to my knowledge, there is no model that links scheduling electrical appliances, temperature change, and consumer behavior.

2. Although there are many similar uncertain models, this model predicts the energy generated by wind and solar systems and accordingly reduces energy consumption while maintaining consumer comfort.

3. The model aims to minimize the daily energy bills considering demand response.

2.   System architecture

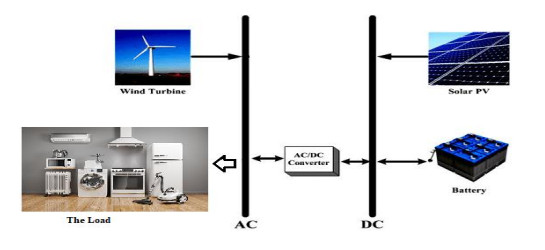
The modeled system consists, firstly, a hybrid off grid system including a solar photovoltaic (PV) system, a wind turbine, and energy storage which provide power to the building. Second the most used residential appliances such as (air conditioning, water heater, Fridge, washing machine, dryer, Lighting and Dishwasher) which are explained in details in the next section. The impact of variables such as fluctuating ambient temperature and the number of end-users in a household on power consumption is analyzed. In order to reduce power costs, the off-grid supply system and electrical demand are controlled. To demonstrate the consumption level tariff, time-of-use electricity tariffs (off-peak, average-peak, and peak) were included. The renewable system is required to meet the electrical needs of a four-room home with one kitchen and one bathroom. Because renewable energy sources are intermittent, loads are supplied with the available energy and excess energy is stored for later use. The configuration of the proposed renewable energy-based micro grid is shown in Figure 1.

2.1.   Off-grid Hybrid power system

Stand-alone hybrid system technologies combine some renewable sources such as (photovoltaic (PV), wind, diesel, hydrogen, and fuel cell) and storage systems (battery, fuel cell) without connecting to the utility grid. These systems are used for rural electrification in places without grid-connected. However, these systems involve many generations of units to reduce the operation and maintenance costs, transmission lines, losses, and increase the power quality. This study investigated the optimal hybrid system design for a rural house.

2.2.   Wind system modeling

Power generation from the wind resource mainly depends on wind speed, shaft height, and turbine characteristics. Wind speed at a particular height can be calculated from the following equation ^[20]:

where $u\left(h\right)$ denotes wind speed at hub height h, $\mathrm{u}\left({\mathrm{h}}_{\mathrm{g}}\right)$ is the wind speed at the anemometer height (h_g), and $\mathit{\boldsymbol{{\alpha }}}$ is the roughness factor which changes according to location and time.

According to the turbine characteristics:

where is Pw is the output power of the wind turbine. $\mathrm{V}\mathrm{c}\mathrm{i}$ represents Cut-in wind speed and ${\mathrm{v}}_{\mathrm{c}\mathrm{o}}$ represents Cut-off wind speed. Table 1 tabulates the wind turbine specification used in the modeled system.

2.3.   PV system modeling

Knowing the solar radiation incident on a tilted panel (${H}_{t}$) and PV panel specifications, the PV system output power (${P}_{pv}$) is calculated as follows:

here ${\mu }_{c}\left(t\right)$ is the instantaneous PV module efficiency which can be estimated using the cell temperature, as stated in (5):

The symbols ${\beta }_{t}$, T_c, and T_cr represent, respectively, temperature coefficient, actual cell temperature, and standard cell temperature (25 ℃).

PVA in (4) is the total area of the solar PV array necessary to meet the load requirement. The PVA can be computed using Eq (6):

where ${F}_{s}$ is the safety factor and ${V}_{F}$ is the variability factor accounting for the influence of radiation fluctuations, ${\eta }_{c}$ represents the efficiency of the system ^[21]. The main specifications of the employed solar PV module are provided in Table 2.

2.4.   Storage system modeling

It is more difficult to maintain an energy balance when renewable generation is integrated into residential buildings. This might result in high frequency variations. Energy storage systems (ESS) are important to sustain the balance between sources and loads, as well as to supply power quality modification in the event of unexpected changes in voltage. Battery configuration, backup duration, temperature, battery capacity time, depth of discharge, and the required reserve power all impact the ESS rating. Eqs (5) and (6) express the battery's charging and discharging schedule.

where ${P}_{PV}$, ${P}_{WT}$ represent the output power of photovoltaic and wind systems, ${P}_{ch}$ and ${P}_{dch}$ depicts the batteries power in charging and discharging mode respectively.

BES can only work in one mode at any given time, either charging or discharging. The battery's charging and discharging voltage is determined as follows:

1- Charging mode:

${E}_{ch}$ represents the charged energy for every hour, and ${\eta }_{conv}$ is the efficacy of the charging system

2- Discharging mode:

${E}_{dch}$ represents the discharged energy for every hour, and ${\eta }_{conv}$ is the efficacy of the charging system

SOC(t): the state of charge at time t

SOC (t − 1): the state of charge at time t − 1

3.   Modeling of smart home appliances

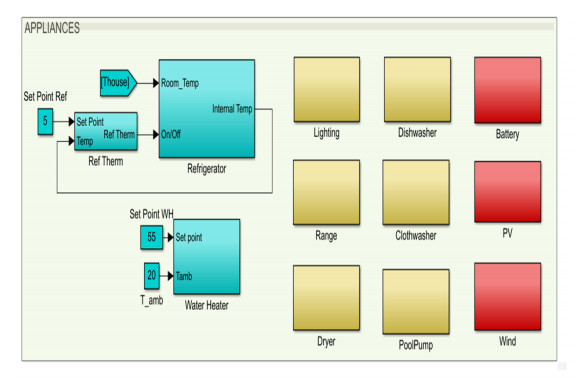
The control panel shown in Figure 2 is modeled to control the operation of household appliances. Firstly, the characteristics of the household appliances, the number of individuals, and weather data such as temperature and wind speed are inputted to create a correlation between energy consumption and people's activity. Concurrently, the generated power from the wind and solar systems as well as the battery state are determined. The modeling of the system, including home appliances such as lights, washing machines, water heaters, air conditioners, thermostats, and refrigerators, was accomplished using MATLAB/Simulink.

Household appliances modeling is highly dependent on the thermal properties of electrical devices, as the thermal response of these appliances impacts their energy consumption (air conditioner, water heater, refrigerator). Furthermore, it is generally understood that the quantity of electricity consumed varies significantly depending on factors such as the size of the home, the number of family members, the ambient temperature, and the energy efficiency of the appliances in use. The domestic thermal system is described by Equations from 14 to 18. In Eq (14), heat capacity (C) is the amount of energy required to increase the system temperature, Q is the transferred heat to the system, m is the mass, and ∆T is the temperature variation during a particular time interval. The heat capacity can be calculated using Eq (15) ${C}_{P}$ symbolizes the volumetric heat capacity at specific heat and $\rho$ represents the density. ${C}_{v}$ in (16) is the heat capacity per unit volume. On the other hand, the transferred heat per unit area, in consideration of stationary heat conduction through a large surface with a thickness $\Delta X = L$, can be calculated using Eqs (17) and (18) ^[25].

3.1.   Modeling of air conditioning and water heating system

The air conditioner (AC) is programmed to turn on when the temperature is higher than a specified set point and turn off when the room reaches the desirable temperature. In contrast, the heating system switches on if the indoor temperature (θ_in) is lower than the set point and switches off otherwise. θ_in strongly affects the power consumed by the AC and heating system. The indoor temperature can be determined from the following equations ^[26]:

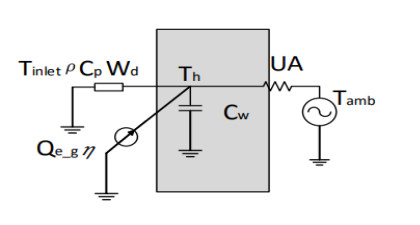
In practice, θ_in depends on the ambient temperature effect on the air conditioner v_ac and the heater v_ht at any instant of time. A(t) is the occupants' house activity level. u_ac and u_ht are the cooling and heating effects of on-state, respectively. I_ac and I_ht are the impacts of outdoor and indoor temperature changes on the AC and heating systems, respectively. The graphical interface of the AC and water heater is shown in Figure 3. Figure 4 illustrates the equivalent thermal circuit of the water heater.

3.2.   Fridge operation constraint

A standard refrigerator has mechanical elements that create a cold environment. The components include an evaporator, compressor, expansion valve, throttling device, and condenser, all of which manage the refrigeration cycle and maintain the desired level of cooling. The following formula represents the mathematical model of the refrigerator:

where ${\theta }_{fr}$ stands for the inner temperature of the fridge at a time that is dependent on a previous state ${\theta }_{fr}$ (t − 1). Fridge temperature ${A}_{fr}\left(t\right)$ is directly related to the house activity level i.e., as the consumer activity increases, cooling demand also increases; thus, power consumption is intensified. ${v}_{fr}$ represents the fridge off-state temperature effect, ${u}_{fr}$ represents the fridge on-state temperature effect, and ${S}_{fr}\left(t\right)$ describes the switching control of the fridge. The symbol ${\alpha }_{fr}$ symbolizes the heating impact of the off mode on the refrigerator. It is worth mentioning that the intensive usage of the fridge may raise the indoor temperature above the set point, resulting in higher energy consumption to cool it down once again. Figure 5 presents the graphical user interface to control the refrigerator.

3.3.   Operational constraints of washing machine and dryer

The washer and dryer are considered to be controllable loads. The formulae below are used to constrain the machine's operation under different conditions:

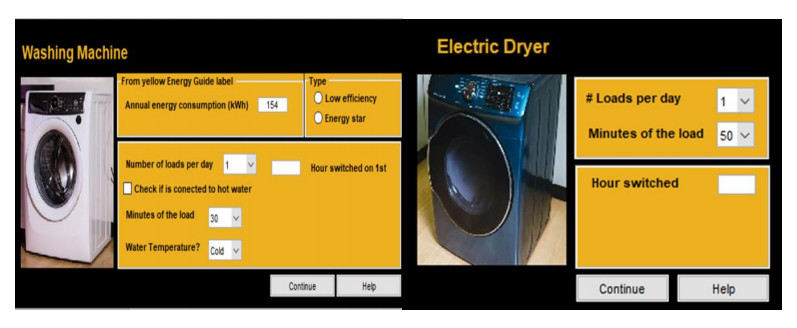
Regarding the operation constraints stated in Eqs (23) and (24), ${S}_{i}\left(t\right)$ is the switching mode of both machines; it is 1 in the case of the machine being turned on and 0 when turned off. u_i(t) and v_i(t) are, respectively, the start and stop of the same machine (i) at a specific time (t). Restraining the appliance to function at a specified operation time ${O}_{i}^{rt}$ is accomplished with Eq (25). Equation (26) states the operation constraints at a maximum time ${O}_{i}^{mst}$ where m is a positive integer. Limiting the device to operate between an upper time U_i and a lower time D_i is achieved with Eqs (27) and (28). The user-defined interface of both machines is shown in Figure 6.

3.4.   Lighting

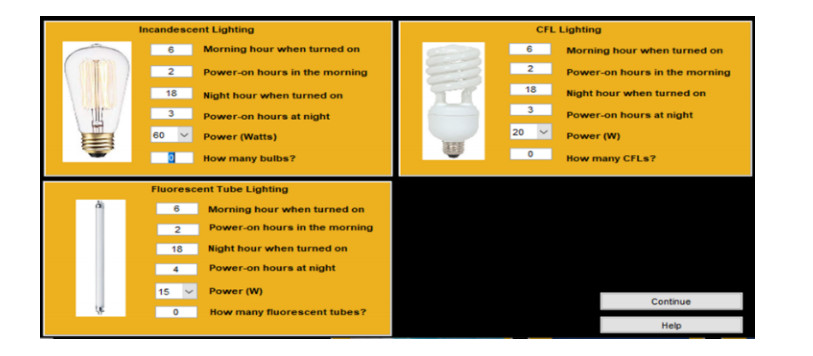
Incandescent lamps, compact fluorescent lights (CFL), and fluorescent tubes are commonly used in buildings. In fact, most residential homes use a combination of these types. Although CFLs and fluorescent tubes are initially expensive, they work for longer and with greater efficiency, bringing about significant long-run savings. The simulation of the lighting system has also been executed, with the graphical user-defined interface being shown in Figure 7. Input data include the number of lights, power rating, and operation time

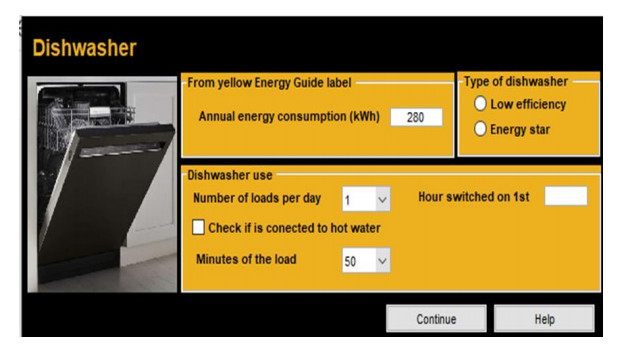
3.5.   Dishwasher modeling

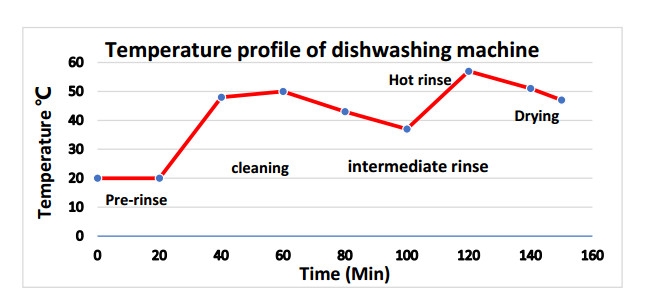
The dishwasher (DW) draws a large amount of power over a short period, so it is important to be involved within the demand management program ^[23]. A typical graphical interface and operation process for DW are shown in Figure 8. Firstly, the DW is filled up with water for about 15 minutes, during which it consumes a constant power P₁. Then, it heats the water, thus consuming a different amount of power P₂. The duration of P₂ varies depending upon the water temperature compared to the set point counterpart. Next, the plates are sprayed with hot water and detergent; rinse water and draining then take place. This consumes a different amount of power P₃. According to ^[24], 55% of the consumed energy by DW is accounted for heating water. The temperature profile of DW is exhibited in Figure 9.

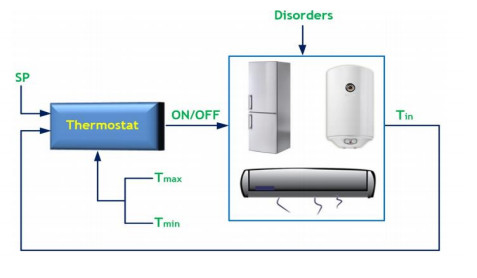
3.6.   Smart thermostat

The intelligent thermostat accompanies heating and cooling devices. Such a thermostat is a regulator programmed to adjust the temperature over the course of the day. They are efficiently used to regulate a house's temperature all year round. A scheme of the programmable thermostat is shown in Figure 10. Moreover, the user can specify the operation period of the device.

4.   Data and system simulation

The goal of this study was to find out how much consumed power in a single household, taking into account factors like the temperature inside and outside, the number of family members, the size of the house, electrical appliances time of use and residence location. The study was conducted based on data for two scenarios, one on a winter day and the other on a summer day. Renewable energy generation systems have been simulated, and temperature, solar radiation, and wind speed data are entered for each scenario to obtain the maximum power. The following section explains in detail each scenario.

4.1.   Scenario Ⅰ

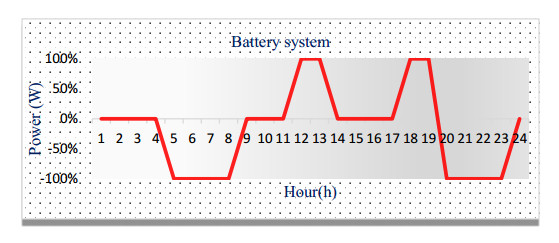
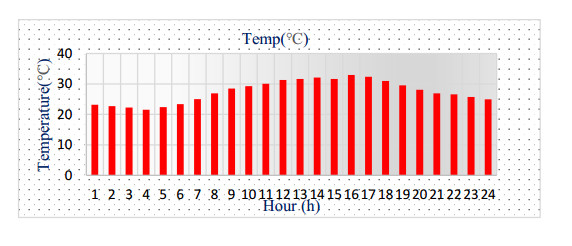
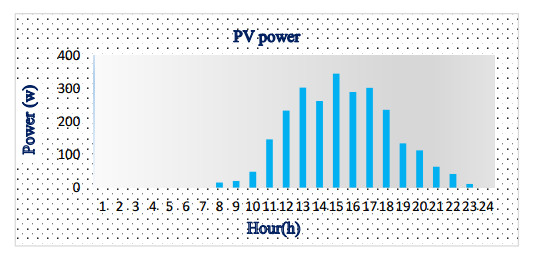
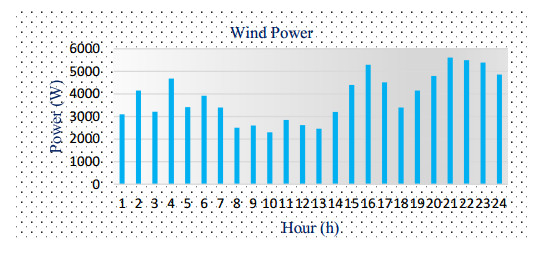
In this case, we simulated household energy profiles on a 24-hour horizon in one summer day to create home EMSs by modeling optimal on/off decisions of the electrical appliances. The simulation takes into account power generation units such as PV and wind generators, as well as battery energy storage which will be delivered to the building loads. The output power varies over time because of the change of wind speed and solar irradiation. All the appliances and power sources were modeled using MATLAB Simulink. The Household has four rooms, one kitchen, a bathroom and a balcony. Table 4 below states the contraction of the house with each room having a number of appliances as presented in Table 2. Figures 11 to 14 show the power generation data from (the PV, WT, ESS), and the weather temperature, respectively.

4.2.   Scenario Ⅱ

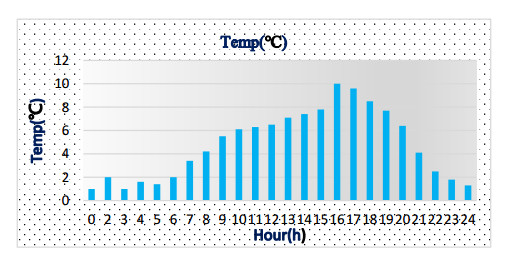
During a one winter day, the specified household appliances and generating systems described in the first scenario are simulated and managed again. The user behavior in terms of power usage changes when the weather conditions (ambient temperature and humidity) fluctuate. Similarly, seasonal variations in weather conditions impact the production of renewable units, which must be taken into account by the management model. Figures 15, 16 and 17 show the ambient temperature, PV output power, and wind systems. The scheduling of household appliances is based on user preference. This mode of operation involves an hourly computation of consumed and required power.

5.   Results and discussion

The tasks performed by household appliances can be programmed and scheduled to start and stop at a specific time while considering a typical smart home with a water heater, lights, washing machine, dryer and refrigerator. Assuming residents usually arise at 6 am and since solar PV energy is available only for a limited number of hours during the day, and that wind energy can be obtained throughout the entire day, the operation of some loads has been shifted to the daytime period to ensure that there is sufficient power.

The optimal hybrid system is designed to supply the household with a full load. Due to the fact that wind energy is produced for most of the day and PV energy can only be produced during daytime hours, batteries are required to provide electricity during the hours when no solar energy is available. In this study, the installation costs for solar energy systems are lower than those for wind energy systems. Greater fluctuation in wind power generation requires additional storage capacity to deal with uncertainty and ensure system reliability. Therefore, the most reliable hybrid power system design incorporates both wind, photovoltaic generation and storage systems.

5.1.   Scenario Ⅰ

The suggested scheduling method is applied on a smart home in two distinct scenarios, each of which includes the various configurations listed in Table 4. Simulations are run on a one-day scheduling horizon with H = 24 one-hour time periods.

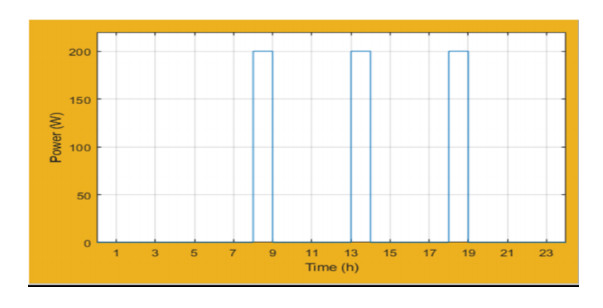
Different temperature values and numbers of consumers were simulated to demonstrate the efficacy of the model. The obtained results show that the power consumption rates of electrical appliances depends principally on customer behavior and fluctuations of indoor and outdoor ambient temperatures. Figure 18 shows the consumed power of the dishwasher over an entire summer day. As can be seen, the machine is switched on three times for two complete hours each starting from 10:00 until 23:30. The three basic operational cycles for the dishwasher are washing, rinsing, and drying. The first 30 minutes are subject to the wash cycle; the rinse cycle begins between the 60th and 80th minutes. The remaining time is for the drying cycle. The pre-wash phase, which lasts for the first 10 minutes of the wash cycle, includes the water fill and spray. At a 0.85 power factor, the dishwasher consumes 1350 W.

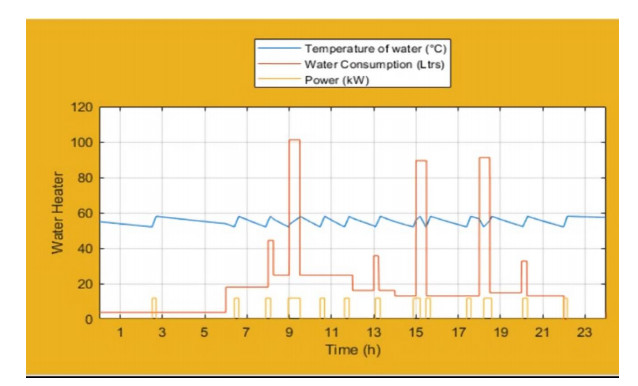
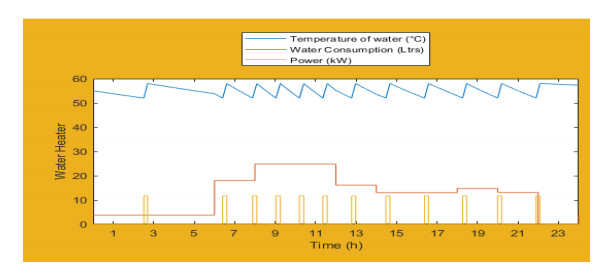
Figure 19 exhibits 24-hour power consumption, water use, and water heater temperature. The power consumption amounts maximally to 10 kW. This amount is drawn when the heater heats the water to raise its temperature from 50 to 60 ℃. The figure shows that the water heater runs throughout the day, reflecting the user activity. The water consumption ranges from 20 to 100 liters a day.

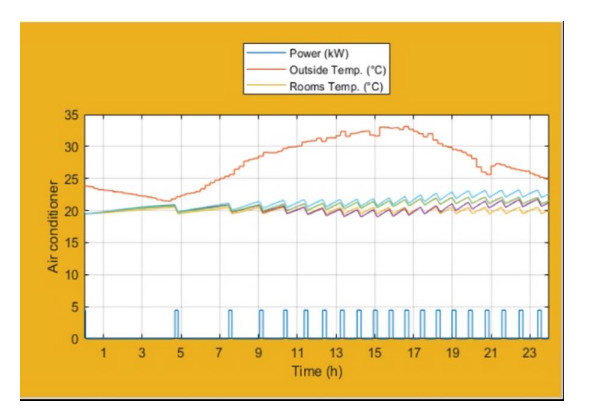
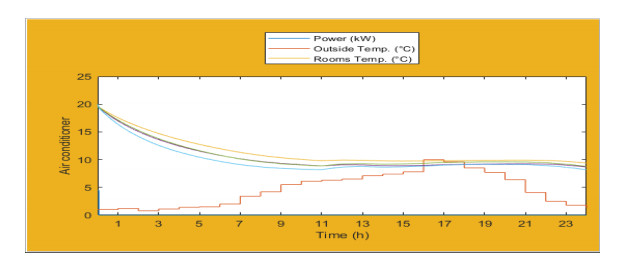
Figure 20 illustrates the consumed power by the air conditioner accompanied by the indoor and outdoor temperature over the course of a day. It is clear from the figure that as the ambient temperature increases, the operation time of the AC also increases. The AC is activated starting from 5:00. It can be observed that the AC consumes as much as 4 kW when the compressor runs. When the sensed room temperature is higher than the specified temperature, the compressor operates for almost 15 minutes to bring the temperature down to the programmed set point. The compressor's operation time (T_on) is governed by the temperature difference between ambient and indoor set point temperatures. The controller shuts down the compressor once the temperature reaches the comfortable specified value.

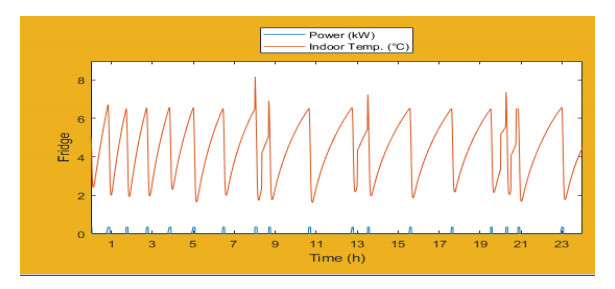
Figure 21 presents the power consumption tendency of the refrigerator for 24 hours. The consumed power during its on-state is about approximately 350 W at 0.88 PF. The regular turn-on time is 15 minutes, and the off-time is about 45 minutes if the refrigerator door is kept closed. It can also be noticed that the indoor temperature ranges from 1.5 ℃ to 7 ℃, subject to the door opening.

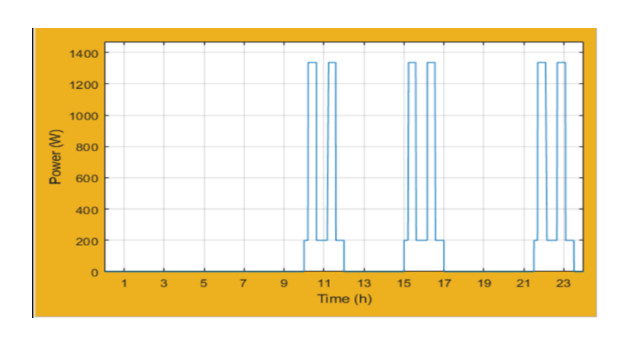
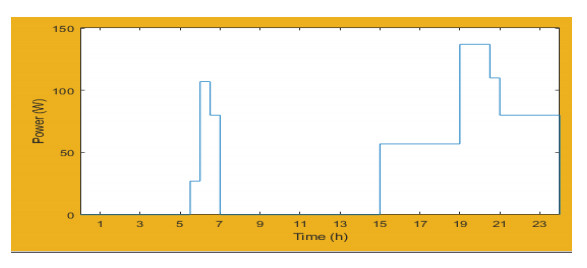
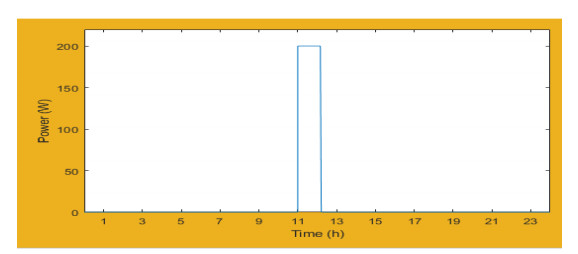
The operational characteristics of the washing machine are shown in Figure 22. The machine has three operational phases: wash, rinse, and spin. During the first minute of the wash cycle, the washing machine is filled with water, drawing 20 to 40 W. Various motor speeds occur during the wash cycle, requiring between 120 and 180 W. The rinse stage then follows, where the machine spins at varying speeds. The spinning phase is the most power-consuming cycle during which the machine consumes exactly 200 W at 0.85 PF. The three processes mentioned above are simulated in one period three times a day.

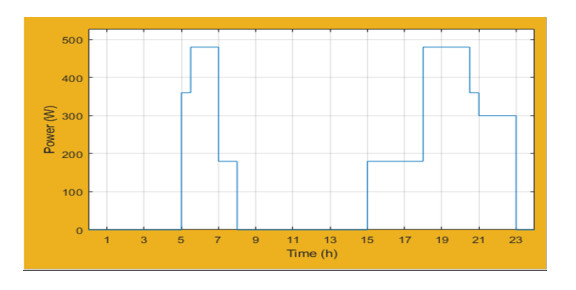
The simulated lighting system uses two types of lamps (incandescent and CFL). As presented in Figure 23, during the first 25 minutes, the consumed power amounts to 360 W. Consumption then rises to approximately 480 W, lasting for 1.5 hours. It can clearly be seen that the lighting load is intensified in the afternoon starting from 15:00 to reach its greatest value at 18:00 at 480 W. The proposed scenario assumes a partial alleviation by the consumer at 21:00 as a result of reducing the consumption to 300 W, reaching a total shutdown at 23:00.

5.2.   Scenario Ⅱ

The meteorological parameters, i.e., temperature, wind speed, and solar irradiation of a winter day, were introduced to obtain a realistic output power from the PV and WT systems. A noticeable change in energy consumption and consumer behavior was observed. Compared with the first scenario, it is found that the consumption has decreased to approximately 35%. The power and water consumption of the water heater is shown in Figure 24. It can be observed that the power consumption increased as compared to the first case, reaching 10 kW on average. The energy consumption reaches 12 kW during some operational periods. Figure 25 shows a different consumption pattern for the lighting system due to the decrease in ambient temperature. Compared to the first case, a general reduction in electricity consumption is experienced. Attributed to the home activity of the consumer, a few lamps were used in the evening with a maximum aggregate consumption of 140 W between 15:00 and midnight.

The air conditioner operational status is illustrated in Figure 26. It can be observed that the room temperature is low throughout the day, and there is almost no drawn power by the AC because it is in standby mode. Figure 27 shows the operational characteristics of the fridge. The consumed power is reported as 350 W for 15 minutes, and the indoor temperature ranges between 2 ℃ and 6.8 ℃.

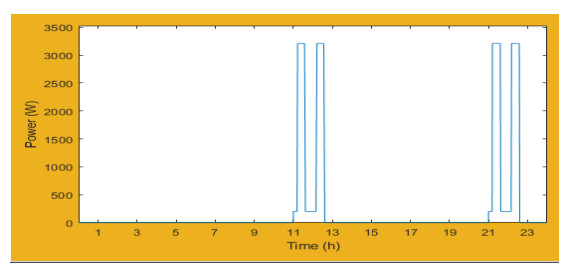
Figures 28 and 29 present the power consumption pattern for the washing machine and dishwasher, respectively. There is a very significant difference between the two appliances in terms of power use; the washing machine consumes as little as 200 W, whereas the dishwasher draws 3,200 W. The washing machine is used once compared to the three operational periods in the first scenario. This leads to a decrease in overall power consumption. Due to the water heating requirement, the consumed power by the dishwasher exceeded 3,000 W in comparison to less than 1,400 W in the first scenario.

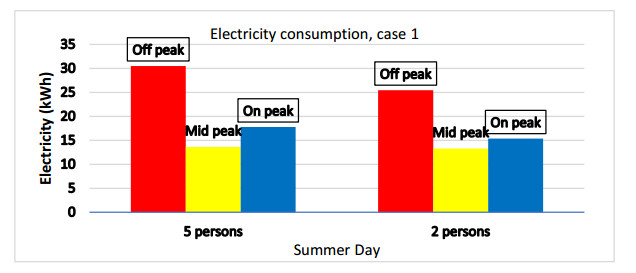
Total electrical energy consumption in a typical summer day for three demand classes is illustrated in Figure 30. Interestingly, the off-peak period saw the highest energy consumption with 30.51 kWh. During the mid-peak period, the consumed power reached 13.62 kWh, while during the on-peak period, 17.77 kWh was consumed. The figure indicates that consumers apply a demand management strategy to lower their electricity bills. This is clear from the shifted electric loads to the off-peak time. It can also be observed that the consumed power is affected by the number of family members. Influenced by the individuals' activities, the 2-person family consumes approximately 25.46 kWh during the off-peak period instead of more than 30 kWh consumed by a 5-person family

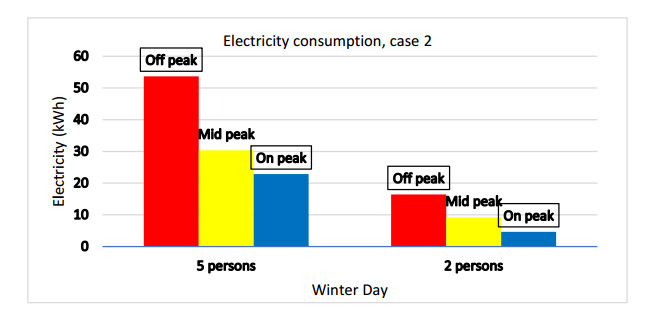
On the winter day, as shown from Figure 31, there is a significant increase in power consumption by the larger family compared to its consumption in the summer. Contrastingly, a decrease in power consumption for the smaller family can be clearly noticed. This is attributed to the reduction in ambient temperature in addition to people's behavior in using electrical appliances. For example, during the off-peak period, consumption decreased from 53.69 kWh to only 16.4 kWh. Similarly, consumption reduced from 30.34 kWh to 9.2 kWh in the mid-peak period and from 22.84 kWh to 4.63 kWh in the on peak-time. Moreover, being an economic practice, this reduction in power consumption also helps manage the available energy sources.

The table below shows the cost of energy consumption and average hourly consumption for three periods (off peak, mid peak and on peak). Consumption was highest during off peak times (30.51 kWh). During mid peak times, the consumed power was 13.62 kWh. The power consumption during peak demand times was approximately 17.77 kWh. Because the end user tends to use electrical appliances at low electricity price tariffs, it becomes clear from the table that the consumer shifts some electric devices to off peak times. Table 5 illustrates the electricity consumption for Case 1.

Table 7 shows electricity consumption in the first case with the same specifications and factors, but with only a change in the number of family members where it was reduced from five persons to two persons, which led to a decrease in the rate of consumption in the three periods with 25.46 kWh consumed off peak, 13.28 kWh mid peak, and 15.35 kWh being consumed during peak times.

In a comparison between the energy consumption in the second cases, we noticed a significant decrease in the rate of consumption due to the decrease in weather temperature and the change in the behavior of using electrical appliances. For example, off peak the consumption decreased from 25.46 kWh to 16.4 kWh. During mid peak times, consumption decreased from 13.28 kWh to 9.2 kWh. During peak times, consumed power decreased from 15.35 kWh to 4.63 kWh. This reduction of power consumption leads to increases in cost savings, as shown in Table 8.

In summary, solar energy is available only during a limited number of hours during the day, whereas wind energy can be obtained throughout the all day. So, the operation of some loads has been shifted to the daytime period to ensure that there is sufficient power from renewable energy system. Moreover, scheduling devices can contribute to reducing consumption, which in turn contributes to conserving energy or increases some necessary loads at other times. This model helps to determine the best power system design compared to the number of loads that must be operated per hour.

6.   Conclusions

Firstly, scheduling of household loads was carried out in this paper to reduce energy consumption and manage available energy sources, especially renewable ones. Household electrical appliances were simulated using MATLAB taking into consideration their usage profile in both the summer and winter seasons. The impact of indoor and outdoor temperature variations and the number of consumers were also examined. It is concluded that both factors, i.e., the temperature and the number of family members, have a significant impact on the rate of electricity consumption. Large families and increasing surrounding temperatures certainly increase power consumption. However, consumption can be significantly reduced by applying an effective management strategy in addition to changing consumer behavior. Practical benefit from this work is the consumer can control and manage household loads based on the available energy from the wind and solar systems. There is also the possibility of scheduling loads at different times to conserve energy and use only what is necessary. Secondly, this model has proven effective in simulating and controlling the work of household appliances. An energy management algorithm is implemented and evaluated to help the end-user to create schedules and to determine any required renewable energy for existing appliances. The limitation of this work can be concluded as follows, determinants of renewable energy generation and consumption can vary based on weather data and consumer consumption priority, for this reason the fast change in input weather and end user behavior make the model work slow. As future work, the operation of electrical loads will be scheduled based on the available energy at a particular hour by using particle swarm optimization (PSO) and genetic (GA) algorithms and finally comparing with the current results.

Acknowledgments

I would like to express my very great appreciation to Libyan authority for scientific research for providing financial support to complete this work.

Conflict of interest

The authors have no conflict of interest.