Citation: Ivan Ramljak, Amir Tokić. Harmonic emission of LED lighting[J]. AIMS Energy, 2020, 8(1): 1-26. doi: 10.3934/energy.2020.1.1
| [1] | Essam A. Al-Ammar, Ghazi A. Ghazi, Wonsuk Ko, Hamsakutty Vettikalladi . Temperature impact assessment on multi-objective DGs and SCBs placement in distorted radial distribution systems. AIMS Energy, 2020, 8(2): 320-338. doi: 10.3934/energy.2020.2.320 |
| [2] | Giovanni Gritti, Claudio Adragna . Analysis, design and performance evaluation of an LED driver with unity power factor and constant-current primary sensing regulation. AIMS Energy, 2019, 7(5): 579-599. doi: 10.3934/energy.2019.5.579 |
| [3] | Anthoula Menti, Dimitrios Barkas, Pavlos Pachos, Constantinos S. Psomopoulos . Contribution of a power multivector to distorting load identification. AIMS Energy, 2023, 11(2): 271-292. doi: 10.3934/energy.2023015 |
| [4] | Andrey Dar'enkov, Aleksey Kralin, Evgeny Kryukov, Yaroslav Petukhov . Research into the operating modes of a stand-alone dual-channel hybrid power system. AIMS Energy, 2024, 12(3): 706-726. doi: 10.3934/energy.2024033 |
| [5] | Nagaraj C, K Manjunatha Sharma . Fuzzy PI controller for bidirectional power flow applications with harmonic current mitigation under unbalanced scenario. AIMS Energy, 2018, 6(5): 695-709. doi: 10.3934/energy.2018.5.695 |
| [6] | Shafiuzzaman Khan Khadem, Malabika Basu, Michael F. Conlon . Capacity enhancement and flexible operation of unified power quality conditioner in smart and microgrid network. AIMS Energy, 2018, 6(1): 49-69. doi: 10.3934/energy.2018.1.49 |
| [7] | Dimitrios Barkas, Anthoula Menti, Pavlos Pachos, Constantinos S. Psomopoulos . Experimental investigation of the impact of environmental parameters on the supraharmonic emissions of PV inverters. AIMS Energy, 2024, 12(4): 761-773. doi: 10.3934/energy.2024036 |
| [8] | Nadwan Majeed Ali, Handri Ammari . Design of a hybrid wind-solar street lighting system to power LED lights on highway poles. AIMS Energy, 2022, 10(2): 177-190. doi: 10.3934/energy.2022010 |
| [9] | Yatindra K. Ramgolam, Kaviraj Bangarigadu . Simple and effective method for evaluating performance of Si based photovoltaic cell technologies. AIMS Energy, 2018, 6(4): 632-644. doi: 10.3934/energy.2018.4.632 |
| [10] | Nganyang Paul Bayendang, Mohamed Tariq Kahn, Vipin Balyan . Thermoelectric Generators (TEGs) modules—Optimum electrical configurations and performance determination. AIMS Energy, 2022, 10(1): 102-130. doi: 10.3934/energy.2022007 |
Nonlinear loads are generally source of harmonics. Harmonics can be defined as periodic state distortions of voltage and/or current waveforms. Harmonics are often used for defining distorted sine waves. Distortion is associated with currents and voltages of different amplitudes and frequencies [1]. Conventional analysis assumes power system as linear system. Today, nonlinear loads inject great amount of harmonic currents in system. Regarding system impedance, voltage harmonics can occur. This can cause potential problems in power system. As stated in [2,3], harmonics have harmful effects in: conductors, electrical machines, different types of electrical devices etc. They can cause series or parallel resonances, considering corresponding grid properties [1,2]. Harmonics can cause increased technical losses in grid (decreasing grid efficiency). Problem solution of harmonics is in filtering of them (active or passive filtering [1]). Mathematically, harmonic can be stated as a sinusoidal component of a periodic wave having a frequency that is an integral multiple of the fundamental frequency [1,2].
Voltage and current can be presented by Fourier series (when steady-state harmonics are present):
| u(t)=∑∞h=1uh(t)=∑∞h=1√2Uhsin(hω0t+θh) | (1) |
| i(t)=∑∞h=1ih(t)=∑∞h=1√2Ihsin(hω0t+δh) | (2) |
where Vh and Ih are Root Mean Square—RMS values for h-th order of harmonic voltage and current, respectively.
Applying orthogonal relation [1,2], the RMS values for (1) and (2) are:
| URMS=√1T∫T0u2(t)dt=√∑∞h=1U2h | (3) |
| IRMS=√1T∫T0i2(t)dt=√∑∞h=1I2h | (4) |
Unlike load, power system is robust enough to withstand considerable amount of harmonic currents without causing problems. It is due to smaller impedance of system compared to load impedance. Power system is not significant source of harmonic. But, it can become contributor of problems by way of resonance (when multiple distortions exist) [1]. LED bulbs represent a common single phase low power nonlinear electronically based loads. There are various approaches for nonlinear loads modeling in harmonics analysis. The most widely used approach to nonlinear loads modeling is current injection method [4]. In addition, the circuit modeling of electronically based loads, represented in [5] overcome some problems related to current injection method. However, parameter estimation algorithm should be applied for determination nonlinear model parameters of these kind of loads [6]. For simplicity, the mentioned current injection method is preferred in this paper. Primary, all nonlinear loads are presented as harmonic current injectors. Then, harmonic voltage at each system bus in power system can be obtained by solving following impedance matrix or nodal admittance equations (for all orders of harmonics under consideration):
| Uh=Zh⋅Ih | (5) |
| Ih=Yh⋅Uh | (6) |
where Vh is the vector consisting of h-th harmonic voltage at each bus that must be determined. Zh is system harmonic impedance matrix. Yh is system harmonic admittance matrix and Ih is vector of measured or estimated harmonic current representing harmonic-generating loads at connected buses. Zh can be obtained by using Z-bus building algorithm for each harmonic of interest or from the inverse of Yh. This approach is usually used in conjunction with fundamental frequency load flow analysis. Providing the grid harmonic impedance or admittance and harmonic currents injected by nonlinear loads (for consideration harmonics), the individual and total harmonic voltage distortions at each bus can be computed. More complicated analysis is necessary for unbalanced system.
There are multiple indices used to describe the effects of harmonics on power system components. Those are described in [1]. In case of this paper, Total Harmonic Distortion (THD)—Distortion Factor will be presented for voltage (THDU) and current (THDI). Those are the most used harmonic index. Where:
| THDU=√∑∞h=2U2hU1 | (7) |
| THDI=√∑∞h=2I2hI1 | (8) |
Those indices are defined as ratio of the RMS value of harmonic components to the RMS of the fundamental component. It is usually expressed in percent values. For perfect sine wave, fundamental frequency, THD values are zero. The connection of nonlinear load in the grid will change the total distorted harmonic voltage at the point of common coupling (PCC). Generally, THDU is a function of load currents I, THDI and system impedance Z at concerned PCC:
| ΔTHDU=f(I,THDI,Z) | (9) |
The component of current I supplying nonlinear load produces across supply grid equivalent impedance Z voltage drop ΔU (Eq 10).
| ΔU=Z⋅I | (10) |
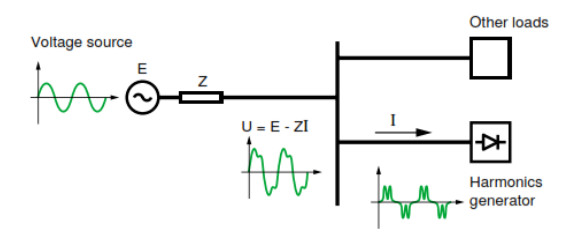
On Figure 1 degradation of grid voltage caused by nonlinear load is shown [7].
This voltage drop is the cause of voltage distortion at the PCC of a harmonic sensitive load. Figure 2 illustrates propagation of distortion along the supply feeder [8].
LED bulbs belong to the distinctive group of low power, single phase nonlinear lightning loads. In addition to LED bulbs, this group of nonlinear loads also includes compact fluorescent lightning lamps (CFL) and fluorescent lamps (FL) with electromagnetic ballast. CFL was described in [9] where it is shown that THDI value for these loads is in range of 150%, while the paper [10] represents the FL model with measurements and simulations showing that the harmonic emission of these loads is in the range of 10%. It is important to analyze different loads as light sources in cumulative action due to the diversity and attenuation effects in electrical networks [11]. The main purpose of this paper is to analyze harmonic emission of LED lighting sources. Further, aim is to model these sources. Modeling and comparing real measurements and modeled results is research method applied in this paper. Modelling of known LED lighting sources enables knowledge of their impact on PCC in planning stage (for electrical grid or installation system). It is wide applicable in grid analyzing, electrical installation design, power quality analyzing etc. These applications are effects of this research.
Standards for harmonic value limits are issued by different authority. There are now three the most exploited standards in area of harmonic value limits definition. Those are:
- EN 50160, issued by CENELEC [12],
- IEC Series 61000 Series [13], issued by IEC and
- IEEE 519 [14], issued by IEEE organization.
The existing international Standards dealing with harmonics as power quality parameter can be divided on Standards for measurement techniques and Standards for harmonic value limits. IEC 61000-4-7 [15] is Standard which defines maximum error when measuring harmonics. It is a Standard that defines the desired accuracy of the instrument. According to IEC 61000-4-7 [15], instruments for measuring harmonic values can be Class A and class B. Uncertainty in harmonic measurement is linked to maximum permissible measurement errors. Those permissible errors are defined in IEC 61000-4-7 for instruments Class A and Class B. Instruments Class A are more rigorous than instruments Class B.
Norm EN 50160 [12] is issued in mid-nineties by CENELEC. It deals with various power quality parameters. For harmonics, this Norm defines maximum allowed THDU values and individual harmonics in percentage values. Summary of this Norm for harmonics is presented in Table 1. Norm EN 50160 does not deal with current harmonic emission.
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
IEC Series 61000 Series [13] are Standards in area of power quality issued by IEC. In these Standards, THDU values and individual voltage harmonics in percentage values are defined. Further, THDI values and individual current harmonics in percentage values are defined. Summary of this Norm for voltage harmonics is presented in Table 2.
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
Table 3 presents current harmonics according to IEC 61000 Series Standard [13] for lighting loads with rated current ≤16 A.
| Individual harmonics | |||||
| 2 | 3 | 5 | 7 | 9 | 9–39 |
| 2% | 30%·cosϕ | 10% | 7% | 5% | 3% |
IEEE 519 Standard [14] deals with permitted values of harmonics in power system. For distribution system, THDU values and individual voltage harmonics in percentage values are defined in Table 4. Individual current harmonic values in percentage are defined in Table 5.
| Individual harmonics | |
| THD | 3-n |
| 5.0% | 3.0% |
| ISC/IL | < 11 | 11 ≤ h ≤ 17 |
| < 20 | 4.0 | 2.0 |
| 20–50 | 7.0 | 3.5 |
| 50–100 | 10.0 | 4.5 |
| 100–1000 | 12.0 | 5.5 |
| > 1000 | 15.0 | 7.0 |
In Table 5, ISC presents available short circuit current in PCC and IL 15 or 30-minute average maximum demand current.
Generally, EN 50160 [12] does not deals with current harmonics. For THDU, IEC 61000 Standard series [13] and IEEE 519 [14] are more rigorous than EN 50160 [12]. IEEE 519 [14] has the most rigorous conditions for harmonic values. Individual current harmonics are defined in IEEE 519 [14] regarding strength of the grid. In IEC 61000 Standard series [13], current harmonics are defined based on rated current of load and load type. In this paper we deal with lighting loads, rated current ≤16 A.
LED technology is being increasingly penetrated in modern homes and industrial facilities. LED lamps are more efficient than any conventional lamp. On other side, they are still more expensive than any conventional technology. But generally, this technology is becoming cheaper each year. It is expected that this technology will be more represented in every future year and it is due large investments in this technology development. As each load, LED lighting system has some influence on PCC in subjected grid regarding power quality. This phenomenon is under research in recent years. From the available literature, the most exploited power quality parameter are harmonics [16,17,18,19,20]. Harmonics generation from LED lamps are conditioned by rating and brand of LED manufacturer [16]. In each case is concluded that LED lamps generate harmonics regardless of manufacturer and type. In [16], several LED lamps are examined regarding harmonic emission. Additionally, for comparison, some CFL and conventional lamps were examined too. Generally, LED lamps have more THD in current than conventional lamps. CFL and LED lamps have similar emission of THD. These results are for single lamp examination (bulbs). THDI values for single LED lamps varies from 63% to 115%. Further, some examinations were conducted for more lamps used together. If identical lamps are connected together, THDI is increased. If different (different producer and rated power) lamps are connected together, THDI is decreased. However, this increasing is not significantly. When different lamps work together, their harmonics of opposite phase angles mitigate each other. That is why THDI is decreased comparing average THDI of singular lamp [16]. In [17], harmonic emission of single LED lamp is examined regarding type of filter for harmonic emission. It is a filter that can be integrated in lamp for harmonic emission decreasing. They are mostly used in lamps with dimmers. Those filters can be [17]: active filter, passive filter, valley fill. But, lamp can be even without filter. Examining single LED lamps, no matter on filter type, THDI is in each case greater than 30%. It is not foreign level of THDI greater than 150%. Among all examined lamps, THDI has the lowest value for lamps with active filter, and the greatest value is if there is no filter at all. This is similar as in [18]. In [17] too, some examinations were conducted for more lamps used together. In this case too, if different lamps are connected together, THDI is decreased. It is due using of different ballast types. But in this case, connection of more same lamps does not increases harmonic value. In [19], investigation of harmonic generation from dimmable LED lamps was performed. THDI values ranges between 47% and 360% for dimmable LED lamps. Normal LED lamps generate lower harmonic values than dimmable ones. Additionally, dimmable CFL lamps show similar harmonic characteristics as LED lamps. It was concluded that THDI value generated by LED lamp depends on ballast type too. In [20], analysis of harmonic emission on LED street lighting system was performed in one case study. LED street lighting system replaced existing one based on high-pressure sodium (HPS) lamps. After installing LED street lighting system, THDI value decreased comparing with HPS system. Comparison of street lighting systems based on HPS and LED technologies was performed in [21]. For more HPS lamps connected, the higher is current harmonic value in magnitude. For two LED street lamps, decreasing of harmonic current magnitude value was obtained. In that case, because all the complex currents belong to different quadrants, therefore having different phase angles, the combination does result in a decrease in the resultant current harmonic magnitude. Due to diversity, harmonic emission of two LED lamps is lower than one of them individually (measuring in same PCC). In this case were different manufacturers of LED lamps.
Power quality measurements of LED lamps were performed with instrument class A according to IEC 61000-4-30 [22]. Instrument has a software which can be easy installed on computer. Serial communication was used for data transfer between instrument and computer. It is shown on Figure 3. It is important note that current measurements were performed with current clamps and voltage measurements with direct connection on instrument. Sampling time was Δt=1.3310−5s.
Table 6 presents parameters of used LED lamps type. Each type has its own ID number. Lamp parameters are: voltage input, maximum current and power. Some of lamps are shown on Figure 4.
| ID | LED lamp type | Voltage input (V) | Imax. (mA) | Power (W) |
| 1 | Panel (circle) | 85–265 | 280 | 8–12 |
| 2 | Panel (square) | 220–240 | 300 | 9–12 |
| 3 | Downlight | 85–245 | 300 | 5–9 |
| 4 | Downlight | 85–264 | 300 | 12–18 |
| 5 | Downlight | 85–264 | 300 | 4 |
| 6 | Floodlight | 100–265 | 900 | 30 |
Figures 5 to 10 present measured currents (Ⅰ) and voltages (Ⅴ) waveforms for analyzed lamps.
THDU and THDI values for lamps are presented in Table 7.
| ID | THDU (%) | THDI (%) |
| 1 | < 5 | 85–265 |
| 2 | < 5 | 220–240 |
| 3 | < 5 | 85–245 |
| 4 | < 5 | 85–264 |
| 5 | < 5 | 85–264 |
| 6 | < 5 | 100–265 |
Results show that THDU values for each case are below 5%. It is relatively low value in accordance with all known Standards. Current measurements in each case show similar waveform of current. It is high non-sinusoidal waveform. It can be concluded that higher harmonic level is present.
Generally, any analyzed lamp does not have filter for reducing higher harmonic level. Harmonic analysis of measured and simulated waveform of current will be presented below. Figure 11 presents model of analyzed LED lamps.
LED lamp was modeled within EMTP-ATP. For this case study lamp ID 4 was chosen. Similar analysis is for each lamp type.
Source voltage is presented as a sum of first 15 harmonics, according to:
| e(t)=∑15n=1Ensin(nωt+θ) | (11) |
where:
| ω=2πf,f=50Hz | (12) |
Amplitude and phase angle of all harmonics is shown in Table 8.
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 317.97 | 0.9631 | 1.0554 | 0.3630 | 2.6777 | 0.2181 | 1.7832 | 0.2398 |
| Phase angle [°] | -1.0 | 179.9 | 149.2 | 188.4 | 227.2 | 151.5 | 32.0 | 168.5 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 1.6621 | 0.1075 | 1.3569 | 0.1213 | 0.8587 | 0.1059 | 0.7248 | |
| Phase angle [°] | 201.6 | 187.7 | 4.4 | 165.9 | 137.7 | 147.6 | 252.9 |
Figure 12 presents simplified equivalent model of used LED lamp. The model is applicable in harmonic analysis within EMTP-ATP.
Basic simulation parameters are:
- simulation time: Tsim = 40 ms,
- numerical method: trapezoidal,
- integration step: Δt=10−5s,
Below, simulation results will be presented and compared to measured results.
Measured/simulated parameters which will be compared are:
- waveform of LED lamp input current and
- harmonic analysis of LED lamp input current (up to 15th harmonic).
For selected LED lamp type, Table 9 presents measured numerical current values up to 15th harmonics. Table 10 presents simulated numerical current values up to 15th harmonics for selected lamp.
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1229 | 0.0082 | 0.1060 | 0.0076 | 0.0791 | 0.0097 | 0.0548 | 0.0082 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0457 | 0.0020 | 0.0447 | 0.0039 | 0.0424 | 0.0066 | 0.0390 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1289 | 0.0010 | 0.1089 | 0.0014 | 0.0787 | 0.0013 | 0.0542 | 0.0010 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0511 | 0.0011 | 0.0565 | 0.0017 | 0.0528 | 0.0021 | 0.0383 |
Comparing Tables 9 and 10 it is visible that odd harmonics are predominant. Even harmonics are more pronounced in measurement than in simulating. It is due to imperfections of instrument (measurement error of instrument, relatively low signal sampling and electromagnetic noise in laboratory). In contrary, there is good matching of predominant odd harmonics, measured and simulated.
A little disagreement is present due to measurement constraints (noise, instrument accuracy class etc.) and simulation constraints (chosen numerical method accuracy, model imperfection).
Table 11 presents measured numerical current values up to 15th harmonics in percent value (in comparison to fundamental harmonic of 100%). Table 12 presents simulated numerical current values up to 15th harmonics in percent value (in comparison to fundamental harmonic of 100%).
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 100 | 6.67 | 86.25 | 6.18 | 64.36 | 7.89 | 44.59 | 6.67 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 37.18 | 1.62 | 36.37 | 3.17 | 34.49 | 5.37 | 31.73 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 0.1289 | 0.78 | 84.48 | 1.09 | 61.06 | 1.01 | 42.05 | 0.78 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 39.64 | 0.85 | 43.83 | 1.32 | 40.96 | 1.63 | 29.72 |
The conclusion is the same as for case of comparing measured and simulated values in absolute values (A).
For selected lamp, measured and simulated THDU value is the same: 1.38%. It is acceptable value according to IEC/IEEE Standards.
Measured value of THDI is 144.2% and simulated value of THDI is 141.9%. It is relative similar value.
Current harmonics can be decreased using properly CFL filters.
On other side, comparing THD measured and simulated values, it is visible that LED lamp was simulated very good within EMTP-ATP.
Figure 13 presents comparison of measured and simulated LED lamp current. Figure 14 presents comparison of harmonic emissions of measured and simulated LED lamp current.
Thus, LED bulbs represent nonlinear loads, since according to the equivalent model of Figure 11, they contain diodes (Greatz bridge). It is clear that due to its voltage-current characteristics, diodes, or LED bulbs, represent nonlinear loads. Nonlinear loads, when connected to a sinusoidal source voltage, result in a non-sinusoidal (distorted) current. Distorted currents primarily have increased Joule losses of local loads. In addition, these currents contain higher harmonic components, which create non-sinusoidal voltage drop through the impedances of the system. This results in a distortion of network voltage that powers other loads and a general degradation of power quality. For these reasons, it is necessary to develop appropriate models of LED bulbs in order to predict different scenarios in electrical networks.
This section deals with street lighting system in low voltage distribution grid. Two systems will be analyzed. First system is one which consists of LED luminaries, placed on poles in one feeder. Installed power of system is 20 kW. Power quality measurement of LED system was performed with instrument class B according to IEC 61000-4-30 [22]. Harmonic measurements were in accordance with EN 61000-4-7 [15], class B. Current measurement was performed on feeder phases and voltage measurement on facility buses. There were no other feeders loaded at this facility during measurements. Measurement lasted for a week and it was performed according to EN 50160 [12], in 10-minutes interval.
Figure 15 shows voltage variations for measurement period. There were no any un allowed voltage deviations in this period.
Figure 16 shows current values in phases and neutral conductor for measurement period.
Figure 17 presents THDU in phases for this LED lighting system. Values are < 5% during measuring period and are in accordance with Standards.
Figure 18 presents predominant voltage harmonics. The highest values are for 5th voltage harmonic, < 4%. Still, these values are in accordance with Standards.
Figure 19 presents THDI for phases and neutral conductor in percent value. THDI in phases is up to 30%. But, it is interesting value of THDI in neutral conductor. Harmonics are predominant in period of lamps starting.
Table 13 shows measured current harmonic values up to 15th harmonics in percent value. Percent values are shown for phase and neutral conductor. For example: 3/p presents harmonic value of 3rd harmonic in phase conductor and 3/n presents harmonic value of 3rd harmonic in phase conductor.
| Harmonics | 1/p | 1/n | 3/p | 3/n | 5/p | 5/n | 7/p | 7/n |
| Amplitude [%] | 100 | 100 | 8.81 | 300 | 16.15 | 50 | 19.50 | 103 |
| Harmonics | 9/p | 9/n | 11/p | 11/n | 13/p | 13/n | 15/p | 15/n |
| Amplitude [%] | 3.70 | 58.5 | 6.16 | 20 | 1.70 | 3.60 | 0.65 | 5 |
In neutral conductor, 3rd harmonic is predominant; in phase conductors, 5th and 7th harmonics are predominant.
THDI numerical values are shown on Figure 20. These currents present losses due to harmonics. Neutral current harmonic values are predominant in most of the measured periods.
Figure 21 presents 3rd, 5th and 7th harmonic of current (numerical value) in neutral conductor. Predominant current harmonic is 3rd harmonic. Other harmonics are with considerably lower value.
Figure 22 presents 3rd, 5th and 7th harmonic of current (numerical value) in phase L2. Predominant current harmonics in phase conductors are 5th and 7th harmonic. It is the same for other phases. Other harmonics are with considerably lower value.
Conclusions for great LED lighting system can be as follows:
- THDU values are acceptable, under 5%. It is in accordance with any Standard,
- Predominant voltage harmonic is 5th voltage harmonic (under 4%). Still, these values are in accordance with Standards,
- THDI in phases is up to 30%. THDI in neutral conductor is predominant during lamp starting. Those values after starting are around 160%,
- In neutral conductor, 3rd harmonic is predominant; in phase conductors, 5th and 7th harmonics are predominant,
- 5th and 7th harmonics in phase conductors are above permitted value according to IEEE 519 [14], where for ISC/IL > 1000, max. current harmonic value is 15% (for harmonics up to 11th),
- For numerical values, greatest THDI values are for neutral conductor and phase L2 and
- Generally, numerical values of current harmonics in this case can increase technical losses in system. Still, those values are far from values needed for neutral conductor overloading.
Small street lighting system in low voltage distribution grid will be analyzed here. LED lighting system will be analyzed and compared with sodium lighting system. Generally, five high pressure sodium lamps were replaced with five LED street lamps. Voltage quality measurement was performed for sodium and LED system. Presented system is one phase system and presents lighting of one short street.
The goal is to compare harmonic parameters for old lighting system performed with sodium lamps and new installed LED system.
Power quality measurement was performed according to EN 50160. Instrument of class B according to IEC 61000-4-30 was used. Harmonic measurements were in accordance with EN 61000-4-7 [15], class B. Current measurement was performed on feeder phase and voltage measurements on facility bus. There were too other feeders loaded at this facility during measurement. Measurement lasted few days for sodium lamps and for LED lamps too.
Figure 23 shows voltage variations for measurement period. There were no any voltage deviations in this period. It is not possible to distinguish in which period sodium lamps were measured and in which LED lamps.
Figure 24 shows current values in phase conductor for measurement period. Even number of lamps and illuminate area is the same, current values are very different. It is due to lower rated current of installed LED lamps.
Figure 25 presents THDU values for measured period, parallel with current values. There is no impact of light source on THDU values. Values are < 2.5% during all measuring period and are in accordance with Standards.
Figure 26 presents predominant voltage harmonics. The highest values are for 5th voltage harmonic, < 2.2%. This is valid for sodium and for LED light system. Still, these values are in accordance with Standards.
Figure 27 presents THDI in percent value. THDI in phase conductor is up to 18.4% for sodium lamp system, and up to 11% for LED system.
For numerical values, THDI values for sodium system are higher 10 times compared to LED system. It is shown on Figure 28.
Figure 29 presents 3rd, 5th and 7th current harmonic in percent values. Predominant current harmonic in phase conductor for sodium lamp system is 3rd harmonic. Other harmonics are with considerably lower value. For LED lighting system, predominant is 5th harmonic, but similar values are for 3rd and 7th harmonic.
Conclusions for compared small sodium and LED lighting systems can be as follows:
- THDU values are acceptable, under 2.5%. It is in accordance with any Standard,
- Predominant voltage harmonic is 5th voltage harmonic (under 2.2%). Still, these values are in accordance with Standards,
- THDI is up to 18.4% for sodium lighting system and up to 11% for LED lighting system,
- For sodium system, 3rd harmonic is predominant; for LED system, 5th harmonic is predominant and
- 3th harmonics in phase conductors for sodium lighting is 15.3% and it is above permitted value according to IEEE 519 [14], where for 100 < ISC/IL < 1000, max. current harmonic value is 12% (for harmonics up to 11th),
- According to IEEE 519 [14], all current harmonic values are under permitted value for LED lighting system and
- For numerical values, THDI values are 10 times greater for LED lighting system compared to sodium system.
Now, comparison of great and small LED lighting system can be presented. Conclusions are as follows:
- THDU values are acceptable in each case (in accordance with Standard) and predominant is 5th voltage harmonic,
- THDI is up to 11% for single phase small LED lighting system and up to 30% for great system.
- For small and for great LED system to, 5th and 7th harmonics are predominant in phase conductor(s) and
- Current harmonic values are under permitted value for small LED lighting system, what is not case for 5th and 7th harmonics in great LED lighting system.
LED lighting loads are nonlinear loads. Those loads are source of higher harmonics of current. If grid is weak, current harmonics can increase voltage harmonics. Different literature describes negative influences of harmonics increased value. This paper deals with measuring and modeling of different LED lighting sources. Single LED loads (bulbs) were examined. Measurements showed high level of current harmonics emitted by single LED loads. THDI values were up to 150%, what is high level of harmonics. Single harmonic values were analyzed and some of them were above permitted value according to IEC Standards. The highest value is of 3rd harmonic. Obtained values are similar to those in literature. Single LED lights were modeled within EMTP-ATP. Similar results were obtained by measurement and by modelling. More, two LED lighting systems were examined. One system is great system of LED lighting and second one is smaller system. THDI is up to 11% for single phase small LED lighting system and up to 30% for great system. For small and for great LED system to, 5th and 7th harmonics are predominant in phase conductor(s). It is obviously that single LED bulbs have more negative impact on distribution grid in PCC than whole LED lighting systems. THDI values in neutral conductor of great LED lighting system have very high value. Further, small sodium system was compared with small LED system by replacing light loads (sodium was replaced by proper LED loads in one small urban street). THDI is up to 18.4% for sodium lighting system and up to 11% for LED lighting system. For sodium system, 3rd harmonic is predominant; for LED system, 5th harmonic is predominant. 3th harmonics in phase conductors for sodium lighting is 15.3% and it is above permitted value according to IEEE 519. THDU values were < 5% for each case study. Obtained results implies that LED lighting sources can have negative effect on voltage quality in PCC and/or in power grid. For example, neutral conductor can be overloaded due higher harmonics. This is important for installation designers in manner of correct design of installation cables. Further, impact of these results can have influence on power losses in systems. Higher harmonic increase non-active power in system. These losses should be financial validated. In industry, reactive power compensation has increasing problems due to higher harmonics. Compensation systems should have filters for predominated harmonics. For lighting, presented results of measuring and modeling can be helpful in this area. Further work would consist of modelling great LED lighting systems.
All authors declare no conflict of interest in this paper.
| [1] | Das JC (2015) Power system harmonics and passive filter design, Wiley: New Jersey. |
| [2] | Tokić A, Milardić V (2015) Power Quality, Printcom doo Grafički inženjering, Tuzla. |
| [3] | Hernandez JL, Castro MA, Carpio J, et al. (2009) Harmonics in power systems. International Conference on Renewable Energies and Power (ICREPQ), Valencia, Spain, 1-5. |
| [4] |
Chang G, Hatziadoniu C, Xu W, et al. (2004) Modeling devices with nonlinear voltage-current characteristics for harmonic studies. IEEE Trans Power Delivery 19: 1802-1811. doi: 10.1109/TPWRD.2004.835429

|
| [5] | Tokić A, Jukan A, Suljkanović V (2015) Modelling of low power electronic loads in harmonic analysis. IFAC-PapersOnLine 48: 675-676. |
| [6] |
Tokić A, Jukan A, Smajić J (2016) Parameter estimation of single-phase rectifier-based loads: Analytical approach. IEEE Trans Power Delivery 31: 532-540. doi: 10.1109/TPWRD.2015.2424914

|
| [7] | Ferracci P (2001) Cahier technique no. 199-power quality, Schneider electric. |
| [8] | Baggini A (2008) Handbook of power quality. Wiley: West Sussex. |
| [9] |
Molina J, Sainz M (2015) Compact fluorescent lamp modeling for large-scale harmonic penetration studies. IEEE Trans Power Delivery 30: 1523-1531. doi: 10.1109/TPWRD.2014.2363143

|
| [10] | Tokić A, Jukan A, Uglešić I, et al. (2018) Nonlinear model of fluorescent lamp in harmonic studies. IEEE PES Innovative Smart Grid Technologies Conference, Sarajevo, Bosnia and Herzegovina, 1-6. |
| [11] |
Lom GM, Laverty D, Morrow DJ, et al. (2019) Load and harmonic distortion characterization of modern low-energy lighting under applied voltage variation. Electr Power Syst Res 169: 124-138. doi: 10.1016/j.epsr.2018.12.029

|
| [12] | European Std. EN 50160:2011 (2011) Voltage Characteristics of Electricity Supplied by Public Distribution Systems. |
| [13] | IEC 61000-3-2 (2014) Electromagnetic Compatibility (EMC)-Part 3-2: Limits-Limits for Harmonic Current Emissions (Equipment Input Current ≤16 A Per Phase). |
| [14] | IEEE Std. 519-2014 (2014) IEEE Recommended Practice and Requirements for Harmonic Control in Electric Power Systems. |
| [15] | IEC 61000-4-7 (2002) Electromagnetic Compatibility (EMC), Testing and measurement techniques-General guide on harmonics and interharmonics measurements and instrumentation. |
| [16] | Akila A, Etman M, Youssed K (2019) Passive mitigation technique for the harmonics caused by LED lamps. International Conference on Electricity Distribution (CIRED), Madrid, Spain, 1-5. |
| [17] |
Uddin S, Shareef H, Mohamed A, et al. (2013) Power quality performance of energy-efficient low-wattage LED lamps. Measurement 46: 3783-3795. doi: 10.1016/j.measurement.2013.07.022

|
| [18] |
Uddin S, Shareef H, Krause O, et al. (2015) Impact of large-scale installation of LED lamps in a distribution system. Turkish J Electr Eng Comput Sci 23: 1769-1780. doi: 10.3906/elk-1404-243

|
| [19] | Uddin S, Shareef H, Mohamed A, et al. (2013) Investigation of harmonic generation from dimmable LED lamps. Przegl Elektrotech 89: 151-155. |
| [20] | Veloso P, Lebre A, Bastiao F (2019) LED technology in public lighting-Analysis of the impact in power quality in the low voltage grid distribution. International Conference on Electricity Distribution (CIRED), Madrid, Spain, 1-5. |
| [21] | Gil-de-Castro A, Bollen M, Moreno-Munoz A (2013) Street lamps aggregation analysis through IEC 61000-3-6 approach. International Conference on Electricity Distribution (CIRED), Stockholm, Sweden, 1-4. |
| [22] | IEC 61000-4-30 (2015) Electromagnetic Compatibility (EMC). Testing and measurement techniques-Power quality measurement methods. |
| 1. | Claudio Adragna, Giovanni Gritti, Angelo Raciti, Santi Agatino Rizzo, Giovanni Susinni, Analysis of the Input Current Distortion and Guidelines for Designing High Power Factor Quasi-Resonant Flyback LED Drivers, 2020, 13, 1996-1073, 2989, 10.3390/en13112989 | |
| 2. | Matouš Havrlík, Martin Libra, Vladislav Poulek, Pavel Kouřím, Analysis of Output Signal Distortion of Galvanic Isolation Circuits for Monitoring the Mains Voltage Waveform, 2022, 22, 1424-8220, 7769, 10.3390/s22207769 | |
| 3. | Wojciech Frydlewicz, Łukasz Michalec, Paweł Kostyła, Dominika Kaczorowska, Tomasz Sikorski, Influence of the Supply Voltage Variation on the Conducted Emission in the Frequency Range up to 150 kHz Injected into the Power Grid by CFL and LED Lamps—Case Study, 2024, 14, 2076-3417, 2590, 10.3390/app14062590 | |
| 4. | Madhu Palati, M. C. Madhu, 2023, Chapter 80, 978-981-99-1287-2, 775, 10.1007/978-981-99-1288-9_80 | |
| 5. | Krzysztof Skarżyński, Andrzej Wiśniewski, The reflections on energy costs and efficacy problems of modern LED lamps, 2024, 12, 23524847, 4926, 10.1016/j.egyr.2024.10.038 | |
| 6. | Kazimierz Kuryło, Wiesław Sabat, Dariusz Klepacki, Kazimierz Kamuda, Piotr Jankowski-Mihułowicz, Determinants of the Generation of Higher Current Harmonics in Different Operating States of the RGB LED Lamp, 2024, 13, 2079-9292, 531, 10.3390/electronics13030531 |
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
| Individual harmonics | |||||
| 2 | 3 | 5 | 7 | 9 | 9–39 |
| 2% | 30%·cosϕ | 10% | 7% | 5% | 3% |
| Individual harmonics | |
| THD | 3-n |
| 5.0% | 3.0% |
| ISC/IL | < 11 | 11 ≤ h ≤ 17 |
| < 20 | 4.0 | 2.0 |
| 20–50 | 7.0 | 3.5 |
| 50–100 | 10.0 | 4.5 |
| 100–1000 | 12.0 | 5.5 |
| > 1000 | 15.0 | 7.0 |
| ID | LED lamp type | Voltage input (V) | Imax. (mA) | Power (W) |
| 1 | Panel (circle) | 85–265 | 280 | 8–12 |
| 2 | Panel (square) | 220–240 | 300 | 9–12 |
| 3 | Downlight | 85–245 | 300 | 5–9 |
| 4 | Downlight | 85–264 | 300 | 12–18 |
| 5 | Downlight | 85–264 | 300 | 4 |
| 6 | Floodlight | 100–265 | 900 | 30 |
| ID | THDU (%) | THDI (%) |
| 1 | < 5 | 85–265 |
| 2 | < 5 | 220–240 |
| 3 | < 5 | 85–245 |
| 4 | < 5 | 85–264 |
| 5 | < 5 | 85–264 |
| 6 | < 5 | 100–265 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 317.97 | 0.9631 | 1.0554 | 0.3630 | 2.6777 | 0.2181 | 1.7832 | 0.2398 |
| Phase angle [°] | -1.0 | 179.9 | 149.2 | 188.4 | 227.2 | 151.5 | 32.0 | 168.5 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 1.6621 | 0.1075 | 1.3569 | 0.1213 | 0.8587 | 0.1059 | 0.7248 | |
| Phase angle [°] | 201.6 | 187.7 | 4.4 | 165.9 | 137.7 | 147.6 | 252.9 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1229 | 0.0082 | 0.1060 | 0.0076 | 0.0791 | 0.0097 | 0.0548 | 0.0082 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0457 | 0.0020 | 0.0447 | 0.0039 | 0.0424 | 0.0066 | 0.0390 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1289 | 0.0010 | 0.1089 | 0.0014 | 0.0787 | 0.0013 | 0.0542 | 0.0010 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0511 | 0.0011 | 0.0565 | 0.0017 | 0.0528 | 0.0021 | 0.0383 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 100 | 6.67 | 86.25 | 6.18 | 64.36 | 7.89 | 44.59 | 6.67 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 37.18 | 1.62 | 36.37 | 3.17 | 34.49 | 5.37 | 31.73 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 0.1289 | 0.78 | 84.48 | 1.09 | 61.06 | 1.01 | 42.05 | 0.78 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 39.64 | 0.85 | 43.83 | 1.32 | 40.96 | 1.63 | 29.72 |
| Harmonics | 1/p | 1/n | 3/p | 3/n | 5/p | 5/n | 7/p | 7/n |
| Amplitude [%] | 100 | 100 | 8.81 | 300 | 16.15 | 50 | 19.50 | 103 |
| Harmonics | 9/p | 9/n | 11/p | 11/n | 13/p | 13/n | 15/p | 15/n |
| Amplitude [%] | 3.70 | 58.5 | 6.16 | 20 | 1.70 | 3.60 | 0.65 | 5 |
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
| Individual harmonics | ||||||||
| THD | 3 | 5 | 7 | 9 | 11 | 13 | 15 | 17 |
| 8.0% | 5.0% | 6.0% | 5.0% | 1.5% | 3.5% | 3.0% | 0.5% | 2.0% |
| Individual harmonics | |||||
| 2 | 3 | 5 | 7 | 9 | 9–39 |
| 2% | 30%·cosϕ | 10% | 7% | 5% | 3% |
| Individual harmonics | |
| THD | 3-n |
| 5.0% | 3.0% |
| ISC/IL | < 11 | 11 ≤ h ≤ 17 |
| < 20 | 4.0 | 2.0 |
| 20–50 | 7.0 | 3.5 |
| 50–100 | 10.0 | 4.5 |
| 100–1000 | 12.0 | 5.5 |
| > 1000 | 15.0 | 7.0 |
| ID | LED lamp type | Voltage input (V) | Imax. (mA) | Power (W) |
| 1 | Panel (circle) | 85–265 | 280 | 8–12 |
| 2 | Panel (square) | 220–240 | 300 | 9–12 |
| 3 | Downlight | 85–245 | 300 | 5–9 |
| 4 | Downlight | 85–264 | 300 | 12–18 |
| 5 | Downlight | 85–264 | 300 | 4 |
| 6 | Floodlight | 100–265 | 900 | 30 |
| ID | THDU (%) | THDI (%) |
| 1 | < 5 | 85–265 |
| 2 | < 5 | 220–240 |
| 3 | < 5 | 85–245 |
| 4 | < 5 | 85–264 |
| 5 | < 5 | 85–264 |
| 6 | < 5 | 100–265 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 317.97 | 0.9631 | 1.0554 | 0.3630 | 2.6777 | 0.2181 | 1.7832 | 0.2398 |
| Phase angle [°] | -1.0 | 179.9 | 149.2 | 188.4 | 227.2 | 151.5 | 32.0 | 168.5 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 1.6621 | 0.1075 | 1.3569 | 0.1213 | 0.8587 | 0.1059 | 0.7248 | |
| Phase angle [°] | 201.6 | 187.7 | 4.4 | 165.9 | 137.7 | 147.6 | 252.9 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1229 | 0.0082 | 0.1060 | 0.0076 | 0.0791 | 0.0097 | 0.0548 | 0.0082 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0457 | 0.0020 | 0.0447 | 0.0039 | 0.0424 | 0.0066 | 0.0390 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [V] | 0.1289 | 0.0010 | 0.1089 | 0.0014 | 0.0787 | 0.0013 | 0.0542 | 0.0010 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [V] | 0.0511 | 0.0011 | 0.0565 | 0.0017 | 0.0528 | 0.0021 | 0.0383 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 100 | 6.67 | 86.25 | 6.18 | 64.36 | 7.89 | 44.59 | 6.67 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 37.18 | 1.62 | 36.37 | 3.17 | 34.49 | 5.37 | 31.73 |
| Harmonics | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Amplitude [%] | 0.1289 | 0.78 | 84.48 | 1.09 | 61.06 | 1.01 | 42.05 | 0.78 |
| Harmonics | 9 | 10 | 11 | 12 | 13 | 14 | 15 | |
| Amplitude [%] | 39.64 | 0.85 | 43.83 | 1.32 | 40.96 | 1.63 | 29.72 |
| Harmonics | 1/p | 1/n | 3/p | 3/n | 5/p | 5/n | 7/p | 7/n |
| Amplitude [%] | 100 | 100 | 8.81 | 300 | 16.15 | 50 | 19.50 | 103 |
| Harmonics | 9/p | 9/n | 11/p | 11/n | 13/p | 13/n | 15/p | 15/n |
| Amplitude [%] | 3.70 | 58.5 | 6.16 | 20 | 1.70 | 3.60 | 0.65 | 5 |