Soft-switching cells for Modular Multilevel Converters for efficient grid integration of renewable sources

1.   Introduction

1.1.   MMC

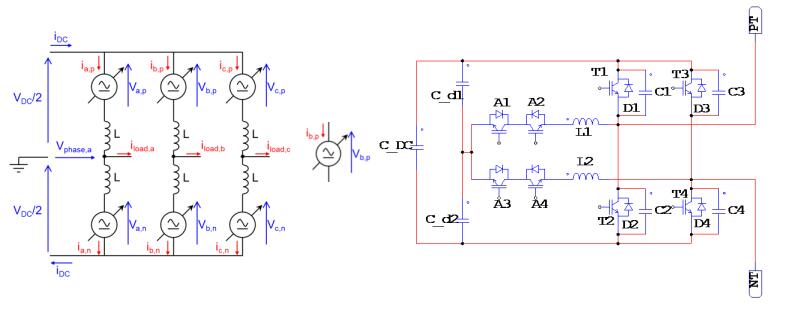
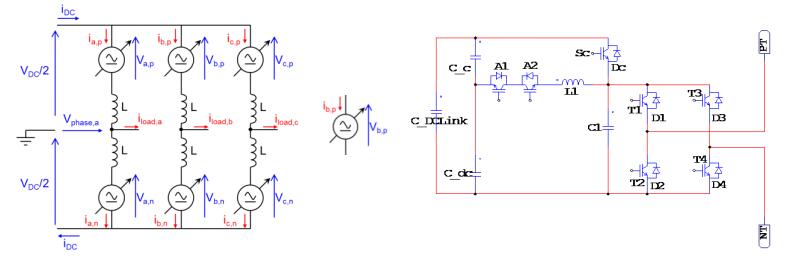
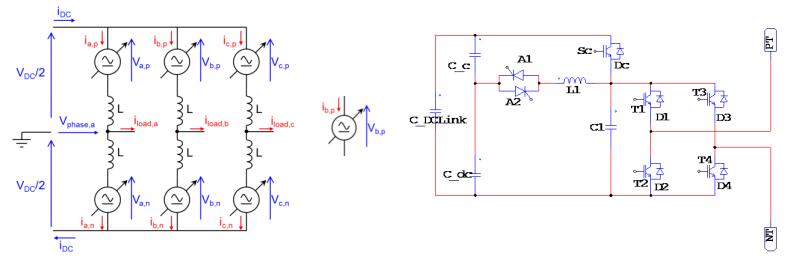
The High Power Electronics field research, in the last 10 years, has tried to generalize the converters in order to achieve higher output voltages ^[1,2,3,4,5]. Some of the most important issues are to guarantee a low converter capacitors voltage ripple ^[6,7,8] and to manage and detect the converter fault events ^[9,10,11,12] in order to have a high reliability ^[13,14]. Today, the converter that fulfils almost all the above requirements is the Modular Multilevel Converter (MMC) ^[15], shown in Figure 1. This concept doubtless represents, currently, one of the most complex and advanced multilevel energy conversion topology.

Several features, such as the remarkable modularity and the high quality of the output voltage and the input current, make the MMC concept attractive especially for high-power and high-voltage applications; the MMC gives also the opportunity to realize—with the same concept—various types of energy conversion, like DC/AC or AC/AC with any number of phases, and offers a great availability and fault tolerance, because a fault submodule can be easily bypassed and the operation can continue with the healthy submodules.

These features require, on the other hand, a high number of power devices and somehow complex control strategies: for each branch, the cascade-connected submodules can be seen like variable voltage sources that operate in dependence of the actual voltage of their capacitors.

So substantially different from the more traditional conversion systems, the MMC principle of operation is based on proper management and input/ouput balance of the energy stored inside the submodules capacitors.

In the world of Power Systems, especially in the energy transmission and distribution areas, the MMC architecture has rapidly gained wide interest and application opportunities for the so-called FACTS–Flexible Alternating Current Transmission Systems. Its distinctly modular structure permits to build effective very high-voltage/very high-power converters like STATCOMs—Static Synchronous Compensators for reactive power, HVDCs—High Voltage Direct Current DC/AC (inverters) or AC/DC (rectifiers) converters for efficient energy transmission ^[16,17] and more recently for the transmission from the marine wind generation plants (commonly referred as offshore wind farms) to the AC grids on the mainland, and SFC—Static Frequency Converters for the interconnection and energy exchange between large AC grids with different frequencies, for example AC/AC three-phase/three-phase 50/60 Hz, or AC/AC three-phase/single phase 50/16.7 Hz, being 16.7 Hz the frequency for railway traction systems in some North-Europe countries (15 kV–16.7 Hz) or for the grid frequency control ^[18].

In these 'top-end' static power conversion systems a high efficiency is mandatory, also because the gain of a fraction of a percentage in the efficiency can mean the saving of a large amount of power, maybe several thousands of kilowatts, and therefore of energy. At the same time, high quality of the conversion (reduced harmonic content of the input/output waveforms) and high reliability are other primary important features required.

Furthermore, interest around the MMC is related to the realization of the Medium Frequency Transformer or Solid State Transformer (SST) ^{[19,20,21,22]}, an evolution of the classical electromagnetic transformer toward a complex power conversion system in which the magnetic coupling between two circuits, operating at different voltages, is realized by a transformer working at a frequency level of some kHz, with expected advantages in terms of size, weight and efficiency. A marked development effort is aimed nowadays toward this technology; it looks attractive especially for medium voltage levels, so as a part of the power distribution area. The MMC acts as a frequency converter between the grid and medium frequency transformer.

Another field of research for the MMC is represented by the motor drive applications, in particular how to mitigate the very high capacitors voltage ripple that arises, especially in the motor low speed range ^[23].

The MMC concept, whatever type of conversion is considered, includes several branches, each comprising a cascade connection of n submodules equal to each other, in Figure 1 (left) the six branches of the classical MMC can be appreciated, each one represented by a variable voltage source, indicated in Figure 1 (center). A single submodule is organized as a half H-bridge, shown in Figure 1 (right) or full H-bridge, with a DC-link capacitor acting as an energy storage device. So, the MMC is originally conceived as a hard-switching converter. Then, taking into account the expectations about efficiency and quality of the processed power, the question was raised whether, by adopting some soft-switching strategy inside the submodules ^[24], it is possible to improve the efficiency of the MMC converter and increase its switching frequency so as to enhance the waveforms quality, without cause an intolerable increase of the power losses. This paper is devoted to examine these opportunities.

1.2.   Resonant topologies for power converters

Several circuital solutions have been proposed to make soft the switches commutation process in power converters, especially in the late '80s and early '90s, when the semiconductor devices then available had much more limited performance than now, especially with reference to the maximum allowed switching frequency. Planned applications were the most varied: aeronautical, PV solar, induction heating or variable speed drives. The main soft-switching principle was based on a resonant circuit, able to set up Zero Current Switching (ZCS) and/or Zero Voltage Switching (ZVS) conditions for the commutation process of the semiconductor devices. Power levels for these converters commonly ranged from a few kW to a few hundred kW; only some solutions have been dedicated to higher power levels. Compared to the hard-switching topologies, the greatest number of the necessary components-active and passive-was balanced by a series of advantages, the main ones being: the net reduction or cancellation of the switching losses, with consequent increase of the conversion efficiency; the improvement of the quality of the input/output waveforms with a reduction of the harmonic content and improved THD; the maximization of the specific power [kW/kg] and the power density [kW/m³] of the converters; the knocking-down of the acoustic noise and a more convenient sizing of the magnetic components.

In the following years, with the availability of higher performance snubberless power components, the interest in soft-switching solutions was reduced; nevertheless, the soft-switching technology showed to be an interesting idea, potentially capable to improve performance of the modern multilevel converters, where the total power is shared between multiple modules or subassemblies.

Among the several proposed soft-switching solutions, it was necessary to identify the most suitable ones with reference to the present research, that is the submodules (full H-bridges or half H-bridges) for the MMC converters, looking at an effective balancing between the largest number of components required and the performances offered. Typically, several functional characteristics linked to the presence of resonant circuits can introduce limitations or inadequacies: increase in voltage or current stresses on the main converter power components (in some cases very pronounced, more than 2 p.u., so requiring their net oversize); not safe soft-switching operations in all operating conditions of the converter (not fully controllable Zero-Voltage-Switching (ZVS) or Zero-Current-Switching (ZCS) process, depending of the load current or other constraints); 'impact' of the soft-switching circuit with the overall structure of the converter; inadequacy or only partial adequacy to the PWM modulation.

The soft-switching solutions can be classified in three main families: 1) resonant switch, if resonant circuit is applied to the individual switches of the converter; 2) resonant pole, if the resonant circuit acts on a branch of the converter; 3) resonant link, if the resonant circuit acts on the DC-link of the converter. With the obvious purpose of reducing as much as possible the number of additional components, our research it was directed toward the last two ones. Several topologies were examined: A) Resonant DC-link Converter (RDCL) ^{[25,26,27,28,29,30,31]}; B) Active Clamped Resonant DC-link Converter (ACRDCL) ^[32]; C) Notch Commutated PWM Inverter ^[25,33,34]; D) Quasi-Resonant PWM Inverter (or Modified Active Clamped Resonant DC-link Inverter) ^[25,35]; E) Zero Switching Loss PWM Converter with Resonant Circuit ^{[25,36,37,38]}; F) Active Resonant Commutated Pole Converter (ARCP) ^[25,39,40]; G) Auxiliary Quasi Resonant DC-link Inverter (AQRDCL) ^[41].

Almost all these topologies were originally proposed for three-phase inverters for electric drives long time ago; anyway, their adaptation to a single-phase H bridge is still quite easy and their interest with the new converters will be shown in the next sections.

Taking into account the various constraints described above, the ARCP and the AQRDCL topologies seem to be the most suitable to realize soft switching submodules for a MMC converter.

2.   Results

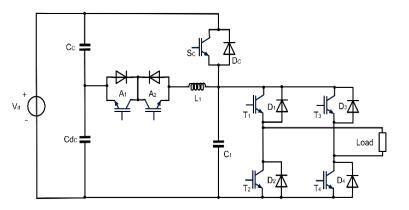
The ARCP circuit is a resonant-pole type soft-switching topology, as shown in Figure 1, so each branch of the converter includes a L-C resonant circuit built by two switches + diode pairs, A₁ and A₂, a resonant inductor L₁, a capacitive divider with a central tap C_d1–C_d2 and two snubber capacitors in parallel to the main devices, C₁ and C₂. The ARCP is able to operate in PWM without limitations, in contrast to other topologies that cannot, instead, operate in PWM, or they can do so with limitations, requiring a somehow adapted PWM modulation (quasi-PWM).

The commutation of the main switches T₁ and T₂ occurs in ZVS: the turn-off is ZVS, by itself, thanks to the capacitive snubber; the turn-on is ZVS for the effect of the resonant circuit, because the snubber capacitors C₁ and C₂ also act as a resonant capacity. The auxiliary switches A₁ and A₂ turns-on and off in zero-current soft-switching mode, requiring a minimal turn-off current capability. Unlike other competing topologies, an important property, advantageous for this application, is the resonant circuit connected in parallel to the commutating switch only in a small time interval around the switching; outside the switching conditions, all the components of the resonant circuit are not influenced by the load current. So, the operation " to the terminals" of the ARCP resonant cell is almost similar to that of a common hard-switching cell, and therefore it does not influence or 'disturb' the overall operation of the MMC. More, this is a benefit for the resonant inductance size, which must not sustain continuously the load current. The auxiliary switches A₁–A₂ and the inductor L₁ can be sized in a convenient mode too, because they conduct the resonant current, whose peaks can be relatively high (1.6–1.8 p.u. according to ^[39]), but the duty cycle is low, so the RMS current is low as well, and therefore the losses are small, too. On the contrary, in other simpler topologies, requiring less auxiliary components, the resonant inductance is series-connected to the DC-link cell, so it must conduct the load current; furthermore, the width control of the ZVS interval is more difficult and often depends on the load current.

The ARCP presents several advantageous features compared to other competing resonant solutions: it is claimed to be suitable for high power converters (≈1 MVA), can operate with relatively high switching frequency, 10–30 kHz, its efficiency is high and the silicon area increase, compared to a conventional hard-switching solution, is about 20% due to a favourable sizing of the auxiliary switches.

The analyzed MMC with the full H bridges soft-switching ARCP submodules is shown in Figure 3.

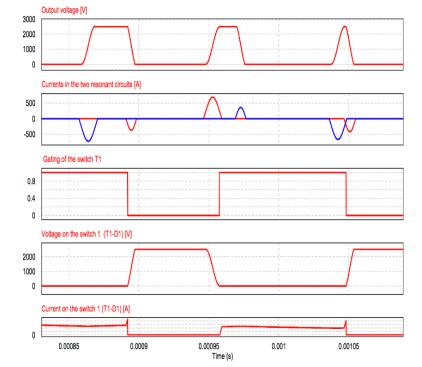
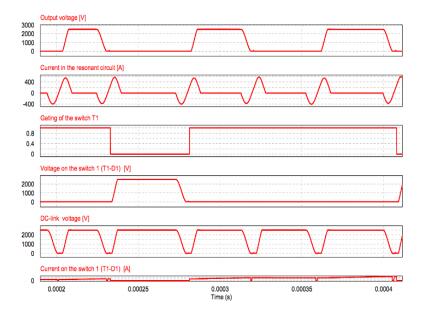
In Figure 4 some simulation diagrams, referred to a single cell (one MMC submodule, full H-bridge as shown in Figure 3 Right) ARCP, are shown. The ZVS turn-on and turn-off are clearly appreciable; the edges of the output voltage are less steep than in hard-switching, so the power components are less subjected to dv/dt stress.

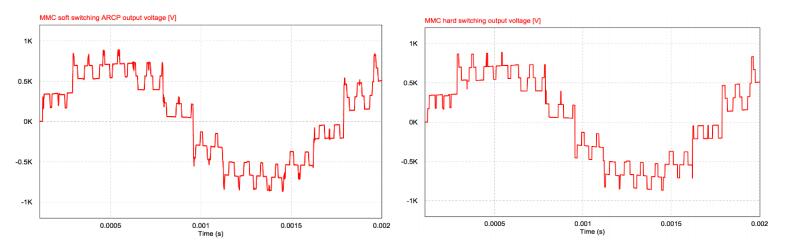
Figure 5 shows the simulation diagram of the output voltage of a MMC (only 4 submodules for branch, for simulation speed reasons) equipped with full H-bridges soft-switching ARCP submodules: one can appreciate a waveform that does not differ appreciably from that of an usual hard-switching MMC converter, represented in the same figure. The results of a study about the implementation of the ARCP topology to the submodules for MMC for high-power, high-voltage STATCOM and HVDC (1100 MW, 400 kV) are shown in ^[42].

The power components examined are 4.5 kV–4 kA IGCTs: the improvement of efficiency is up to 45% compared to a conventional hard-switching solution.

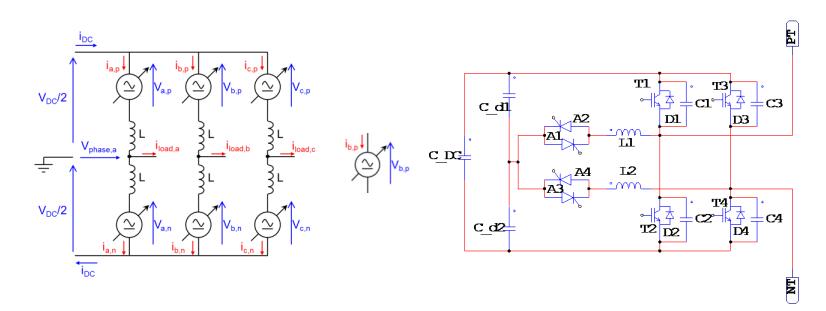
Another soft-switching topology that seemed to be worthy of consideration is the AQRDCL: it belongs to the resonant-link family instead to the resonant-pole one, but it is closely derived from the ARCP and it presents some similar modes of operation. Furthermore, this scheme shows a topological similarity with the Divan's ACRDCL ^[32], being also provided by a clamp switch, as shown in Figure 6.

Unlike the ARCP, where each converter arm requires a dedicated resonant circuit, (so two resonant circuits for a full H-bridge, and only one resonant circuit for a half H-bridge), the AQRDCL exploits only a single resonant circuit for the whole converter, acting on its DC-link. This means that, compared to the ARCP, this scheme needs less auxiliary components (active and passive), but at the price of an increased control complexity and somehow higher limitations.

The resonant circuit includes the auxiliary switches + diode pairs A₁ and A₂, the input capacitive divider with central tap C_c–C_dC and the passive resonant components L₁ and C₁. Please note that, differently from the ARCP, the snubber capacitors, in parallel to the main switches, here are not necessary. Nevertheless, a clamp switch (IGBT S_c and parallel diode D_c) here is included: substantially it disconnects the DC link from the source during the commutation intervals.

When the PWM modulation commands the commutation of the main switches T₁ ÷ T₄ of the H-bridge, the turn-off of the clamp switch S_c and the sequential turn-on of the auxiliary switches A₁–A₂, appropriately coordinated, occur. This triggers the resonance between L₁ and C₁: the effect is the zeroing, for a short interval, of the DC-link voltage, so the commutation (turn-off and turn-on) of the main switches occurs in ZVS. At the end of the process the resonant circuit is deactivated, the clamp switch S_c starts conducting and the DC-link voltage grows to its value. The auxiliary switches commutate in soft-switching zero-current mode, like in the ARCP. This topology exploits a full resonant cycle (a complete period), while the ARCP completes a soft-commutation in one-half period of resonance only. The clamp switch S_c introduces conduction losses because it conducts the load current, out of the commutation conditions. It commutates at the switching frequency, and its commutations should take place in ZVS both in turn-on and turn-off, like the main devices, but it is not yet assessed whether this is true in any operational situation.

Also the AQRDCL can operate in full PWM modulation, without limits. About the maximum power levels controllable with this topology, we haven't found reliable information; anyway, considering that a proper control requires that the gating of the power devices must occur respecting times of the order of a few µs, and which therefore requires the use of very fast power devices, it is safe to assume that the realistic level of maximum power for this topology is in the range of hundreds of kW.

Still wanting to express an aspect of comparison of this topology with the ARCP one, we can observe that, being there in this case only one resonant circuit for the whole converter, if the switching frequency is high and the modulation index is close to the unity, at equal operating conditions it is likely that it is necessary to employ a higher resonant frequency to ensure proper soft-switching in every situation. Also, for the same reason, with the AQRDCL topology overlap situations are frequent, events where a new resonant cycle (a new switching) is triggered before the previous one (the previous switching) is concluded. A correct control strategy must take into account these overlap situations, inserting an opportune delay if a commutation is commanded while another one is still in progress. This is a situation that however does not occur with the ARCP topology, which of course is more flexible due to resonance acting separately on each branch.

The analyzed MMC with the full H bridges soft-switching AQRDCL submodules is shown in Figure 7.

In Figure 8 some simulation diagrams referred to a single cell (one MMC submodule, full H-bridge) AQRDCL, are shown. Even with this topology, the ZVS turn-on and turn-off are observable, as well as the smooth edges of the output voltage.

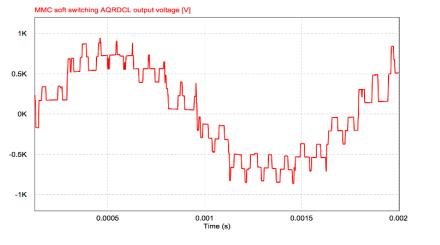
Figure 9 shows the simulation diagram of the output voltage of a 4-submodules for branch MMC, equipped with full H-bridges soft-switching AQRDCL submodules: also in this case, one can see a waveform substantially identical to that of a hard-switching MMC converter.

The authors aimed to quantify, at least in an indicative mode in this preliminary stage of the research, the potential advantages in term of losses and switching frequency, related to the use of these soft switching topologies compared to the conventional hard switching one, in view of the specific applications on high power and high voltage power converters.

Obviously, one must take into account that the resonant topologies involve a number of not negligible disadvantages, such as the increased complexity of the circuitry, a much greater number of components than a conventional hard-switching converter (therefore, in principle, a lower reliability), greater control limits and complexity, and an overall costs increase. It must be observed, in particular, that the higher number of components and related costs are not only referred to the auxiliary active and passive components included in the scheme, but also to the highest number of additional gate drivers, current/voltage transducers and heatsinks required and the increased sophistication of the cooling system. It is clear, therefore, that the innovative solution is actually befitting than the traditional to the extent that these limitations are compensated by much better performance in terms of efficiency.

By the use of PSIM software equipped with the Thermal Module, two full H-bridges, one of which hard switching and the other soft switching, with identical ratings and in the same operation conditions, have been put in comparison, especially looking to compare the losses—both the conduction and the switching ones—of the power semiconductor devices.

The voltage and power ratings of the H-bridges have been chosen coherently with the application of the H-bridge as a single submodule for a MMC intended for an application on Power Systems for a medium-high power level. So, the DC-link submodule voltage (that is, the voltage impressed by the energy storage capacitor of the single submodule) was fixed in 2500 V; for the AC-side current of the submodule (it would be the current of the MMC branch) a relatively restrained level, around 240 A, has been chosen. Taking into account the research results presented in ^[42] about a very high power MMC intended for STATCOMs and HVDCs, and equipped with ARCP resonant submodules employing large size IGCTs, here, considering a lower power level for the MMC, the authors have intended to examine the behaviour of the IGBTs as H-bridge power semiconductors (main devices).

The power devices were selected from those commercially available by known manufacturers; their electrical and thermal characteristics, deducted from the respective data-sheets, have been integrate in the Thermal Module of the PSIM software. Large power semiconductors are available in rather few sizes, so in some cases is evident a certain oversizing with respect to the voltage/current levels used in the performed tests. The switching frequency has been chosen f_sw = 6 kHz, a value unsustainably high for a hard switching converter and proposed to appreciate the advantages of the resonant topology, knowing that it can produce inacceptable high switching frequency losses ^[43] in hard switching architectures; tests were conducted with a medium value of the modulation index, ma = 0.65. The resonant frequency has been set 70 kHz; the fundamental output frequency is 50 Hz. It is worth to note how the modulation index is a low one for actual applications and this fact causes higher currents ^[44] and higher losses, nevertheless this value was chosen in order to compare the classical MMC and the resonant proposed MMC in the same non optimal point.

For the hard-switching model, considering the (main) devices to be IGBT modules ABB 5SNA 650J450300, 4500 V–650 A, the simulation yields the following losses for the four switches, IGBTs and diodes (average values in the simulation interval):

● overall conduction losses: 1070 W;

● overall switching losses: about 28.7 kW.

It is obvious that such a high value of the switching frequency f_sw causes a large amount of switching losses, completely incompatible with a correct operation of the converter.

Using IGBT modules Infineon FZ400R33KL2C-B5, 3300 V–400 A, 'low losses' class, a slightly better result has been obtained, but however switching losses are still too high:

● overall conduction losses: 1262 W;

● overall switching losses: about 22.1 kW.

As expected, to obtain an acceptable value of the switching losses it would be necessary to lower f_sw at or below 1 kHz (for f_sw = 1 kHz the switching losses would drop to 4.5 kW with ABB devices, and 3.2 kW with Infineon 'low losses' devices, and in this way their junction temperature T_j would be maintained well below the maximum limits).

In the same simulation conditions, the authors have subsequently tested an ARCP soft-switching submodule. Also for these tests the same devices of the hard-switching case have been considered. Therefore, using all ABB 4500 V–650 A modules (the same for both the main and the auxiliary switches), with f_sw = 6 kHz, the following results have been found:

● overall conduction losses on the main devices: 846 W;

● overall conduction losses on the auxiliary devices: 467 W;

● overall switching losses on the main devices: 262 W;

● overall switching losses on the auxiliary devices: 537 W.

Instead, using all Infineon 3300 V–400 A modules:

● overall conduction losses on the main devices: 1032 W;

● overall conduction losses on the auxiliary devices: 524 W;

● overall switching losses on the main devices: 450 W;

● overall switching losses on the auxiliary devices: 571 W.

It is noticeable how the switching losses on the main devices are greatly reduced, almost cancelled using the soft switching technology; the same losses on the auxiliary devices are very low, too, especially if compared to the ones related to the high switching frequency case. The turn-on and turn-off of the auxiliary devices really occur at zero-current, but this implies, anyway, a moderate amount of losses in these IGBT devices, due to the fact that turn-on and turn-off commutations do not occur completely in ZCS, because of the relatively high steep of the resonant current pulses. To maximize the circuit efficiency and therefore to obtain a perfect exploitation of the resonant effect with a maximum loss reduction, a careful tuning of the control functions that regulate the gating of the switching devices is mandatory. These simulations are based on a reasonable accurate gating; it is likely that even better results would be achieved with a more accurate one.

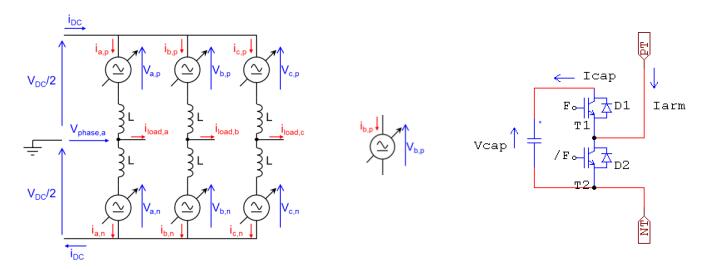
In addition, we can observe how the conduction paths in the auxiliary devices branch include the series connection of an IGBT and the antiparallel diode of the other IGBT, as shown in Figure 2, worsening the conduction losses. These losses do not vanish the efficiency gain of the soft-switching solution with respect to the hard switching one, but reduce its the advantage, because substantially a part of the losses on the main devices is 'transferred' to the auxiliary ones. This drawback can be overcome by using two antiparallel fast switching thyristors, or medium frequency thyristors, instead of the counter-phase series connection of the IGBT/diode pairs. This solution also has the virtue to simplify the auxiliary branch, because the two conduction paths include always one component only. Suitable components for this variant can be fast thyristors with ratings of the same class as those developed for induction furnaces and other medium frequency applications. Moreover, these components are quite inexpensive and can benefit from a very favourable design for a particular application, because they are very suitable to conduct current pulses with high peaks and short duration and low duty-cycle, like the resonant pseudo-sinusoidal current pulses in this application; so, it is possible to use devices with relatively low current rating. Then, in this soft-switching scheme, the auxiliary devices are loaded with one half the DC-link voltage only. Turn-off times t_q of some tens of microseconds appear adequate for these thyristors, compatible with the duty-cycle of the resonant current. The turn-on occurs by a gate pulse; the turn-off occurs when the resonant current falls below the holding value, usually very small. It is useful to keep in mind, however, that the correct choice of the thyristor ratings must be tuned with the design of adequate values for the resonant frequency and the resonant current peak.

About the size of the auxiliary devices, it can be placed in relief, at this point, given that the soft switching topologies require a number of active devices greater than the one needed by the conventional hard switching scheme, it makes sense to try to balance—at least partially—this unfavourable aspect by using a total silicon area slightly higher than that corresponding to the conventional solution: in other words, it is convenient to design the resonant circuit so that the auxiliary components are of the smallest size possible. Under this aspect, thyristors seems to be advantageous components, thanks to their ability to conduct high current peaks for a short time.

Currently few manufacturers produce medium frequency thyristors rated for a voltage of some kV and a current of a few hundreds A; a possible choice for the present study was the device Proton-Electrotex TFI643-500-22 rated for 2200 V–500 A. Another suitable components of the same manufacturer could have been the TFI233-400-24, 2400 V–400 A, (all disc-type devices) but the available data-sheets does not include all the necessary data for a complete modelling. Another device tested is the Poseico (Power Semiconductor Italian Corporation) ATF820 2000 V–725 A, superabundant, perhaps, about the current rating.

For sizing these auxiliary fast switching thyristors, it is important to observe that, although they conduct a current with a high peak value but with limited mean and RMS values, the high frequency operation seems to involve some derating with respect to the nominal current I_T(AV). This aspect needs further insights, as it impacts on the silicon area (then on the cost of the components), which should be limited as much as possible. In contrast, the voltage stress on these devices is limited only to half the DC-link voltage.

Another parameter which must be taken into account for the auxiliary thyristors is the turn-off time t_q, which must be adequately shorter than the period of the resonant current. Normally the medium frequency and fast switching thyristors feature t_q of some tens of microseconds, which allows a resonant frequency of some tens of kHz for the ARCP circuit. The AQRDCL topology, under this aspect, is most disadvantaged because it requires the auxiliary switches to operate with a higher frequency.

Anyway it would make sense if, in view of this soft-switching application, manufacturers develop a special devices family with suitably tuned ratings.

So, equipping the soft-switching ARCP submodule with Infineon 3300 V–400 A IGBTs power modules for the main switches, and the Proton-Electrotex 2200 V–500 A for the auxiliary switches, i.e., for analyzing the resonant MMC depicted in Figure 10, the following results have been obtained:

● overall conduction losses on the main devices: 1024 W;

● overall conduction losses on the auxiliary devices: 163 W;

● overall switching losses on the main devices: 375 W;

● overall switching losses on the auxiliary devices: 6.4 W.

That is a net improvement with respect to the use of the IGBTs for the auxiliary switches.

Again, using ABB 4500 V–650 A IGBTs for the main devices, and Poseico 2000 V–725 A thyristors for the auxiliary switches:

● overall conduction losses on the main devices: 1025 W;

● overall conduction losses on the auxiliary devices: 156 W;

● overall switching losses on the main devices: 374 W;

● overall switching losses on the auxiliary devices: 9.2 W.

A result only very similar to the previous one.

We can observe, in both cases, how the losses in the auxiliary devices—and particularly the switching ones—are much lower using thyristors instead of IGBTs.

Finally, the simulation results of the soft switching submodule AQRDCL, equipped with ABB 4500 V–650 A IGBTs power modules for the main switches, and the Proton-Electrotex 2200 V–500 A for the auxiliary switches, for analyzing the resonant MMC depicted in Figure 11, are reported as follows. The simulation is related to slightly different conditions from the previous ones: DC voltage 2000 V; load current about 100 A; resonant frequency about 70 kHz, switching frequency 6 kHz, output frequency 450 Hz; modulation index 0.65.

● overall conduction losses on the main devices: 143 W;

● overall conduction losses on the auxiliary devices: 155 W;

● overall conduction losses on the clamp device: 17 W;

● overall switching losses on the main devices: 335 W;

● overall switching losses on the auxiliary devices: 6.6 W;

● overall switching losses on the clamp device: 129 W.

Switching losses on thyristors used as auxiliary devices seem to be mainly due to the reverse recovery.

As already stated, these results are only orientative and are based on well-defined operating conditions and with certain components. Regarding the sizes selected for the devices, these were conditioned by the fact that the major manufacturers of large components produce only a few sizes of standardized ratings for voltage and current, in particular for the IGBTs single switch version. Nevertheless, these results suggest that the resonant soft switching solutions possess the potential to introduce significant improvements in the efficiency of the MMC, allowing the individual sub-modules to operate at high frequencies, unsustainable with common components in the usual hard-switching mode of operation. It must not be forgotten that, anyway, an extensive and reliable study should be based on a realistic application of the whole MMC converter, with well-established electrical quantities and operating conditions, taking into account the losses in the resonant inductor(s) as well as the effect of parasitic parameters, no longer negligible at high frequencies as the resonant one.

As a next step of this research it would be interesting to investigate regarding the benefits of the soft-switching technology using Silicon Carbide (SiC) devices, still under development, repeating the comparison between a hard-switching converter and a soft- switching one. At the present time, only devices with a modest voltage rating (1200 V max), are commercially available; anyway it seems likely the launching, in the short, of 3.3 kV rating devices.

3.   Conclusions

The authors have shown, how despite their age, soft-switching topologies like the ARCP and the AQRDCL still show interesting properties that suggest their application in modern high power/high voltage converters like the Modular Multilevel Converter.

In an effort to elevate as much as possible the conversion efficiency, particularly important aspect at the highest power levels, the use of the resonant topologies inside the MMC elementary converters (submodules) appear a very promising way. In this paper, the ARCP and the AQRDCL schemes have been briefly discussed and some preliminary simulation results of their comparison with the usual hard-switching solution have been shown: the advantages of these resonant topologies do emerge, capable of a net knocking-down of the switching losses inside the main semiconductor devices, thus allowing an overall increase in the submodule efficiency. In particular, two versions of resonant cells have been shown, the first version with IGBT as secondary switches and the second one with thyristors as secondary switches, showing how the latter is the more efficient, but has the problem that there are not many high frequency thyristors present in the market that can suit the resonant MMC; however this kind of converter can be an stimulus for silicon manufacturers to develop new high frequency thyristors.

The advantage of a resonant MMC increase while increasing f_sw, as the related losses are highly reduced. In HVDC applications, where f_sw is very low, the possible advantages are low, but in applications where f_sw is higher, i.e., where the number of cells is low, like in Medium Voltage applications or where the Medium Frequency Transformer is involved, advantages are higher, as the MMC is composed by full H bridges cells that need to be switched at medium frequency.

Most likely, even better results can be obtainable by the development of power semiconductors—particularly medium frequency thyristors—capable to optimize the operation and efficiency of the resonant tank.

As a further step, investigations will be done considering converters equipped with SiC devices.

Acknowledgements

Authors acknowledge financial support by the University of Genova under the project 2018-FRA.

Conflict of Interest

All authors declare no conflicts of interest in this paper.