Robust optimal excess-of-loss reinsurance and investment problem with p-thinning dependent risks under CEV model

1.   Introduction

Reinsurance and investment becomes more and more important in the insurance risk management. Recently, there has been a great deal of interest to investigate the optimal reinsurance and investment problems. For instance, Hipp and Plum (2000), Schmidli (2001) and Promislow and Young (2005) considered the optimal investment and/or reinsurance strategies for insurers to minimize the ruin probability; Irgens and Paulsen (2004), Yang and Zhang (2005), Liang et al. (2011), Guan and Liang (2014), Zhu (2015), Pan et al. (2019) and Zhang and Zhao (2020) studied the optimal problems under the criterion of maximize the survival probability or the expected utility of the insurer's terminal wealth; Bai and Zhang (2008), Zeng et al. (2013), Li et al. (2015), Zeng et al. (2016), Liang et al. (2016) and Tian et al. (2020) investigated the optimal reinsurance and investment problems under mean-variance criterion.

However, most of the above-mentioned literatures usually assume that there is only one class of insurance business in the insurer's wealth process. In reality, there usually are more than one classes of insurance businesses in the operation and management of an insurer, i.e., car claims and medical claims. Moreover, different insurance businesses are usually correlated through some way. A typical example is that a traffic accident (or fire accidents or earthquakes or aviation accidents and so on) may cause medical claims or property loss or death claims. Therefore, it is necessary to investigate the dependent risks in the actuarial literature. Liang and Yuen (2016) and Yuen et al. (2015) began to investigate optimal proportional reinsurance problems with dependent risks, they assumed that there are two or more dependent classes of insurance business in the insurer's wealth process and claim number processes are correlated through a common shock component, and their aims were to maximize the expected utility of the insurer's terminal wealth and derived the optimal proportional strategies. Then Bi et al. (2016) studied the optimal proportional investment and reinsurance problems under mean-variance criterion for the risk model with common shock dependence. Later, Zhang and Zhao (2020) considered the optimal proportional reinsurance and investment problem with p-thinning dependent risks. For other research about dependent risks, one can refer to Yuen et al. (2002), Gong et al. (2012), Liang and Wang (2012), Liang et al. (2016) and the references therein.

Moreover, ambiguity is still being worthy of further exploration. In practice, model uncertainties do exist widely in finance, especially in insurance and portfolio selection. In the recent years, optimal investment-reinsurance problems with ambiguity have been paid more attention. For example, Yi et al. (2013) and Zheng et al. (2016) optimized proportional reinsurance and investment problems with model uncertainty for an insurer. Huang et al. (2017) considered a robust optimal proportional reinsurance and investment problems for both an insurer and a reinsurer. Yang et al. (2017) took multiple dependent classes of insurance business into account and studied the optimal mean-variance proportional reinsurance-investment problem with delay. Zeng et al. (2016) analyzed a robust optimal proportional reinsurance-investment problem under the mean-variance criterion for an ambiguity-averse insurer (AAI) who worries about model uncertainty. Besides, excess-of-loss reinsurance is also more important, as shown in Asmussen et al. (2000), it is even better than the proportional reinsurance in most situations. Later, A and Li (2015), Li et al. (2017), A et al. (2018) and Zhang and Zhao (2019) analyzed robust optimal excess-of-loss reinsurance and investment problems for an AAI.

To the best of our knowledge, few researches focus on the robust optimal excess-of-loss reinsurance and investment problem with p-thinning dependent risks. Inspired by Zhang and Zhao (2019), this paper not only takes excess-of-loss reinsurance into account, but also considering the model uncertainly. Suppose that there are two p-dependent classes of insurance business in the insurer's wealth process. The insurer can purchase excess-of-loss reinsurance and invest in a risk-free asset and a risky asset, where the price process of risky asset follows CEV model. Firstly, a robust optimal control problem with p-thinning dependent risks is formulated. Secondly, the robust optimal excess-of-loss reinsurance and investment strategies are derived under the criterion of maximizing the expected exponential utility of AAI's terminal wealth. This paper has three following high contributions: (ⅰ) both p-thinning dependent risks and excess-of-loss reinsurance are considered in an optimal problem; (ⅱ) ambiguity is taken into account in this problem; (ⅲ) some special cases such as the case of investment-only and ambiguity-neutral insurer are given, which means that our results generalized some existing results, e.g., Gu et al. (2012), A et al. (2015), A et al. (2018) and Zhang and Zhao (2020).

The rest of this paper is organized as follows. Formulation of our model is presented in section 2. Section 3 derives robust optimal strategies by maximizing the utility of AAI's terminal wealth. We give the verification theorem in Section 4. Section 5 provides some special cases of our model. In Section 6, some numerical simulations are presented to illustrate our results. Section 7 concludes the paper.

2.   Model formulation

Let ($\mathit \Omega, \mathscr{F}, P$) be a complete probability space with filtration${\rm{\{ }}{\mathscr{F}_t}{\rm{, }}t \in {\rm{[}}0, T{\rm{]\}, }}$where ${\mathscr{F}_t}$ stands for the information of the market available up to time t and$T$is a positive finite constant which represents the terminal time. All processes introduced below are assumed be well-defined and adapted processes in this space. Assume that trading takes place continuously, without taxes or transaction costs, and that all securities are infinitely divisible.

2.1.   Wealth process

The insurer's wealth process ${\rm{\{ }}R(t), t \in [0, T]{\rm{\} }}$ with two dependent classes of insurance business is described by

where ${x_0} \geqslant 0$ is the initial surplus, $c > 0$ represents the premium rate; ${X_i}$ is the ith claim size from the first class of business; ${\rm{\{ }}{X_i}, i \geqslant 1{\rm{\} }}$ are assumed to be i.i.d. positive random variables with common distribution function denotes ${F_X}(\cdot).$ Denote finite first moment $E[{X_i}] = {\mu _X} > 0$ and second moment $E[X_i^2] = \sigma _X^{\rm{2}}$; ${Y_i}$ is the ith claim size from the second class of business and ${\rm{\{ }}{Y_i}, i \geqslant 1{\rm{\} }}$ are assumed to be i.i.d. positive random variables with common distribution function ${F_Y}(\cdot)$. Denote finite first moment $E[{Y_i}] = {\mu _Y} > 0$ and second moment denote $E[Y_i^2] = \sigma _Y^2$. The claim number process $\left\{ {N(t), t \geqslant 0} \right\}$ is assumed to be a Poisson process with intensity $\lambda > 0$ representing the number of claims occurring in time interval $[0, t],$ while $\left\{ {{N^p}(t), t \geqslant 0} \right\}$ is a p-thinning process of $\left\{ {N(t), t \geqslant 0} \right\}$ which is another Poisson process with intensity $p\lambda.$ As we know, each claim ${X_i}$ in reality may or may not cause another claim ${Y_i}$, if yes, we assume that the claim ${Y_i}$ is caused with probability p. This paper assumes that the events whether each claim ${X_i}$ causes another claim ${Y_i}$ or not are mutually independent. Thus, the claim number process ${N^p}(t)$ for the claims ${Y_i}$ is a p-thinning process of $N(t)$ for claims ${X_i}$. The compound Poisson process $\sum\nolimits_{i = 1}^{N(t)} {{X_i}}$ and $\sum\nolimits_{i = 1}^{{N^p}(t)} {{Y_i}}$ are the cumulative amount of claims ${X_i}$ and ${Y_i}$ in time interval $[0, t],$ respectively. Moreover, we assume that $\left\{ {{X_i}, i \geqslant 1} \right\}, \left\{ {{Y_i}, i \geqslant 1} \right\}$ and $\left\{ {N(t), t \geqslant 0} \right\}$ are mutually independent.

As we all know, a serious epidemic like Covid-19 pandemic has been caused medical claims or death claims. The thinning-dependent structure in (1) can be interpreted as that medical insurance claims (i.e., ${X_i}$) causes death insurance claims (i.e., ${Y_i}$) with probability p due to Covid-19 pandemic, then the death claim number process (i.e.${N^p}(t)$) is a p-thinning process of the claim number process of medical insurance (i.e.$N(t)$).

In addition, the insurer's premium rate is calculated according to the expected value principle, i.e.

where ${\eta _i} > 0$ is the insurer's safety loading of the ith class of insurance business.

Denote by ${D_1} = \sup \{ x:{F_X}(x) \leqslant 1\} < + \infty,$ then ${F_X}(0) = 0, 0 < {F_X}(x) < 1$ for $0 < x < {D_1}$ and ${F_X}(x) = 1$ for $x \geqslant {D_1};$ denote by ${D_2} = \sup \{ y:{F_Y}(y) \leqslant 1\} < + \infty,$ then ${F_Y}(0) = 0, 0 < {F_Y}(y) < 1$ for $0 < y < {D_2},$ and ${F_Y}(y) = 1$ for $y \geqslant {D_2}.$

2.2.   Excess-of-loss reinsurance

Assume that the insurer is allowed to purchase excess-of-loss reinsurance in order to reduce the underlying claims risk. Let ${m_i}(i = 1, 2)$ be (fixed) excess-of-loss retention levels, and let

be the parts of the first claims and the second claims held by the insurer, respectively. Then by (1), the wealth process $\{ {X^m}(t), t \in [0, T]\}$ after considering reinsurance with retention levels $m = ({m_1}, {m_2})$

becomes

with the premium rate

where ${\xi _i}$ is the reinsurer's safety loading of the ith class of insurance business. This paper assumes ${\xi _i} > {\eta _i}$ which represents that the reinsurance is not cheap.

According to Grandell (1991) and Promislow and Young (2005), the claim process ${\rm{d}}\sum\limits_{i = 1}^{N(t)} {\bar X_i^{{m_1}}}$ can be approximated by a diffusion risk model as follows

similarly,

Then the wealth process (2) can be approximated by the following diffusion model

where

${W_X}(t)$ and ${W_Y}(t)$ are two standard Brownian motions, and their correlation coefficient is given by

and ${W_0}(t)$ is another standard Brownian motion which is dependent of ${W_X}(t)$ and ${W_Y}(t)$.

Remark 1. If $p = 0$, the model (1) will reduce to the classical C-L model in Zhang et al. (2020) and (3) will reduce to that in A and Li (2015), A et al. (2018) and Li et al. (2016), which implies that our model can generalize the optimal formulation of existing results to the case with p-thinning dependent risks.

For convenience, let

where ${\bar F_X}(x) = 1 - {F_X}(x), {\bar F_Y}(y) = 1 - {F_Y}(y).$

2.3.   Financial market

Moreover, the insurer is allowed to invest in one risk-free asset (bound) and one risky asset (stock). The price process $B(t)$ of the risk-free asset follows

and the price process $S(t)$ of risky asset is given by the following CEV model

where ${r_0}\; (>0)$ is the interest rate of the bond, $\mu \; (>{r_0})$ represents the expected instantaneous rate of the risky asset, $\sigma (>{\rm{0}})$ is the volatility of the risky asset price and $\beta \, (\geqslant {\rm{0)}}$ is the elasticity parameter, respectively.$\left\{ {{W_{\rm{1}}}(t), t \geqslant 0} \right\}$ is a standard ${F_t}$-adapted Brownian motion, independent of $\left\{ {{X_i}, i \geqslant 1} \right\}, \left\{ {{Y_i}, i \geqslant 1} \right\}$ and $\left\{ {N(t), t \geqslant 0} \right\}.$

Remark 2. If $\beta = {\rm{0}}$ in (7), the CEV model will reduce to the GBM model.

2.4.   Optimal problem for an AAI

Let $u: = \{ u(t): = {m_1}(t), {m_2}(t), \pi (t), t \in [0, T]\}$ be the reinsurance-investment strategy, where ${m_i}(t)$ is the excess-of-loss retention level for ith claim at time t, note that ${m_i}(t) = {D_i}$ represents "no reinsurance", ${m_i}(t) = 0$ represents "full reinsurance", and $\pi (t)$ represents the money amount invested in the risky asset at time t, so the amount of money invested in the risk-free asset at time t is ${X^u}(t) - \pi (t),$ here ${X^u}(t)$ represents the wealth of the insurer after adopting strategy $u.$ Therefore, the evolution of ${X^u}(t)$ is governed by

with ${X^u}(0) = {x_{\rm{0}}}.$

For notational convenience, we write ${m_i}(t)$ and $\pi (t)$ as ${m_i}$ and $\pi$ in below.

In traditional, the insurer is assumed to be ambiguity-neutral with the objective function as follows

where ${E_{t, {\kern 1pt} x, {\kern 1pt} s}}(\cdot)$ denotes the expectation under P considering the dynamics of the process $({{\rm{X}}^u}, S)$ with initial condition $(t, x, s) \in [0, T] \times R \times {R^ + },$ and ${\rm{\tilde \Pi }}$ is the set of admissible strategies given by Definition 2.1.

However, most of insurers are ambiguity-averse and they always try to be against worse-case scenarios. Thus, it is reasonable to consider an ambiguity-averse insurer (AAI) in the field of insurance. In what follows, a robust portfolio choice with uncertainty will be presented for an AAI. Assume that there is a relatively good estimated model (also called reference model) for the AAI to describe the risky assets prices and claim process, but the AAI is always skeptical about the chosen reference model and always hopes to take alternative models into consideration. According to Anderson (1999), the alternative models can be defined by the following set of probability measures $\mathcal{Q}$ which are equivalent to the P:

Definition 2.1. The strategy is said to be admissible if it is $\mathscr{F}_{t}$-progressively measurable and satisfies

(ⅰ) ${m_i}(t) \in [0, {D_i}], \forall t \in [0, T], i = {\rm{1, }}\, {\rm{2}};$

(ⅱ) ${E^{{Q^*}}}\left({\int_0^T {{{\left\| {u(w)} \right\|}^2}{\rm{d}}w} } \right) < \infty,$ and ${E^{{Q^*}}}\left({\int_0^T {{\pi ^2}(w){S^{2\beta }}(w){\rm{d}}w} } \right) < \infty,$ where ${\left\| {u(t)} \right\|^2} = m_1^2(t) + m_2^2(t) + {\pi ^2}(t);$

(ⅲ) $\forall (t, x, s) \in [0, T] \times R \times {R^ + },$ the SDDE (8) has a pathwise unique solution $\{ {X^u}(t), t \in [{\rm{0}}, T]\}$ with $E_{t, x, s}^{{Q^*}}[U({X^u}(T))] < \infty,$ where ${Q^*}$ is the chosen model to describe the worst case, ${E_{t, x, s}}[ \cdot ]$ is the condition expectation given ${X^u}(t) = x \in R, S(t) = s \in {R^ + }.$ Let ${\rm{\Pi }}$ be the set of all admissible strategies.

Next, define a process $\{ \theta (t) = ({\theta _{\rm{0}}}(t), {\theta _{\rm{1}}}(t))|t \in {\rm{[0}}, T]\}$ satisfying that

(ⅰ) $\theta (t)$ is $\mathscr{F}_{t}$-measurable, $\forall t \in {\rm{[0}}, T];$

(ⅱ) $E[{\rm{exp}}\{ \tfrac{1}{2}\int_0^T {{{\left\| {\theta (t)} \right\|}^2}{\rm{d}}t} \} ] < \infty,$ where ${\left\| {\theta (t)} \right\|^2} = \theta _0^2(t) + \theta _1^2(t).$ We denote $\mathit \Theta$ for the space of all such processes $\theta.$

For $\forall \theta \in \mathit \Theta,$ we define a real-valued process $\left\{ {{\mathit \Lambda ^\theta }(t)|t \in {\rm{[0}}, T]} \right\}$ on ($\mathit \Omega$, F, P) by

where $W{\rm{(}}t) = ({W_{\rm{0}}}{\rm{(}}t), {W_{\rm{1}}}{\rm{(}}t))'.$ By Ito's differentiation rule,

So, we know that ${\mathit \Lambda ^\theta }(t)$ is a P-martingale and $E[{\mathit \Lambda ^\theta }(t)] = {\rm{1}}.$ For each $\theta \in \mathit \Theta,$ define a new real-world probability measure Q absolutely continuous to P on ${F_T}$ as follows

Now, a family of real-world probability measures Q parameterized by $\theta \in \mathit \Theta$ have been constructed. Applying Girsanov's theorem, we know that ${W^Q}{\rm{(}}t) = (W_{\rm{0}}^Q{\rm{(}}t), W_{\rm{1}}^Q{\rm{(}}t))'$ with ${\rm{d}}{W^Q}{\rm{(}}t) = {\rm{d}}W{\rm{(}}t) + \theta {\rm{(}}t)'dt$ under Q is a standard two-dimension Brownian motion.

Noting that the alternative models in class $\mathcal{Q}$ are different due to the drift terms. Thus, the risky asset's price (7) under Q is

and the wealth process (8) under Q can be rewritten as

by recalling that $\pi = \pi (t), {m_i} = {m_i}(t), {c_i} = {c_i}({m_i}(t)), i = 1, 2, \gamma _{\rm{1}}^{\rm{2}} = \lambda {G_X}({m_1}(t))$ and $\gamma _{\rm{2}}^{\rm{2}} = \lambda p{G_Y}({m_2}(t)).$

Suppose that the insurer tries to seek a robust optimal control which is the best choice in some worst-case models. Inspired by Maenhout (2004) and Branger and Larsen (2013), the robust control problem to modify problem (9) can be formulated as follows

where

and $E_{t, x, s}^Q$ is calculated under Q; $\phi$ is a strictly positive deterministic function and stands for the strength of the preference for robustness; the deviation from the reference model is penalized by the second term in the expectation, which depends on the relative entropy arising from the diffusion risks.

To solve (12), we define the optimal value function $J(t, x, s)$ as

For convenience, some notations are first provided. Let ${\mathcal{O}_0} \subset R \times {R^ + }$ be an open set and $\mathcal{O} = [0, T] \times {\mathcal{O}_0}.$ Denoted by ${C^{{\rm{1, 2, 2}}}}$ the space of $J(t, x, s)$ such that $J$ and its partial derivatives ${J_t}, {J_x}, {J_s}, {J_{xx}}, {J_{xs}}, {J_{ss}}$ are continuous on $O.$ To solve the problem (14), applying dynamic programming principle, we can derive the following robust Hamilton-Jacobi-Bellman (HJB) equation (see Kraft(2005) and Maenhout(2006)):

with boundary condition

where ${\mathcal{A}^{\theta, u}}$ is the generator of (14) under Q given by

Note that $J$ is a short notation for $J(t, x, s).$

3.   Robust optimal results

This section is devoted to derive the robust optimal strategy ${u^*}$ under the worst-case scenario. Suppose that the AAI has the exponential utility function given by as follows

where the constant v (>0) represents the absolute risk aversion coeffcient. As we all know, the exponential utility function plays an important role in insurance mathematics and actuarial practice. It is independent of the level of insurers' wealth and it is the only utility function under the principle of "zero utility" giving a fair premium (see Gerber, 1979).

In what follows, we set out to solve the HJB equation (15). At first, we show the form of $\phi$. For analytical tractability, following Maenhout(2004, 2006), we set

which is state-dependent, where $m\; (\geqslant 0)$ represents the ambiguity-aversion coefficient, which describes the AAI's attitude to the diffusion risk.

Then, to solve (15) preference parameter $\phi$, we conjecture the form of the value function as follows

with $G(T, s) = {\rm{0}}.$ Let ${G_t}, {G_s}, {G_{ss}}$ be the partial derivatives of $G(t, s).$ According to (19), we have

Step1: Substituting (20) into (15) and rearranging terms, since we get

Step2: According to (21), fixing and maximizing over, we derive the first-order condition for the following minimum point :

Observe that $\theta _0^*$ is a function on time and the retention levels $({m_1}, {m_2})$, while $\theta _1^*$ is a function on the investment quote $\pi$ and (t, s). Replacing (22) back into (21) leads to

where

Step3: According to the first-order condition for yields

Observe that ${\pi ^*}$ is time and state-dependent since it is a function on (t, s). Plugging (25) into (23) derives,

For fixed $t \in [0, T],$ let

To find the minimizer $m_1^*(t)$ and $m_2^*(t)$ of $f,$ we assume that ${F_X}(x)$ and ${F_Y}(y)$ are continuous and differentiable, and ${F'_X}(x) = {f_X}(x), {F'_Y}(y) = {f_Y}(y).$ According to (27) and recalling that $\gamma _{\rm{1}}^{\rm{2}} = \lambda {G_X}({m_1}(t))$ and $\gamma _{\rm{2}}^{\rm{2}} = \lambda p{G_Y}({m_2}(t)),$ for any $t \in [0, T],$ differentiating $f$ with respect to ${m_1}, {m_2}$ yields

We first consider that $m_i^*(t) \in [0, {D_i}),$ then $0 < {\bar F_X}(m_1^*(t)) \leqslant 1$ and $0 < {\bar F_Y}(m_2^*(t)) \leqslant 1.$ Suppose that there exists at least one point $(m_1^*, m_2^*)$ satisfying the following equation

Taking $(m_1^*, m_2^*)$ into the second derivatives of $f$ arriving at the following Hessian matrix

then we know that this Hessian matrix is positive definite at the point $(m_1^*, m_2^*).$ Therefore, if the point $(m_1^*, m_2^*)$ is found such that (28) holds, then the point $(m_1^*, m_2^*)$ is indeed the minimizer of $f.$

In order to determine the point $(m_1^*, m_2^*)$ clearly, we transform (28) into

or equivalently,

Define three following auxiliary functions

For convenience, we assume that ${\xi _1} \geqslant {\xi _2}.$ It's easy to verify that both ${l_Y}(x)$ and $k(x)$ are strictly increasing functions for $x \geqslant 0,$ so their inverse functions $l_Y^{ - 1}(x)$ and ${k^{ - 1}}(x)$ exist. From (29), we get ${l_X}({m_1}) = {l_Y}({m_2}),$ then

Similarly, if ${\xi _1} \leqslant {\xi _2},$ we can easily verify that both ${l_X}(x)$ and $k(x)$ are strictly increasing functions for $x \geqslant 0,$ so their inverse functions $l_X^{ - 1}(x)$ and ${k^{ - 1}}(x)$ exist. Then

Taking (31) for example, and inserting (31) into the second equation of (28) yields

Let

If $h(x) = 0$ has a solution on $[0, {D_1}],$ the solution will be indeed $m_1^*$ we try to derive, as a result, we will easily determine the value of $m_2^*$. Thus, the minimizer of $f({m_1}, {m_2}, t)$ in (27) are derived, which is the candidate robust optimal excess-of-loss reinsurance strategy for the optimal control problem (14). These results are summarized in the following theorem.

Theorem 3.1. Assume that and let

The candidate robust optimal excess-of-loss reinsurance strategy $(m_1^*(t), m_2^*(t))$ of the optimal control problem (14) is given as follows.

(ⅰ) If $\frac{{{\xi _1}}}{{(m + v){a_l}}} \geqslant 1$ or ${a_l} = 0,$ we have ${t_0} \leqslant T.$ For ${t_0} \leqslant t \leqslant T,$ we have $(m_1^*(t), m_2^*(t)) = (\hat m_1^*(t), \hat m_2^*(t)),$ where

and ${x_0}(t) \in [{a_l}, {D_1}]$ is the unique solution to $h(x) = 0$ if $h({D_1}) \geqslant 0$ holds.

For $0 \leqslant t < {t_0},$ if the solution to $h(x) = 0$ exists, we have $(m_1^*(t), m_2^*(t)) = (\tilde m_1^*(t), \tilde m_2^*(t)),$ where

(ⅱ) If $\frac{{{\xi _1}}}{{(m + v){a_l}}} < 1,$ we have ${t_0} > T.$ For $0 \leqslant t \leqslant T < {t_0},$ we have $(m_1^*(t), m_2^*(t)) = (\tilde m_1^*(t), \tilde m_2^*(t)).$

Proof. (ⅰ) If $a_{l} = 0$ or $\frac{\xi_{1}}{(m+v) a_{l}} \geq 1$, then we have $t_{0} \leq T$.

For ${t_0} \leqslant t \leqslant T,$ we have $0 < {a_l} \leqslant {a_k}.$ Since ${l_Y}(x)$ and $k(x)$ are increasing functions on $[{a_l}, + \infty),$ it's easy to verify that $h(x)$ increases on $[{a_l}, + \infty).$ What's more, we can get $h({a_l}) = k({a_l}) \leqslant k({a_k}) = 0$ by the fact that ${l_X}({a_l}) = 0.$

If $h({D_1}) \geqslant 0,$ the equation $h(x) = 0$ admits a unique solution ${x_0}(t) \in [{a_l}, {D_1}],$ thus the robust optimal excess-of-loss strategy is

Since ${m_2}(t) \leqslant {D_1},$ we get

If $h({D_1}) < 0,$ we know that the equation $h(x) = 0$ has a unique solution on $({D_1}, + \infty).$ Due to ${m_1}(t) \leqslant {D_1},$ we choose $m_1^*(t) = {D_1}.$ At this time, substituting $m_1^*(t) = {D_1}$ back into $f({m_1}, {m_2}, t)$ in (27), and taking the first derivative of $f({D_1}, {m_2}, t)$ with respect to ${m_2}$, the minimizer of $f({D_1}, {m_2}, t)$ is derived as follows

Consequently, from (36), (37) and (38), $m_1^*(t)$ and $m_2^*(t)$ can be expressed as $\hat m_1^*(t)$ and $\hat m_2^*(t)$ in (35) and (36), respectively.

For $0 \leqslant t < {t_0},$ we know ${a_l} > {a_k}$ holds, then $h({a_l}) = k({a_l}) > k({a_k}) = 0.$ Meanwhile, $h(x)$ is a strictly increasing function on $[{a_l}, + \infty)$ and $h(x) > 0, x \in [{a_l}, + \infty).$ As a result, the equation $h(x) = 0$ has no solution on $[{a_l}, + \infty).$ In other words, there does not exist the solution $(m_1^*, m_2^*)$ satisfying (28) when ${m_1} \in ({a_l}, + \infty)$ and ${m_2} \in [0, + \infty).$ However, it doesn't mean that (28) has no solution on ${m_1} \in [0, {a_l})$ and ${m_2} \in [0, + \infty).$ It is not difficult to prove that ${l_X}(x)$ is a convex function on $[0, {a_l}).$ So for $0 \leqslant t < {t_0},$ if the solution of the equation $h(x) = 0$ exists, it will only be obtained on $x \in [0, {a_l})$ which is indeed $m_1^*(t)$ we try to derive.

Because ${l_X}(0) = {l_X}({a_l}) = 0,$ and ${l_X}(x)$ is a convex function on $[0, {a_l}),$ we can derive that ${l_X}({m_1}) \leqslant 0$ on ${m_1} \in [0, {a_l}).$ What's more, $l_Y^{ - 1}(x)$ is a strictly increasing function and $l_Y^{ - 1}(0) = 0,$ so we can obtain from (31) that ${m_2} = l_Y^{ - 1}({l_X}({m_1})) \leqslant 0.$ Due to ${m_2} \geqslant 0,$ we get $m_2^*(t) = 0.$ Plugging $m_2^*(t) = 0$ into $f({m_1}, {m_2}, t)$ in (27) and taking the first derivative of $f({m_1}, 0, t)$ with respect to ${m_1}$, we can derive the following minimizer of $f({m_1}, 0, t)$

To sum up, for the case of $0 \leqslant t < {t_0},$ we derive (36).

(ⅱ) If $\frac{{{\xi _1}}}{{(m + v){a_l}}} < 1,$ we have ${t_0} > T.$ Thus for $0 \leqslant t \leqslant T < {t_0},$ the inequality ${a_l} \geqslant {a_k}$ holds. The optimal problem is similar to that of $0 \leqslant t < {t_0}$ in case (i). At this time, we can obtain the candidate robust optimal excess-of-loss reinsurance strategy for $0 \leqslant t \leqslant T$ is (36). This ends the proof of Theorem 3.1.

In order to give the expressions of ${\pi ^*}$ and the value function $J(t, x, s),$ we first have to derive the expression of $G(t, s)$ in (25). According to (27), we can rewrite (26) as

Now this problem should be discussed in two cases as follows:

Case Ⅰ: If for the candidate optimal reinsurance strategy of the problem (14) is (35). Denote by the function in (40), we have

In what follows, we employ power transformation technique along with variable change method to solve the problem. Let

with ${K_1}(T) = 0, {L_1}(T) = 0.$ Then substituting (42) into (41) leads to

By matching coefficients, we derive

and we have the solution of (43)

Similarly, for $0 \leqslant t < {t_0},$ the candidate optimal excess-of-loss strategy is (36). Denote the function $G$ by ${G_2}$ in (40), and let

Similar to (43)–(45), we obtain

and

Hence for $\frac{{{\xi _1}}}{{(m + v){a_l}}} \geqslant 1,$ we have

Since $J(t, x, s)$ is continuous at $t = {t_{\rm{0}}}$, we have

and derive

Based on (46)–(49), it is not difficult to find that ${L_{\rm{1}}}(t) = {L_{\rm{2}}}(t).$ Since ${K_{\rm{1}}}({t_{\rm{0}}}) = {K_{\rm{2}}}({t_{\rm{0}}}),$ we get

As a result, we can rewrite (48) as

By (25), (42) and (44), it can easily be seen that the candidate optimal investment strategy ${\pi ^*}$ for ${\rm{0}} \leqslant t < {t_{\rm{0}}}$ is the same as that for ${t_{\rm{0}}} \leqslant t \leqslant T,$ which is

Case Ⅱ: If we have For the candidate optimal excess-of-loss strategy is (36). Denote by the function in (40), we have

with boundary condition ${G_{\rm{3}}}(T, s) = {\rm{0}}.$ Similar to the analysis for ${t_0} \leqslant t \leqslant T$ in case I, we conjecture a solution to (54) of the following form

with ${K_3}(T) = 0, {L_3}(T) = 0.$ Then by the same method as that in Case I and a direct calculation, we have ${L_3}(t) = {L_1}(t),$ and

Therefore, the expression of the candidate optimal investment strategy for $0 \leqslant t \leqslant T$ can be derived, which is the same as (53) and the corresponding candidate value function can also be obtained.

We summarize the above analysis in following theorem.

Theorem3.2. Recall functions defined in (42), (46) and (55), respectively. For the problem (14), the candidate robust optimal investment strategy is given by

and (ⅰ) if $\frac{{{\xi _1}}}{{(m + v){a_l}}} \geqslant 1$ holds, the candidate optimal value function is

(ⅱ) if $\frac{{{\xi _1}}}{{(m + v){a_l}}} < 1$ holds, the candidate optimal value function is

Remark 3. According to Theorem 3.1 and Theorem 3.2, we can see that

(1) the candidate robust optimal reinsurance strategy in Theorem 3.1 is similar to Theorem 13 in Zhang and Zhao (2019). Moreover, if $p = 0,$ we have ${\eta _2} = {\xi _2} = 0,$ then Theorem 3.1 will coincide with Corollary 3.6 in A and Li (2015) and A et al. (2018).

(2) if $m = {\rm{0}},$ the candidate optimal investment strategy in Theorem 3.2 will be the same as Theorem 3.1 in Gu et al. (2012) and Theorem 3.10 in Zhang and Zhao (2020). This implies that our results generalize the existing results to the case of dependent risks and ambiguity.

(3) the insurer's wealth has no influence on the optimal strategies due to the exponential utility function.

4.   Verification theorem

This section will apply the result of Kraft (2004, Corollary 1.2) to verify the candidate optimal strategies ${m_i} = m_i^*(t), {\theta _i} = \theta _i^*, i = 1, 2$ and $\pi = {\pi ^*}(t)$ are indeed optimal, and the value function given by (20) is just the value function $J(t, x, s)$ defined by (14). The main theorem is summarized as follows.

Theorem 4.1. For the optimal control problem (14), if there exists a function and a measurable function which satisfy the HJB Equation (15) and the parameters satisfy

then $({u^*}(t) = \hat u(t, {S_t}), {\theta ^*}(t) = \hat \theta (t, {S_t})),$ is an optimal strategy and $V(t, x, s)$ is the corresponding value function.

Proof. From Kraft (2004, Corollary 1.2), the above theorem will hold if $({u^*}, {\theta ^*})$ and the corresponding candidate value function $V(t, x, s)$ have the following three properties:

(1) ${u^*}$ is an admissible strategy and ${Q^*}$ is well-defined by ${\mathit \Lambda ^{\theta *}}(t)$ with $\theta _0^*$ and $\theta _1^*$;

(2) ${E^{{Q^*}}}\left[ {\mathop {\sup }\limits_{t \in [0, T]} {{\left| {V(t, {X^{{u^*}}}(t), S(t))} \right|}^4}} \right] < \infty$;

(3) ${E^{{Q^*}}}\left[ {\mathop {\sup }\limits_{t \in [0, T]} {{\left| {{\rm{\Psi }}(t, {X^{{u^*}}}(t), S(t), {\theta ^*}(t))} \right|}^2}} \right] < \infty.$

Next, we shall verify the properties (1)–(3), respectively.

Proof of (1). $m_1^*(t)$ and $m_2^*(t)$ are deterministic and bounded on $[0, T]$, thus condition (i) in Definition 2.1 is met. By Itô's lemma and (7), we have

According to Zhao et al. (2017) and Jeanblanc et al. (2009), we know that (59) has a unique strong solution. Therefore, for $\beta \geqslant {\rm{0, }}$ (7) has a unique solution such that $S(t) \in ({\rm{0}}, + \infty).$ Moreover, by (57), condition (ⅱ) in Definition 2.1 is met. Condition (ⅲ) in Definition 2.1 can be obtained by Property (2).

Proof of (2). Substituting $({u^*}, {\theta ^*})$ into (11), we have the wealth process under $({u^*}, {\theta ^*})$

where $B(t) = \frac{{{{(\mu - r)}^2}(1 - {e^{ - 2r\beta (T - t)}})}}{{4r\beta {\sigma ^2}(m + v)}}, {B_1}(t) = \frac{{\mu - r}}{{{\sigma ^2}(m + v)}} + 2\beta B(t).$

Inserting (60) into (19), we obtain the following estimate with appropriate constants ${M_1} < {M_2},$

where

and

Because ${L_1}(t)$ and $K(t)$ are deterministic and bounded on $[0, T]$ and $S(t) \in ({\rm{0}}, + \infty),$ we obtain that the first estimate in (61) is valid. Since $m_{\rm{1}}^*{\rm{(}}t{\rm{), }}\, m_{\rm{2}}^*{\rm{(}}t{\rm{), }}\theta _{\rm{0}}^*{\rm{(}}t{\rm{)}}$ and ${e^{r(T - t)}}$ are deterministic and bounded on $[0, T],$ the second inequality holds.

In what follows, we consider the four integrals about $\exp \{ {E_i}(t)\}, i = 1, 2, 3, 4.$

Firstly, note that $m_{\rm{1}}^*{\rm{(}}t{\rm{)}}$ and $m_{\rm{2}}^*{\rm{(}}t{\rm{)}}$ are bounded on $[0, T],$ it easy to check that

Secondly, because ${B_1}(t)$ is deterministic and bounded on $[0, T],$ then by the Lemma 4.3 in Zeng and Taksar (2013), it is easily be seen that $\exp \{ 4{E_1}(t)\}$ and $\exp \{ 4{E_3}(t)\}$ are martingales under ${Q^*},$ consequently,

Thirdly, according to Theorem5.1 in Zeng and Taksar (2013), we obtain a sufficient condition for

is

for $\forall w \in [0, T].$

Note that $\frac{{\mu - r}}{{(m + v){\sigma ^2}}} \leqslant {B_1}(t) \leqslant \frac{{{\mu ^2} - {r^2}}}{{2r(m + v){\sigma ^2}}}, \forall t \in [0, T]$ and $T \in [0, \infty),$ and by (58), then (66) holds for $\forall t \in [0, T]$ and $T \in [0, \infty),$ because of the property of quadratic function. According to (63)–(65) and Cauchy-Schwarz inequality, we have

Hence, property (2) holds.

Proof of (3). By (22), with an appropriate constant ${M_3},$ we get

where the first estimate in (68) follows from the deterministic and bounded $\theta _0^*(t)$ on $[0, T],$ and by (59) and Theorem 5.1 in Zeng and Taksar (2013), the last estimate is easily derived.

Moreover, according to, we can see that ${Q^*}$ is well-defined.

Inserting ${\theta ^*}(t)$ and ${u^*}(t)$ into (13) arrives at

where the first estimate follows from the Cauchy-Schwarz inequality and the last estimate from (67) and (68). Thus, property (3) holds.

With all the properties are satisfied, the result of Kraft (2004, Corollary 1.2) guarantees $({u^*}, {\theta ^*})$ is an optimal strategy and $V(t, x, s)$ is the corresponding value function.

5.   Special cases

This section considers some special cases of the problem (14), such as the investment-only case and the case with ambiguity-neutral insurer (ANI), respectively. These results here are only provided without giving the proofs.

5.1.   Investment-only case

If there is no reinsurance, i.e., ${m_{\rm{1}}}(t) = {D_{\rm{1}}}, {m_{\rm{2}}}(t) = {D_{\rm{2}}},$ then $X_i^m = {X_i}, Y_i^m = {Y_i}, {\xi _1} = {\xi _2} = 0,$ and (3) can be rewritten as

According to (11), the above wealth process for an AAI under the probability Q is

and the HJB equation is given by

where $\tilde J$ is a short notation for $\tilde J(t, x, s{\rm{), }}$ representing the optimal value function of the investment-only problem with the boundary condition $\tilde J(T, x, s{\rm{)}} = U(x),$ and

Theorem 5.1. For the investment-only problem (71) under exponential utility (18), i.e. in (15), then the robust optimal investment strategy is

and the optimal value function is given by

where ${L_{\rm{1}}}(t)$ is given by (44),

and $\tilde M = \lambda {\eta _1}{\mu _X} + \lambda p{\eta _{\rm{2}}}{\mu _Y}$ and ${\tilde f_1}(t)$ is given by

In addition, if $p = 0, {m_{\rm{1}}}(t) = {D_{\rm{1}}},$ it means that only one class of insurance business is considered, in other words, there are no dependent risks.

Corollary 5.2. If for the investment-only problem, the robust optimal investment strategy is the same as (73), and the optimal value function is

where

and

5.2.   ANI case

If ambiguity-aversion coefficient $m = \rm{0},$ our model will reduce to an optimal control problem for an ANI. Then, the wealth process under probability measurers P is given by (8). Let the optimal value function be

where $\bar u = \left\{ {{\rm{(}}{{\bar m}_1}{\rm{(}}t{\rm{), }}{{\bar m}_2}{\rm{(}}t{\rm{)), }}\bar \pi {\rm{(}}t{\rm{), }}t \in [0, T]} \right\},$ and HJB equation is

Note that $\bar J$ is the short notation for $\bar J(t, x, s)$ with $\bar J(T, x, s) = U(x).$ Let

Note that, if ${\xi _1}/v{\bar a_l} \geqslant 1$ and $\bar h({D_1}) \geqslant 0,$ according to the proof of Theorem 3.1, by the similar analysis of that the equation $\bar h(x) = 0$ in (32) has a unique solution ${\bar x_0}(t) \in [{\bar a_l}, {D_1}]$ when $\bar h({D_1}) \geqslant 0$, it's easy to prove that equation $\bar h(x) = 0$ has a unique solution ${\bar x_0}(t) \in [{\bar a_l}, {D_1}].$

Theorem 5.3. When for the optimal control problem (74) of an ANI who ignores ambiguity with utility (18), the optimal investment strategy is

and (ⅰ) if ${\bar a_l} = 0$ or $\frac{{{\xi _1}}}{{v{{\hat a}_l}}} \geqslant 1,$ ${\bar t_0} \leqslant T$holds. For ${\bar t_0} \leqslant t \leqslant T,$ the optimal reinsurance strategy $(\bar m_1^*(t), \bar m_2^*(t))$ is given by

For $0 \leqslant t < {\bar t_0},$ if the solution to $\bar h(x) = 0$ exists, the optimal reinsurance strategy $({\tilde {\bar m}}_1^*(t), {\tilde {\bar m}}_2^*(t))$ is expressed by

and the optimal value function is

(ⅱ) if $\frac{{{\xi _1}}}{{v{{\bar a}_l}}} < 1,$ in this case ${\bar t_0} > T.$ For $0 \leqslant t \leqslant T < {\bar t_0},$ the optimal reinsurance strategy is expressed by (76), and the optimal value function is given by

$\bar J(t, x, s) = - \frac{{\rm{1}}}{v}\exp \{ - vx{e^{r(T - t)}} + {\bar K_{\rm{3}}}(t) + {s^{ - {\rm{2}}\beta }}{\bar L_{\rm{1}}}(t)\}, {\rm{0}} \leqslant t \leqslant T,$

where

and $M$ is given by (24).

Furthermore, if $p = 0$ in Theorem 5.3, the optimal reinsurance-investment will coincide with Corollary 4.5 in Gu et al. (2012) and A et al. (2018). This means that our model extends the results in Gu et al. (2012) and A et al. (2018) to the case of robust optimal formulation under p-thinning dependent risks.

6.   Numerical examples and discussion

This section investigates the impacts of parameters on the optimal strategies. Here only the analysis for the case of ${\xi _1}/((m + v){a_l}) \geqslant 1$ is taken for consideration. For drawing convenience, the claim sizes ${X_i}$ and ${Y_i}$ are assumed follow uniform distribution $U(0, 1).$ Throughout this section, unless otherwise stated, the basic parameters are given by ${\xi _1} = 2, {\xi _2} = 1, r = 0.05, \mu = 0.09, m = 0.5, v = 0.5, t = 10, T = 20, p = 0.2, s = 5, \sigma = 0.16, \beta = 1/3.$ The conclusions are drawn from Figures 1–10.

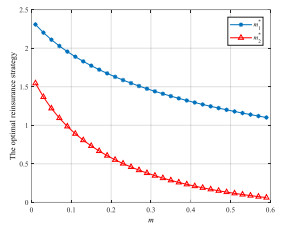
(1) Effect of parameters $m$ and v on the optimal reinsurance-investment strategy.

Form Figures 1–2, we can see that both the optimal excess-of-loss reinsurance strategy $m_i^{\rm{*}}$ and the optimal investment strategies ${\pi ^{\rm{*}}}$ decrease with m. As we know, m is the ambiguity-aversion coeffcient representing the insurer's attitude toward the uncertainty of the model, which is reflected in the claim process and the risky assets' price process. The larger m is, the more uncertain risk aversion the insurer is, so the insurer tends to adopt a lower reinsurance-investment strategy. Then, the risk from model uncertainty is spread to the reinsurer.

Figures 3–4 shows that $m_i^{\rm{*}}, {\pi ^*}$ and $\pi _{\rm{1}}^{\rm{*}}$ decrease with respect to v. Note that ν represents the insurer's risk aversion coefficient, the larger ν is, the more risk averse the insurer is, thus, as v increases, the insurer would like to reduce the investment amount to avoid risk, meanwhile, he/she prefers to reduce the retention level of reinsurance so as to transfer more underlying risks to a reinsurer.

(2) Effect of parameters $t, r, p$ on the optimal reinsurance strategy.

From Figure 5, we can see that $m_i^*$ increases with respect to the time t. As time passes, the insurer will obtain much wealth from insurance business and investment, so he/she is able to take more insurance risk and prefers to raise the retention level of reinsurance.

Figure 6 demonstrates that $m_i^*$ decreases with the risk-free interest rate r. The larger r is, the more attractive the risk-free asset is, so as r increases, the insurer prefer to invest more wealth in the risk-free asset rather than purchase more reinsurance.

Figure 7 indicates that $m_i^*$ decreases with respect to p. Since a larger p implies greater values of two expected claim numbers, so the insurer prefers to retain a less share of each claim.

(3) Effect of parameters $\mu, \sigma, s$ on the optimal investment strategy.

Figure 8 reports that $\mu$ exerts positive effects on the optimal investment strategies ${\pi ^{\rm{*}}}$ and $\pi _1^{\rm{*}}$. Note that $\mu$ is the rates of the risky assets' return, as $\mu$ increases, the insurer will gain more from investment. Therefore, the insurer would like to increase the investment.

As shown in Figure 9, ${\pi ^{\rm{*}}}$ and $\pi _1^{\rm{*}}$ decrease with respect to the standard volatility $\sigma.$ As $\sigma$ increases, the volatility of risky asset will fluctuate a little drastically, then the insurer prefer to decrease the investment so as to avoid risks.

We see from Figure 10 that ${\pi ^{\rm{*}}}$ and $\pi _1^{\rm{*}}$ increase with respect to s. A larger s means higher the risky asset's price, hence the insurer will wish to increase the investment in the risky asset.

In addition, in Figure 4 and Figures 8–10, we compare that ${\pi ^{\rm{*}}}$ with model uncertainty and $\pi _1^{\rm{*}}$ without model uncertainty, and we find that $\pi _1^{\rm{*}}$ is larger than ${\pi ^{\rm{*}}}.$ It means that it is necessary to study the robust optimal control problems which can help us make more reasonable decisions.

7.   Conclusions

This paper investigates a robust optimal excess-of-loss reinsurance and investment problem with p-thinning dependent risks for an AAI under CEV model. We aim to maximize the expected exponential utility of AAI's terminal wealth. Applying the stochastic control theory, we obtain the explicit expressions of the optimal excess-of-loss reinsurance and investment strategies and provide some special cases. Finally, we present some numerical examples to illustrate our results.

There are still some problems needed to be investigated in this direction. Firstly, we only deal with the excess-of-loss reinsurance, in fact, one can consider other reinsurance such as stop-loss reinsurance in our model. Secondly, other kinds of dependent risks can be taken into consideration in our problem, in addition, this paper assume the risky asset's price process and wealth process are independent, it is more realistic to consider the correlation between the risky asset's price process and wealth. Thirdly, it is meaningful to discuss other objectives such as the general utility function and mean-variance criterion in our model. Although these problems are challenging, they are meaningful and interesting to be investigated, thus we will focus on these optimal problems so as to enrich our research in the future.

Conflicts of interest

The authors declare that there are no conflicts of interest regarding the publication of this paper.