Career plans and wage structures: a mean field game approach

1.   Introduction

The mean field game (MFG in short) approach introduced in ^[1,2,3] (see also ^[4,5] for recent presentations), describes the relationship between individual decisions and the full population behavior. Here, we use the MFG formalism to analyze the relationship between individual career decisions and the labor market structure. More precisely, we wish to describe the ideal wage structure which allows firms, whatever its size and the employee career stage, to guarantee the individual's optimal career track in terms of his income. Note that if MFG applications to the labor market exists (^[6]), and to the general labor theory (see ^[7] for example on promotion theory), we have not found elements associated to notion of workforce dynamics and labor mobility (see ^[8] for a review on behavioral economic application to the labor theory). Also MFG have been used in other fields of economics as banks ^[9], mining ^[11], see ^[10] for a survey. Another class of problems related to MFG are the so-called finite horizon planning problem, which has been studied in ^[12,13,14]. Other related problems have also been explored, as optimal hiring policies from a cost-experience ^[15] and optimal organizational structure design ^[16].

Note that the principal difficulty associated with the MFG methodology lays in its inherent analytical complexity. Closed formula or even analytically tractable conclusions are rare and the MFG field heavily relies on numerical solutions ^[17,18]. However, this is not the case with our present framework. If we provide a couple of general results and properties, we have adopted a more empirical approach. We have indeed directly shaped our problem based on standard assumption on the labor market structure to infer analytic solutions on the wage distribution and the search cost structure. To this extent, the interest of the paper relies on the modeling issue and we leave open the complex mathematical questions which range in the field of inverse problems.

The paper is organized as follows. In the second section, we present our model and detail the intuitions upon which it was constructed. We then compute analytical solutions and discuss results under specific hypotheses. The last section discusses potential implications and shortcomings and offer perspectives on how to expand this research.

Important Legal Remarks. The findings and opinions expressed in this paper are those of the authors and do not reflect any positions from any company or institution.

2.   Approach to labor dynamics

Career optimization. In a given uniform economy, ignoring outsourcing and offshoring, a labor group $G$ is composed of a large number of individuals acting as rational economic agents. We assume that those agents seek to maximize their earnings. To do so, they can move between firms of various size $q \in [0, +\infty)$, while increasing their hierarchical position $z \in[0, +\infty)$. Note that those evolutions are stochastic in nature. The agents' density in the continuous "firm size - experience" plane is noted $m(t, q, z)$. Given the knowledge of the market wage structure $\omega (t, q, z)$, an individual can choose, at any time, to change firm. The associated "control'' is denoted by $v(s) = \frac{dQ(s)}{ds}$. However these changes come at a cost represented by a general friction term $C(t, Q(t), Z(t), v(t))$. For the individual taking a decision in a given a state $(Q(s), Z(s))$ at time $s$, his optimal average earning is given by the following formula :

Here $\mathbb{E}$ represents the expectation with respect to the randomness in hierarchical progress, $\rho>0$ acts as a discount rate representing the preference of individuals for short term returns in their employment strategy. Note that a framework of infinite horizon has been chosen to simplify the approach. We assume that from an agent standpoint, the labor market structure (e.g. $m$) and the wage system $\omega$ can be fully observed. So the key questions are about understanding the optimal trajectory that the market offers (e.g. optimal $v$) and the associated search costs $C$.

Labor market hypothesis. We assume that the hierarchical evolution of an individual is assumed to be random and position dependent. This will be transcribed by the following formulation:

Where $r(t, z, q)$ represents the average hierarchical evolution speed, $W_t$ stands for the classical standard Brownian motion. Hierarchical promotion uncertainty $\sigma$ will be assumed to grow with company size $\partial_q\sigma>0$ and hierarchical position $\partial_z\sigma>0$. We indeed suppose that within bigger firms there is less visibility on people and projects so that learning opportunities become more random. Additionally learning uncertainty tends to increase with the experience level.

The inter firm evolution (on the $q$ axis) is considered deterministic as it is associated to a turnover decision that fully belongs to the individual:

Based on data that we will review in the last section of this paper, we assume wages to increase with respect to the experience level and the company size (see ^[19] or ^[20] for an empirical justification),

The cost $C$ of moving within the labor market space accounts for frictions in the job search process (see ^[21]) such as possible unemployment periods. Therefore, we assume that $C$ depends strongly on $v(s) = \frac{dQ(s)}{ds}$ and that

which means that cost are incurred because of job changes.

Associated PDEs. This optimization problem eq. (2.1) can be solved by using classical control theory tools such as dynamic programming ^[22,23]. To do so, we introduce the Bellman's average cost function $J$ for an individual in a state $(z, q)$ at time $t$. The individual follows a strategy $v(\cdot) \in \mathbb{C}^1:[0, \infty[ \to \mathbb{R}$,

and

It is standard that this optimal cost solves the Hamilton-Jacobi-Bellman (HJB in short) equation

where $\mathbb{A}$ represents the differentiation operator associated with the dynamics (2.2)-(2.3). This equation is backward and thus we do not state an initial condition. In this case, we have:

The solution $u(t, q, z)$ of the HJB equation gives the optimal strategy under the form of a feedback

At any time $t$, for an individual in the state $(q, z)$, the best company change speed is ${\widehat v}(t, q, z )$.

Mean field games. Mean field games come when the above optimal control problem is coupled to the full worker population dynamics, which we describe now. Assume that in the labor group $G$ each individual optimizes its gain according to the optimal strategy ${\widehat v}(t, q, z)$. The Kolmogorov equation for the density of workers $m(t, q, z)$ reads:

Here $\mu$ represents the departure rate (retirement, change of labor group). We also assume the following boundary conditions. At $z = 0$, there is an entry flux formed by new generations, with generation rate $g(q) b(z, q')$, and transfer from a different labor group with rate $b_0$,

3.   Labor market behaviors: possible explanations

The framework detailed in the previous section will now be leveraged to understand possible labor market behaviors. Individuals are assumed to know the average wage structure across company size. First, the framework is used in its one dimensional version (e.g. only the company size variable $q$ is kept) to understand the implications of the population distribution in a labor market. In a second part, the two dimensional framework is explored and the effect of stochasticity is considered. To generate some insights, the current approach will assume a steady state. This means that neither $\omega, C$ nor $m$ will depend on time $t$.

3.1.   Individual careers with search costs

Assume a one dimensional framework in $q$ (the firm size axis) such that $q\in ]0, \infty[$. Suppose that individuals have a full knowledge of the wage structure $\bar w(q)$. Suppose also that they understand the labor market friction $C(q, v)$ which we assume of the form

We will sometime use that $F(\cdot)$ is decreasing, and propose the following interpretation behind this assumption: big companies have a huge reputation on the market place which makes it easier for their employees to find a new job. Individuals will seek to adopt a strategy $\widehat v(q)$ (i.e. change company at a certain pace) to maximize their earnings. This is translated by the previous HJB equation (2.6), which first order condition leads to the expression

Then, the HJB equation can be solved with

Differentiating the above equation with respect to $q$, we find the following non trivial link between the individual strategy $\widehat v(q)$ and the wage structure $\omega$:

This is a scalar conservation law which is understood in the entropy sense since the HJB equation is understood in the viscosity sense.

The Fokker-Planck equation (2.9) can be used with the appropriate adaptation of the boundary condition to determine the overall working population repartition. We take

Note that, because the total population is finite and we expect that ${\widehat v}$ has a limited growth for $q$ large. Integrating in $q$, we find

The following statement asserts that, as expected for an inverse problem, the appropriate wage structure exists under some conditions on the population distribution $m$.

Proposition 3.1. Assume (3.1) and that $m(q)$ satisfies the two conditions, for all $q \geq 0$,

then, up to an additive constant, there is an unique wage structure $\omega(q)$ for which both (3.2) and (3.3) hold true, moreover $\omega(q)$ is increasing.

It has to be noted that adding a constant to $\omega (q)$, adds up a constant to the value $u(q)$ but does not change the optimal strategy ${\widehat v} (q)$. This explains that the solution admits a free parameter and this reflects again the homogeneity in the labor group $G$ because only variations in the wages counts in the career progress. With regards to the assumptions, it is expected that $m$ is decreasing while $F$ is increasing if large companies, with high salaries, induce large search costs. Thus the first assumption is fulfilled in a wide range of data. Also the positivity in the second assumption is automatic from the observation (3.4). It is more difficult to figure out the meaning of the upper bound in the second assumption and the examples given after the proof show that it means that $\rho$ is large enough compared to $\mu$ or the decay of $m$ is fast compared to the growth of $F$.

Proof of Proposition 3.1. On the one hand, we can solve the Fokker-Planck equation as an equation on $\widehat v(q)$ which gives us the individual strategy

In view of the relation (3.4) this also written

On the other hand, we may also rewrite (3.3) as

Therefore the equation (3.2) can be replaced by a second order polynomial in ${\widehat v}$, namely

that is also written

From our assumption, the expression ${\widehat v}$ in (3.5) satisfies $v_M -{\widehat v} >0$ and thus, the above equality gives $\partial_q \omega>0$ and thus $\omega(q) >0$ up to a constant.

Example 1. Assume a firm wants to structure its wage to create a specific internal labor market (represented as a continuum of departments of various size $q$). The firm aims at having an exponential decay for the workers population distribution $m(q)$. Additionally the firm wants to structure its search costs $F(q)$ in such a way that they follow a power law (e.g. individual from large departments can move more freely). This is written as

assuming here that $1$ is the smallest company size. We compute

Then, the second assumption of Proposition 3.1 is reduced to the above inequality which imposes

We deduce from the expression (3.6) that

This wage structure is compatible with a bounded average salary profile obtained as $q \gg1$, only if we impose $\beta >1$.

Example 2. In the case of power laws

The second assumption of Proposition 3.1 is reduced to

which imposes

We deduce from the expression (3.6) that

and thus

The condition that the average salary $\omega$ remains bounded leads us again to impose $\beta >1$.

Example 3. We now ask another question, an individual agent observes the workers population distribution $m(q)$ and the wage structure $\omega(q)$ and wants to infer the cost $F$ if he changes company (or department).

We assume an exponential decay (resp. increase) for $m(q)$ (resp. $\omega(q)$)

From an individual perspective, this means that the optimal evolution speed is given by:

Leveraging (3.6), this leads us to the following search cost structure:

This means that, up to a constant $F_0$, the employee can identify the search cost

3.2.   What are the trade-offs between hierarchical and lateral moves?

We come back to the two dimensional framework in $q \in[0, +\infty[$ (the size of the firm) and $z \in [0, +\infty[$ (the hierarchical level). Suppose that the labor market friction associated to a change in company is the same as in equation (3.1). We still aims at presenting an example where the cost can be determined with simple rules from the knowledge of the job market. We assume that promotion rates are only depending in the hierarchical level $r(z)$ and deterministic ($\sigma = 0$). Finally, we assume a separated variable format for the labor market

We are going to solve the problem with separated variables for the solution $u = u_1(z) u_2(q)+ C_u$ of the HJB equation (2.6), where the constant $C_u$ is adjusted to $\omega$ since we are going to determine $\partial_q \omega$ as in Section 3.1. Similar to the previous section, individuals seek to adopt a strategy $\widehat v(q)$, i.e., change company at a certain pace, in order to maximize their earnings. With separated variables, the first order condition of the HJB equation (2.6) leads to $\widehat v(z, q) = \widehat v_1(z) \widehat v_2(q)$ because

where, to define the $\widehat v_i$, the free multiplicative constant is useless as we can see it later.

Then, the HJB equation can be solved with

Furthermore, differentiating in $q$ this equation leads again to the wage structure. We write:

On the other side, the Fokker-Planck equation (2.9) determines $\widehat v_1$ and $\widehat v_2$ thanks to

which gives, with a free parameter $b>0$,

Following Section 3.1, this determines explicitly $\widehat v_2(q)$ and $\partial_q \widehat v_2(q)$ as

Therefore, using (3.12), we arrive at a non-trivial relationship between the individual strategy and the wage structure

It is immediate, by its homogeneity, that the above formula does not depend on $b$. Therefore, we conclude that

Proposition 3.2. We assume that the population distribution $m(z, q) = m_1(z)m_2(q)$ satisfies

that for some constant $b_1>0$ (we take $b = 1$ in (3.12))

and assume that, for all $q>0$,

Then, there is a cost function $\omega (z, q)$ (unique up to the addition of a constant), increasing in $q$, for which the individual worker's optimal strategy has separate variables and generates the distribution $m$.

Example 4. Let us consider a working population distribution that follows a power law

From the relations (3.13), (3.14), we infer that (assuming $b = 1$)

Assuming, for simplicity of the calculations, that

(e.g hierarchal progression speed is convex decreasing in $z$) forces $\widehat v_1 = r_0\; (\nu- \alpha_1 + \mu_0)(z+1)^{\nu-1}>0$. Using (3.15) and adding the assumption that $F(q) = F_0(q+1)^{\beta}$ leads to a simple wage equation:

The condition $\partial_q\omega>0$ then leads to following structural condition:

3.3.   How does promotion uncertainty impact employees choices?

Assume that the same framework as in the previous subsection except that promotions rate are stochastically depending in the hierarchical level $\sigma = \sigma(z)$. This changes the HJB equation and the Fokker-Planck equation. However the first order optimality condition of the HJB equation (2.6) is unchanged and still leads to (3.11). Therefore, equation (3.12) becomes

The individual strategy $\widehat v(q)$, the promotion dynamics ($r(z)$ and $\sigma(z)$) and the Fokker-Planck equation (2.9) for the overall working population repartition are linked by the following identity

which gives again two equalities

These determine directly $\widehat v_1(z)$ and the expression (3.14) for $\widehat v_2(q)$ still holds. They can be inserted in the form (3.15) of the equation for the wage. Again the wage structure follows explicitly from the knowledge of the workers population distribution $m$.

Example 5. Leveraging the example of the Section 3.2, we assume that

The expression of $\widehat v_1$ has to be updated and becomes:

The new condition indeed becomes

Note that the case with uncertainty is much more complex from an analytical standpoint than the deterministic case from Section 3.2.

Therefore, introducing uncertainty increases movement speed and decreases the firm size threshold $q^*(z)$ where wages stop increasing.

4.   Discussion

We now calibrate our framework to real world data and explain what the associated findings are in light of the previously developed framework. Note that the data that are used in this section represent the professional services firms (PSFs in short) in the US (NAICS code 54). The public data used in this paper has been extracted from the US Census Bureau 2015 files https://www.census.gov/programs-surveys/susb.html.

4.1.   PSFs labor market structure in the US

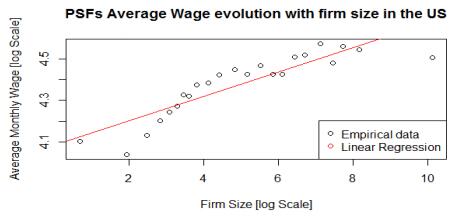
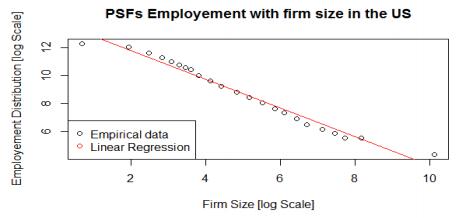
The Figure 1 confirms the well established observation that employees distribution follows a power law in firm size. A linear regression on the log-log plane can be performed and leads to:

which is in accordance with the formulas (3.7) in second example of Section 3.1. Note that $\alpha$ is extremely close to 1, which not only entails a fat tail distribution but also pushes the framework to the limit of integrability.

The wage increases with the company size as shown in the Figure 2 and we can try to fit the parameter $\beta$ (when considering that $\omega(q) = \omega_0.q^\beta$) with a linear regression in the log-log plane. The data in Figure 2 leads to an estimate of $\beta \approx 0.059$. But one can see that the power model can be challenged as it doesn't fit properly the wage evolutions observed on the far end of the firm size landscape. The wage evolution indeed appears concave, meaning that small and large firms tend to pay less than what can be anticipated based on the mid size firms wage evolution.

4.2.   Search cost estimations

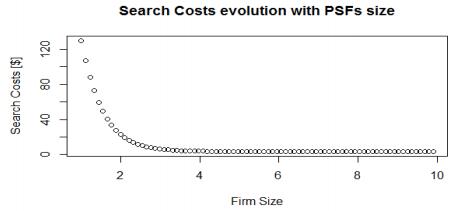
With the data of the previous subsection, it is possible to estimate the internal dynamics of the US PSFs sector. Assume that $\mu$ is on par with the US mortality rate (e.g. workers exits the labor market because they die). This means that $\mu$ would be around 0.1% & 0.9% per year according to the CDC. Note that this doesn't account for retirement.

This estimate and the fat tail distribution (4.1) directly translate into an evolution speed $\widehat v = \mu \frac{q}{\alpha-1}$ per year which means that the individual evolution speed in the firm size landscape is linearly increasing in $q$. This of course stresses the need for a robust estimation of $\mu$.

As per the search costs $F$, they can be estimated with a simple update to the equation (3.9). This leads to the following equation:

Therefore, we find the expression

As displayed on the Figure 3, search costs are convex in firm size. Interestingly search costs are higher for small firms and then quickly decrease when entering mid sized firms, where they quickly become negligible.

4.3.   Internal labor market wage structure

We have seen how individual workers can leverage publicly available information such as wage structure (see Glassdoor) and employment distribution (records can be maintained by countries). Yet this paper shows that the relationship between labor market structure and individual careers is not a one way street. So let's assume that a firm has a target in terms of structure $m(z, q)$ across its multiple departments. Assume that the firm has a promotion rule given by $r$ that is tenure based. Given the framework in the previous section, the firm can understand the implication in terms of lateral move for its employee pool. The firm can also determine the best way to structure its wage given a search cost structure $F$.

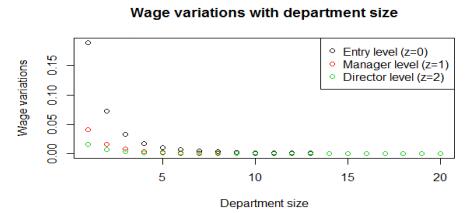
Assume that the firm employment across departments is distributed according to a power law (size wise). Assume also that from a hierarchical standpoint managerial ranks are also distributed according to a power law. This means that the span of control of a manager is not fixed but gets lower in the higher ranks. These assumptions can be used to describe a flat organization structure. This can be described by the equation (3.16) used in our 4th example. Assume for example that $\alpha_2 = \alpha_1 = 2$. This means that for 4 entry level workers ($z = 0$) there is one first level manager ($z = 1$) and that for about 2 first level manager there is one director ($z = 2$).

As we are looking at an internal labor market, let's assume that the promotion speed $r$ is decreasing with the hierarchical position with a power law form. Assume it takes 3 years to get promoted from entry level to a manager level $r_0 \approx 0.33$ and that $\nu = -1.5$ (e.g promotion from manager to director will take about 8 years etc...). As per organizational exit rate, assume that the organization looses $33\%$ (e.g. $\mu_0 = 1$) of its entry level population per year due to turnover and that the turnover has the same structure as the one described in example 4.

This leads to the following evolution internal evolution speeds:

This means that the bigger the employee's department is, the slower the lateral progression is. Additionally the higher the employee is in the pyramid, the less chances there is for a lateral move. This raises an interesting question of trade off between lateral and vertical moves. The ratio $\frac{\widehat v}{r} = v_0 \frac{z+1}{q+1}$ shows that lateral moves will prevail over vertical ones when $z+1>\frac{q+1}{v_0}$.

Finally assume that search costs are increasing with $q$ in a similar fashion that in example 4 with $\beta = 2$. To incentivize this framework, the firm has to set a wage structure that obeys

As depicted in Figure 4, this means that wages have to change at entry level between department to incentivize the required dynamics, but that the higher the employee gets in the hierarchy, the less difference there will be in terms of wage because of department size (e.g. span of control).

Although interesting, note that this example still suffer some limitations. It indeed assumes that the firm is an isolated labor market (e.g wages are not subject to competition). Additionally we assume that external hiring is limited to the bottom of the hierarchical pyramid (e.g $z = 0$), which may not hold in a real set up.

5.   Conclusions and perspectives

This paper uses the MFG approach to understand the relationships between individual careers and wage structure. We have shown, in a steady state set up how company can internally structure their wage distribution to incentivize mobility. We have also shown that individuals can estimate their job search costs from simple market information. These two questions range in the field of inverse problems for the MFG system of equations.

From the application point of view, our analysis presents a couple of shortcomings. First of all, one may challenge the steady state assumption that was made throughout the model. We believe that finding non steady analytical solutions to the framework will require additional assumptions. Otherwise numerical simulations will most probably be required. Second, the model relies on the idea that workers are interested in maximizing their income. This is quite a narrow view of what may happen in reality. A natural expansion of the framework would be to enhance the workers objective function with some utility consideration. One may want to add leisure considerations for instance or discuss how income taxation may affect labor dynamics. Finally we have made very strong assumptions regarding the structure of search costs. Even though this was helpful to construct our model, we have not found any formal search costs analytical structure in our literature review. As such, we believe that it may be interesting to run an empirical study to better understand the nature of the search costs. This can then quickly be re-embedded into our framework to yield career planning considerations. One may also wish to relax the assumptions of homogeneity in the labor group under consideration and of the full observability of the wage structure among this group.

From the mathematical point of view, one may wish to determine general conditions on the population distribution to ensure that the inverse problem is solvable. In view of the difficulties encountered by the planning problem, we can expect this question raises considerable difficulties also. One may also wish to find a formalism which determines the full wage structure and avoids the undetermined additive constant.

A.   Formal justification of the HJB equation

To simplify, we consider the deterministic case and the general notations

We compute, using the notation $Q(s) = \frac{\partial X(s, t, x)}{\partial t}$

The last term requires some manipulations. The quantity $Q(s)$ satisfies

that is also written

But we may also compute $\bar Q(s) = \frac{\partial X(s, t, x)}{\partial x}$ which satisfies

This means that

Back to the formula for $J$, we find

and we observe that

We conclude that

which indicates that this has to be considered as a backward problem, with $J_{v(\cdot)} (t = \infty, x)$ given (because $\rho$ is an absorption term, $F\geq 0$ implies $J\geq 0$).

The optimal cost is defined as

It is obtained when choosing $v(t)$ so as to increase as much as possible $J$ at each time $t$ in the formula (A.1), which means maximizing the backward derivative

For a rigorous derivation, see ^[4,5,22,23].

Acknowledgments

B.P. has received funding from the European Research Council (ERC) under the European Union's Horizon 2020 research and innovation program (grant agreement No 740623).

B.P. and D.S. acknowledge partial funding from the ANR blanche project Kibord ANR-13-BS01-0004 funded by the French Ministry of Research.

Conflict of interest

All authors declare no conflicts of interest in this paper.