This research is aimed to examine the effect of environmental performance on firm value with environmental disclosure as a mediation variable. Sample of research is non-financial companies at the Indonesia Stock Exchange that have followed the Environmental Performance Assessment Program (PROPER) held by the Ministry of Life Environment and Forestry. The data analysis method is Structural Equation Modeling-Partial Least Square (SEM-PLS), and the analysis operation is facilitated by the software of WarpPLS 6.0. The result of analysis has given a few findings. One is that environmental performance has a positive effect on firm value and environmental disclosure. Other result shows that environmental disclosure does not affect firm value and does not mediate the effect of environmental performance on firm value. In general, all the results support the stakeholder theory and the resource-based view theory. Companies with environmentally friendly commitments can be a strategy in creating higher firm value.
1.
Introduction
The company’s main goal is to maximize the firm value. Company success to increase firm value does not merely rely on firm performance. Some literature said that successful companies are those with the ability to satisfy the demand of stakeholders, including environment, employee and community (Freeman and Evan, 1990; Marcus and Geffen, 1998; Sharma et al., 1999). The environment is considered as a stakeholder because the environment conserver group has successfully urged the company to see the environment as a factor that determines production. Therefore, natural resources should not be excessively exploited without conservation effort. Irresponsible behavior to the environment has a serious impact on the environment that can influence natural balance, and the impact is mostly bad such as global warming, climate change, natural disaster, and water, air, and soil pollutions. A negative impact on the environment is the price that must be paid by the community due to the company’s negligent activities. Non-shareholding stakeholders (community, government, and environmentalist) and company shareholders are always in conflict. Since the possibility of a conflict of interest between stakeholders and shareholders is high, then the right of stakeholders must be accommodated, and this right is actualized through a term corporate social responsibility, which is, company’s responsibility to implement environmental conservation (Jo et al., 2012).
Previous literature shows that environmentally friendly companies can gain economic benefits, which among others is attaining competitive advantages such as more efficient operations, productivity improvement, and minimum environmental destruction (Alexopoulos et al., 2011; Majumdar and Marcus, 2001; Hart, 1995; Porter and Linde, 1995; Caracuel and Mandojana, 2013; Utomo et al., 2018). Companies with strong environmental performance can reduce operational cost, improve access to resources, and minimize employee turnover (Berrone and Gomez-Mejia, 2009). The increase of environmental performance is potentially improving firm value (Al-Tuwaijri et al., 2004; Wassmer et al., 2014; Muhammad et al., 2015; Iqbal et al., 2013; Utomo et al., 2019).
Companies with superior environmental performance always conduct environmental disclosure. Environmental disclosure improves firm value. Environmental disclosure is not only a form of obedience to the law but also useful to produce public legitimacy to the company (Kuo and Chen, 2013; Iatridis, 2013). Company consistency in providing environmental disclosure can surely maintain company legitimacy, which then delivers a superior impact on firm value (Mousa and Hassan, 2015).
The result of research shows that environmental performance has positive effect on environmental disclosure and firm value. Meanwhile, environmental disclosure does not affect firm value and does not mediate the effect of environmental performance on firm value.
This research gives a significant contribution to works of literature concerning the relationship between environmental performance, environmental disclosure, and firm value. This research provides insight into strategies for increasing company value by increasing environmental performance. Efforts to improve environmental performance will encourage companies to comply with environmental regulations and conduct environmental management activities based on the principles of sustainable development. In addition, this research provides insight that companies in Indonesia do not yet have standard environmental disclosure reports. The implementation of environmental disclosures varies according to the interpretations of each company.
This paper comprises five sections. Second section is Theoretical Base and Hypothesis Development. Third section is Methodology. Fourth section is Result and Discussion, while the final section is Conclusion.
2.
Materials and method
2.1. Theoretical base and hypothesis development
2.1.1. Environmental performance and firm value
Stakeholder theory states that companies that have a relationship with many stakeholders will more or less influence the activities of the company. Two kinds of stakeholders are known, namely internal and external. Like shareholders, stakeholders can demand something from the company. Sometimes, like or not, the company must face criticism from non-shareholding individuals whose negativity can be the reason for the decline of the company’s shareholding value because these individuals can impose their demand through boycott, lawsuit, and others. Brouwers et al. (2014) explained that social and environmental performances must be achieved by satisfying the demand of many stakeholders. Satisfying the demand of stakeholders has been considered as inevitable business costs but controlled at a certain amount. Moreover, Jensen (2001) emphasized that during value maximization activity, the company should not ignore stakeholder interest. In other words, the company should not only maximize economical goal but also environmental goals.
The argument of stakeholder theory is supported by another theory, which is, Resource-Based View (RBV). By using RBV theory perspective, Hart (1997) described that companies with competitive advantages are those that have been successfully developing organizational values, building good communication with all stakeholders, and integrating environmental goals with managerial strategies. Shortly, RBV theory stipulates that environmental responsibility is a way toward competitive advantage and better firm value.
Che-Ahmad and Osazuwa (2015) investigated the effect of eco-efficiency on overall firm value. The research sample consisted of 667 non-financial companies listed on the Bursa Malaysia in 2013. Eco-efficiency was measured by a dummy variable where 1 was for companies implementing environmental policies (eco-efficiency) and 0 otherwise. While the value of the company is measured by the natural logarithm of the stock market price. The main results show that companies implementing eco-efficiency can reduce the cost of equity and increase the market value of the company.
Muhammad et al. (2015) had conducted empirical tests on the relationship between environmental performance and company performance among the companies listed at Australia Stock Exchange (ASX). Data on environmental performance were collected from environmental reports submitted by the companies to the National Pollutant Inventory (NPI), while data of company performance were obtained from the ASX database. Company performance was measured by two indicators, namely accounting-based measure (ROA) and market-based measure (Tobin’s Q). The result of the empirical study by Muhammad et al. showed that environmental performance had a positive impact on financial performance and firm value before the world financial crisis but did not have a positive impact after the crisis. This result is supporting the finding of Al-Tuwaijri et al. (2004), which is, that the environmental and economical performances of the company must be walking together. Al-Tuwaijri implied that environmental performance is measured based on management’s activeness in dealing with problems concerning environmental norms and in making a trade-off with all stakeholders.
Sarumpaet et al. (2017) were testing the effect of environmental performance rating on company stock price. Environmental performance was measured with the PROPER rating given by the Ministry of Life Environment and Forestry. Sarumpaet et al. used a sample of 60 companies that were participants in PROPER in the period 2002–2012, which signifies that during this period, they were available to be assessed by the Ministry. Of these companies, 246 observation samples were obtained. The result indicated that environmental performance has a positive and significant effect on firm value. This position only prevails for companies with good environmental performance but not for those with bad environmental performance. It indicates that excellent environmental performance is associated with the high stock price, while low environmental performance is not relevant to market value.
Based on theoretical and empirical reviews above, the following hypothesis is made:
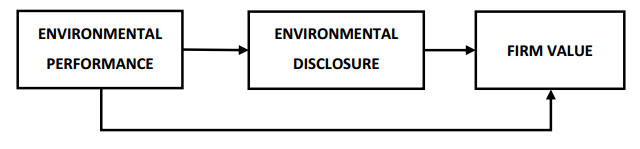
Hypothesis 1: High Environmental Performance is associated with high Firm value.
2.1.2. Environmental performance and environmental disclosure
Disclosure Theory asserted that companies with good performance have the incentive to make environmental disclosure in order to differ themself from companies with bad performance (Verrecchia, 1983; Dye, 1985). Companies with good environmental performance will always conduct environmental disclosure (Kuo and Chen, 2013; Zhang et al., 2014). According to disclosure theory, high environmental performance is producing high environmental disclosure. Al-Tuwaijri et al. (2004) said that good environmental performance influences environmental disclosure, which precisely makes the disclosure become more open and factual. Clarkson et al. (2008) have carried out the empirical study and the result showed that companies with excellent environmental performance will produce actually discretionary disclosure. This result is consistent with voluntary disclosure-based economic theory.
According to Latridis (2013), companies with less waste and good environmental performance tend to do environmental disclosure, and this environmental disclosure decreases information asymmetry. Fontana et al. (2015) found that environmental performance positively influences environmental disclosure of large companies because environmental disclosure can preserve company reputation. Handayati and Rochayatun (2015) discovered from their study that the environmental audit committee’s performance has a positive and significant effect on corporate social responsibility (CSR) disclosure.
After reviewing theoretical connection and empirical studies above, another hypothesis is proposed:
Hypothesis 2: High Environmental Performance is associated with high Environmental Disclosure.
2.1.3. Environmental disclosure and firm value
Environmental disclosure provides information about environmental management of the company in the past, present and future. Information concerning environmental management can be disclosed or expressed in many forms including qualitative statements, quantitative facts, financial reports or footnotes (Al-Tuwaijri et al., 2004). Signaling theory is a theory that undergirds environmental disclosure by the company. This theory says that information provided by the company will be used by investors for their assessment and decision. Signaling theory declares that if the company is good in delivering performance information, it will produce a positive signal to investors and potentially increase company stock price (Ross, 1977). Moreover, companies with the capability to provide better information to investors are those given easy access to capitalization (Bini et al., 2010). Environmental disclosure is aimed to show to the community about what is done by the company and the effect of company activity to the community. Some empirical studies have proved the effect of environmental disclosure on firm value. Empirical study by Latridis (2013) found that companies with high-quality environmental disclosure, which is done by giving relevant information, will provide a positive effect on stock price valuation.
Saka and Oshika (2014) declared that reducing carbon emission and improving environmental disclosure have positive effect on market price of company stock. Furthermore, Chang (2015) said that companies with high environmental performance are those with the capability to bear more social and environmental responsibilities, to strengthen communication with stakeholders, to reduce stakeholders’ anxiety concerning environmental management problems, and to improve company image and company’s competing ability. In other words, communicating environmental activity (through environmental disclosure) can improve firm value.
Khlif et al. (2015) found that social and environmental information has improved the value of South African companies but this information negatively influenced company performance in Marocco. Khlif et al. used stakeholder theory and legitimacy theory to understand the law of the host countries (African countries) and also the care of both managers and investors to social and environmental issues. Moreover, Plumleea et al. (2015) believed that the improvement of environmental disclosure quality is positively related to firm value, which is indicated by the increase of the expected cash flow and the decrease of capital cost.
Based on theoretical review above, two hypotheses are made as following:
Hypothesis 3: High Environmental Disclosure is associated with High Firm value.
Hypothesis 4: Environmental Disclosure mediates the effect of Environmental Performance on Firm value.
Pursuant to all hypotheses above, then empirical model is made as presented in Figure 1.
2.2. Research method
2.2.1. Research sample
The sample of this research includes non-financial companies whose stocks are traded publicly at the Indonesia Stock Exchange. The research period is 7 years from 2012 to 2018. Two criteria are used to determine the sample. One is that the companies must be listed on the Indonesia Stock Exchange (ISE), and the other is that the companies participate in Environmental Performance Assessment Program (PROPER). The data source is a secondary data panel comprising financial reports and annual reports of the companies listed at ISE, and also PROPER’s rating report (company performance in environmental management) published by the Ministry of Life Environment and Forestry. The data collection technique is documentation. After using these criteria, 44 companies are obtained as the sample, consisting of 33 companies in the manufacturing sector, 6 companies in the mining sector, 4 companies in the agriculture sector, and 1 company at the conglomeration sector (miscellaneous). After deciding to use the research period of 7 years, the total panel data observed is 308 (44 sample × 7 years).
2.2.2. Variable measurement
Dependent variable of this research is Firm value. In this research, firm value is measured with Tobin’s Q (Q). Some researchers have used Tobin’s Q as a proxy for firm value, such as Jaafar and El-Shawa (2009); Farooque et al. (2010); Caixe and Krauter (2013); Nguyen et al. (2015); Wahla et al. (2012); Warrad et al. (2013); Al-Saidi and Al-Shammari (2015); Lin (2011); Rashida et al. (2010). The current research will use Tobin’s Q formula modeled by Lindenberg and Ross (1981) as the proxy of firm value. This formula is written as following:
where,
MVS: market value of equity (market price x number of circulated stock sheet)
DA: book value of total debt
TA: total asset
Independent variable of this research is environmental performance, which is measured by PROPER Score. The score represents environmental performance rating released by the Ministry of Life Environment and Forestry (PROPER 2011). Five ratings are available in PROPER, which respectively is, (1) Gold Rating: Very Good, Score = 5; (2) Green Rating: Good, Score = 4; (3) Blue Rating: Adequate, Score = 3; (4) Red Rating: Bad, Score = 2; and (5) Black Rating: Very Bad, Score = 1.
Mediator variable of this research is environmental disclosure. This variable is measured using score given by Global Reporting Initiative (GRI) based Environmental Disclosure that comprises 30 items. The scoring is done by dividing the number of the item disclosed by company with the number of GRI environmental indicator item (Iatridis, 2013; Fontana et al., 2015). In the current research, the scoring of environmental disclosure also uses dummy variable, which respectively is:
● Zero (0): If company did not disclose GRI environmental indicator item.
● One (1): If company disclosed GRI environmental indicator item.
Environmental disclosure scoring process is formulated as following:
where,
X = Number of item disclosed by company.
n = Number of GRI environmental indicator item.
There are two control variables used in this research, namely company size (SIZE) and leverage (LEV). Many studies often used these variables to control firm value (Desoky and Mousa, 2013; Krivogorsky and Grudnitski, 2010), (Al-Tuwaijri et al., 2004; Wassmer et al., 2014; Muhammad et al., 2015; Iqbal et al., 2013). Company size is determined using natural logarithm of total asset, while leverage is measured with ratio of total debt to total asset.
2.2.3. Analysis method
Analysis method used in this research is the statistic alternative to Structural Equation Modeling (SEM), which is based on variance, or also called Partial Least Square (PLS) (Sholihin and Ratmono, 2013). Hereafter, research will use term SEM-PLS. This method is used due to some reasons. First is that the research model is relatively complex and involving more than one dependent variable. Therefore, SEM-PLS is considered suitable to analyze the relationship within the model. Second, the theories used and explained in this research are in a new category and therefore, SEM-PLS is the proper instrument for analysis. Third, it must be noted that SEM-PLS can work efficiently in complex models and small sample sizes. The computer software of WarpPLS 6.0 is used to facilitate the operation of SEM-PLS.
All hypotheses are tested using the following model equation:
where, ED = Environmental Disclosure, EP = Environmental Performance, SIZE = Company Size, LEV = Leverage, and Q = Tobin’s Q.
3.
Results and discussion
Table 1 shows descriptive data of research variables, which include minimum value, maximum value, mean value, and standard deviation. Concerning with environmental performance, it is shown that the companies already did environmental management in a way that is required by the law, and therefore, they have good environmental performance (3.15). After understanding company size of the sample, it is found that all companies in the sample are large companies (28.77) and their capital structure is averagely funded by debt at range above 30% of company asset (0.44). Environmental disclosure is in low category (0.227), which can be said that of 30 indicator items of Global Reporting Initiative (GRI), only 8 items are frequently disclosed by sample companies (30 x 0.227). For firm value (Tobin’s Q), it is indicated that market value of the company is higher than the value of company asset (2.32).
Analysis process in SEM-PLS usually involves two stages, which begins with measurement model evaluation and ends with structural model evaluation. Measurement model evaluation is done to determine reliability and validity of indicators that shape latent constructs. Structural model evaluation is aimed to predict the relationship across latent variables by examining how many variance that can be explained and counting significance value (p-value) (Latan and Ghozali 2016). However, the current research does not implement measurement model evaluation because research variables are manifest variable. Therefore, it is not necessary to estimate reliability and validity of the constructs.
Before testing main structural model, researcher evaluates goodness of fit of research model. The output of this evaluation is presented in Table 2.
Based on what is indicated by Table 2, research model is said as having good fit. It is proved by the fulfillment of some criteria. The first three criteria, precisely APC, ARS and AARS, have significance value (P-value) < 0.001, which respectively are APC = 0.167, ARS = 0.229 and AARS = 0.220. Next criteria, namely AVIF and AFVIF, have value < 3.3, which signifies that there is no multicollinearity across indicators and across exogenous variable. Tenenhaus GoF has value of 0.478 > 0.36, and this value is in big category, which then can be said that model fit is very good. Moreover, for SPR, the value is 0.857 > 0.7, while the criterion of SSR has value of 0.857 > 0.7. The criterion of NLBCDR has value of 0.714 > 0.70, whereas the value of RSCR is 0.995 > 0.90. Pursuant to the values of the last four criteria, research model is acceptable because there is no causality problem in the model (Latan and Ghozali, 2016). The estimated relationship across the constructs and the level of variance and effect size are shown in Table 3.
Pursuant to what is shown by Table 3, adjusted R-squared (R2) value of environmental disclosure is 0.352. This value is in moderate category (>0.25), which is showing that number of variation that can be explained by independent variable is 35.2%. Adjusted R-squared (R2) value of Tobin’s Q is 0.106. This value is included into small category (<0.25) indicating that number of variation explainable by independent variable and mediation variable is 10.6 %. Moreover, Q-squared values for each of environmental disclosure and Tobin’s Q are 0.357 > 0 and 0.128 > 0, which signifies that research model has predictive validity.
By virtue of the outputs in Table 3, then the relationship across variables can be estimated. Environmental performance has positive effect (significant at level < 0.1) on firm value (Tobin’s Q) with coefficient value of 0.072. Therefore, Hypothesis 1 stating that High Environmental Performance is associated with High Firm value is accepted. This result is supporting the findings given by previous studies conducted by Muhammad et al. (2015) and Sarumpaet et al. (2017), which in general said that high environmental performance is associated with high firm value. Companies that have successfully reduced negative impact of their production wastes on environment are those that have higher valuation than those with less environmentally friendly.
Result of another hypothesis test shows that environmental performance has positive effect (significant at level < 0.01) on environmental disclosure with coefficient value of 0.408. Therefore, it can be said that Hypothesis 2 stating that High Environmental Performance is associated with High Environmental Disclosure is supported. This result corroborates the findings of previous studies conducted by Al-Tuwaijri et al. (2004), Latridis (2013), Fontana et al. (2015), and Handayati and Rochayatun (2015), which generally concluded that companies with high environmental performance have incentive to disclose the information regarding their environmental activity to stakeholders. Result of descriptive statistic shows that mean value of environmental disclosure is low, and it is clearly contrasting with the result of hypothesis test. The explanation of this situation is that although sample companies have conducted environmental disclosure, but they do not follow environmental disclosure standard required by GRI. Indonesian companies that adopt GRI based environmental disclosure reports are still few in numbers.
Further hypothesis test indicates that environmental disclosure does not influence (at significance level of > 0.1) firm value (Tobin’s Q). It surely rejects Hypothesis 3 that states that High Environmental Disclosure is associated with High Firm value. This result is in contrast with the findings of empirical studies carried out by Saka and Oshika (2014), Chang (2015), Khlif et al. (2015) and Plumleea et al. (2015), which in general showed that environmental disclosure has positive impact on firm value. Surprisingly, the result of current research is consistent with Arafat et al. (2012) who found that environmental disclosure in Indonesia does not impact firm value. As previously explained, environmental disclosure among Indonesian companies is not much adopting GRI indicators. Other reason is that environmental disclosure through GRI indicators is voluntary in nature. Therefore, it is not surprising if environmental disclosure conducted by companies is not the main consideration of investors during valuation.
Mediation test is implemented to examine Hypothesis 4 stating that environmental disclosure mediates the effect of environmental performance on firm value. Result of mediation test or indirect effect test is presented in Table 4.
In line with the outputs shown in Table 4, it is said that environmental disclosure does not mediate the effect of environmental performance on firm value. There is not enough proof to accept Hypothesis 4 that states that environmental disclosure mediates the effect of environmental performance on firm value. Therefore, Hypothesis 4 is rejected. The question of why environmental disclosure is not mediating the effect of environmental performance on firm value can be answered by the fact that Indonesian companies rarely adopt GRI indicators in their environmental disclosure. As a consequence, the impact of environmental disclosure on firm value is not significant. Based on observations on data from 44 sample companies in this study there were only 13 companies that had implemented environmental disclosures in accordance with GRI’s environmental indicators. Environmental disclosure according to GRI reporting environmental indicators has not yet become the standard format required in Indonesia. Companies can disclose their environmental reports without having to follow the format set by GRI. In addition, company management assumes that following GRI standards is not necessarily beneficial to market value, because something that has not been required by regulation can be ignored by market perception (investors). As Stocken (2000) argues that in the absence of a mechanism to uphold the truth (regulation), voluntary disclosure becomes doubtful and can be ignored by the market. This opinion justifies why the environmental disclosure in this study does not have a significant impact on firm value and does not mediate the effect of environmental performance on firm value.
4.
Conclusion
The current research has conducted empirical tests on the mediation of environmental disclosure to the effect of environmental performance on firm value. Some results were obtained. One is that environmental performance has a positive effect on firm value. This result is supporting stakeholder theory, which states that in maximizing firm value, stakeholder interest should not be ignored. The company must not only maximize economical goal but also the environmental goal (Jensen, 2001). That result is also consistent with RBV theory, which says that environmental responsibility is a way toward competitive advantage and better firm value (Hart, 1997).
Another result indicates that environmental performance has a positive effect on environmental disclosure. This finding proves that companies with good performance have the incentive to disclose their environmental activity to differ themself from companies with bad performance (Verrecchia, 1983; Dye, 1985). Comapnies with good environmental performance will do environmental disclosure in high quality (Kuo and Chen, 2013; Zhang et al., 2014).
The result of the research shows that environmental disclosure does not have a meaningful impact on firm value. Environmental disclosure does not mediate the relationship between environmental performance and firm value. Companies that carry out environmental disclosures with GRI indicators in Indonesia are very low, due to environmental norms that are more normative and do not set by default how large environmental information items the company must disclose. According to Law No. 40 of 2007 paragraph 66 (2c) concerning Limited Liability Companies in Indonesia requires all companies to report on the implementation of corporate social and environmental responsibility in the annual report.
Although environmental disclosure is mandatory disclosure (mandatory disclosure) there are no standard guidelines governing how broad the number of items of information that must be disclosed. Law No. 40 of 2007 is more normative, the interpretation in the application of disclosure of environmental performance reports is very subjective and varied. Based on this the environmental disclosure according to the GRI indicator becomes voluntary. Companies only carry out environmental disclosures based on what is only required in the law (mandatory disclosure) and consideration of economic rationality (Deegan, 2002). Therefore companies that do not adopt environmental disclosure according to the GRI indicator will not receive punishment from the regulation. The results of this study can recommend that governments establish environmental disclosures that are standard and mandatory for all companies. Standard environmental disclosures are useful information for all stakeholders in decision making.
The final results of this study state that Environmental performance can create high firm value directly without the presence of environmental disclosure. The practical implication of this research is that companies with a commitment to be environmentally friendly will obtain an economical benefit in the form of higher firm value.
Limitation
This study has limitations especially in measuring environmental disclosure using GRI environmental indicators resulting in a low environmental disclosure score. These results indicate the still limited number of sample companies in the research period using the GRI indicator. Future research can use other measurement alternatives in measuring company environmental disclosure. Measurement of environmental disclosure using the GRI indicator would be appropriate if many public companies in Indonesia had adopted it and the sample size was adequate.
Conflict of interest
The author declares no conflicts of interest in this paper.









 DownLoad:
DownLoad:



