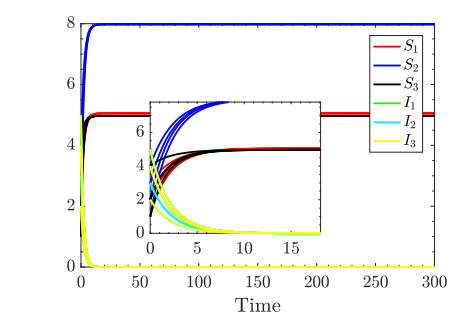
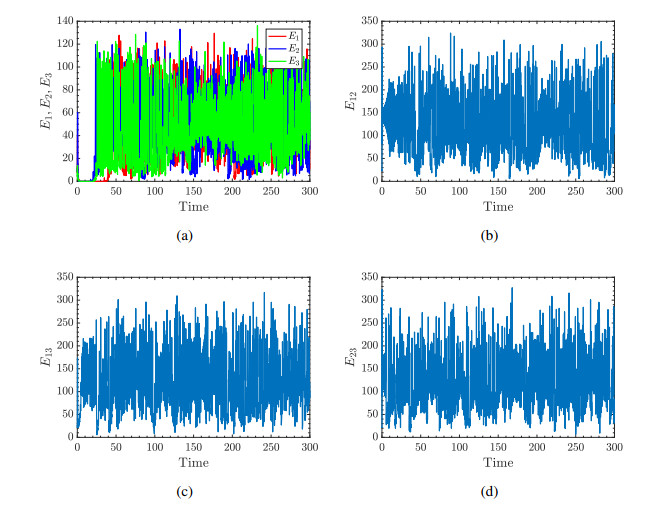
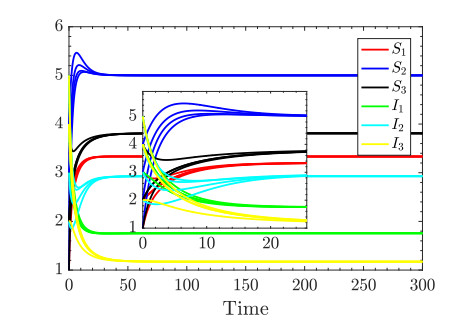
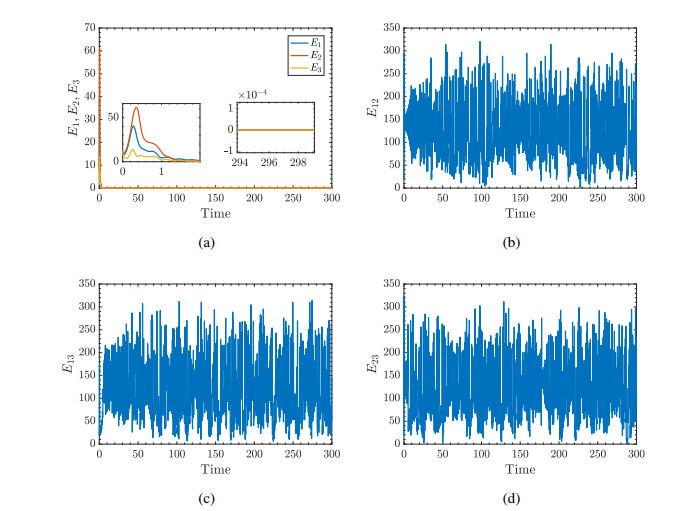
In this paper we investigate cluster collective behaviors aroused by epidemic spread in a patchy population via feedback pinning control strategy. We construct a class of complex network system combined with states feedback behavior synchronization network systems and epidemic spread constant population systems with patch dispersal. In which the dispersal effect among patches are included in the susceptible systems, as well as considering the contact willing function for each patch, determined by the disease's information and individuals' behaviors, as the added cofactor before bilinear incidence. On the one hand, we derive conditions about the global asymptotical stability of disease-free equilibrium, and the existence of positive equilibrium. On the other hand, we design states feedback controllers related with the patch disease information to achieve cluster behaviors synchronization. By using the Lyapunov stability analysis method, the global asymptotical stability of the positive equilibrium is discussed, and meanwhile, the criteria for cluster collective behaviors are obtained. Numerical simulations are performed to support our theoretical results.
1.
Introduction
Recent research on lactic acid bacteria (LAB) has enhanced our understanding of their many advantageous properties; they can be used as biopreservatives, feed grade enzymes, veterinary medicines, health care products and, especially, food and beverage additives [1,2,3,4]. Lactobacilli are a fermentative, facultative anaerobe that is one of the first-evolving groups of bacteria to have beneficial effects for both humans and animals. LAB have been used as bio-additives in ensiling forage to improve fermentation and preserve nutritional content. Silage results from fermentation of a green chopped forage crop and storage of the product with removal of O2 in a silo (ensiling). Ensiled forages contribute 40–60% of all nutrients for livestock animals. The process involves a wide range of factors, including plant cultivation, harvesting and storage practices [5,6]. Lactobacilli play a critical role in preservation of silage nutrient quality through production of organic acids and inhibition of deleterious spoilage from the remaining microbial community. Also, LAB effectively controls secondary fermentation within inoculated forages [7]. In silage, secondary fermentation is an undesirable acidification that is carried out mainly by Enterobacteria, Clostridia (butyric acid producer), and yeasts (ethanol producer).
LAB utilize water-soluble carbohydrates (WSC) and convert them into mixtures of organic acids. The use of LAB in starter cultures can enhance feed quality while minimizing the loss of nutrients (such as carbohydrates, crude proteins, volatile free fatty acids and minerals). The use of selected LAB additives enhances the fermentation process, leading to control of dry matter (DM) loss and pathogenic activity [8]. In silage production, homofermentative LAB are used most broadly due to the high amounts of lactic acid production that occur during fermentation (by e.g: Lactobacillus, Pediococcus, Streptococcus spp., and Enterococcus). Currently, many researchers are using heterofermentative LAB (e.g: Leuconostoc spp. and some Lactobacillus strains) as silage additives with a view towards production of acetic acid and butanol for fuel production [9,10,11].
Silage microflora can be categorized into two main groups, desirable and undesirable organisms. LAB are desirable microbes, while undesirable microorganisms (Enterococcus, yeast and molds) can cause anaerobic or aerobic spoilage during silage fermentation. These undesirable epiphytic microorganisms can decrease the nutritional quality of the silage and also affect animal health and/or milk production [12,13]. Silage additives can be classified into six main groups: homofermentative LAB (hoLAB), heterofermentative LAB (heLAB), customized inoculants (such as hoLAB + heLAB), chemicals, and enzymes. The hoLAB quickly decrease pH and increase lactic acid production in corn, sorghum, and sugarcane silages [14]. Also, the LAB suppresses the growth of undesirable microorganisms and thus reduce proteolysis and DM loss in early fermentation [15]. Blajman et al. [16] reported that LAB inoculation reduces undesirable yeast and mold growth in treated silage, and improves aerobic stability and LAB count in corn silage. However, the effect is highly dependent on the types of inoculants (hoLAB or heLAB) used during silage fermentation. Also, Oliveira et al. [17] explored meta- analysis using silage, LAB species, and silo scale (laboratory or farm-scale) as important factors that determine silage quality.
In recent decades, silage research has driven novel innovations by private companies, which have experimented with silage inoculums, forage harvesters and plastic films for making in silos [5,18]. In addition, recent silage research has addressed the different parameters of the process, including biochemical, microbiological, agronomical and nutritional aspects. The purpose of silage production is to preserve the nutritional value and original energy content of the forage. Energy loss may occur as a result of many factors, such as field loss, harvesting-related loss, secondary metabolism during fermentation of ensilage material, aerobic spoilage and oxidation when the silage is unwrapped to serve to ruminants [19]. Aerobic spoilage is mainly associated with penetration of O2 into the silage during the storage or feeding period. The nutritional composition and feed value of the silage is dependent on the effectiveness of the silage inoculums in the fermentation process. Proper production and management of silage is very important since contaminated or poorly prepared silage can host undesirable organisms, such as Listeria monocytogenes, Bacillus spp., Clostridium spp., Salmonella, Lactococcus and molds [5]. Growth of pathogenic organisms in silage may reduce the safety and quality of the feed and decrease dairy cattle performance, in particular milk and meat production [20,21]. Some concepts necessary to consider when inoculating a silage ball/pack with LAB are exclusion of air, availability of adequate water-soluble carbohydrates, suitable moisture content and the initiation of an early and prompt fermentation [22]. Under appropriate conditions, silage fermentation should preserve over 90% of the harvested energy, sugar, crude protein and metabolites over long storage periods [5]. In this review, we summarize recent information about the functions of LAB strains in silage fermentation as well as their primary fermented products and methods of improving the quality of silage and its shelf life.
2.
Biological properties of LAB
LAB is gram-positive, microaerophilic, non-spore forming, cocci- or rod-shaped bacteria. They consist of different genera, such as Lactobacillus, Leuconostoc, Pediococcus, Streptococcus, Enterococcus and Lactococcus [23]. Classification of LAB into different genera is largely dependent on morphology, action of fermentable carbohydrates, growth at different temperatures, ability to survive under acid or alkaline conditions and production of various organic acids. Though the majority of silage LAB can grow under mesophilic conditions, including different temperatures (20–50 ℃), the optimum temperature for growth is between 25 and 40 ℃. LAB strains are naturally present in various resources, including fermented foods (yogurt, kimchi, meat), forage crops (Italian rye grass, alfalfa, rye, sorghum, corn and triticale), animal manure, dairy products, rumen juice and infant feces [24,25,26]. Some LAB are facultative aerobes, but some have a preference for anaerobic conditions. LAB are able to reduce silage pH to between 3–5 depending on the selected strains and the type of forage crop [27]. The main function of LAB is to ferment WSC to organic substances as their major end product. LAB generally belong to the genera Lactobacillus and Bifidobacterium, which are most widely recognized and used as probiotics in feed development, including dairy foods and beverages. Also, the consumption of probiotics is associated with a wide range of health benefits in humans and livestock animals [28].
3.
LAB as silage additives in livestock feed
In order to improve the quality of silage fermentation, different silage additives have been developed. Recently, biological additives (inoculants) are becoming more widely used as silage preservatives; these can be added to forage in order to increase organic acid production and rapidly decrease silage pH from 6.5 to 3.5. The most important inoculants consist of preferred strains of hoLAB, for example, Lactobacillus spp. (L. plantarum, L. acidophilus,
L. lactis, L. bulgaricus), Enterococcus faecium and Pediococcus spp. (P. pentosaceus, P. acidilactici, P. cellicola); these can produce precise quantities of lactic acid in a short fermentation period and so stabilize the silage with minimal nutritional and DM losses [29,30]. Various factors reduce DM and quality loss during the ensiling steps, including the field and pre-ensiling conditions, temperature, fermentation patterns, moisture content during ensiling, methods and materials used to pack the silage and aerobic deterioration during the feed out phases [31].
In recent years, customized bacterial inoculants have been introduced to enhance organic acid production in silage [32,33]. The primary purpose of these customized inoculants, which include hoLAB strains, is to encourage early fermentation in order to increase the efficiency of fermentation and quickly reduce silage pH. Driehuis et al. [34] LAB inoculated perennial ryegrass with three treatments, L. buch neri alone; L. buchneri with L. plantarum, and P. pentosaceus and non-inoculated silage served as control. The combination inoculants exhibited similar fermentation to the homofermentative L. plantarum and P. pentosaceus treatment over the first 14 days. However, the combination of inoculants and L. buchneri alone resulted in reduced yeast colony counts and improved aerobic stability of treated silage compared with the untreated control during a 90-day fermentation period. Aerobic stability is defined as the length of storage time the silage remains as well‐preserved dry matter content with the lowest amount of yeast and mold spores, even after it is exposed to air. Reports of the use of combination inoculants in various types of silage have been published, with successful outcomes [35].
The production of organic acids by LAB depends on the amount of WSC present in the fresh crop and the precise nature of the bacterial strains used [30,36]. The carbohydrates in the fresh crop are catabolized to produce organic acids and other substances. Table 1 illustrates the effect of LAB inoculation on organic acid production in silage fermentation. Different types of fermentation may take place in the silo environment, mainly depending on the concentration of WSC, volatile free fatty acids, crude proteins, minerals, and moisture in the forage crop and the microbial community present during the ensiling period [17,31].
3.1. Carbohydrate metabolism in silage production
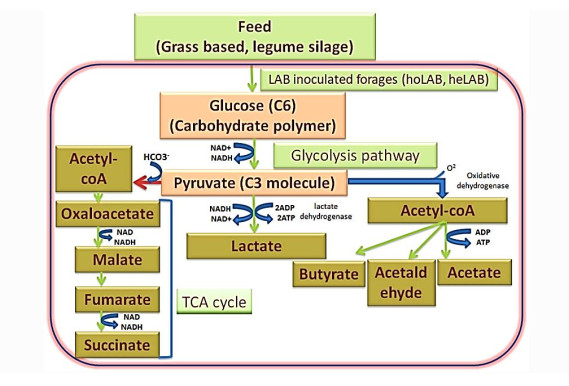
Silage microflora ferment carbohydrates to obtain energy for growth and survival and produce organic acids and other metabolites as end products. In general, silage fermentation utilizes hexoses as the primary substrate. Hexoses are degraded by a different class of silage bacteria and produce organic acids as metabolic end products [46,47,48]. The concentrations of these carbohydrates may vary depending on the type of forage, the time of harvest and environmental conditions [49]. The main pathway used by LAB is glycolysis (Embden-Meyerhoff), in which glucose-containing substrates are catabolized to pyruvate, and acetyl coA is formed as an intermediate product of the Krebs cycle [50,51]. During the ensiling process, both homofermentative and heterofermentative LAB partially hydrolyze hemicellulose to yield pentoses, such as xylose and arabinose, which are fermented into organic acids by the phosphoketolase pathway [52].
There are three main metabolic pathways found in LAB, the homofermentation, heterofermentation and bifidum pathways. Homofermentation converts glucose to lactic acid at a rate that exceeds 80% of the theoretical efficiency. The most abundant organic acid produced by homofermentation is lactic acid. Many LAB strains, such as Lactobacillus, Lactococcus and some Streptococci, carry out homolactic acid fermentation. Very small quantities of formic acid, acetic acid, propionic acid and tartaric acid are also produced as fermentation products (Figure 1). The concentration of other organic acids formed is lower, and negligible for our purposes [53]. LAB uses the EMP (glycolysis) pathway to oxidize one glucose molecule into 2 pyruvate molecules, along with 2 ATP and 2 NADH. Both pyruvates are reduced to lactate by the oxidation of NADH to NAD+ [54,55]. Some LAB strains (e.g: Lactobacillus and Bifidobacterium) utilize the heterolactic acid pathway, in which a phosphoketolase enzyme breaks down a pentose sugar molecule into a 3-carbon phosphate and a 2-carbon phosphate molecule [56,57,58]. As a heterolactic acid fermentation pathway, is mainly initiated by the heLAB, which include Lactobacillus and Leuconostoc sp. The hetero lactic fermentation major end product is ethanol and CO2 in addition to lactic acid. The precursor glucose molecules are first metabolized to pyruvate, acetic acid and CO2 by Pentose phosphate pathway. In addition, heLAB and yeasts use the EMP pathway to synthesize ethanol and CO2, and also to produce diverse gases, fatty acids and alcohols. In LAB, the primary form of reduced coenzymes nicotinamide adenine dinucleotide (NADH) and nicotinamide adenine dinucleotide phosphate (NADPH) greatly affect decomposition of carbon sources and trends of metabolic synthesis, respectively. NADPH is required to provide the reducing power and to promote synthesize cellular components such as amino acids, nucleotides and lipids, among others, which have glucose intermediates, thereby providing the raw materials for further production of lactic acid [54].
3.2. Organic acid production using LAB in silage
Normally, ensiled forage crops contain different organic acids, with the quantity and variety of these acids varying depending on LAB species [22,59]. Jones and Barnes [60] compared several forage varieties and found mostly organic acids, such as malic, citric, and succinic acids, with minor concentrations of fumaric and shikimic acids. Figure 1 schematically illustrates the metabolism of organic acids by LAB during silage fermentation. On the other hand, undesirable microbial fermentation in the silo can produce various end products that alter the nutritive value of the forage. LAB produce high-quality silage and control the formation of undesirable compounds from secondary fermentation which can negatively affect animal performance, the environment, and net farm income [61].
4.
Biological activity of Lactobacillus in silage production
The scientific community has recently been focused on finding novel drugs to inhibit the colonization of multidrug-resistant pathogenic organisms. Some LAB strains can be used as probiotics for ruminants, not only because of the production of lactic acid, but also because they synthesize bacteriocins, bacteriocin-like substances (BLS) and exo-polysaccharides, all of which improve the health of livestock [62,63,64]. Generally, LAB produce different types of antimicrobial compounds that they are safe substances for animal and human. These metabolites which are synthesized from bacterial ribosomes and inactivated by digestive proteases [65,66]. The most common pathogenic microorganisms found in silage are E. coli, Salmonella spp., L. monocytogenes, Clostridium spp. and Bacillus spp.
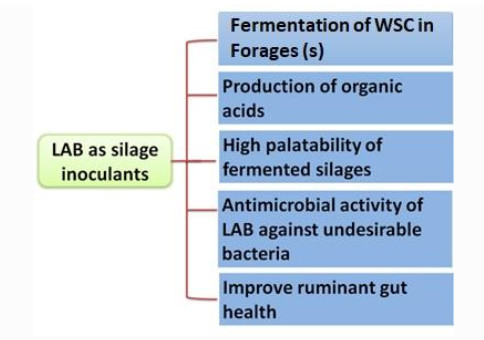
[20]. LAB inoculation of silage rapidly reduces the pH to below 4 and has also been shown to prevent E.coli multiplication in fermented silage [67]. Similarly, Pedroso et al. [68] reported the effectiveness of three combined LAB commercial inoculants at controlling E. coli O157: H7 in corn silages. LAB silage inoculation suppressed pathogen activity within 3 d of ensiling, when the pH dropped below 4.0. The growth inhibition of pathogens in silage was considered to be due to lower pH, and presence of antimicrobial compounds such as organic acids, bacteriocin and BLS in the preserved silages. These metabolites may eliminate the activity of unwanted food-borne pathogens [69]. Similarly, the production of microbial secondary metabolites at lower pH level may also directly reduce the growth of pathogens. Furthermore, pure cultures of L. buchneri and L. plantarum have shown pH-independent antibacterial activity against E. coli O157: H7 [70]. All of these biological additives should be uniformly distributed in the silage for maximum efficiency. It is apparent that to inhibit aerobic spoilage, one must inhibit the growth and activity of microorganisms that cause silage deterioration (i.e. Clostridia, Enterobacteria, molds and yeasts). In addition, the bacteriocin did not affect the other bacterial communities involved in silage fermentation [71]. These were L. lactis CECT 539 and P. acidilactici NRRL B-5627, both of which are bacteriocinogenic strains that have shown antilisterial activity in vitro, and effectiveness for controlling L. monocytogenes in the silo [72]. Figure 2 shows the livestock applications of LAB inoculants in silage production. Gavrilova et al. [73] reported that new LAB strains isolated from clover silage were able to control biofilm formation and inhibit bacterial growth originating from biofilm. In addition, these strains exhibit high organic acid production that completely suppresses the growth of E. coli and S. aureus.
Silage additives contain specific LAB strains, such as L. plantarum, P. acidilacti, P. pentosaceous, and E. faecium, which enhance homolactic fermentation by inhibiting undesirable bacteria or dominating the normal flora and increasing the rate of acidification in preserved forage. The antibacterial effect of LAB cell-free supernatant (CFS) against pathogens increased with lower pH levels, and no antibacterial activity was identified at pH 6.0. The CFS of the LAB strains showed moderate antibacterial effects against pathogenic bacteria, except S. aureus and L. monocytogenes, at pH below 5. [74]. Previously, Ogunade et al. [75] demonstrated that E. coli O157: H7 growth was suppressed in silage extract when the pH was below 4. The low pH in silage was achieved due to rapid production of organic acids by the inoculants. When the pH of the silage falls below 4, lactic acid and acetic acid are primarily in their undissociated form. The cell membranes of yeasts and filamentous fungi are more permeable to the acids in this form. The acid is dissociated (carboxyl cation and hydrogen ion) within the cell, due to the high pH (>6.0), releasing H+ ions, which reduce proliferation and survival of pathogenic microorganisms in the silage [76,77].
When ryegrass was inoculated with L. plantarum, alone and in combination with either L. lactis or P. acidilactici, the DNA band for the spoilage microorganism disappeared after the 5th day of silage fermentation, indicating a synergistic effect of bacteriocin-producing bacteria. This synergistic effect can be attributed to the low pH achieved (<4.0) within 2 d of fermentation when the combination strains were used in the forage [19]. Low pH values stimulate the release of nisin and pediocin from the cell surfaces of L. lactis and P. acidilactici, respectively, and both bacteriocin metabolites exhibit strong antibacterial activity against spoilage bacteria [78]. Amado et al. [72] evaluated the effect of pediocin alone or in combination with a mixture of L. plantarum, E. faecium, and L. buchneri strains against L. monocytogenes. The LAB strains effectively suppressed growth of L. monocytogenes in corn silage via production of pediocin metabolites. Broberg et al. [79] reported that 3-hydroxydecanoic acid (100 µg/mL) inhibited P. roqueforti, Pichia anomala and Aspergillus fumigatus. Also, antifungal activity was seen at pH 4 in the presence of lactic acid (100 mM), 3-hydroxydecanoic acid, 2-hydroxy-4-methylpentanoic acid, benzoic acid and catechol in LAB-inoculated grass silage.
5.
Effect of environmental factors on LAB forage ensiling
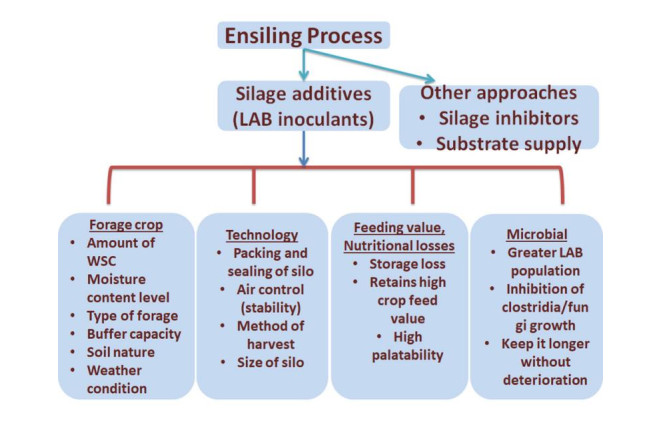
Although some forages are deficient in essential dietary nutrients for ruminants, a wide range of forages crops has been cultivated for livestock animal feeds. The nutritional value of these crops, including crude protein, VFA (volatile free fatty acids), ADF (Acid detergent fiber), NDF (neutral detergent fiber), and DM, can be enhanced by addition of LAB additives at the time of ensiling. Furthermore, biological additives have beneficial effects on silage fermentation due to improvements in the aerobic stability of silage and via control of the growth of pathogenic bacteria [20]. Some crops, like corn and sorghum, have better nutritional value to begin with and good ensiling characteristics. However, high-quality silage production often requires extensive processing, involving harvesting, crop wilting, selection of additives and ensiling steps, which can make production more expensive [80,81]. In general, hoLAB strains are preferable to the heLAB for preserving. However, some heLAB strains are also quite efficient because of their weaker acids production and possibly their higher pKa in heLAB contribute to aerobic stability effect of silage. [82]. Figure 3 shows the various factors which influence silage production. Importantly, Tanizawa et al.
[52] clarified silage fermentation mechanisms from a genomic point of view, especially in cold conditions. Several genes were inactive in the cold condition, but some of the L. vaccinostercus strains, clearly demonstrating the ability to adapt to specific ecological niches. Also, Doi et al. [83] reported that LABs grown at room temperature have a significant capacity to improve the quality of silage. On the other hand, it is not clear whether strains showing strong lactic acid production can dominate butyric acid bacteria or coliform bacteria at higher temperatures (>37 ℃). Different processes have been established to improve the fermentation quality and reduce dry matter losses of whole-crop silages of paddy rice, legumes and grasses [84,85]. Overall, biological silage additive-induced hoLAB fermentation is more suitable to crop silage than other types of fermentation because it leads to greater dry matter recovery and improved fermentation end products [86]. LAB inoculated forages did not alter biochemical and nutritional components and it could preserve its quality of silage in terms of crude protein (CP) content, ADF (acid detergent fiber), NDF (neutral detergent fiber), and TDF (total detergent fiber). In addition, the nutrient values are nothing but the quality of the silage such as organic acids, crude protein content, ADF (acid detergent fiber), NDF (neutral detergent fiber), and TDF (total detergent fiber). Although, the organic acids levels and pH can reflect quality of fermentation and silage mass stability. The low pH shows the silage is stable and cannot further develop undesirable microbes too in the in-silo.
6.
Advantage of LAB use in livestock industries
The diversity and function of LAB microbes present in a wide range of fermented foods that improve the nutritional value of fermented foods is now well‐appreciated [87]. The fermented functional foods have presence of live microorganisms inclusing different bioactive molecules, vitamins other constituents during the fermentation that enhanced safety, functionality, sensory, and nutritional properties of silage product [88]. Table 2 shows the merits of LAB inoculants in silage production. Also, the lactic acid bacteria ensiling benefits and uses of chemical additives were discussed.
Ruminal microbiota plays a vital role in the conversion of lignocellulose-rich plants into nutrients for ruminants. It has been proposed that different genera, such as bacteria, fungi, and protozoa, hydrolyze complex flavonoid glycosides to the corresponding less polar aglycones prior to gastrointestinal absorption [89].There is a direct relationship between rumen pH and bacterial community, with significant reductions in bacteria at pH values around 6.8. Table 3 shows the degradation of different plant secondary metabolites by LAB in rumen fluid. In LAB-inoculated potato with wheat straw silage, the chemical composition, silage fermentation characteristics, and in vitro gas production were improved after anaerobic storage for 90 d, compared to untreated silage [90]. Contreras-Govea et al. [7] found that, in ruminal in vitro fermentation, gas and VFA production were not significantly different between non-inoculated and inoculated silage, although the L. plantarum MTD/1-inoculated model produced greater microbial populations than were seen in non-inoculated silage.
Those authors suggested that enhanced protein preservation during ensiling with a silage inoculant could be one of the reasons behind disturbances in ruminal fermentation. Zhang et al. [32] evaluated the effects of Lactobacillus rhamnosus GG on growth performance and hepatotoxicity in calves fed a single dose of aflatoxin B1 (AFB1). L. rhamnosus GG administration reduced the concentrations of free AFB1 and AFM1 in rumen fluid and reduced the release of toxins into plasma and urine. Also, Goel et al. [92] reported that even typical hydrolysable tannins are toxic to both ruminants and monogastrics, particularly when present in the forage of such animals in excessive amounts. However, ruminants have developed an adaptation that allows degradation of tannins to simple isomers via the microbial ecosystem and subsequent excretion in feces.
6.
Conclusion
LAB inoculants improve silage quality and reduce DM losses under long-term storage. LAB is one of best organic additives that allow for precise control over undesirable bacterial growth in silage. LAB inoculants utilize water-soluble carbohydrates and complex secondary metabolites in forage crops into organic acids and mineral acids, which effectively control pathogenic growth and enhance the nutritional quality of silage for livestock animals. However, organic acid production and its associated biochemical mechanisms differ depending on the moisture content of forage crops and the storage period. Many studies have been done to investigate the effect of different temperature conditions, harvesting times, moisture levels on forage ensiling quality. In addition, further investigation into the optimal fermentation duration for various kinds of forage crops must be considered, and the effect of LAB additives on fermentation must be investigated, with the overall goal being to save time and cost and to minimize nutrient losses.
Glossary
Forage
Forage is defined as feed for domestic and/or livestock animals.
Silage
Silage is feedstuff prepared from grass or other green crops (such as corn, wheat, sorghum, legumes, grass and triticale) that has been cut and preserved through fermentation under anaerobic conditions in a silo. Purpose, to provide winter feed for livestock animals.
Ensiling
Ensiling is the name given to the process of preparing and storing forage in order to induce conversion to silage in a packed enclosure (silo).
Silage additives (inoculums)
The purpose of using silage additives is to enhance feed quality by encouraging lactic acid fermentation and inhibiting pathogenic microbial growth and by improving its nutritional value. The silage additives may include bacterial cultures, inhibitors of aerobic damage, acids, and nutrients.
Homofermentative and heterofermentative
LAB can be classified into two main groups. Homofermentative LAB catabolize glucose into lactic acid, while heterofermentative LAB convert glucose into ethanol and CO2 as well as organic acids.
Dry matter
Dry matter refers to foodstuff that remains after removal of water, and the moisture content reflects the amount of water present in the feed ingredient.
Livestock
Livestock commonly refers to animals, such as cattle, pigs, sheep, horses and goats that are kept on a farm for commodity and profit.
Fermented feedstuff
Fermented feedstuff is formed by probiotic microorganisms and mainly encompasses agricultural end products. Fermented feedstuffs contain essential nutrition and gut microbiota because they contain inoculating microorganisms.
Buffering capacity
Silage resistance to pH lowering is called buffering capacity.
Plant respiration
The immediate stage of ensiling involves plant cell respiration and oxidation of WSC (hexose). This is also called the aerobic phase because it can only take place when O2 is present in a silo. For example, the biochemical reaction that occurs when plant respiration happens in a silo is:
C6H12O6 + 6O2→6CO2
+ 6H2O + heat energy
Acknowledgments
We acknowledged Cooperative Research Program for Agriculture Science and Technology Development supported funds for this research work (Project No. PJ01358902). The project titled "technique development for manufacture of high-quality legume silage" sponsored by RDA, Korea. This study was also supported by the Postdoctoral Fellowship Program of the National Institute of Animal Science funded by RDA, Korea.
Conflicts of interest
We declare no conflicts in this work.









 DownLoad:
DownLoad: