1.
Introduction
1) Technological growth
To confront globalization, expanded item complexity, and increased demand, it is essential for companies to take up up-gradation in technology to convert their supply chain (SC) from a traditional one to a smart one. Utilizing Radio-Frequency Identifier (RFID) and a fast web network, forward-thinking companies can collect information at each checkpoint, from the status of crude materials stream to the location and condition of wrapped-up products. Artificial intelligence (AI), machine learning, and progressed autonomation convey experiences that advance efficiencies by making on-the-fly route changes to quicken item conveyance. Many researchers in modern SC models apply these progressed technologies. For illustration, numerous firms are using the "internet of things" (IoT) to offer proactive support for maintenance that ensures the service for the product. In the competitive market, up-gradation of the production house and warehouse is essential; otherwise, that industry must be losing its place in the market [1]. Using innovative technology like drone transportation, drone inspection, RFID tag, RFID scanner, RFID signal receiver, Bar code reader (BCR), QR code reader, Computers, Close circuit camera system, WIFI and networking, conveyor belts, air conditioning, a traditional manufacturing house, and warehouse can be made a smart one [2]. Innovative technology can prevent the inaccurate demand problem due to unreliable retailers in his study [3]. Recently, Tseng et al. [4] discussed the data analysis regarding the challenges and changes for industry 4.0 regarding technological growth.
2) Flexible production rate
A flexible manufacturing system (FMS) is a planned production strategy to effectively adjust to changes in the quality and amount of the item being produced. In general, manufacturers favor making items with culminating quality. But quality depends on various facts such as the crude material, breakdown of machines, the inefficiency of skilled labor, and long-run production system. A production system may create imperfect things ceaselessly for these components, which should be settled to stay within the competitive market. Along with the technological growth, by applying a flexible production rate, the industry has mechanically up-grade the traditional production system to an intelligent production system [5]. Flexible production can help to improve the quality of the produced defective product even in a out-of-control stage of the production system [6]. For that, the flexible production rate is also imperative for an SC.
3) Smart produce
Industry 4.0 has driven world-view shifts and changes for arranging and upgrading production processes. This significant change influences not the integration of the technical and physical situations but, most critically, the connections between customers and the production house. To effectively grasp this up-gradation and stand up to the various challenges, production houses ought to adapt to the requirements for innovative technical progression and give feasible preparation and instruction for their workforce. The industry directors ought to take under consideration the human-in-the-loop for this technical up-gradation challenges and also consider a 3I (Interaction, Intellect, and Interface) angle for manufacturing plants to extend the habitation of smart production using technologies [7]. Smart produce strategy points to include information on production technologies, explains suitable ways for customers to know the insights of technologies used for a production system, and targets the collaboration between customers and the production system. Again for an unreliable production system, it is essential for smart production to upgrade the traditional production into a smart one by implementing innovative technology [8], which helps to prevent the shortage problem due to the unreliability of the production system. In a smart production system, the inspection process can be controlled by automated machines which remove the defective items produced by the production house and move those items for remanufacturing [9]. For all the above reasons, smart production is essential for an SC.
4) Transportation policy
Transportation is the major fundamental component of an SC. It includes the conveyance of items from the beginning to the conclusion of an SC. It involves shipping an item from a provider to a client or a warehouse through roadways, railways, pipelines, airways, or waterways. Transportation is habitually utilized within the SC facilitation. Implementation of an efficient transportation strategy is essential for an SC to make it successful. A suitable transportation strategy may be utilized to diminish the SC's total cost, upgrade client service, smooth shipments within the SC, synchronize with smart technologies of the SC, give more flexibility in the SC, bypass geological confinements, and boost the economy of the company. Therefore transportation policies should implement in SC for reducing transportation time [10], transportation cost [11], take care about social commitment and logistic challenges [12]. Some authors applied strategies like single-setup-single-delivery (SSSD) policy [13], single-setup-multi-delivery (SSMD) [14], single-setup-multi-unequal-delivery (SSMUD) [15], single-setup-multi-unequal-increasing-delivery (SSMUID) [16] for smooth functioning of the transportation system.
5) Unreliability
Nowadays, an increasing headache for industry managers is the unreliability of players. In a traditional SC, players are honest and do not hide any information from other players. Still, in today's environment, players of an SC are opportunistic and hide information from other players. As a result, the industry lost the demand for its product, lost its reputation, lost revenue, and got in trouble for smooth conduction. So, it's a challenge for the industry managers to solve the unreliability player problem and improve the performance of the SC. The unreliable player may be the retailer [17], supplier [18] may be the manufacturer [19] or maybe the buyer [13]. The unreliable manufacturers often hide the number of manufactured products from the retailers and produce less than the retailer's ordered quantity. Because of this reason, a shortage arose, which caused lost sales (some are backlogged due to the good reputation of the retailers and the product). Also, the unreliable retailer hides actual sales information from the manufacturer, making it difficult to estimate the manufacturer accurately. The raw material quality of the manufacturer is so good. Thus, instead of the unreliability of the manufacturer, the retailers don't want to lose that manufacturer.
1.1. Objective and motivation of this study
This study's main objectives are to solve the retailer's shortage problem due to the manufacturer's unreliability and inaccurate estimation problem of the manufacturer due to unreliability of the retailers by using smart warehouses and implementing technological development for both players. Thus in this study, the technological development is conducted using smart technologies like RFID readers and tags, CCTV, web, and LAN.
Due to a smart manufacturing system, a flexible production system is required, which reduces the manufacturing cost of the manufacturer and increases labor efficiency, improves machine productivity, upgrades the system's reliability, enhances the quality, and shorter lead times, boosts production.
In the traditional SC model, it is assumed that the produced items are always of good quality. But in reality, due to the long-run process and other factors, the production system may go to an out-of-control stage for which continuously defective items are produced. By smooth produce, minimize the number of faulty items build in an imperfect production system.
Take into account the improvement of environmental sustainability, implement a suitable transportation strategy (SSMUID policy) to reduce the total cost of the SC and solve the shortage problem for the retailer.
1.2. Orientation
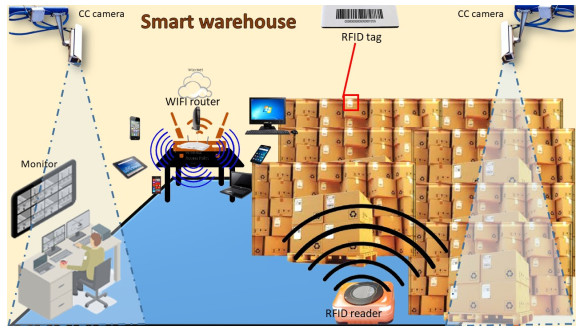
Finally, with proper examples and representation, minimize the total cost of the SC with suitable optimization techniques and explain the optimization. A sketch of a smart warehouse is shown in Figure 1. A brief introduction is described in Section 1 whereas some previous work on this field is illustrated in Section 2. In Section 3, all notations and assumptions used throughout the study are given, and the problem is explained. The model is formulated in Section 4. The solution process of the model is interpreted in Section 5 and the model is analyzed with a numerical example and sensitivity analysis. The conclusion, future extensions of the model, and the managerial insights are explored in Section 8 and Section 7.
2.
Literature review
An SC is a network among various players like manufacturers, suppliers, retailers, buyers, transporters, and third-party logistics to manufacture and encase a specific item to the final player, including different actions, persons, organizations, particulars, and assets. It deals with the flow of products and services and covers the whole system that converts the raw product into final material. Several SC management models have started from Scarf [20] to the date where Pal and Adhikari [21] gave a recent study that described the hazards problem of machines in a sustainable SC with multi-retailers, variable demand, flexible production rate and. A multi-echelon stochastic sustainable closed-loop SC with Multi-objective optimization for multi-echelon, multi-product was recently discussed by Elfarouk et al. [22].
In the present marketing trends, a customer wants quick and on-time delivery. In the traditional SC, players of an SC are honest and provide appropriate information. But in today's marketing environment, players are opportunistic and hide sales information from the other players to increase their profits. The industry managers cannot provide good customer service for this kind of unreliability of players and cannot ensure the smooth functioning of the SC. Companies adopt intelligent technologies and upgrade their traditional production house into a smart production houses for that purpose. Also, transportation plays a vital role in an SC. Delivery of the whole produced unit to the retailer in a cycle is not beneficial to the industry as it increases the holding cost of the retailer. So to reduce the retailer's holding cost, the single setup multiple unequal increasing delivery policy, which is applied in this study, is necessary.
Chen et al. [23] studied unreliable channels whereas Entezaminia et al. [24] discussed about unreliable manufacturing system and Romero-Silva et al. [25] unequal processing time, merging line performance. In the study of Sardar et al. [3], and Sardar and Sarkar [26] the retailer of the SC is unreliable and hide sales information from the manufacturer, whereas in the study of Guchhait et al. [13], buyers are not reliable. Uncertain cost of the product is considered in the study of Sarkar et al. [27], and Hota et al. [16] consider unreliable manufacturer in their study. Park and Lee [28] considered multiple unreliable suppliers. Recently, Hota et al. [57] solved a problem due to transportation hazards in an SC with an unreliable manufacturer. But none of these authors considered applying smart technology in an SC with unreliable manufacturers who adopt the SSMUID policy. So there is a gap in research that is fulfilled in this study.
To upgrade a traditional production house into a smart production house, one of the essential items needed is RFID. RFID gives an accurate estimation of the number of produced items and which helps to prevent shortage problems. The reusable RFID tags are scanned automatically by an RFID scanner, and the industrial managers get the accurate number of produced items through online mode. The implementation of the RFID technology to control the unreliability problem and maximize the SCM's profit was described by Guchhait et al. [13]. In contrast, Ullah and Sarkar [29] applied RFID to increase the recycling rate of electronic products. Sardar et al. [3] used RFID to follow the movement of the product, and RFID was used by Sardar and Sarkar [26] in shops to get information about the exact amount of demand for the product among customers. None of these authors applied RFID to a production house to get the accurate number of produced items. In this study, a technological growth cost is used to install RFID scanners, RFID tags, internet, and other intelligent technologies to upgrade the production house into a smart one and prevent inaccurate estimation of produced items due to unreliability of the manufacturer.
Dalenogare et al. [30], and Wang et al. [31] about the technologies of industry 4.0, which can be used as a part of a smart manufacturing system. Chen et al. [32] gave a brief discussion about the smart production house, while Different types of technologies applied for upgrading a production house into a smart one in industry 4.0 were proposed by Frank et al. [1]. Bueno et al. [2] explained how smart production houses improved the technologies of industry 4.0 to reach smart production planning and control. In contrast, Bueno et al. [2] explained how industries 4.0 technologies assist the control process and production planning by using artificial intelligence to enhance the production activity. In the smart production system of Dey et al. [33] an automated system was installed to deeply inspect the produced items and reject the defective items without any error. Bhuniya et al. [34] smart production system manufactured smart products at the least possible cost with saving energy. The study by Sarkar et al. [5] deals with how a smart production system can control the carbon footprint, obtaining the net present value of products for the four sub-systems associated with the logistics system. Sarkar et al. [9] got the net current worth of the product and controlled the carbon footprint by a smart production system. Sarkar and Sarkar [35] optimizes the ramification of production size, production rate, and safety stocks underutilization of energy in a smart production system. But none of them considered transportation policies in their models, which plays an essential role in SCM. This study fulfills this research gap by applying a suitable transportation policy named SSMUID policy.
The holding cost of the Retailer is practically greater than the holding cost of the manufacturer because the production houses are usually situated in rural areas. In contrast, the retail houses are situated in towns or cities or metro cities. So transporting the whole manufactured amount in a single lot, the SSSD (single-setup-single-delivery) policy is not scientific, increasing the manufacturer's holding cost. Goyal [36] upgrades the SSSD policy by dividing the delivery of the whole produced quantity into a finite number of lots called SSMD (single-setup-multiple-delivery) policy. Some authors considered that the lot sizes of each lot are equal [14], but the demand for the product may not always be fixed, so delivering an equal amount in each lot may not be scientific. Hoque [37] considered equal/unequal shipment size in his study but Hariga et al. [38] consider only unequal shipment size in their studies. Sarkar et al. [15] introduced the SSMUD (single-setup-multiple-unequal-deliver) policy in which the lot sizes of each lot differed. Hota et al. [16] introduced the SSMUID (single-setup-multiple-unequal-increasing) policy in which the lot size of each lot is a multiple of the previous by a fixed multiplier for items of increasing demand. But none of the authors consider SSMUID policy in a smart SC with unreliable players.
Based on the above discussion following research questions are raised:
2.1. Research questions and objective
● In the literature, several SC models were discussed about reliable player [39,40], however unreliable players where manufacturer and retailer both are unreliable still a questionable research direction. In this research, we try to fulfill the research gap.
● There were many SC model developed under the consideration of different transportation policy, like SSSD [41], SSMD [42], however SSMUID transportation policy in an unreliable SC system is very rare [16]. Thus in this research study, an attempt is made to fill this gap.
● Many SC models were constructed under the consideration of distribution robust approach [43]. But utilization of some smart technology in the SC model along with the metaheuristic solution approach is still a big research gap in the SC research direction. A pioneering attempt is made in this study to fill this gap.
2.2. Contribution
Finally, the research contribution can be summaries as the shortage problem of a smart SC with a single unreliable manufacturer and unreliable multi retailers is solved in the study by applying innovative technology and SSMUID policy. For smooth conduction of the SC, a smart product house, variable transportation cost, material-dependent product cost, and transportation are applied in this study. In contrast, electricity consumption-dependent carbon emission costs are involved for enhanced environmental sustainability. Since the mean and the standard deviation of the random variable associated with the lead time demand are unknown, a robust distribution approach is applied to solve the model. For the presence of multiple retailers, a metaheuristic solution approach is utilized, and the model is solved numerically by the two popular methods, GA and PSO. Also, the result of the two methods are compared to find the most beneficial method, and four special cases are explained for the reality of the study. A summary of the literature is given in Table 1.
3.
Notation, assumption, and problem definition
3.1. Notation
3.2. Assumptions
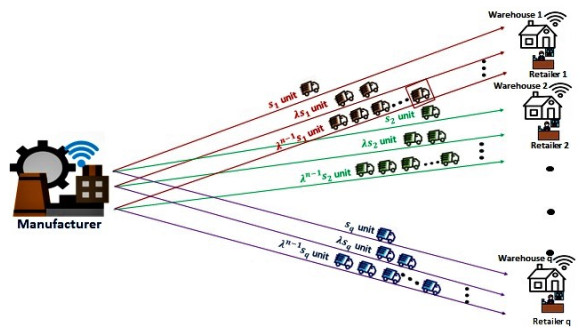
1. A SC with a single manufacturer, $ q $ retailers, and a single type of products are studied ([48]). Both the manufacturer and retailers are unreliable. The production house and warehouse are equipped with the latest technologies and upgraded from a traditional one to a smart one.
2. The manufacturer is unreliable who produced $ \alpha Q $ unit of items $ {\textbf{Q}} = \left(Q_1, Q_2, ..., Q_q\right) $ is a row vector the ordered quantity of the two retailers and $ \alpha = \left(\alpha_1, \alpha_2, ..., \alpha_q \right) $ is another row vector where $ 0 < \alpha_i < 1 $ and $ \alpha Q = \sum_{j = 1}^{q}\alpha_j Q_j $ ([28]).
3. The manufacturer produces $ \alpha Q $ quantity at a time that is less than the total order amount. Still, to save the holding costs of the retailer, the manufacturer delivers these products in $ n $ lots, and the lot sizes are increased by a multiplier in each lot. For $ j^{th} $ retailer the first lot size is $ s_j $ unit, second lot size is $ \lambda s_j $ unit, ......, the $ n^\rm{th} $ lot size is $ \lambda^{n-1} s_j $ where $ j = 1, 2, ..., q $. Therefore $ \alpha Q = \sum_{j = 1}^{q}{ \left[ s_j + \lambda s_j +... +\lambda^{n-1} s_j \right]} = \frac{\lambda^n -1}{\lambda - 1} \sum_{j = 1}^{q} s_j = \frac{\lambda^n -1}{\lambda - 1} S $ ([16]).
4. To upgrade the traditional warehouses into smart warehouses and solve the unreliable retailer problem by proper inspection, the manufacturer initiated a technological up-gradation in the warehouses of the retailer and production house. The costs associated with this technology include installing and maintaining RFID tags and scanners, CCTV, WIFI and internet, and other expenses related to the technical up gradation. In the smart manufacturing house, all RFID scanners [13], RFID tag, CC Camera, and WIFI router are symmetric. The lenses of the CC cameras are rectangular.
5. A flexible production rate with production cost depending on smart tool cost is applied to upgrade the traditional manufacturing house into a smart manufacturing house. The unit production cost $ C_P = C_m+\frac{C_D}{P}+\tau P $ where $ C_D = A + B e^{k\left(\frac{\theta_{max}-\theta}{\theta-\theta_{min}}\right)} $; $ \theta $ is the designed reliability defined as $ \theta = \frac{\rm{Number \; of\; failures}}{\rm{Total\; units\; of\; used \; hours}} $ and a $ \theta \in \left[\theta_{min}, \theta_{max} \right] $, $ A $ is the fixed cost of manufacturing, $ B $ is the resource cost when $ \theta = \theta_{max} $, $ k $ is a constant representing the difficulties in the increasing reliability, $ \tau $ is the tool cost and $ C_m $ is the material cost ([46]).
3.3. Problem definition
$ q $ unreliable retailers orders $ Q = \left(Q_1, Q_2, ..., Q_q \right) $ units of products to the unreliable manufacturer, and the manufacturer produces $ \alpha Q $ quantity of units (Figure 2). Therefore, shortages arise, shortages are partially backlogged and reorder points of the retailers are variables.
The manufacturer uses a smart manufacturing house and a technological growth designed to set up the smart manufacturing house. The retailers have a smart warehouse, and the inventory level goes down due to demand. Due to the unreliability of the manufacturer, the retailers receive less quantity of items of the ordered quantity. The lead time demand is found as a random variable and the expected shortages of the $ j^{th} $ retailer is $ E\left(X_j-r_j\right)^+ $ where $ r_j $ are the re-order points of the $ j^{th} $ retailer. Thus, the number of backorder and lost sales of $ j^{th} $ retailer are $ \beta_j E\left(X_j - r_j\right)^+ $ and $ \sum_{j = 1}^{q} \left(1 - \beta_j\right) E\left(X_j-r_j\right)^+ $, respectively, where $ 0 < \beta_j < 1, j = 1, 2, ..., q $ are rate of backorders of the $ j^{th} $ retailers.
4.
Formulation of the model
4.1. Mathematical model of the manufacturer
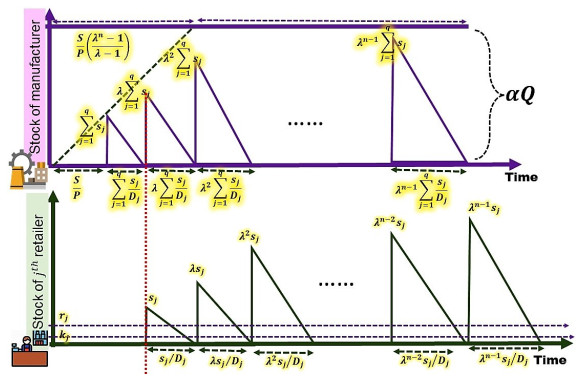
The inventory position of the manufacturer is described in Figure 3. By Assumption 2, the number of production cycle is $ \frac{\sum_{j = 1}^q {D_j}}{\alpha Q} $, i.e. $ \frac{D}{\frac{\lambda^n - 1}{\lambda - 1} S}, $.
The manufacturer introduces smart technology like RFID tags, RFID scanners, CCTV, air conditioning, and others to make the smart manufacturing house. Thus, there are some extra costs due to this up-gradation.
The average inventory of the manufacturer is
The costs of the manufacturer are given below:
4.1.1. Technological growth cost
As Assumption ???, to prevent the unreliability of the retailer, the manufacturer installs RFID tags and scanners, CCTV, and internet in warehouses and upgrades them from traditional to smart ones. The total cost for technological growth per cycle is given by
TGCM = RFID tag cost + RFID scanner cost + CCTV system cost + Internet connectivity cost + Other technological growth cost
subject to the condition $ d \leq \sqrt{3} \left(\mu - \frac{Log \left[1 - \sqrt[3]{1 - \phi} \right]}{\rho} \right) $ and $ S_t \geq 2 S_s $.
See Appendix 9 for a detailed calculation of RFID tag cost, RFID scanner cost, CCTV system cost, Internet connectivity cost, and Other technological growth costs.
4.1.2. Production cost
As per assumption 5 the unit production cost $ C_P = \left[ C_m + \frac{C_D}{P} + \tau P \right] \alpha Q $. Therefore the production cost of the manufacturer is $ \frac{D}{\alpha Q} C_P $, that is
4.1.3. Set up cost of manufacturer
Since the cycle length of the manufacturer is $ \frac{\alpha Q}{D} $, and the setup cost is $ C_{set} $, the setup cost of the manufacturer per cycle is given by
4.1.4. Holding cost of manufacturer
The average holding inventory as calculated in equation no 4.1 is $ \Upsilon_A $, and the manufacturer's holding cost per unit item is $ C_{hm} $. Therefore the holding cost per cycle of the manufacturer is as follows.
4.1.5. Carbon emission and Transportation cost of the manufacturer
Due to the increasing number of transportation besides a fixed carbon emission and transportation cost, consumed fuel-dependent carbon emission and transportation cost and consumed electric-dependent carbon emission cost are considered ([45]). The fixed transportation cost of the manufacturer is
The variable transportation cost of the manufacturer is
where $ g_{0j} $ is the fuel consumption of the unloaded (empty) vehicle when returning from $ j^{th} $ retailer, and $ g_{1_j} $ is the unit fuel consumption factor when the vehicle is loaded with goods for $ j^{th} $ retailer per unit of goods.
Again the fixed carbon emission cost of the manufacturer is
The variable carbon emission cost has two components, the 1st one is for transportation, and the 2nd one is for electricity consumption in the manufacturing house. In a similar way, the carbon emission cost due to transportation is
and the carbon emission cost for electricity consumption in the warehouse is
where $ \omega_1 $ and $ \omega_2 $ are carbon emission factors for fuel and electricity and $ w_0, w_1 $ are fixed and variable electricity consumption per time unit for storage. Therefore, the total carbon emission and transportation cost of the manufacturer is [ see Tang et al. [45]]
4.1.6. Total cost of the manufacturer
Thus the total cost of the manufacturer is
subject to the condition $ d \leq \sqrt{3} \left(\mu - \frac{Log \left[1 - \sqrt[3]{1 - \phi} \right]}{\rho} \right) $ and $ S_t \geq 2 S_s $ and $ j = 1, 2, ..., q $.
4.2. Mathematical model of the retailer
Figure 3 is the figure of the $ j^{th} $ retailer. $ q $ retailers of this model has $ q $ smart warehouse. The inventory decreases due to demand only. The production batch transported form manufacturer to $ j^{th} $ retailer is
Thus, according to Sarkar et al. [15] the number of production cycle of $ j^{th} $ retailer is given by
Again, from figure 3 the total inventory of the $ j^{th} $ retailer is
Thus, the total inventory of retailers is
4.2.1. Ordering cost
The ordering cost of the retailer is
4.2.2. Annual stock out cost of the retailer
Since the manufacturer is unreliable and delivers less ordered products to the retailer, there must be a shortage. When shortages arise, customers are moved to other retailers to purchase the product and lose sales. But in reality, because of the retailer's good reputation, some of them wait until replenishment of the product. So their backlogging is partial. Retailers offer price discounts $ \pi_{x_j} $ for customers to reduce the lost sales. According to Lin [49] total annual stockout cost of the retailers is
where $ \beta_j = \frac{\beta_{0_j}\pi_{x_j}}{\pi_{0_j}} $ be the backorder rate and $ \beta_{0_j} $ be the upper bound of backorder ratio and $ \pi_{0_j} $ be the marginal profit per unit of the $ j^{th} $ retailer and $ 0\leq \beta_{0_j} < 1 $, $ 0\leq \pi_{x_j} \leq \pi_{0_j} $.
4.2.3. Holding cost of the retailer
Units of holding items of $ j^{th} $ retailer is
for Total holding cost of the retailer is
where $ 0\leq \beta_{0_j} < 1 $, $ 0\leq \pi_{x_j} \leq \pi_{0_j} $.
4.2.4. Total cost of the retailer
Thus the total cost of the retailer is
where $ j = 1, 2, ..., q $ and $ 0\leq \beta_{0_j} < 1 $, $ 0\leq \pi_{x_j} \leq \pi_{0_j} $.
4.3. Joint total cost of the supply chain
The joint total cost of the SC is
subject to the condition $ d \leq \sqrt{3} \left(\mu - \frac{Log \left[1 - \sqrt[3]{1 - \phi} \right]}{\rho} \right) $, $ S_t \geq 2 S_s $, $ 0\leq \beta_{0_j} < 1 $, $ 0\leq \pi_{x_j} \leq \pi_{0_j} $ and $ j = 1, 2, ..., q $.
5.
Solution methodology
The Kuhn-Tucker (KT) method is applied to find the optimal result. The manufacturer's cost function constraints are modified and transferred into an unconstrained function by introducing two multipliers $ \zeta_1 $ and $ \zeta_2 $. Again the uncertain demands $ D_i $ of the retailers are represented by random variables with mean $ D_j L_j $ and standard deviation $ \sigma_j \sqrt{L_j} $. Then according to the lemma of Moon and Choi [50], the shortage amount is
and $ R_j = D_j L_j + k_j \sigma_j \sqrt{L_j} $. Applying the KT-multipliers and
where $ 0\leq \beta_{0_j} < 1 $, $ 0\leq \pi_{x_j} \leq \pi_{0_j} $.
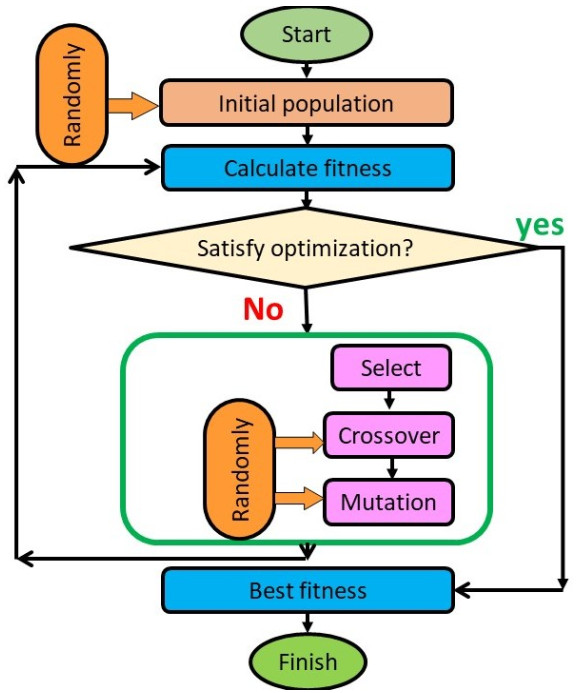
5.1. Application of genetic algorithm (GA)
Presently, it is essential to illuminate the optimization issue of the cost function with a suitable strategy. In this, the optimization issue could be a mixed-integer nonlinear programming problem. For fathoming these issues, a Real Coded Genetic Algorithm (RCGA) is created for continuous and integer variables. Most probably, GA is the widely applied and most popular algorithm for optimization. The working rule of the implemented RCGA is described in the algorithm given below:
Step 1: Set the initial values of the parameters of RCGA, bounds of the decision variables, and the values of the cost and other parameters of the problem;
Step 2: $ \varsigma\rightarrow0 $ (where $ \varsigma $ represents the number of current iteration/generation);
Step 3: Initialize the chromosomes of populace P($ \varsigma $) where P($ \varsigma $) implies the $ \varsigma $'th stage iteration/generation;
Step 4: The fitness function $ P(\varsigma) $ is to be evaluate for each chromosome of $ P(\varsigma) $.
Step 5: According to the fitness value of each chromosome, Trace out the ideal chromosome from $ P(\varsigma) $;
Step 6: Repeat the steps given below until the optimal condition is satisfied:
1. increase $ \varsigma $ by $ 1 $, ($ \varsigma →
\varsigma+1 $);
2. Upgrade the old population to a new one;
3. Improve the existing population by mutation and crossover operations;
4. Find the fitness of every chromosome of $ P(\varsigma) $;
5. Find the fittest chromosome;
6. Find the best chromosome among the current $ (P(\varsigma)) $ and previous $ (P(\varsigma-1)) $ population and save the better value.
Step 7: Print the best solution;
Step 8: End
Various parameters, like population size, probability of crossover, the maximum number of generations, and probability of mutation, are used throughout GA. There's no particular rule for the choice of population size, and however, for a large population size, some problems may arise in GA. Again GA cannot work for a small population size. Also, there is no proper rule for estimating the maximum number of generations. The probability of crossover can't be less than the probability of mutation. The most important task for the smooth running of GA is to design an appropriate chromosome of solutions.
For more explanation, a flow chart of GA is described in Figure 4.
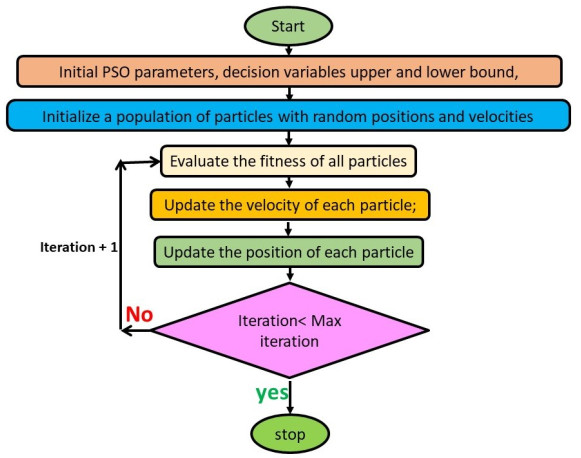
5.2. Application of particle swam optimization (PSO)
Particle Swarm Optimization (PSO) is a population-based worldwide optimization algorithm built on social interaction and personal experience. This calculation is motivated by the social behavior of flocking of birds or schooling of fish. The search strategy of PSO can be summarized as bellow:
Step 1: Initialize the parameters of PSO and decision variable's upper and lower bounds of the of the problem;
Step 2: Initialize particles population with random velocity and positions;
Step 3: Find the particles fitness;
Step 4: Invigilate the highest fitness locations;
Step 5: Invigilate the global maximum fitness position;
Step 6: Update each particle's velocity;
Step 7: Update each particle's position;
Step 8: Go to Step 9 if the optimal condition satisfied, otherwise go to step 3;
Step 9: Print the result;
Step 10: End
For more explanation, a flow chart of PSO is described in Figure 5.
6.
Numerical examples
A numerical example is explained with the following data to demonstrate the proposed study.
Values of the parameters
$ q = 3 $, $ D_1 = 400 $ unit, $ D_2 = 450 $ unit, $ D_3 = 105 $ unit, $ \eta_1 = 4 $ unit, $ C_{rft} = 25 $ $/unit, $ S_t = 120 $ m, $ S_s = 60 $ m, $ C_{rfs1} = 300 $ $/unit, $ C_{rfs2} = 200 $ $/unit, $ l = 300 $ m, $ b = 300 $ m, $ \mu = 12 $ $ m^2 $, $ \rho = 0.05 $, $ \phi = 0.8 $, $ \eta_2 = 12 $ $ m^2 $, $ C_{cc} = 10 $ $/unit, $ C_{ot} = 4 $ $/square unit, $ C_m = 200 $ $/unit, $ A = 1500 $ $, $ B = 1000 $ $/unit, $ \kappa = 0.7 $, $ \theta_{max} = 0.9 $, $ \theta_{min} = 0.1 $, $ \tau = 10 $ $/tool, $ C_{set} = 200 $ $/unit, $ C_{hm} = 2 $ $/unit, $ C_{ftm} = 0.7 $ $/unit, $ C_{fcm} = 0.2 $ $/unit, $ C_{vtm} = 0.1 $ $/unit, $ C_{vcm} = 0.2 $ $/unit, $ g_{01} = 5 $ unit, $ g_{02} = 7 $ unit, $ g_{03} = 8 $ unit, $ \omega_1 = 5 $, $ \omega_2 = 7 $, $ w_0 = 8 $ unit, $ w_1 = 5 $ unit, $ A_1 = 10 $ $/unit, $ A_2 = 15 $ $/unit, $ A_3 = 20 $ $/unit, $ \pi_{01} = 150 $ $/unit, $ \pi_{02} = 140 $ $/unit, $ \pi_{03} = 160 $ $/unit, $ \beta_{01} = 0.3, \beta_{02} = 0.4, \beta_{03} = 0.5 $, $ \sigma_1 = 7, \sigma_2 = 6, \sigma_3 = 9 $, $ L_1 = 3 $ day, $ L_2 = 3 $ day, $ L_3 = 3 $ day, $ k_1 = 5 $ unit, $ k_2 = 7 $ unit, $ k_3 = 8 $ unit, $ C_{rh} = 4 $ $/unit
6.1. Values of the decision variables and total cost obtained by GA and PSO
The values of the over parameters considered in this numerical case are taken from; in any case, these values considered here are practical. Two soft-computing methods, i.e., GA and PSO, are utilized to get the optimal solution to this nonlinear optimization problem. These calculations are coded in MatLab programming and run on a PC with Intel(R) Core(TM) i5-2400 3.10 GHz processor and 16.0 GB RAM in 64-bit Windows 10 operating systems. From these outcomes, the solution is illustrated in Table 2. This illustration is illuminated in arrange to find the values of $ s_i, \lambda, n, d, \zeta_1, \zeta_2, \theta, P, $ and $ \pi_{x_j} $ along with the joint total cost $ JATC $ of the system.
Within the proposed GA, the taking after values of GA parameters have been utilized:
Population size$ = 500 $, Maximum number of generation $ = 1000 $, Function tolerance $ = 10^{-6} $.
Within the proposed PSO, the taking after values of PSO parameters have been utilized:
Population size $ = 1000 $, Maximum number of generation $ = 10 $, $ c_1 = 1.5 $, $ c_2 = 1.5 $
6.2. Comparative Study
PSO is comparable to the GA as these two strategies are evolutionary methods for searching for optimum values. In both methods, using some deterministic and probabilistic rules, the values of the decision variables are upgraded to another set of values of the decision variables. PSO is a comparatively recent heuristic algorithm based on the behavior of characteristics of swarming living beings; on the other hand, GA is a well-set and prevalent algorithm with numerous applications and distinctive forms. GA is a discrete optimization technique that converts the variables to binary digits 0's and 1's, so it can effectively handle discrete issues. In contrast, PSO is for continuous functions and subsequently must be altered to address the discrete problems. Unlike GA, based on the current position, the factors in PSO can take any values within the space of particles and the comparing vector of velocity. The calculation complexity cannot be handled by GA efficiently because, in GA, the number of components transforms into exceptionally large, by which the search space increases considerably. Again, GA converges to a local extremum, whereas PSO tries to get the global extremum. So, PSO is better because it requires a small number of parameters and a correspondingly less number of iterations.
In this study, from Table 2 the minimum cost of the SC using PSO is $ $ 521712.49 $ whereas the minimum cost obtained by using GA is $ $ 523374.80 $, which is more than the previous. Thus, in this study, it is also established that PSO gives $ 0.32 $ % less cost than GA; hence, PSO is a better optimization technique than GA.
6.3. Special cases
For more practicality, some special cases due to various conditions are explained in this study. These cases compare this study with other research and establish the nobility of the study.
Case - 1: When manufacturer applied SSSD policy
Instead of the SSMUID policy, if the manufacturer applied the simple single-setup-single-delivery (SSSD) policy, then optimum values of the parameters and the minimum cost obtained by using GA are as follows:
$ s_1 = 60.62 $ (unit), $ s_2 = 31.88 $ (unit), $ s_3 = 51.42 $ (unit), $ d = 9.99 $ (meter), $ \zeta_1 = 0.99 $, $ \zeta_2 = 0.50 $ $ \theta = 0.044 $, $ P = 100.06 $ (unit), $ \pi_{x_1} = 75.28 $ ($unit), $ \pi_{x_2} = 66.17 $ ($unit), $ \pi_{x_3} = 83.45 $ ($unit), and the Total cost $ JATC = $ 525070.83 $.
Also, the optimum values of the parameters and the minimum cost obtained by applying PSO are as follows:
$ s_1 = 55.27 $ (unit), $ s_2 = 32.23 $ (unit), $ s_3 = 68.49 $ (unit), $ d = 10 $ (meter), $ \zeta_1 = 2 $, $ \zeta_2 = 1.5 $ $ \theta = 0.001 $, $ P = 100 $ (unit), $ \pi_{x_1} = 80 $ ($unit), $ \pi_{x_2} = 90 $ ($unit), $ \pi_{x_3} = 85 $ ($unit), and the Total cost $ JATC = $ 524917.94 $.
It is clear from the results of both the techniques that the SSMUID policy reduces the total cost of the SC than the SSSD policy, and hence SSMUID policy is more profitable than the SSSD policy.
Case - 2: When manufacturer applied SSMD policy
Instead of the SSMUID policy, if the manufacturer applied the single-setup-multi-delivery (SSMD) policy, then optimum values of the parameters and the minimum cost obtained by using GA are as follows:
$ s_1 = 89.99 $ (unit), $ s_2 = 79.99 $ (unit), $ s_3 = 69.99 $ (unit), $ \lambda $ = 1, $ n = 4 $, $ d = 9.99 $ (meter), $ \zeta_1 = 0.99 $, $ \zeta_2 = 0.05 $ $ \theta = 0.09 $, $ P = 100.06 $ (unit), $ \pi_{x_1} = 75.54 $ ($unit), $ \pi_{x_2} = 70.42 $ ($unit), $ \pi_{x_3} = 81.71 $ ($unit), and the Total cost $ JATC = $ 524065.35 $.
Also, the optimum values of the parameters and the minimum cost obtained by applying PSO are as follows:
$ s_1 = 90 $ (unit), $ s_2 = 80 $ (unit), $ s_3 = 70 $ (unit), $ \lambda = 1 $, $ n = 4 $, $ d = 10 $ (meter), $ \zeta_1 = 2 $, $ \zeta_2 = 1 $ $ \theta = 0.002 $, $ P = 100 $ (unit), $ \pi_{x_1} = 80 $ ($unit), $ \pi_{x_2} = 90 $ ($unit), $ \pi_{x_3} = 85 $ ($unit), and the Total cost $ JATC = $ 523845.67 $.
It is clear from the result that the SSMUID policy reduces the total cost of the SC than the SSMD policy; hence, the SSMUID policy is more profitable than the SSMD policy. It is also noticed that the SSMD policy is more beneficial than the SSSD policy.
Case - 3: No backorder price discount offered by the retailer
To reduce the number of lost sales, the retailer usually offers backorder price discounts for the customers. Now consider the special case when the retailer does not provide any discounts for backorder. Then optimum values of the parameters and the minimum cost obtained by applying GA are as follows:
$ s_1 = 89.67 $ (unit), $ s_2 = 79.52 $ (unit), $ s_3 = 69.23 $ (unit), $ \lambda $ = 1.03, $ n = 4 $, $ d = 9.99 $ (meter), $ \zeta_1 = 0.99 $, $ \zeta_2 = 0.54 $ $ \theta = 0.1 $, $ P = 100 $ (unit), and the Total cost $ JATC = $ 524153.46 $.
Also the optimum values of the parameters and the minimum cost obtained by applying PSO are as follows:
$ s_1 = 90 $ (unit), $ s_2 = 80 $ (unit), $ s_3 = 70 $ (unit), $ \lambda = 1.02 $, $ n = 4 $, $ d = 10 $ (meter), $ \zeta_1 = 2 $, $ \zeta_2 = 1.5 $ $ \theta = 0.002 $, $ P = 100 $ (unit), and the Total cost $ JATC = $ 524065.79 $.
It is clear from the result that if the retailer doesn't provide any backorder price discount for customers, it affects the SC's total cost. The total cost increases for not offering any backorder price discount, and hence backorder price discount is essential for the SC to reduce the total cost.
6.4. Sensitivity analysis
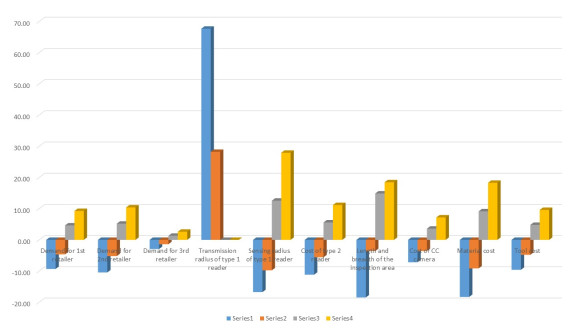
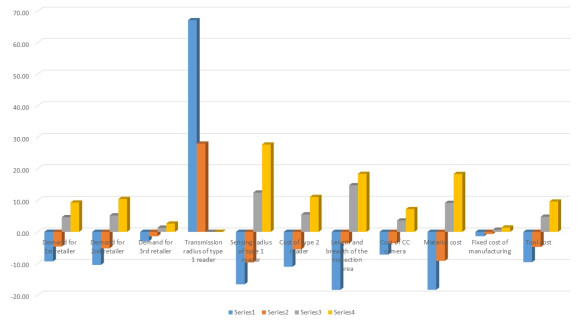
An analysis of the parameters' sensitivity is explained numerically by changing that particular parameter from $ -50\% $ to $ +50\% $ and keeping all the remaining parameters the same for both the optimization techniques, GA and PSO. Table 3 shows the changes in both the strategies (GA and PSO). The changes are also graphically presented in Figure 6 and Figure 7 for GA and PSO, respectively.
From the sensitivity table (Table 3) the following conclusion may made:
● The total cost is affected enough due to demand. Suppose the demand for a product decreases, then the amount of production, holding, and transportation decrease parallelly. As expected in Table 3, it is clear that the total cost decreases and increases due to the decrease and increase in retailers' demand.
● The upside or drawback in the parameter $ \eta_1 $, that is, the number of items in each box causes a significant change in the total cost. Moreover, if 25% and 50% expand $ \eta_1 $, then the total cost decreases; on the other hand, if $ eta_1 $ is diminished by 50% and 25%, the total cost increases. It is quite natural as the manufacturer has to buy more RFID tags to reduce the number of items in each box.
● The transmission and sensing radius ($ S_t $ and $ S_s $) of the type 1 scanner incorporates an extraordinary effect on the total cost of the SC. Total cost increases for the diminish of transmission radius and increments of detecting radius and vice versa.
● The rate increment or diminish in the parameters $ C_{rfs2} $ (cost of Type 2 RFID scanner), $ l $ and $ b $ (length and breadth of the inspection area), $ C_{cc} $ (cost of CC camera), $ C_m $ (material cost), and $ \tau $ (tool cost) are sensitive for the total cost. An increment within the parameters increases the total cost and diminishes within the parameters diminishes the cost.
● The parameters $ C_{rft} $ (RFID tag cost), $ C_{rfs1} $ (cost of Type 1 RFID scanner), $ A $ (fixed manufacturing cost), $ C_{set} $ (setup cost of the manufacturer), $ C_{hm} $ (holding cost of the manufacturer), $ g_{11}, g_{12}, g_{13} $ (amount of fuel consumption for returned vehicles), $ \omega_1, \omega_2 $ (carbon emission factors for fuel and electricity), $ w_1 $ (variable electricity consumption) has mild impact on the total cost. The total cost increases or decreases slightly for the increment or decrease of the value of these parameters.
● The transmission radius of the Type 1 scanner ($ S_t $) is the most sensitive parameter for the study, whereas the length and breadth ($ l $ and $ b $) of the inspection area are the 2nd most sensitive parameter.
● The parameters not shown in the figure is significantly less impact on the total cost.
7.
Managerial insights
The result illustrates the vital and key bits of knowledge for managers in overseeing unreliability issues and shortage issues within the SC with the joining of variable carbon emission costs, variable production, and technical up-gradation. As today's customers want quick and on-time delivery, the industry managers need to prevent the shortage problem that occurred due to unreliable players of the SC. The managers have to take suitable policies for solving the shortage problem of the SC.
Technological up-gradation is too much essential for an SC as, without an upgrade, a company may lose its identity in the market. So the managers must take the necessary steps to upgrade the system by applying suitable technologies. This up-gradation helps for smooth conduction of the SC and also reduces the shortage problem in the SC. Also, since the transmission radius of the Type 1 RFID scanner is highly sensitive to the total cost, the managers should have to take care of this.
The managers also have to focus on the shipment strategy the manufacturer applies as the retailer's holding cost directly depends on it. A suitable shipment strategy can diminish the "holding cost" of the retailer and helps to run the SC smoothly. Also, the managers should pay attention to environmental sustainability and have to apply strategies to save nature and reduce global warming.
The production cost and production rate may not be fixed, so it is essential to apply a reasonable production cost depending on realistic items like material cost, time, and tool cost. Finally, the managers have to concentrate on minimizing the total cost of SC. In brief, a practical guideline is proposed in this study for the decision-makers to smooth the SC flow by reducing the shortage problem and minimizing the total cost of the SC.
8.
Conclusions and future extensions
The disaster created in an SC for unreliable manufacturers and retailers is illustrated in this study. The unreliable manufacturer produced less quantity than the ordered quantity, which caused a shortage problem for the retailer. On the other hand, the unreliable retailer provided wrong information reading sales, which caused inaccurate estimation for the manufacturer. The shortage problem due to unreliable manufacturers is solved by applying a backorder price discount by the retailer. Whereas to solve the inaccurate estimation problem, the manufacturer does a technical up-gradation in the warehouse. Also, to reduce the "holding cost" of the player, the SSMUID technique is applied by the manufacturer to deliver the produced items in different shipments, which also helps to reduce the shortage problem. The variable carbon emission cost depending on fuel consumption and electricity consumption helps to improve environmental sustainability, which is beneficial for our society. Also, material and tool-dependent production costs are helping the manufacturer with the cost of production accurately. The model is solved through a realistic robust technique due to randomness in lead time demand. Finally, the total cost of the SC is optimized numerically by using two famous and popular techniques - one in GA and another in PSO. These numerical tests demonstrate the reality of the study trailed by the in-depth sensitivity analysis. {The numerical test shows that the total cost obtained by applying PSO is $ 0.32 $% less than the cost obtained using GA. It established that PSO is a more beneficial optimization method than GA.}
To show the practicability of this model, some case studies are explained. The explained case studies show that the SSMUID technique is $ 0.62\% $ beneficial compared to the SSSD policy and $ 0.35\% $ compared to the SSMD policy. Offering a backorder price discount is $ 0.45\% $ profitable for an SC. Lead time is one of the limitations of the study. So one can extend the study by considering lead time and proper investment for reducing the lead time [42]. Fixed ordering cost is another limitation of this study. By considering variable ordering cost, one can reduce the total system cost [16]. Consideration of stochastic demand is an interesting extension in this research field. Moreover, by enabling the role of blockchain technology in SC [51,52,53,54] and by increasing the number of the manufacturer, one can extend the current study.
Conflict of interest
All authors declare no conflicts of interest in this study.
A.
Technological growth cost
A.1. Symmetric RFID tag cost
An RFID tag is an upgraded tracking device utilized to identify objects by radiofrequency technology. These radio waves turn information from the tag to an RFID reader, which transmits the data to an RFID program on the computer. In this study, each box contains $ \eta_1 $ items, then the total number of boxes is $ \left\lceil {\frac{\alpha Q}{\eta_1}} \right\rceil $, where $ \left\lceil {x} \right\rceil $ is the the least integer greater than or equal to $ x $, called ceiling function of $ x $. Thus, the cost of each RFID tag is $ C_{rft} $ unit, so total cost for the symmetric RFID tags is $ \left\lceil {\frac{\alpha Q}{\eta_1}} \right\rceil C_{rft} $ unit and hence the symmetric RFID tag cost per cycle is
A.2. Symmetric RFID scanner cost
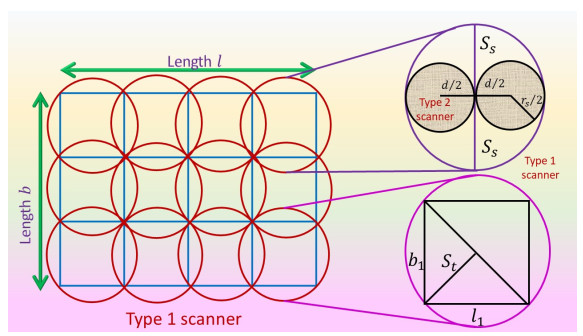
RFID could be an innovation comparable in hypothesis to bar codes. An RFID scanner could be a gadget to assemble data from an RFID tag to track objects. However, the RFID tag does not need to be scanned directly, nor does it require line-of-sight to a scanner. The RFID tag must be inside the range of an RFID scanner, which ranges from 3 to 300 feet, for perfect scanning. RFID permits several things to be scanned rapidly and quickly and recognize a specific item when a few other things encompass it. In this study, to overcome the shortage issues coming from the inaccurate estimation of the manufacturer, the manufacturer will oversee the manufacturing products' total stock in the warehouse by applying RFID tags and scanners. RFID confirms the number of items stored in the warehouses. The network between the radius of sensing and the radius of transmitting is given by condition $ 2S_s \leq S_t $ [55]. It isn't essential to utilize a continuously effective RFID Type 1 scanner. Moreover, that consistently low effective Type 2 scanner ought to be used, and optimization is required to optimize the ideal number of Type 1 and Type 2 scanners inside the complete range.
If for each subdivided region, $ l_1 $ the length and $ b_1 $ be the breadth, then according to right-angled triangle's property (Figure 8), it takes after that $ S_t = \sqrt{\frac{l_1^2 + b_1^2}{4}} $. For every square area $ S_t = \frac{l_1}{\sqrt{2}} $ (since, $ l_1 = b_1 $). Therefore for the area of length $ l $ and breadth $ b $, the number of Type 1 scanner needed is $ \left\lceil {\frac{l}{\sqrt{2} S_t}} \right\rceil \left\lceil {\frac{b}{\sqrt{2} S_t}}\right\rceil $. Again each subdivided region of detecting radius $ S_s $ is partitioned into two zones with detecting radius $ r_s $. If $ d $ be the maximum distance between any two Type 2 scanners then $ r_s = \frac{d}{2} $ and according to Hefeeda and Ahmadi [56], $ d \leq \sqrt{3} \left(\mu - \frac{Log \left[1 - \sqrt[3]{1 - \phi} \right]}{\rho} \right) $ Finally, circular area for radius of sensing $ S_s $ is $ \pi S_s^2 $ and for radius of sensing $ r_s $ is $ \frac{\pi d^2}{4} $. Subsequently, the overall number of Type 2 scanner for all Type 1 scanner is $ \left\lceil{\frac{4 {S_s}^2}{d^2}} \right\rceil \left\lceil {\frac{l}{\sqrt{2} S_t}} \right\rceil \left\lceil{\frac{b}{\sqrt{2} S_t}}\right\rceil $. If $ C_{rfs1} $ is the cost of each Type 1 scanner and $ C_{rfs2} $ is for each Type 2 scanner, which the manufacturer pays as an speculation, the desired cost for RFID is given by
subject to the condition $ d \leq \sqrt{3} \left(\mu - \frac{Log \left[1 - \sqrt[3]{1 - \phi} \right]}{\rho} \right) $ and $ S_t \geq 2 S_s $, where the length and the breadth of the total search area are $ l $ and $ b $, respectively, $ S_t $, $ S_s $ are the transmission radius and "sensing radius of Type 1 reader" respectively, $ d $ is the distance between two "RFID readers", $ \phi $ is the threshold parameter $ (0 < \phi < 1) $, and $ \rho $ is the decay parameter for sensing.
A.3. CCTV system cost
Day by day, global logistics is getting faster and more complex. Modern video observation (CCTV) can offer people real-time screen security and operations assistance along the SC. At numerous events, it is essential to control other resources continually in movement, which are a part of the manufacturing process. Through CCTV, state-of-the-art video reconnaissance frameworks can track and find thousands of versatile resources simultaneously with remarkable exactness. In this way, the supervision of these resources in development, counting the work of the administrators, gets to be an easy task. This study uses downward-focused CC symmetric cameras with rectangular focus areas for video surveillance. The entire investment for symmetric CC cameras is
A.4. Internet connectivity cost
A wireless local area network (WLAN) is a computer organization that joins two or more gadgets by employing a remote dispersion strategy. WLAN permits clients with devices to move around inside the coverage region. A remote LAN comprises numerous components, including a switch, modem, WIFI router, hub, access points, and competent wireless gadgets such as tablets, laptops, mobiles, or computers. The amount of investment needed for improving WLAN depends on the coverage area. In this study, $ C_{wr} $ represents the investment for improving WLAN per unit area. Hence the total investment for improving WLAN in the manufacturing house is as follows:
A.5. Other technological growth cost
Other costs like maintenance of CCTV, maintenance of WLAN, internet package etc. for technological growth per cycle is









 DownLoad:
DownLoad: