1.
Introduction
The topic of this paper is inspired by one of the recent scientific interests of Rosario Mingione, the so–called "double phase" elliptic problem.
The main example of a double phase integral functional is
where Ω is an open, bounded subset of RN (N≥2),
and
Since it is not assumed that the weight ρ(x) is bounded away from zero (that is, it is not assumed that ∃ρ0∈R+ such that ρ(x)≥ρ0>0), it is not possible to say, even under the assumption p<q, that the term ρ(x)|∇v|q is dominant, so that the set {x:ρ(x)=0} plays an important role.
Few years ago, R. Mingione found a name for such a problem: double phase problems. Since then, these problems and this terminology have become very popular.
Note that, the functional J exhibits unbalanced growth: the (p,q)-growth in the Marcellini terminology (see [16]).
Nowadays, there is a huge literature concerning double phase elliptic problems. Here we only recall the fundamental papers [2,3,12,13], and recently [14,17].
The main example of a double phase elliptic nonlinear differential operator is the derivative of J, that is
In this paper we study the existence of distributional solutions, belonging to some standard Sobolev spaces, of Dirichlet problems with very singular data, and associated to differential operators of double phase type like
with g(0)=0.
Namely, we deal with the existence of solutions of the following boundary value problem
where Ω is an open, bounded subset of RN (N≥2),
a(x) is a measurable function such that
We point out that
● in (1.4) the parameters λ,p play the role of p,q in (1.1)
● the operator presented in (1.3) also depends on a power of u;
● the coefficient a(x) does not need to be smooth.
Our existence results hinge on the presence of the additional term
which strongly helps, even if it has a growth (with respect to the gradient) λ≤p and despite of the degeneracy due to the factor |u|(r−1)λ+1.
As a matter of fact, this term provides a strong regularizing property: roughly speaking, we prove that the solution u of (1.3), under a suitable relationship between the parameters p and λ, is more regular (and it even exists) than the solution y of
studied in [4,7,8].
The regularizing effect of some lower orders terms, in the framework of boundary value problems with L1-data, is already known since the paper [10] by H. Brezis and W. A. Strauss. We also refer to the paper [1,6,9,11], where some Dirichlet problems with lower order terms of order zero or of order one, with natural growth with respect to ∇u are studied.
2.
The case of bounded data
This section deals with the case
In the sequel, given k>0, we denote by Gk(s) and Tk(s) the classical truncated functions defined by
Let us introduce the following sequence of boundary value problems
As a consequence of the classical result due to J. Leray, J. L. Lions (see [15]) there exists un∈W1,p0(Ω) which is a weak solution of the above problem in the sense that the following integral identity holds
Moreover, due to the boundedness of f and adapting the well known method used in [18], each un is a bounded function and there exists a positive contant Cf, independent on n, such that
Thus, for any n>Cf it holds Tn(un)=un and un is a weak solution of the following Dirichlet problem
that is
Taking un as test function in (2.3) and using the assumption (1.5) we have
Dropping the second (positive) term in the left–hand side and using the boundedness of f we obtain
Here, and in the sequel, we denote by Ci positive constants only depending on the data (but not on n).
Thus, there exist a subsequence, not relabelled, and a function u∈W1,p0(Ω)∩L∞(Ω) such that
Moreover, using estimate (2.4) we obtain, since 1<λ≤p,
Thus,
In order to have
it is enough to prove that
Let us take v=un−u as test function in (2.3)
Due to the positivity of the second term we get
We note that the first and the second integral in the right–hand side converge to 0. Moreover,
and (since |∇u|λ−1∈Lp′)
Thus, by the Lebesgue Theorem we get
which in turn implies
Then, (2.9) easily follows taking the limit as n→+∞ in (2.10) and the strong convergence (2.8) is proved. Finally, we take the limit as n→+∞ in (2.3) (using (2.9) and (2.11)) and we obtain the following existence theorem.
Theorem 2.1. Let 1<λ≤p<N. Assume that (1.5), (1.6) hold and let
Then there exists a weak solution u∈W1,p0(Ω)∩L∞(Ω) which solves the problem (1.3) in the following weak sense
for any v∈W1,p0(Ω).
3.
Non regular data
In this section we assume that
and we will prove the existence of a distributional solution of problem (1.3)
3.1. Approximating problems
Let {fn} be a sequence of bounded functions such that
and
Classical examples are fn=Tn[f] and fn=f1+1n|f|.
Let us introduce the following approximate boundary value problems
By Theorem 2.1, there exists un∈W1,p0(Ω)∩L∞(Ω) such that, for any v∈W1,p0(Ω),
Let k>0; by taking Tk(un) as test function in the weak formulation (3.3) of problem (3.2) and dropping the positive second term, we can proceed as in [4] and the following lemma holds.
Lemma 3.1. Let 1<λ≤p<N. Assume that the hypotheses (1.5), (1.6), (3.1) are satisfied. Then, for any k>0 it holds
Moreover, there exists C0>0 such that
and
Next, we will prove the following lemma.
Lemma 3.2. Let 1<λ≤p<N. Assume that the hypotheses (1.5), (1.6), (3.1) are satisfied. Then there exists a positive constant R, independent on n, such that
Proof. We set η=(r−1)λ+1 (note that η>1 since r>1) and we take
as test function in (3.3). Dropping the positive term resulting by the principal part, we obtain
We fix k>0. By the above estimate we have
Thus, putting together estimates (3.4) and (3.8), it follows (3.7).
Further improvements on the boundedness of un and ∇un, depending on the relationship between the parameters p,λ and r, can be derived from the following lemma
Lemma 3.3. Let 1<λ≤p<N. Assume that the hypotheses (1.5), (1.6), (3.1) are satisfied. Then there exist two positive constants R1,R2 independent of n such that
and
with
Proof. By taking v=log(1+|un|)un|un| as test function in the weak formulation (3.3) of problem (3.2) (see [8]), it is easy to see that
Using in the right–hand side the inequality
and the boundedness of {un} in L1(Ω) and, taking into account the positivity of each of the two integrals in the left-hand side and (3.7), the following two estimates hold
and
From estimate (3.11) we also deduce
which, together with inequality (3.4), implies
Now, we can use Sobolev inequality
and the estimate (3.9) follows.
Next, let us prove (3.10). We follow the outline of [8]. Note that since σ<p, by Hölder inequality with exponents pσ,pp−σ and inequality (3.12), we have
and the proof is concluded, since, by the choice of σ, it follows σp−σ=rλ∗.
Remark 3.4. Note that in Lemmas 3.1 and 3.7 we only use the assumption f∈L1(Ω), while Lemma 3.3 requires the additional hypothesis f∈L1logL1(Ω). However, if f is merely summable, the proof of Lemma 3.3 can be repeated in order to obtain the boundedness of {∇un} in W1,σ0(Ω), for any 1≤σ<rpλ∗1+rλ∗.
Remark 3.5. We point out that
Moreover
Thus, Lemma 3.3 improves Lemma 3.7 if 1≤λ<Npr−1Nr−1 and p>1+N−1r. (Note that 1+N−1r∈]1,2−1N[ since r>1).
Remark 3.6. Let 2−1N<p<N. Taking into account only the contribution of the principal part and applying the results of [7] we deduce that the sequence {un} is bounded in W1,N(p−1)N−10(Ω).
Thus the term
has a regularizing effect in the following two cases
i) 2−1N<p<N and (p−1)NN−1<λ≤p,
ii) 1<p≤2−1N and 1<λ≤p.
As a consequence of previous lemmas we prove the following two existence results.
Theorem 3.7. Let 1<λ≤p<N. Assume that hypotheses (1.5), (1.6) and (3.1) hold.
Then there exists u∈W1,λ0(Ω), such that g(u)|∇u|λ−1∈L1(Ω), which solves the problem (1.3) in the following distributional sense
for any v∈C∞0(Ω).
Theorem 3.8. Let 1<λ≤p<N. Assume that hypotheses (1.5), (1.6) and (3.1) hold.
Then there exists u∈W1,σ0(Ω), such that g(u)|∇u|λ−1∈L1(Ω), which solves the problem (1.3) in the distributional sense (3.14).
Remark 3.9. We explicitly remark that, in the case λ=p, Theorem 3.7 gives the existence of at least one solution with finite energy without any additional assumption on the summability of f. A similar regularizing effect occurs for the solution of the Dirichlet problem associated to the equation
where f∈Lm(Ω) with 1<m<(p∗)′, when a suitable balance between m and s holds, (see [11]) or to the equation
with f∈L1(Ω) (see [9]).
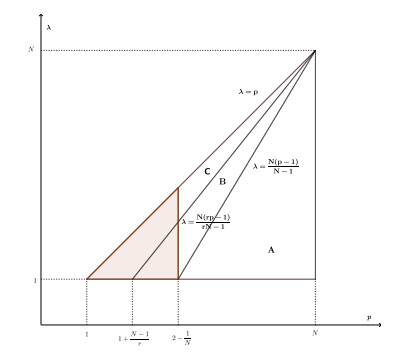
The following Figure 1 summarizes the different regularity results in dependence of p and λ.
If (p,λ) belongs to the region A, the better regularity is the one obtained in [7], i.e., u∈W1,N(p−1)N−10(Ω); otherwise the better regularity is the one proved here.
If (p,λ) belongs to the region B, Theorem 3.8 gives the existence of a distributional solution u∈W1,σ0(Ω); while in the region C the better regularity is the one stated in Theorem 3.7, i.e., u∈W1,λ0(Ω).
At last, if (p,λ) belongs to the colored region the result stated in Theorem 3.7 is new.
3.2. Proof of Theorems 3.7 and 3.8
We begin with the proof of Theorem 3.7.
As a consequence of Lemma 3.1 and Lemma 3.7 there exist a subsequence, not relabelled, and a function u∈W1,λ0(Ω) such that
In order to take the limit as n→+∞ in (3.2) we have to prove that
We follow some techniques of [5]. For any ξ∈RN, we set
Let j,k>0; using v=Tj[un−Tk(u)] as test function in (3.3) we have
We note that
Moreover (since A(x,∇Tk(u))∇Tj[u−Tk(u)]=0)
and
since Bn(0)=0. Thus, from (3.16) we deduce
where we have denoted by ϵ1n(k) and ϵ2n(k) two functions which go to 0 as n→+∞, for any k>0 and
Now, we use the above inequality in order to prove the L1 compactness of the sequence {∇un}.
Let 0<θ<λp (0<θ<1) and k>0. Let us define
and let us prove that the previous integral converges to zero.
Indeed, it holds
where
and
with
We observe that
Using the Hölder inequality, with exponents λpθ and λλ−pθ, and the a priori estimate (3.10), we have
Here and in the sequel, for any measurable set E⊂RN, |E| denotes its N− dimensional measure. Moreover, by ωi(k) we denote some quantities such that limk→∞ωi(k)=0. Now, we have to study the behavior of Jn,Ω; it can be splitted as (j∈N)
We estimate J1n,Ω and J2n,Ω by means of Hölder inequality with exponents 1θ, 11−θ and λpθ, λλ−pθ, respectively and we use inequalities (3.17) and (3.7), getting
Since
and
we obtain
Summing up the above inequality and (3.18) we have
Therefore,
which gives (for a suitable subsequence, still denoted by un)
and also (since θ is positive)
In [15], it is proved that, under our assumptions on the function A(x,ξ), the previous limit implies that
Thus
and, thanks to (3.6) we have
Next, we will prove that
Thanks to (3.19) we also deduce
Moreover, for any measurable set E⊂Ω we have
and the right–hand side goes to 0 as |E|→0 uniformly w.r.t. n, since estimates (3.9) and (3.13) hold. Thus
Thanks to Vitali Theorem the convergence (3.22) is proved and
The above limit and the limit (3.21), allow us to take the limit as n→+∞ in (3.3) and the proof of Theorem 3.7 follows.
In order to prove Theorem 3.8 we note that as a consequence of Lemma 3.1 and Lemma 3.3. there exist a subsequence, not relabelled, and a function u∈W1,σ0(Ω) such that
and the proof can be performed as above. Precisely, in order to obtain the a.e. convergence of {∇un} we just have to replace λ with σ.
Acknowledgements
This work has been supported by Project EEEP & DLaD – Piano della Ricerca di Ateneo 2020-2022–PIACERI.
G. R. Cirmi is member of the Gruppo Nazionale per l'Analisi Matematica, la Probabilità e le loro Applicazioni (GNAMPA) of the Istituto Nazionale di Alta Matematica (INdAM).
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad:



