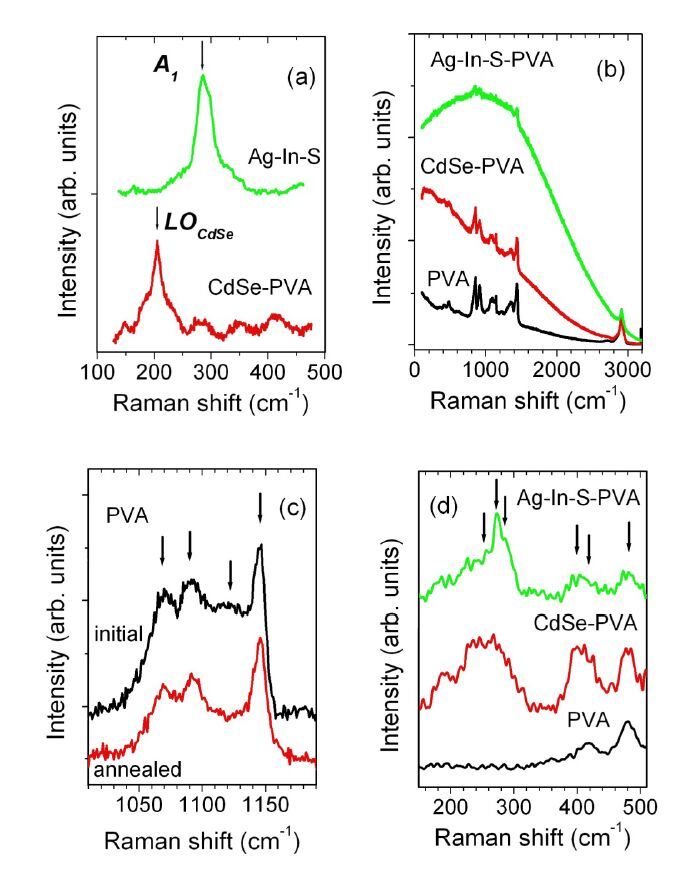
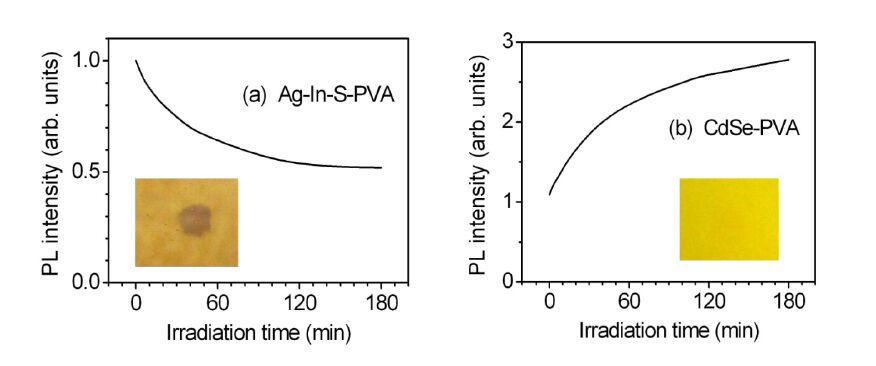
The results of investigation of photostability of the composites of CdSe and Ag-In-S nanocrystals (NCs) embedded in the films of polyvinyl alcohol (PVA) are presented. The films were studied by photoluminescence (PL), optical absorption, micro-Raman and X-ray diffraction methods. It is found that heating of the films to 100 °C promotes PVA crystallization and stimulates an increase of the PL intensity for the NCs of both types. The latter effect is ascribed mainly to the improvement of NC surface passivation by functional groups of PVA. The illumination with the 409-nm LED’s light enhances PL intensity for CdSe NCs and decreases it for Ag-In-S NCs as well as results in the darkening of the films. The color of the Ag-In-S-PVA film restores with time, while the change of the optical properties of the CdSe-PVA composite is irreversible. The possible mechanisms of the revealed effects, such as structural transformations at NC/PVA interface as well as the formation of new light-absorbing species are discussed.
1.
Introduction
Fractional differential equations (FDEs) appeared as an excellent mathematical tool for, modeling of many physical phenomena appearing in various branches of science and engineering, such as viscoelasticity, statistical mechanics, dynamics of particles, etc. Fractional calculus is a recently developing work in mathematics which studies derivatives and integrals of functions of fractional order [26].
The most used fractional derivatives are the Riemann-Liouville (RL) and Caputo derivatives. These derivatives contain a non-singular derivatives but still conserves the most important peculiarity of the fractional operators [1,2,10,11,23,24]. Atangana and Baleanu described a derivative with a generalized Mittag-leffler (ML) function. This derivative is often called the Atangana-Baleanu (AB) fractional derivative. The AB-derivative in the senses of Riemman-Liouville and Caputo are denoted by ABR-derivative and ABC-derivative, respectively.
The AB fractional derivative is a nonlocal fractional derivative with nonsingular kernel which is connected with various applications [3,5,6,8,9,13,14,15,16]. Using the advantage of the non-singular ML kernal present in the AB fractional derivatives, operators, many authors from various branches of applied mathematics have developed and studied mathematical models involving AB fractional derivatives [18,22,29,30,31,32,35,36,37].
Mohamed et al. [25] considered a system of multi-derivatives for Caputo FDEs with an initial value problem, examined the existence and uniqueness results and obtained numerical results. Sutar et al. [32,33] considered multi-derivative FDEs involving the ABR derivative and examined existence, uniqueness and dependence results. Kucche et al. [12,19,20,21,34] enlarged the work of multi-derivative fractional differential equations involving the Caputo fractional derivative and studied the existence, uniqueness and continuous dependence of the solution.
Inspired by the preceding work, we perceive the multi-derivative nonlinear neutral fractional integro-differential equation with AB fractional derivative of the Riemann-Liouville sense of the problem:
where $ ^{\star}_{0} D^{\delta}_{\jmath} $ denotes the ABR fractional derivative of order $ \delta\in(0, 1) $, and $ \varphi \in \mathscr{C}(\mathscr{I} \times \mathscr{R} \times \mathscr{R} \times \mathscr{R}, \mathscr{R}) $ is a non-linear function. Let $ \mathcal{P}_{1}\mathcal{V}(\jmath) = \int^{\jmath}_{0}\mathcal{K}(\jmath, \theta, \mathcal{V}(\theta))d\theta $ and $ \mathcal{P}_{2}\mathcal{V}(\jmath) = \int^{T}_{0}\chi(\jmath, \theta, \mathcal{V}(\theta))d\theta $. Now, (1.1) becomes,
In this work, we derive a few supplemental results using the characteristics of the fractional integral operator $ \varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+} $. The existence results are obtained by Krasnoselskii's fixed point theorem and the uniqueness and data dependence results are obtained by the Gronwall-Bellman inequality.
2.
Preliminaries
Definition 2.1. [14] The Sobolev space $ H^{\mathfrak{q}}(X) $ is defined as $ H^{\mathfrak{q}}\left(X\right) = \left\{\varphi\in L^{2}\left(X\right):D^{\beta}\varphi\in L^{2}(X), \forall \left|\beta\right|\leq \mathfrak{q}\right\}. $ Let $ \mathfrak{q}\in[1, \infty) $ and $ X $ be open, $ X\subset\mathbb{R} $.
Definition 2.2. [11,17] The generalized ML function $ E^{\alpha}_{\delta, \beta}\left(u\right) $ for complex $ \delta, \beta, \alpha $ with Re$ (\delta) > 0 $ is defined by
and the Pochhammer symbol is $ (\alpha)_{t} $, where $ (\alpha)_{0} = 1, (\alpha)_{t} = \alpha(\alpha+1)...(\alpha+t-1), $ $ t = 1, 2...., $ and $ E^{1}_{\delta, \beta}\left(u\right) = E_{\delta, \beta}\left(u\right), E^{1}_{\delta, 1}\left(u\right) = E_{\delta}\left(u\right). $
Definition 2.3. [4] The ABR fractional derivative of $ \mathcal{V} $ of order $ \delta $ is
where $ \mathcal{V} \in H^{1}(0, 1) $, $ \delta \in(0, 1) $, $ B(\delta) > 0 $. Here, $ E_{\delta} $ is a one parameter ML function, which shows $ B(0) = B(1) = 1 $.
Definition 2.4. [4] The ABC fractional derivative of $ \mathcal{V} $ of order $ \delta $ is
where $ \mathcal{V} \in H^{1}(0, 1) $, $ \delta \in(0, 1) $, and $ B(\delta) > 0 $. Here, $ E_{\delta} $ is a one parameter ML function, which shows $ B(0) = B(1) = 1 $.
Lemma 2.5. [4] If $ L\left\{g(\jmath); b\right\} = \bar{G}(b) $, then $ L\left\{^{\star}_{0}D^{\delta}_{\jmath}g(\jmath); b\right\} = \frac{B(\delta)}{1-\delta}\frac{b^{\delta}\bar{G}(b)}{b^{\delta}+\frac{\delta}{1-\delta}}. $
Lemma 2.6. [26] $ L\left[\jmath^{m\delta+\beta-1}E^{(m)}_{\delta, \beta}\left(\pm a\jmath^{\delta}\right); b\right] = \frac{m!b^{\delta-\beta}}{\left(b^{\delta}\pm a\right)^{m+1}}, E^{m}(\jmath) = \frac{d^{m}}{d\jmath^{m}}E(\jmath). $
Definition 2.7. [17,27] The operator $ \varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+} $ on class $ L(m, n) $ is
where $ \delta, \eta, \mathcal{V}, \alpha\in \mathbb{C}\left(Re(\delta), Re(\eta) > 0\right) $, and $ n > m $.
Lemma 2.8. [17,27] The operator $ \varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+} $ is bounded on $ C[m, n] $, such that $ \left\|\left(\varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+}\right)[\mathcal{V}(\jmath)-x(\jmath, y(\jmath))]\right\| \leq \mathcal{P} \left\|\Theta\right\|, $ where
Here, $ \delta, \eta, \mathcal{V}, \alpha\in \mathbb{C}\left(Re(\delta), Re(\eta) > 0\right) $, and $ n > m $.
Lemma 2.9. [17,27] The operator $ \varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+} $ is invertible in the space $\textrm{L}(m, n) $ and $ \varphi \in \textrm{L}(m, n) $ its left inversion is given by
where $ \delta, \eta, \mathcal{V}, \alpha\in \mathbb{C}\left(Re(\delta), Re(\eta) > 0\right) $, and $ n > m $.
Lemma 2.10. [17,27] Let $ \delta, \eta, \mathcal{V}, \alpha\in \mathbb{C}\left(Re(\delta), Re(\eta) > 0\right), n > m $ and suppose that the integral equation is
is solvable in the space $ \textrm{L}(m, n) $.Then, its unique solution $ \Theta(\jmath) $ is given by
Lemma 2.11. [7] (Krasnoselskii's fixed point theorem) Let $ A $ be a Banach space and $ X $ be bounded, closed, convex subset of $ A $. Let $ \mathscr{F}_{1}, \mathscr{F}_{2} $ be maps of S into $ A $ such that $ \mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\varphi \in X $ $ \forall $ $ \mathcal{V}, \varphi \in U $. The equation $ \mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\mathcal{V} = \mathcal{V} $ has a solution on S, and $ \mathscr{F}_{1} $, $ \mathscr{F}_{2} $ is a contraction and completely continuous.
Lemma 2.12. [28] (Gronwall-Bellman inequality) Let $ \mathcal{V} $ and $ \varphi $ be continuous and non-negative functions defined on $ \mathscr{I} $. Let $ \mathcal{V}(\jmath)\leq \mathcal{A}+\int^{\jmath}_{a}\varphi(\theta)\mathcal{V}(\theta)d\theta, \jmath \in \mathscr{I} $; here, $ \mathcal{A} $ is a non-negative constant.
In this part, we need some fixed-point-techniques-based hypotheses for the results:
$ ({\rm{H1}}) $ Let $ \mathcal{V} \in C\left[0, T\right] $, function $ \varphi \in \left(C[0, T]\times \mathscr{R} \times \mathscr{R} \times \mathscr{R}, \mathscr{R} \right) $ is a continuous function, and there exist $ +^{ve} $ constants $ \zeta _{1}, \zeta_{2} $ and $ \zeta $. $ \left\|\varphi(\jmath, \mathcal{V}_{1}, \mathcal{V}_{2}, \mathcal{V}_{3})-\varphi(\jmath, \varphi_{1}, \varphi_{2}, \varphi_{3})\right\|\leq \zeta_{1}\left(\left\|\mathcal{V}_{1}-\varphi_{1}\right\|+\left\|\mathcal{V}_{2}-\varphi_{2}\right\|+\left\|\mathcal{V}_{3}-\varphi_{3}\right\|\right) $ for all $ \mathcal{V}_{1}, \mathcal{V}_{2}, \mathcal{V}_{3}, \varphi_{1}, \varphi_{2}, \varphi_{3} $ in $ Y $, $ \zeta _{2} = max_{\mathcal{V} \in \mathscr{R}}\left\|f(\jmath, 0, 0, 0)\right\| $, and $ \zeta = max\left\{ \zeta _{1}, \zeta _{2}\right\} $.
$ ({\rm{H2}}) $ $ \mathcal{P}_{1} $ is a continuous function, and there exist $ +^{ve} $ constants $ \mathscr{C}_{1}, \mathscr{C}_{2} $ and $ \mathscr{C} $. $ \left\|\mathcal{P}_{1}(\jmath, \theta, \mathcal{V}_{1})-\mathcal{P}_{1}(\jmath, \theta, \varphi_{1})\right\| \leq \mathscr{C}_{1} \left(\left\|\mathcal{V}_{1}-\varphi_{1}\right\|\right) \forall \, \mathcal{V}_{1}, \varphi_{1} $ in $ Y $, $ \mathscr{C}_{2} = max_{(\jmath, \theta) \in D}\left\|\mathcal{P}_{1}(\jmath, \theta, 0)\right\| $, and $ \mathscr{C} = max\left\{ \mathscr{C} _{1}, \mathscr{C} _{2} \right\} $.
$ ({\rm{H3}}) $ $ \mathcal{P}_{2} $ is a continuous function and there are $ +^{ve} $ constants $ \mathcal{D}_{1}, \mathcal{D}_{2} $ and $ \mathcal{D} $. $ \left\|\mathcal{P}_{2}(\jmath, \theta, \mathcal{V}_{1})-\mathcal{P}_{2}(\jmath, \theta, \varphi_{1})\right\| \leq \mathcal{D}_{1} \left(\left\|\mathcal{V}_{1}-\varphi_{1}\right\|\right) $ for all $ \mathcal{V}_{1}, \varphi_{1} $ in $ Y $, $ \mathcal{D}_{2} = max_{(\jmath, \theta) \in D}\left\|\mathcal{P}_{2}(\jmath, \theta, 0)\right\| $ and $ \mathcal{D} = max\left\{ \mathcal{D} _{1}, \mathcal{D} _{2} \right\} $.
$ ({\rm{H4}}) $ Let $ x \in c[0, I] $, function $ u \in (c[0, I] \times \mathscr{R}, \mathscr{R}) $ is a continuous function, and there is a $ +^{ve} $ constant $ k > 0 $, such that $ \left\|u(\jmath, x)-u(\jmath, y)\right\|\leq k \left\| x-y \right\| $. Let $ Y = C[\mathscr{R}, X] $ be the set of continuous functions on $ \mathscr{R} $ with values in the Banach space $ X $.
Lemma 2.13. If $ {\bf{(H_2)}} $ and $ {\bf{(H_3)}} $ are satisfied the following estimates, $ \left\|\mathcal{P}_{1}\mathcal{V}(\jmath)\right\|\leq \jmath(\mathscr{C}_{1}\left\|\mathcal{V}\right\|+\mathscr{C} _{2}), \left\|\mathcal{P}_{1}\mathcal{V}(\jmath)-\mathcal{P}_{1}\varphi(\jmath)\right\|\leq \mathscr{C}\jmath\left\|\mathcal{V}-\varphi\right\| $, and $ \left\|\mathcal{P}_{2}\mathcal{V}(\jmath)\right\|\leq \jmath(\mathcal{D}_{1}\left\|\mathcal{V}\right\|+\mathcal{D} _{2}), \left\|\mathcal{P}_{2}\mathcal{V}(\jmath)-\mathcal{P}_{2}\varphi(\jmath)\right\|\leq \mathcal{D}\jmath\left\|\mathcal{V}-\varphi\right\| $.
3.
Equivalent neutral fractional integral equation
Theorem 3.1. The function $ \varphi \in \mathscr{C}\left(\mathscr{I} \times \mathscr{R} \times \mathscr{R} \times \mathscr{R}, \mathscr{R}\right) $ and $ \mathcal{V}\in\mathscr{C}(\mathscr{I}) $ is a solution for the problem of Eqs (1.3) and (1.4), iff $ \mathcal{V} $ is a solution of the fractional equation
Proof. (1) By using Definition 2.3 and Eq (1.3), we get
Integrating both sides of the above equation with limits $ 0 $ to $ \jmath $, we get
Conversely, with differentiation on both sides of Eq (3.1) with respect to $ \jmath $, we get
Using Definition 2.3, we get Eq (1.3) and substitute $ \jmath = 0 $ in Eq (3.1), we get Eq (1.4).
Proof. (2) In Equation (1.3), taking the Laplace Transform on both sides, we get
Now, using the Laplace Transform formula for the AB fractional derivative of the RL sense, as given in Lemma 2.5, we get
$ \bar{X}(b) = \left[\mathcal{V}(\jmath); b\right] $ and $ \bar{G}(b) = L\left[\varphi\left(\jmath, \mathcal{V}\left(\jmath\right), P_{1}\mathcal{V}(\jmath), P_{2}\mathcal{V}(\jmath)\right); b\right]. $ Using Eq (1.4), we get
In Eq (3.2) applying the inverse Laplace Transform on both sides using Lemma 2.6 and the convolution theorem, we get
Theorem 3.2. Let $ \delta\in(0, 1) $. Define the operator $ \mathscr{F} $ on $ \mathscr{C}(\mathscr{I}) $:
$(A)$ $ \mathscr{F} $ is a bounded linear operator on $ \mathscr{C}(\mathscr{I}) $.
$(B)$ $ \mathscr{F} $ satisfying the hypotheses.
$(C)$ $ \mathscr{F}(X) $ is equicontinuous, and $ X $ is a bounded subset of $ \mathscr{C}(\mathscr{I}) $.
$(D)$ $ \mathscr{F} $ is invertible, function $ \varphi\in \mathscr{C}(\mathscr{I}) $, and the operator equation $ \mathscr{F}\mathcal{V} = \varphi $ has a unique solution in $ \mathscr{C}(\mathscr{I}) $.
Proof. (A) From Definition 2.7 and Lemma 2.8, the fractional integral operator $ \varepsilon^{1}_{\delta, 1, \frac{-\delta}{1-\delta}; 0^{+}} $ is a bounded linear operator on $ \mathscr{C}(\mathscr{I}) $, such that
and we have
Thus, $ \mathscr{F}\mathcal{V} = \varphi $ is a bounded linear operator on $ \mathscr{C}(\mathscr{I} $).
(B) We consider $ \mathcal{V}, \varphi\in \mathscr{C}(\mathscr{I}) $. By using linear operator $ \mathscr{F} $ and bounded operator $ \varepsilon^{1}_{\delta, 1, \frac{-\delta}{1-\delta}; 0^{+}} $, for any $ \jmath \in \mathscr{I} $,
Where, $ P = TE_{\delta, 2}\left(\frac{\delta}{1-\delta}T^{\delta}\right) $, then the operator $ \mathscr{F} $ is satisfied the hypotheses with constant $ P\frac{B(\delta)}{1-\delta} $.
(C) Let $ U = \left\{\mathcal{V}\in \mathscr{C}(\mathscr{I}) : \left\|\mathcal{V}\right\|\leq R\right\} $ be a bounded and closed subset of $ \mathscr{C}(\mathscr{I}) $, $ \mathcal{V}\in U $, and $ \jmath_{1}, \jmath_{2}\in \mathscr{I} $ with $ \jmath_{1}\leq \jmath_{2} $.
Hence, if $ \left|\jmath_{1}-\jmath_{2}\right|\rightarrow 0 $ then $ \left|(\mathscr{F}\mathcal{V})(\jmath_{1})-(\mathscr{F}\mathcal{V})(\jmath_{2})\right|\rightarrow 0. $
$ \therefore $ $ (\mathscr{F}\mathcal{V}) $ is equicontinuous on $ \mathscr{I}. $
(D) By Lemmas 2.9 and 2.10, $ \varphi\in \mathscr{C}(\mathscr{I}) $, and we get
By Eqs (3.4) and (3.5), we have
where $ \beta \in \mathbb{C} $ with $ Re(\beta) > 0 $. This shows $ \mathscr{F} $ is invertible on $ \mathscr{C}(\mathscr{I}) $ and
has the unique solution,
4.
Existence and uniqueness results
Theorem 4.1. Let $ \varphi \in \mathscr{C}\left(\mathscr{I}\times \mathscr{R} \times \mathscr{R} \times \mathscr{R}, \mathscr{R} \right) $. Then, the ABR derivative $ ^{\star}_{0}D^{\delta}_{\jmath}[\mathcal{V}(\jmath)-x(\jmath, y(\jmath))] = \varphi\left(\jmath, \mathcal{V}\left(\jmath\right), \mathcal{P}_{1}\mathcal{V}(\jmath), \mathcal{P}_{2}\mathcal{V}(\jmath)\right), \jmath \in \mathscr{I} $, is solvable in $ \mathscr{C}(\mathscr{I} $), and the solution in $ \mathscr{C}(\mathscr{I}) $ is
where $ \beta\in \mathbb{C}, Re(\beta) > 0 $, and $ \hat{\varphi}(\jmath) = \int^{\jmath}_{0}\varphi\left(\theta, \mathcal{V}\left(\theta\right), \mathcal{P}_{1}\mathcal{V}(\theta), \mathcal{P}_{2}\mathcal{V}(\theta)\right)d\theta, \jmath \in \mathscr{I} $.
Proof. The corresponding fractional equation of the ABR derivative
is given by
Using operator $ \mathscr{F} $ of Eq (3.4), we get
Equations (3.7) and (4.2) are solvable, and we get
Theorem 4.2. Let $ \varphi \in \mathscr{C}\left(\mathscr{I}\times {R \times R \times R}, \mathscr{R}\right) $ satisfy $ {\bf{(H_1)}} $–$ {\bf{(H_3)}} $ with $ L = \sup_{\jmath\in \mathscr{I}}\omega(\jmath), $ where $ \omega(\jmath) = \zeta(1+\mathscr{C}\jmath+\mathcal{D}T) $, if $ L = \min\left\{1, \frac{1}{2T}\right\} $. Then problem of (1.3) and (1.4) has a solution in $ \mathscr{C}(\mathscr{I}) $ provided
Proof. Define
where $ N_{\varphi} = \sup_{\jmath\in \mathscr{I}}\left\|\varphi(\jmath, 0, 0, 0)\right\|. $ Let $ U = \left\{\mathcal{V}\in \mathscr{C}(\mathscr{I}):\left\|\mathcal{V}\right\|\leq R\right\} $. Consider $ \mathscr{F}_{1}:X\rightarrow A $ and $ \mathscr{F}_{2}:X\rightarrow A $ given as
Let $ \mathcal{V} = \mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\mathcal{V}, \mathcal{V}\in \mathscr{C}(\mathscr{I}) $ is the fractional Eq (3.1) to the problems (1.3) and (1.4).
Hence, the operators $ \mathscr{F}_{1} $ and $ \mathscr{F}_{2} $ satisfy the Krasnoselskii's fixed point theorem.
Step (ⅰ) $ \mathscr{F}_{1} $ is a contraction.
By $ {\bf{(H_1)}} $–$ {\bf{(H_3)}} $ on $ \varphi $, $ \forall $ $ \mathcal{V}, \varphi\in \mathscr{C}(\mathscr{I}) $ and $ \jmath\in \mathscr{I} $,
This gives, $ \left\|\mathscr{F}_{1}\mathcal{V}-\mathscr{F}_{2}\varphi\right\|\leq RT\left\|\mathcal{V}-\varphi\right\|, \mathcal{V}, \varphi\in \mathscr{C}(\mathscr{I}). $
Step (ⅱ) $ \mathscr{F}_{2} $ is completely continuous. By using Theorem 3.3 and Ascoli-Arzela theorem, $ \mathscr{F}_{2} = -\mathscr{F} $ is completely continuous.
Step (ⅲ) $ \mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\varphi\in U $, for any $ \mathcal{V}, \varphi\in U $, using Theorem 3.3, we obtain
By definition of $ R $, we get
Using the Eq (4.5) in (4.7), we get condition of Eq (4.4).
$ \therefore\; \left\|(\mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\varphi)(\jmath)\right\|\leq L, \jmath\in \mathscr{I}. $ This gives, $ \mathscr{F}_{1}\mathcal{V}+\mathscr{F}_{2}\varphi\in U $, $ \forall\; \mathcal{V}, \varphi\in X. $
From Steps (ⅰ)–(ⅲ), all the conditions of Lemma 2.11 follow.
Theorem 4.3. By Theorem 4.2, the Eqs (1.3) and (1.4) have a unique solution in $ \mathscr{C}(\mathscr{I}). $
Proof. (1) The problems (1.3) and (1.4) have an operator equation form as:
where,
By Theorem 4.2, Eq (4.7) is solvable in $ \mathscr{C}(\mathscr{I}) $, by Lemma 2.10 we get a unique solution of Eqs (1.3) and (1.4),
Proof. (2) Let $ \mathcal{V}, \varphi $ be solutions of Eqs (1.3) and (1.4). By fractional integral operators and $ {\bf{(H_1)}} $–$ {\bf{(H_3)}}, $ we find, for any $ \jmath\in \mathscr{I} $,
5.
Estimate solution
Theorem 5.1. By Theorem 4.2, if $ \mathcal{V}(\jmath) $ is a solution of Eqs (1.3) and (1.4), then
where, $ N_{\varphi} = \sup_{\jmath\in \mathscr{I}}\left|\varphi(\jmath, 0, 0, 0)\right|. $
Proof. If $ \mathcal{V}(\jmath) $ is a solution of Eqs (1.3) and (1.4), for all $ \jmath\in \mathscr{I}, $
By Lemma 2.12, we get
6.
Data dependence results
We discuss data dependence results for the problem
Theorem 6.1. Equation (4.2) holds, and $ \xi_{k} > 0, $ where $ k = 1, 2 $ are real numbers such that,
$ \varphi(\jmath) $ is a solution of ABR fractional derivative Eqs (6.1) and (6.2), and $ \mathcal{V}(\jmath) $ is a solution of Eqs (1.3) and (1.4).
Proof. Let $ \mathcal{V}, \varphi $ are the solution of Eqs (1.3) and (1.4), (6.1) and (6.2) respectively. We find for any
By Lemma 2.12, we get
7.
Dependence results on parameters
Let any $ \lambda, \lambda_{0}\in \mathscr{R} $ and
Theorem 7.1. Let the function $ \Theta $ satisfy Theorem 4.2. Suppose there exists $ \omega, u \in \mathscr{C}(\mathscr{I}, \mathscr{R}^{+}) $ such that,
If $ \mathcal{V}_{1}, \mathcal{V}_{2} $ are the solutions of Eqs (7.1) and (7.3), then
where $ \mathcal{P} = \sup_{\jmath \in \mathscr{I}}u(\jmath). $
Proof. Let, for any $ \jmath\in \mathscr{I} $,
By Lemma 2.12,
8.
Example
Consider a nonlinear ABR fractional derivative with neutral integro-differential equations of the form:
$ \varphi:(\mathscr{I}\times \mathscr{R}\times \mathscr{R}\times \mathscr{R})\rightarrow \mathscr{R} $ is a continuous nonlinear function such that,
and
We observe that for all $ \mathcal{V}, \varphi\in \mathscr{R} $ and $ \jmath\in \mathscr{I}, $
The function $ \varphi $ satisfies $ (H_{1}) $–$ (H_{4}) $ with constant $ \frac{1}{3} $. From Theorem 4.2, we have $ \delta = \frac{1}{2} $ and T = 2 which is substitute in Eq (4.2), and we get
If the function $ B(\delta) $ satisfies Eq (8.4), then Eqs (8.1) and (8.2) have a unique solution.
9.
Conclusions
In this research article, we explored multi-derivative nonlinear neutral fractional integro-differential equations involving the ABR fractional derivative. The elementary results of the existence, uniqueness and dependence solution on various data are based on the Prabhakar fractional integral operator $ \varepsilon^{\alpha}_{\delta, \eta, \mathcal{V}; c+} $ involving a generalized ML function. The existence results are obtained by Krasnoselskii's fixed point theorem, and the uniqueness and data dependence results are obtained by the Gronwall-Bellman inequality with continuous functions.
Acknowledgments
The research on Existence and data dependence results for neutral fractional order integro-differential equations by Khon Kaen University has received funding support from the National Science, Research and Innovation Fund.
Conflict of interest
The authors declare no conflict of interest.















 DownLoad:
DownLoad: