Citation: H.T. Banks, S. Dediu, H.K. Nguyen. Sensitivity of dynamical systems to parameters in a convex subset of a topological vector space[J]. Mathematical Biosciences and Engineering, 2007, 4(3): 403-430. doi: 10.3934/mbe.2007.4.403
| [1] | Marco Di Francesco, Simone Fagioli, Massimiliano D. Rosini . Many particle approximation of the Aw-Rascle-Zhang second order model for vehicular traffic. Mathematical Biosciences and Engineering, 2017, 14(1): 127-141. doi: 10.3934/mbe.2017009 |
| [2] | Francesca Marcellini . The Riemann problem for a Two-Phase model for road traffic with fixed or moving constraints. Mathematical Biosciences and Engineering, 2020, 17(2): 1218-1232. doi: 10.3934/mbe.2020062 |
| [3] | Léon Masurel, Carlo Bianca, Annie Lemarchand . Space-velocity thermostatted kinetic theory model of tumor growth. Mathematical Biosciences and Engineering, 2021, 18(5): 5525-5551. doi: 10.3934/mbe.2021279 |
| [4] | Le Li, Lihong Huang, Jianhong Wu . Flocking and invariance of velocity angles. Mathematical Biosciences and Engineering, 2016, 13(2): 369-380. doi: 10.3934/mbe.2015007 |
| [5] | Yoichi Enatsu, Yukihiko Nakata, Yoshiaki Muroya . Global stability for a class of discrete SIR epidemic models. Mathematical Biosciences and Engineering, 2010, 7(2): 347-361. doi: 10.3934/mbe.2010.7.347 |
| [6] | John Cleveland . Basic stage structure measure valued evolutionary game model. Mathematical Biosciences and Engineering, 2015, 12(2): 291-310. doi: 10.3934/mbe.2015.12.291 |
| [7] | Max-Olivier Hongler, Roger Filliger, Olivier Gallay . Local versus nonlocal barycentric interactions in 1D agent dynamics. Mathematical Biosciences and Engineering, 2014, 11(2): 303-315. doi: 10.3934/mbe.2014.11.303 |
| [8] | Nawaz Ali Zardari, Razali Ngah, Omar Hayat, Ali Hassan Sodhro . Adaptive mobility-aware and reliable routing protocols for healthcare vehicular network. Mathematical Biosciences and Engineering, 2022, 19(7): 7156-7177. doi: 10.3934/mbe.2022338 |
| [9] | Hans F. Weinberger, Xiao-Qiang Zhao . An extension of the formula for spreading speeds. Mathematical Biosciences and Engineering, 2010, 7(1): 187-194. doi: 10.3934/mbe.2010.7.187 |
| [10] | Carole Guillevin, Rémy Guillevin, Alain Miranville, Angélique Perrillat-Mercerot . Analysis of a mathematical model for brain lactate kinetics. Mathematical Biosciences and Engineering, 2018, 15(5): 1225-1242. doi: 10.3934/mbe.2018056 |
Portfolio theory suggests that investors can lower their risk and increase their returns through diversification. Different security types (ex. bonds, commodities, real estate and etc.) as well as international equities are commonly used to diversify equity portfolios (Solnik and Noetzlin, 1982; Qrauer and Hakansson, 1987; Levy and Lerman, 1988; Eichholtz, 1996; Benartzi and Thaler, 2001).
Despite research evidence for the benefits of diversification, investors are reported to be rather reluctant to truly diversify their portfolios. For instance, French and Poterba (1991) show that investors in the US, Japan and the UK prefer to invest in their own domestic markets. More recently, Goetzmann and Kumar (2008) show that US investors are not too keen on diversification and often tend to own under-diversified portfolios. However, "...benefits from international diversification are so large that they should rapidly resuscitate the development in the U.S. of successful international mutual funds..." (Solnik, 1995).
There are many international investment opportunities that would be appealing to investors. Comparisons of twenty international indexes along with the S & P-500 index are provided in Figure 1. It is evident that at least five of these indexes provide higher returns than S & P-500 at a lower risk. There are indexes that offer much higher returns at a slight increase in risk. Why then, investors under-diversify, especially internationally? According to Goetzmann and Kumar (2008), the reasoning could be related to over-confidence or investor bias for domestic investment choices.
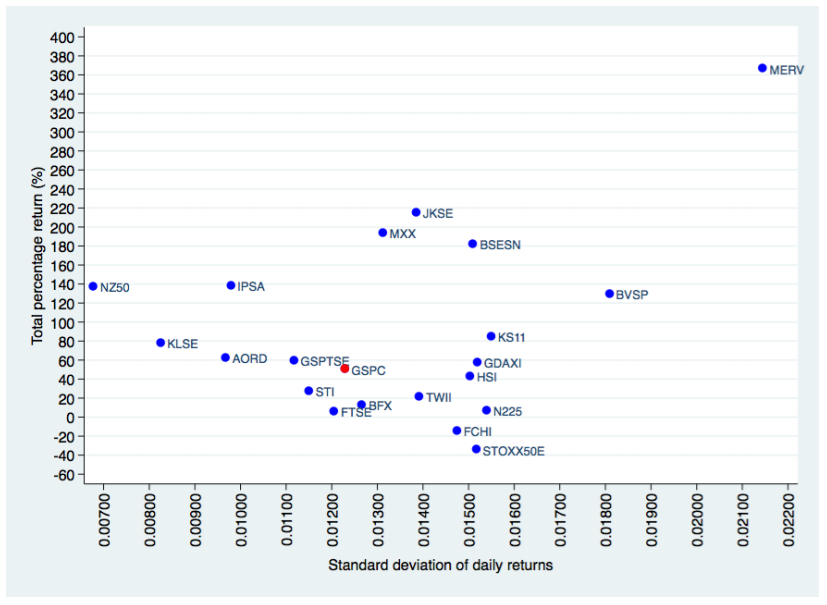 Figure 1. Risk and return chart for all indexes included in the study. Period begins with January, 2000 and ends on July, 2017. End of day closing values for indexes are obtained from Yahoo! Finance (via http://finance.yahoo.com) and confirmed by the data obtained from Google Finance (via http://google.com/finance). Index names and notations are used as provided by Yahoo! Finance. Daily (d) returns are calculated as log difference of daily closing values.
Figure 1. Risk and return chart for all indexes included in the study. Period begins with January, 2000 and ends on July, 2017. End of day closing values for indexes are obtained from Yahoo! Finance (via http://finance.yahoo.com) and confirmed by the data obtained from Google Finance (via http://google.com/finance). Index names and notations are used as provided by Yahoo! Finance. Daily (d) returns are calculated as log difference of daily closing values.We are not arguing that there is no international diversification. In fact, we note that there is a growing population of international mutual funds and ETFs as predicted by Solnik (1995). For instance, a US ETF, EEM (iShares MSCI Emerging Markets ETF), * has assets totaling about $32 billion. Similarly, another popular US ETF, EFA (iShares MSCI EAFE ETF), † has assets totaling about $76 billion. Many of the US corporations have international operations that provide US investors with international risk and return exposure (Agmon and Lessard, 1977; Choi, 1989; Nance et al., 1993).
*More information is available via https://finance.yahoo.com/quote/EEM?p = EEM
†More information is available via https://finance.yahoo.com/quote/EFA?p = EFA
We therefore question whether the reasoning behind under-diversification through international equities is because of the quick adjustments in international returns for US implied volatility. In other words, if international equity returns adjust to US risk quickly, then the investors may be perceiving these markets as highly integrated with the US and thus not offering potential diversification benefits. While in the long run, several of these markets prove to be valuable diversification alternatives, future looking investments may ignore past performances and focus on short-term market reactions.
Equity indexes from twenty countries are evaluated with this study for the period that begins with January, 2000 and ends on July, 2017. US implied volatility leads seventeen of the twenty international index returns with two trading days lag. Statistically significant coefficients for the lags of one and two trading days are all negative. It is therefore evident that international equity returns adjust quickly to US implied volatility.
Earnings yield (i.e. earnings to price, E/P, ratio) is an important measure of risk and return trade off (Basu, 1983; Rogers, 1988; Jaffe et al., 1989; Dicle, 2017). It is based on the idea that stocks, like bonds, provide returns to investors. The earnings per share (realized or expected) for a stock divided by the trading price of the stock results in a measure similar to the bond yield. Within the concept of earnings yield, as risk increases, stock price would be expected to decrease to adjust for risk. This is not a violation of the risk-return tradeoff theory. In fact, Dicle (2017) argues that investors, assuming rational behavior, try to maximize return for given level of risk. At the time of their investment, investors predict the future return and risk levels. Thus, ex-ante, the risk-return tradeoff would be intact. However, ex-post, the risk and return levels can be quite different than the predicted levels. During the investment period, the prices can adjust to changes in risk and they can deviate significantly from their predicted levels. Thus, the return response for increased risk would be negative. This is the response we report with international indexes' returns to US implied volatility. Given this evidence, investors looking to diversify their US equity risk with international equities may find that US risk is adjusted in international equity returns.
In addition to the diversification implication, the evidence also has implications for predictability of returns. Based on the earnings yield adjustments of returns to volatility, it may be possible to predict international equity returns using US implied volatilities.
Random walk theory posits that the security prices do not follow a predictable pattern (Fama, 1965b; Horne and Parker, 1967; Levy, 1967; Jensen and Benington, 1970; Malkiel, 1999). It would imply that neither historic returns or returns of other assets should be able to predict future returns. It would also mean that securities in other countries would have no predictive power. Similarly, market efficiency theory argues that security prices reflect all available information (Fama, 1965a; Malkiel and Fama, 1970; Fama, 1998). Thus, if there is any information that would help investors to predict future prices then, according to the market efficiency theory, it would already be priced. Accordingly, it would not be possible to predict future prices.
There is however extensive research into prediction of returns. For instance, evaluating mean reversion, Poterba and Summers (1988) find that "stock returns exhibit positive serial correlation over short periods and negative correlation over longer intervals". Further evidence of return predictability is offered by Fama and French (1988) analyzing return autocorrelations, by Fama and French (1988b) based on dividend yields, by Fama and French (1989b) based on economic conditions and business-cycles and by Campbell and Shiller (1988) using excess volatility. In fact, in his survey Cochrane (1999) notes that "Now we recognize that stock and bond returns have a substantial predictable component at long horizons". Lewellen (2004) provides evidence of return predictability using dividend yield as well as earnings yield. In a similar analyisis, Campbell and Yogo (2006) provide evidence of return predictability with variables such as earnings to price ratio (i.e. earnings yield), dividend to price ratio (i.e. dividend yield) and measures of interest rates. Our earnings yield discussion about returns adjusting to volatility draws directly from the findings of Lewellen (2004) and Campbell and Yogo (2006) that are based on yield variables. As yields would be expected to react to changes in risk so would the returns. Return reaction to risk is not solely based on risk to return trade-off but also based on earnings yield explanation for stocks. On a contrasting note however Hjalmarsson (2010) provide evidence against the predictive power of yield based variables (i.e. earnings yield). Their evidence is in favor of predictability using interest rate measures similar in part to the findings of Campbell and Yogo (2006).
There is also evidence that international returns can be predicted with US equity returns. For instance, Rapach et al. (2013) "...show that lagged U.S. returns predict returns in numerous non-U.S. industrialized countries substantially better than the countries' own economic variables...".‡ There could be many reasons for the US markets to lead the markets in other countries and therefore to have predictive power over foreign equity returns. For instance, Rapach et al. (2013) "...posit that many investors focus more intently on the U.S. market...". They also recognize that the explanations that are based on risk could also be the reasons for the US markets' lead. Similarly, Morana and Beltratti (2008) find strong evidence for the correlations across equity markets in terms of returns as well as volatility. They also report that these comovements have a positive trend. These findings are in line with earlier evidence (Karolyi and Stulz, 1996; Ang and Bekaert, 1999; Ball and Torous, 2000). In fact, Ang and Bekaert (1999) report "high volatility-high correlation regime which tends to coincide with a bear market". Similarly, Ball and Torous (2000) report dynamically changing correlation structures.
‡A detailed summary of the literature on prediction of returns for the US markets as well as for the international markets is provided by Rapach et al.(2013a, b).
Evidence of the relationship between international return correlations and volatility is provided by Solnik et al. (1996) among others. In fact, they conclude that benefits of international diversification are reduced because of the increased correlations across countries. Ramchand and Susmel (1998) confirm these findings and report increased correlations between the US markets and other international markets during the times of high volatility.
It is therefore well established that several yield based variables can predict returns. It is also established that there is high correlation between international equity returns and in fact these correlations are higher during the times of high volatility. Based on these correlations, US equity returns predict international equity returns and this predictability would be expected to be higher with higher volatility. We argue that the foreign equity markets' returns respond not only to the contemporaneous US returns but also to the US implied volatility. In effect, this is to argue that US implied volatility can predict international equity returns.
Supporting evidence, in part, to our argument is provided by Sarwar (2012a) in terms of US implied volatilities and US market returns. He reports contemporaneous (not predictive) and negative correlations between the volatility and returns. The negative coefficients are further evidence of the earnings yield argument. Sarwar (2012b) extends these findings to evaluate US implied volatility and returns for equities in Brazil, Russia, India, and China (BRIC). Similarly he reports negative contemporaneous correlations for BRIC countries. Furthermore, he confirms the earlier findings by Ramchand and Susmel (1998) that volatility-return relationship is stronger during times of high volatility. Evaluation of the US volatility (VIX) vs. international equity returns is extended to several European markets by Sarwar (2014). Previously reported negative contemporaneous relationship is further confirmed for the European countries. In support of our argument that international equity returns adjust to US volatility, Sarwar (2014) finds that VIX can predict equity returns in the analyzed European counties. However, this predictive ability is limited to the financial crisis periods. Interestingly, the coefficients for the leads and lags provided in Sarwar (2014) (Table 3) have mixed signs. Any proof for our argument would require all negative coefficients for all countries. We also note that since it is well established in the literature that US returns predict foreign equity returns, controlling for US market returns within these estimations would provide more robust results for the predictive power of the VIX. More recent study Sarwar and Khan (2017) extend a similar analysis to emerging markets and report similar results.
Our contribution to the related literature is unique for multiple reasons: ⅰ) we analyze lead and lag relationships as well as causality, ⅱ) list of countries analyzed is the most extensive in the related literature, ⅲ) sample period includes the 2007/2008 US financial crisis which is controlled using a binary variable, ⅳ) empirical analysis recognizes the predictive power of the S & P-500 which is controlled in all estimations, and finally, ⅴ) empirical analysis recognizes the robustness of GARCH(1, 1) model for the lead-lag relationship even in the case of implied volatilities. We also believe that in light of the evidence for the predictive power of S & P-500, any analysis of predictive power for any other variable would fall short of robustness. In fact, a general market model for estimating market correlations would require S & P-500 as the market portfolio.
Our evidence is also unique because: ⅰ) the results point to overwhelming return reaction to US implied volatility, ⅱ) estimated coefficients between implied volatility and returns are consistently negative, and ⅲ) there is consistent and almost uniform Granger causality from US implied volatility towards international returns.
This evidence is important for investors who are looking to diversify US volatility as they create doubt for the importance of international equity markets as a diversification vehicle. It also provides strong support for predictability of returns as well as for the earnings yield arguments.
Following the common practice of using publicly available (for all investors and academics) data (Christensen and Prabhala, 1998; Aggarwal et al., 1999) in the volatility literature, we also obtain our data from public sources. Data include twenty international equity indexes (Indexd), the implied volatility index (VIXd) for the S & P-500 index and the S & P-500 index itself (SP500d). Frequency of the data is daily (d) for all variables. The time span begins with January 2000 except for Chile (2002) and New Zealand (2003). Ending period is July, 2017. End of day closing values for the indexes are obtained from Yahoo! Finance§ (YF) and confirmed by the data obtained from Google Finance.¶ Index names and symbols are used as provided by YF. End of day closing values for the implied volatility index (VIX) are obtained from Chicago Board Options Exchange (CBOE).‖
§Available via http://finance.yahoo.com
¶Available via http://google.com/finance
‖Available via http://cboe.com
Daily returns are calculated as log difference of daily closing values and denoted with Δ. A binary variable, Crisisd is created to control for the 2007/2008 US financial crisis. This variable is assigned a value of one for the period January 1st, 2007 through March 9th, 2009 and zero otherwise. Since the financial crisis began on February 28th, 2007**, our crisis binary variable begins with the calendar year of 2007. Also, since the financial crisis began to fade on December 19th, 2008††, our crisis variable extends a few more months to mark the lowest point S & P-500 index has seen after the financial crisis began.
**"Freddie Mac Tightens Standards" available on http://www.nytimes.com/2007/02/28/business/28mortgage.html
††"Bush announces auto rescue" available on http://money.cnn.com/2008/12/19/news/companies/auto_crisis/
Descriptive statistics for the variables are provided in Table 1. Based on the augmented Dickey-Fuller (Dickey and Fuller, 1979; Fuller, 2009) unit root tests, all variables are stationary at their log difference returns.
| Country | Index | Notation | First | Mean | Min. | Max. | Stdev. | DF-z | |
| Argentina | MERVAL | ΔMERVd | 01/03/2000 | 0.0008 | -0.1295 | 0.1612 | 0.0215 | -61.7973 | *** |
| Australia | All ordinaries | ΔAORDd | 01/03/2000 | 0.0001 | -0.0855 | 0.0536 | 0.0096 | -63.7009 | *** |
| Belgium | BEL 20 | ΔBFXd | 01/03/2000 | 0.0001 | -0.0832 | 0.0933 | 0.0126 | -62.3348 | *** |
| Brazil | IBOVESPA | ΔBVSPd | 01/03/2000 | 0.0004 | -0.1210 | 0.1368 | 0.0181 | -64.4290 | *** |
| Canada | S & P/TSX composite index | ΔGSPTSEd | 01/03/2000 | 0.0001 | -0.0979 | 0.0937 | 0.0112 | -66.6040 | *** |
| Chile | IPSA | ΔIPSAd | 01/03/2002 | 0.0004 | -0.0724 | 0.1180 | 0.0098 | -51.7155 | *** |
| France | CAC 40 | ΔFCHId | 01/03/2000 | -0.0000 | -0.0947 | 0.1059 | 0.0147 | -68.4651 | *** |
| Germany | DAX | ΔGDAXId | 01/03/2000 | 0.0001 | -0.0734 | 0.1080 | 0.0151 | -67.2659 | *** |
| Hong Kong | Hang Seng index | ΔHSId | 01/03/2000 | 0.0001 | -0.1358 | 0.1341 | 0.0150 | -66.0974 | *** |
| India | S & P BSE SENSEX | ΔBSESNd | 01/03/2000 | 0.0004 | -0.1181 | 0.1599 | 0.0151 | -60.8319 | *** |
| Indonesia | Jakarta composite index | ΔJKSEd | 01/05/2000 | 0.0005 | -0.1131 | 0.0762 | 0.0139 | -57.6583 | *** |
| Japan | Nikkei 225 | ΔN225d | 01/05/2000 | -0.0000 | -0.1211 | 0.1323 | 0.0154 | -66.8286 | *** |
| Malaysia | FTSE Bursa Malaysia | ΔKLSEd | 01/03/2000 | 0.0002 | -0.0998 | 0.0450 | 0.0082 | -56.3397 | *** |
| Mexico | IPC | ΔMXXd | 01/03/2000 | 0.0004 | -0.0827 | 0.1044 | 0.0132 | -59.2160 | *** |
| New Zealand | S & P/NZX 50 index gross | ΔNZ50d | 01/06/2003 | 0.0003 | -0.0494 | 0.0581 | 0.0068 | -52.7519 | *** |
| Singapore | STI Index | ΔSTId | 01/03/2000 | 0.0001 | -0.0909 | 0.0753 | 0.0115 | -63.9297 | *** |
| South Korea | KOSPI composite Index | ΔKS11d | 01/05/2000 | 0.0002 | -0.1237 | 0.1128 | 0.0154 | -63.6613 | *** |
| Switzerland | ESTX50 EUR | ΔSTOXX50Ed | 01/03/2000 | -0.0001 | -0.0901 | 0.1044 | 0.0151 | -67.0996 | *** |
| Taiwan | TSEC weighted index | ΔTWIId | 01/05/2000 | 0.0000 | -0.0994 | 0.0652 | 0.0139 | -62.1012 | *** |
| United Kingdom | FTSE 100 | ΔFTSEd | 01/05/2000 | 0.0000 | -0.0926 | 0.0938 | 0.0120 | -68.4001 | *** |
| United States | S & P-500 | ΔSP500d | 01/05/2000 | 0.0001 | -0.0947 | 0.1096 | 0.0123 | -71.0393 | *** |
| United States | VIX | ΔVIXd | 01/05/2000 | -0.0006 | -0.3506 | 0.4960 | 0.0666 | -71.3406 | *** |
The lead-lag relationship between the VIX and the international markets' returns is evaluated using a Granger non-causality model (Granger, 1969). Just as causality is important to establish for the US volatility effect (i.e. the Wald test results), the sign of the effect is also important. A positive coefficient would mean flight from US volatility towards international markets. A negative coefficient would mean international returns adjusting for US expected risk (i.e. earnings yield). Thus, we estimate the lead-lag coefficients using a GARCH model (Engle, 1982; Bollerslev, 1986) and then estimate the Granger model for the causal relationships. For the GARCH model, both GARCH and ARCH terms are used as one (i.e. GARCH (1, 1)) following Hansen and Lunde (2005) and estimated as follows:
| ΔIndexd=β0+β1ΔIndexd−1+β2ΔIndexd−2+β3ΔVIXd−1+β4ΔVIXd−2+β5ΔSP500d+β6Crisisd+ϵdσ2d=α0+α1ϵ2d−1+α2σ2d−1 where ϵd|δd−1∼N(0,σ2d) | (1) |
Equation 1 is estimated for each of the twenty international equity indexes. ΔIndex is assigned a different index for each of the estimations. Table 2 provides the results for the estimation of the Equation 1 for each of the twenty indexes.
| Country | Index | ΔIndexd−1 | ΔIndexd−2 | ΔVIXd−1 | ΔVIXd−2 | ΔSP500d | Crisis | Constant | χ2 | N | |||||||
| Argentina | ΔMERVd | 0.0733 | *** | 0.0089 | -0.0046 | -0.0008 | 0.8775 | *** | -0.0007 | 0.0008 | *** | 2,339.38 | 4,164 | ||||
| Australia | ΔAORDd | -0.1054 | *** | 0.0206 | -0.0370 | *** | -0.0098 | *** | 0.2415 | *** | 0.0002 | 0.0003 | *** | 1,416.64 | 3,893 | ||
| Belgium | ΔBFXd | -0.0565 | *** | -0.0189 | -0.0328 | *** | -0.0035 | * | 0.5198 | *** | -0.0009 | ** | 0.0004 | *** | 3,027.19 | 4,346 | |
| Brazil | ΔBVSPd | -0.0029 | -0.0041 | -0.0086 | *** | 0.0045 | 0.9166 | *** | 0.0010 | * | 0.0001 | 3,368.37 | 4,214 | ||||
| Canada | ΔGSPTSEd | 0.0163 | -0.0165 | -0.0097 | *** | -0.0013 | 0.6097 | *** | 0.0002 | 0.0001 | 6,479.54 | 4,335 | |||||
| Chile | ΔIPSAd | 0.1711 | *** | -0.0445 | *** | -0.0068 | *** | -0.0008 | 0.3584 | *** | 0.0003 | 0.0004 | *** | 1,733.03 | 3,758 | ||
| France | ΔFCHId | -0.1342 | *** | -0.0486 | *** | -0.0414 | *** | -0.0092 | *** | 0.7145 | *** | -0.0003 | 0.0002 | 4,112.15 | 4,348 | ||
| Germany | ΔGDAXId | -0.0983 | *** | -0.0369 | *** | -0.0392 | *** | -0.0111 | *** | 0.7413 | *** | 0.0001 | 0.0003 | * | 4,091.56 | 4,328 | |
| Hong Kong | ΔHSId | -0.0521 | *** | 0.0006 | -0.0605 | *** | -0.0131 | *** | 0.2014 | *** | 0.0004 | 0.0002 | 1,003.37 | 4,198 | |||
| India | ΔBSESNd | 0.0406 | ** | -0.0113 | -0.0320 | *** | -0.0072 | *** | 0.2432 | *** | 0.0001 | 0.0006 | *** | 793.32 | 4,195 | ||
| Indonesia | ΔJKSEd | 0.0798 | *** | -0.0171 | -0.0377 | *** | 0.0045 | * | 0.0967 | *** | -0.0003 | 0.0008 | *** | 478.03 | 4,107 | ||
| Japan | ΔN225d | -0.0895 | *** | 0.0273 | * | -0.0739 | *** | -0.0183 | *** | 0.1624 | *** | -0.0006 | 0.0003 | * | 996.37 | 4,143 | |
| Malaysia | ΔKLSEd | 0.1176 | *** | 0.0164 | -0.0238 | *** | -0.0021 | * | 0.0483 | *** | 0.0004 | 0.0002 | *** | 602.95 | 4,172 | ||
| Mexico | ΔMXXd | 0.0797 | *** | -0.0302 | ** | -0.0048 | ** | 0.0008 | 0.6753 | *** | -0.0003 | 0.0004 | *** | 5,139.27 | 4,265 | ||
| New Zealand | ΔNZ50d | 0.0519 | *** | 0.0264 | -0.0013 | 0.0000 | 0.2828 | *** | -0.0006 | ** | 0.0004 | *** | 1,246.25 | 2,801 | |||
| Singapore | ΔSTId | -0.0333 | ** | 0.0044 | -0.0373 | *** | -0.0063 | *** | 0.1815 | *** | -0.0001 | 0.0002 | * | 894.90 | 4,269 | ||
| South Korea | ΔKS11d | -0.0465 | *** | -0.0118 | -0.0453 | *** | -0.0112 | *** | 0.1914 | *** | 0.0003 | 0.0004 | ** | 843.35 | 4,180 | ||
| Switzerland | ΔSTOXX50Ed | -0.1285 | *** | -0.0448 | *** | -0.0425 | *** | -0.0103 | *** | 0.7325 | *** | -0.0003 | 0.0001 | 3,765.37 | 4,224 | ||
| Taiwan | ΔTWIId | 0.0020 | 0.0144 | -0.0434 | *** | -0.0074 | *** | 0.1639 | *** | -0.0002 | 0.0003 | * | 857.67 | 4,164 | |||
| United Kingdom | ΔFTSEd | -0.1329 | *** | -0.0530 | *** | -0.0357 | *** | -0.0084 | *** | 0.5435 | *** | -0.0003 | 0.0001 | 3,834.85 | 4,318 | ||
The autoregressive components for index returns (ΔIndexd−1) for almost all of the indexes are statistically significant at 5% or better. The sign however is not as uniform. While seven of the indexes have positive autoregressive coefficients, ten have negative coefficients.
The effect of contemporaneous S & P-500 index returns (ΔSP500d) is statistically significant at 1% and positive for all of the indexes tested. These results suggest the importance of the US markets' returns over the international markets' returns. Countries such as Brazil, Argentina and Germany have the highest coefficients with the S & P-500 index. On the other hand, Malaysia, Indonesia and Taiwan have the lowest coefficients. The statistical significance of the S & P-500 index returns prove that omission of this variable in econometric models of implied volatility would clearly lead to omitted variable bias.
Interestingly, the results for the binary variable for the the 2007/2008 US financial crisis (Crisis) are quite insignificant. We posit that the lack of statistical significance for the US financial crisis binary variable is due to having S & P-500 index as a control variable. The impact of the 2007/2008 crisis on international equity returns is captured by the market model that includes the daily US market returns.
In terms of the US volatility index (ΔVIX), eighteen of the twenty indexes are statistically significant at 5% or better for the one day lag (ΔVIXd−1). All significant coefficients are negative which implies return adjustment (earnings yield) by international equities for US implied volatility. Similarly for two days lag (ΔVIXd−1), eleven of the twenty indexes are statistically significant at 1% level and negative.
Now that we established the lead-lag coefficients between US implied volatilities and international equity returns, we turn our attention to causality. The Granger non-causality model is estimated as follows:
| ΔVIXd=γ0+γ1ΔVIXd−1+γ2ΔVIXd−2+γ3ΔIndexd−1+γ4ΔIndexd−2+γ5ΔSP500d+γ6Crisisd+ϵ1dΔIndexd=ω0+ω1ΔIndexd−1+ω2ΔIndexd−2+ω3ΔVIXd−1+ω4ΔVIXd−2+ω5ΔSP500d+ω6Crisisd+ϵ2d | (2) |
Similar to the Equation 1, Equations 2 and 3 are estimated with trading day lags of two. Also, effect of the S & P-500 index returns on international equity returns is controlled with the contemporaneous SP500d returns. Even though the estimation results for Equation 1 did not provide any evidence of importance for the US financial crisis of 2007/2008, we still control for the crisis using the Crisis binary variable. Equations 2 and 3 are estimated as a system using seemingly unrelated regressions.(Zellner, 1962; Geweke, 1982) Causality of US implied volatilities and international equity returns are tested with Wald test (Engle, 1984) as follows:
| ΔIndexd→ΔVIXd:γ3=γ4=0 | (4) |
| ΔVIXd→ΔIndexd:ω3=ω4=0 | (5) |
The results for Equations 4 and 5 are provided in the Table 3. We find that seventeen of the twenty indexes have a statistically significant (at 5% or better) causal relationship from US implied volatility to international equity markets' returns. This important evidence, coupled with the evidence from Garch (1, 1) Equation 1, imply that international equity markets' returns adjust to US implied volatility with a very short lag. The responsiveness of these markets to US expected risk shows how integrated these international markets are to the US financial markets. Investors who seek to diversify US risk may not able able to find shelter with international equity markets as they seem to adjust to US risk fairly quickly.
| Country | Index (y) | VIX (x) | Fy→x | Fx→y | ||
| Argentina | ΔMERVd | ΔVIXd | 1.6352 | 3.1867 | ||
| Australia | ΔAORDd | ΔVIXd | 3.2358 | 433.9916 | *** | |
| Belgium | ΔBFXd | ΔVIXd | 4.1014 | 241.9900 | *** | |
| Brazil | ΔBVSPd | ΔVIXd | 6.1516 | ** | 7.0556 | ** |
| Canada | ΔGSPTSEd | ΔVIXd | 27.0668 | *** | 34.9830 | *** |
| Chile | ΔIPSAd | ΔVIXd | 2.3663 | 17.4938 | *** | |
| France | ΔFCHId | ΔVIXd | 5.6285 | * | 348.7822 | *** |
| Germany | ΔGDAXId | ΔVIXd | 4.2677 | 264.5841 | *** | |
| Hong Kong | ΔHSId | ΔVIXd | 0.1643 | 580.2403 | *** | |
| India | ΔBSESNd | ΔVIXd | 6.0031 | ** | 165.6883 | *** |
| Indonesia | ΔJKSEd | ΔVIXd | 1.0822 | 235.3652 | *** | |
| Japan | ΔN225d | ΔVIXd | 5.9154 | * | 667.4963 | *** |
| Malaysia | ΔKLSEd | ΔVIXd | 0.2587 | 293.2344 | *** | |
| Mexico | ΔMXXd | ΔVIXd | 8.3291 | ** | 4.1649 | |
| New Zealand | ΔNZ50d | ΔVIXd | 0.7931 | 1.6962 | ||
| Singapore | ΔSTId | ΔVIXd | 1.8026 | 407.6022 | *** | |
| South Korea | ΔKS11d | ΔVIXd | 0.6222 | 386.3308 | *** | |
| Switzerland | ΔSTOXX50Ed | ΔVIXd | 10.4257 | *** | 312.5596 | *** |
| Taiwan | ΔTWIId | ΔVIXd | 3.3380 | 330.6689 | *** | |
| United Kingdom | ΔFTSEd | ΔVIXd | 9.1058 | ** | 411.4937 | *** |
In terms of the application of financial research it is imperative that our findings are economically significant as well as statistically significant. Our final empirical analysis tests the economic significance of US implied volatility as a predictor of international returns. We employ a simple trading strategy‡‡ based on our econometric results to test for economic significance.
‡‡The strategy provided here is for educational and informational purposes only. It is not financial advice and should not be employed for any purpose other than education and further research. We disclaim any and all liability.
The trading rule has a buy signal when the US implied volatility (VIX) falls more than 2.5%. As risk goes down, we would expect the yield on the indexes to go down as well. For yield on the index to go down, we would need the stock price to go up. Thus, a drop in the VIX would be expected to produce positive returns. The trading rule is implemented on a daily basis for each of the 18 years included in our study. Trading rule returns are summed into annual returns. To compare the trading rule returns for economic significance, we also calculate the total annual returns for each year as a buy-hold strategy.
Table 4 provides the t-test results comparing total annual returns for the trading rule and for the buy-hold strategy. All of the indexes, except for Argentina, Brazil, Chile, Mexico and New Zealand, show significantly higher returns for the trading rule compared to the buy-hold strategy returns. We note that among the indexes that do not have significantly higher returns, only the returns for Argentina and for New Zealand are actually lower compared to the returns for the buy-hold strategy. For indexes such as Hong-Kong and Japan, average annual returns for the trading strategy are above 40%. These economically significant results are in support of our previous econometric evidence.
| Trading rule (x) | Buy-hold strategy (y) | ||||||||||
| Country | Index | μ | σ | N | μ | σ | N | t | x−y<0 | x−y≠0 | x−y>0 |
| Argentina | MERV | 0.1465 | 0.1567 | 18 | 0.1822 | 0.3779 | 18 | -0.4047 | |||
| Australia | AORD | 0.2102 | 0.0948 | 18 | 0.0267 | 0.1908 | 18 | 4.6212 | *** | *** | |
| Belgium | BFX | 0.1870 | 0.0941 | 18 | 0.0136 | 0.2417 | 18 | 2.6013 | ** | *** | |
| Brazil | BVSP | 0.0862 | 0.1585 | 18 | 0.0829 | 0.2905 | 18 | 0.0673 | |||
| Canada | GSPTSE | 0.0961 | 0.0860 | 18 | 0.0280 | 0.1585 | 18 | 1.9022 | * | ** | |
| Chile | IPSA | 0.1295 | 0.0908 | 16 | 0.0887 | 0.1987 | 16 | 0.8932 | |||
| France | FCHI | 0.2241 | 0.1225 | 18 | -0.0054 | 0.1922 | 18 | 3.4418 | *** | *** | |
| Germany | GDAXI | 0.1865 | 0.1106 | 18 | 0.0260 | 0.2331 | 18 | 2.6856 | ** | *** | |
| Hong Kong | HSI | 0.4084 | 0.2095 | 18 | 0.0244 | 0.2381 | 18 | 5.0133 | *** | *** | |
| India | BSESN | 0.2387 | 0.1683 | 18 | 0.0920 | 0.3139 | 18 | 1.8288 | * | ** | |
| Indonesia | JKSE | 0.2613 | 0.2044 | 18 | 0.1107 | 0.3196 | 18 | 1.9475 | * | ** | |
| Japan | N225 | 0.4337 | 0.1985 | 18 | -0.0033 | 0.2304 | 18 | 5.5651 | *** | *** | |
| Malaysia | KLSE | 0.1768 | 0.1060 | 18 | 0.0436 | 0.1839 | 18 | 2.9748 | *** | *** | |
| Mexico | MXX | 0.1255 | 0.1370 | 18 | 0.1033 | 0.1916 | 18 | 0.5793 | |||
| New Zealand | NZ50 | 0.0226 | 0.0723 | 15 | 0.0585 | 0.1510 | 15 | -1.2657 | |||
| Singapore | STI | 0.2614 | 0.1461 | 18 | 0.0218 | 0.2374 | 18 | 3.9074 | *** | *** | |
| South Korea | KS11 | 0.3682 | 0.1865 | 18 | 0.0429 | 0.2805 | 18 | 3.9891 | *** | *** | |
| Switzerland | STOXX50E | 0.2082 | 0.1125 | 18 | -0.0163 | 0.1999 | 18 | 3.4946 | *** | *** | |
| Taiwan | TWII | 0.2725 | 0.1649 | 18 | 0.0027 | 0.2910 | 18 | 3.9516 | *** | *** | |
| United Kingdom | FTSE | 0.2011 | 0.1150 | 18 | 0.0029 | 0.1445 | 18 | 3.6737 | *** | *** | |
Is it possible to predict returns? This study provides evidence that, in the case of equity returns in twenty countries, US implied volatility has predictive power. There are two main implications for this finding: ⅰ) international equities may not offer much of a diversification benefit for US volatility, ⅱ) returns may be predictable using volatility. We argue that the channel in which returns react to volatility is based on the earnings yield argument. If the earnings yield for stocks is treated similar to bond yields then it would be natural to expect bond like reaction to higher risk for stocks. As risk increases, higher yields would compensate investors for the higher risk. This happens through lower bond prices. Based on the earnings yield argument then higher risk would lead to higher yield which is provided immediately through lower stock prices. Expected result would be negative correlations between risk and returns. This is the evidence we report for US implied volatility and non-US equity market returns.
We believe that the stocks behave similar to bonds reacting to risk. It would be interesting for future research to see if bond price reactions coincide with stock price reactions. It would also be interesting to see which maturity structure of bonds would closely mimic the reactions of the stocks. This would extend our understanding of investment horizon for stocks.
All authors declare no conflicts of interest in this paper.
| Country | Index | Notation | First | Mean | Min. | Max. | Stdev. | DF-z | |
| Argentina | MERVAL | ΔMERVd | 01/03/2000 | 0.0008 | -0.1295 | 0.1612 | 0.0215 | -61.7973 | *** |
| Australia | All ordinaries | ΔAORDd | 01/03/2000 | 0.0001 | -0.0855 | 0.0536 | 0.0096 | -63.7009 | *** |
| Belgium | BEL 20 | ΔBFXd | 01/03/2000 | 0.0001 | -0.0832 | 0.0933 | 0.0126 | -62.3348 | *** |
| Brazil | IBOVESPA | ΔBVSPd | 01/03/2000 | 0.0004 | -0.1210 | 0.1368 | 0.0181 | -64.4290 | *** |
| Canada | S & P/TSX composite index | ΔGSPTSEd | 01/03/2000 | 0.0001 | -0.0979 | 0.0937 | 0.0112 | -66.6040 | *** |
| Chile | IPSA | ΔIPSAd | 01/03/2002 | 0.0004 | -0.0724 | 0.1180 | 0.0098 | -51.7155 | *** |
| France | CAC 40 | ΔFCHId | 01/03/2000 | -0.0000 | -0.0947 | 0.1059 | 0.0147 | -68.4651 | *** |
| Germany | DAX | ΔGDAXId | 01/03/2000 | 0.0001 | -0.0734 | 0.1080 | 0.0151 | -67.2659 | *** |
| Hong Kong | Hang Seng index | ΔHSId | 01/03/2000 | 0.0001 | -0.1358 | 0.1341 | 0.0150 | -66.0974 | *** |
| India | S & P BSE SENSEX | ΔBSESNd | 01/03/2000 | 0.0004 | -0.1181 | 0.1599 | 0.0151 | -60.8319 | *** |
| Indonesia | Jakarta composite index | ΔJKSEd | 01/05/2000 | 0.0005 | -0.1131 | 0.0762 | 0.0139 | -57.6583 | *** |
| Japan | Nikkei 225 | ΔN225d | 01/05/2000 | -0.0000 | -0.1211 | 0.1323 | 0.0154 | -66.8286 | *** |
| Malaysia | FTSE Bursa Malaysia | ΔKLSEd | 01/03/2000 | 0.0002 | -0.0998 | 0.0450 | 0.0082 | -56.3397 | *** |
| Mexico | IPC | ΔMXXd | 01/03/2000 | 0.0004 | -0.0827 | 0.1044 | 0.0132 | -59.2160 | *** |
| New Zealand | S & P/NZX 50 index gross | ΔNZ50d | 01/06/2003 | 0.0003 | -0.0494 | 0.0581 | 0.0068 | -52.7519 | *** |
| Singapore | STI Index | ΔSTId | 01/03/2000 | 0.0001 | -0.0909 | 0.0753 | 0.0115 | -63.9297 | *** |
| South Korea | KOSPI composite Index | ΔKS11d | 01/05/2000 | 0.0002 | -0.1237 | 0.1128 | 0.0154 | -63.6613 | *** |
| Switzerland | ESTX50 EUR | ΔSTOXX50Ed | 01/03/2000 | -0.0001 | -0.0901 | 0.1044 | 0.0151 | -67.0996 | *** |
| Taiwan | TSEC weighted index | ΔTWIId | 01/05/2000 | 0.0000 | -0.0994 | 0.0652 | 0.0139 | -62.1012 | *** |
| United Kingdom | FTSE 100 | ΔFTSEd | 01/05/2000 | 0.0000 | -0.0926 | 0.0938 | 0.0120 | -68.4001 | *** |
| United States | S & P-500 | ΔSP500d | 01/05/2000 | 0.0001 | -0.0947 | 0.1096 | 0.0123 | -71.0393 | *** |
| United States | VIX | ΔVIXd | 01/05/2000 | -0.0006 | -0.3506 | 0.4960 | 0.0666 | -71.3406 | *** |
| Country | Index | ΔIndexd−1 | ΔIndexd−2 | ΔVIXd−1 | ΔVIXd−2 | ΔSP500d | Crisis | Constant | χ2 | N | |||||||
| Argentina | ΔMERVd | 0.0733 | *** | 0.0089 | -0.0046 | -0.0008 | 0.8775 | *** | -0.0007 | 0.0008 | *** | 2,339.38 | 4,164 | ||||
| Australia | ΔAORDd | -0.1054 | *** | 0.0206 | -0.0370 | *** | -0.0098 | *** | 0.2415 | *** | 0.0002 | 0.0003 | *** | 1,416.64 | 3,893 | ||
| Belgium | ΔBFXd | -0.0565 | *** | -0.0189 | -0.0328 | *** | -0.0035 | * | 0.5198 | *** | -0.0009 | ** | 0.0004 | *** | 3,027.19 | 4,346 | |
| Brazil | ΔBVSPd | -0.0029 | -0.0041 | -0.0086 | *** | 0.0045 | 0.9166 | *** | 0.0010 | * | 0.0001 | 3,368.37 | 4,214 | ||||
| Canada | ΔGSPTSEd | 0.0163 | -0.0165 | -0.0097 | *** | -0.0013 | 0.6097 | *** | 0.0002 | 0.0001 | 6,479.54 | 4,335 | |||||
| Chile | ΔIPSAd | 0.1711 | *** | -0.0445 | *** | -0.0068 | *** | -0.0008 | 0.3584 | *** | 0.0003 | 0.0004 | *** | 1,733.03 | 3,758 | ||
| France | ΔFCHId | -0.1342 | *** | -0.0486 | *** | -0.0414 | *** | -0.0092 | *** | 0.7145 | *** | -0.0003 | 0.0002 | 4,112.15 | 4,348 | ||
| Germany | ΔGDAXId | -0.0983 | *** | -0.0369 | *** | -0.0392 | *** | -0.0111 | *** | 0.7413 | *** | 0.0001 | 0.0003 | * | 4,091.56 | 4,328 | |
| Hong Kong | ΔHSId | -0.0521 | *** | 0.0006 | -0.0605 | *** | -0.0131 | *** | 0.2014 | *** | 0.0004 | 0.0002 | 1,003.37 | 4,198 | |||
| India | ΔBSESNd | 0.0406 | ** | -0.0113 | -0.0320 | *** | -0.0072 | *** | 0.2432 | *** | 0.0001 | 0.0006 | *** | 793.32 | 4,195 | ||
| Indonesia | ΔJKSEd | 0.0798 | *** | -0.0171 | -0.0377 | *** | 0.0045 | * | 0.0967 | *** | -0.0003 | 0.0008 | *** | 478.03 | 4,107 | ||
| Japan | ΔN225d | -0.0895 | *** | 0.0273 | * | -0.0739 | *** | -0.0183 | *** | 0.1624 | *** | -0.0006 | 0.0003 | * | 996.37 | 4,143 | |
| Malaysia | ΔKLSEd | 0.1176 | *** | 0.0164 | -0.0238 | *** | -0.0021 | * | 0.0483 | *** | 0.0004 | 0.0002 | *** | 602.95 | 4,172 | ||
| Mexico | ΔMXXd | 0.0797 | *** | -0.0302 | ** | -0.0048 | ** | 0.0008 | 0.6753 | *** | -0.0003 | 0.0004 | *** | 5,139.27 | 4,265 | ||
| New Zealand | ΔNZ50d | 0.0519 | *** | 0.0264 | -0.0013 | 0.0000 | 0.2828 | *** | -0.0006 | ** | 0.0004 | *** | 1,246.25 | 2,801 | |||
| Singapore | ΔSTId | -0.0333 | ** | 0.0044 | -0.0373 | *** | -0.0063 | *** | 0.1815 | *** | -0.0001 | 0.0002 | * | 894.90 | 4,269 | ||
| South Korea | ΔKS11d | -0.0465 | *** | -0.0118 | -0.0453 | *** | -0.0112 | *** | 0.1914 | *** | 0.0003 | 0.0004 | ** | 843.35 | 4,180 | ||
| Switzerland | ΔSTOXX50Ed | -0.1285 | *** | -0.0448 | *** | -0.0425 | *** | -0.0103 | *** | 0.7325 | *** | -0.0003 | 0.0001 | 3,765.37 | 4,224 | ||
| Taiwan | ΔTWIId | 0.0020 | 0.0144 | -0.0434 | *** | -0.0074 | *** | 0.1639 | *** | -0.0002 | 0.0003 | * | 857.67 | 4,164 | |||
| United Kingdom | ΔFTSEd | -0.1329 | *** | -0.0530 | *** | -0.0357 | *** | -0.0084 | *** | 0.5435 | *** | -0.0003 | 0.0001 | 3,834.85 | 4,318 | ||
| Country | Index (y) | VIX (x) | Fy→x | Fx→y | ||
| Argentina | ΔMERVd | ΔVIXd | 1.6352 | 3.1867 | ||
| Australia | ΔAORDd | ΔVIXd | 3.2358 | 433.9916 | *** | |
| Belgium | ΔBFXd | ΔVIXd | 4.1014 | 241.9900 | *** | |
| Brazil | ΔBVSPd | ΔVIXd | 6.1516 | ** | 7.0556 | ** |
| Canada | ΔGSPTSEd | ΔVIXd | 27.0668 | *** | 34.9830 | *** |
| Chile | ΔIPSAd | ΔVIXd | 2.3663 | 17.4938 | *** | |
| France | ΔFCHId | ΔVIXd | 5.6285 | * | 348.7822 | *** |
| Germany | ΔGDAXId | ΔVIXd | 4.2677 | 264.5841 | *** | |
| Hong Kong | ΔHSId | ΔVIXd | 0.1643 | 580.2403 | *** | |
| India | ΔBSESNd | ΔVIXd | 6.0031 | ** | 165.6883 | *** |
| Indonesia | ΔJKSEd | ΔVIXd | 1.0822 | 235.3652 | *** | |
| Japan | ΔN225d | ΔVIXd | 5.9154 | * | 667.4963 | *** |
| Malaysia | ΔKLSEd | ΔVIXd | 0.2587 | 293.2344 | *** | |
| Mexico | ΔMXXd | ΔVIXd | 8.3291 | ** | 4.1649 | |
| New Zealand | ΔNZ50d | ΔVIXd | 0.7931 | 1.6962 | ||
| Singapore | ΔSTId | ΔVIXd | 1.8026 | 407.6022 | *** | |
| South Korea | ΔKS11d | ΔVIXd | 0.6222 | 386.3308 | *** | |
| Switzerland | ΔSTOXX50Ed | ΔVIXd | 10.4257 | *** | 312.5596 | *** |
| Taiwan | ΔTWIId | ΔVIXd | 3.3380 | 330.6689 | *** | |
| United Kingdom | ΔFTSEd | ΔVIXd | 9.1058 | ** | 411.4937 | *** |
| Trading rule (x) | Buy-hold strategy (y) | ||||||||||
| Country | Index | μ | σ | N | μ | σ | N | t | x−y<0 | x−y≠0 | x−y>0 |
| Argentina | MERV | 0.1465 | 0.1567 | 18 | 0.1822 | 0.3779 | 18 | -0.4047 | |||
| Australia | AORD | 0.2102 | 0.0948 | 18 | 0.0267 | 0.1908 | 18 | 4.6212 | *** | *** | |
| Belgium | BFX | 0.1870 | 0.0941 | 18 | 0.0136 | 0.2417 | 18 | 2.6013 | ** | *** | |
| Brazil | BVSP | 0.0862 | 0.1585 | 18 | 0.0829 | 0.2905 | 18 | 0.0673 | |||
| Canada | GSPTSE | 0.0961 | 0.0860 | 18 | 0.0280 | 0.1585 | 18 | 1.9022 | * | ** | |
| Chile | IPSA | 0.1295 | 0.0908 | 16 | 0.0887 | 0.1987 | 16 | 0.8932 | |||
| France | FCHI | 0.2241 | 0.1225 | 18 | -0.0054 | 0.1922 | 18 | 3.4418 | *** | *** | |
| Germany | GDAXI | 0.1865 | 0.1106 | 18 | 0.0260 | 0.2331 | 18 | 2.6856 | ** | *** | |
| Hong Kong | HSI | 0.4084 | 0.2095 | 18 | 0.0244 | 0.2381 | 18 | 5.0133 | *** | *** | |
| India | BSESN | 0.2387 | 0.1683 | 18 | 0.0920 | 0.3139 | 18 | 1.8288 | * | ** | |
| Indonesia | JKSE | 0.2613 | 0.2044 | 18 | 0.1107 | 0.3196 | 18 | 1.9475 | * | ** | |
| Japan | N225 | 0.4337 | 0.1985 | 18 | -0.0033 | 0.2304 | 18 | 5.5651 | *** | *** | |
| Malaysia | KLSE | 0.1768 | 0.1060 | 18 | 0.0436 | 0.1839 | 18 | 2.9748 | *** | *** | |
| Mexico | MXX | 0.1255 | 0.1370 | 18 | 0.1033 | 0.1916 | 18 | 0.5793 | |||
| New Zealand | NZ50 | 0.0226 | 0.0723 | 15 | 0.0585 | 0.1510 | 15 | -1.2657 | |||
| Singapore | STI | 0.2614 | 0.1461 | 18 | 0.0218 | 0.2374 | 18 | 3.9074 | *** | *** | |
| South Korea | KS11 | 0.3682 | 0.1865 | 18 | 0.0429 | 0.2805 | 18 | 3.9891 | *** | *** | |
| Switzerland | STOXX50E | 0.2082 | 0.1125 | 18 | -0.0163 | 0.1999 | 18 | 3.4946 | *** | *** | |
| Taiwan | TWII | 0.2725 | 0.1649 | 18 | 0.0027 | 0.2910 | 18 | 3.9516 | *** | *** | |
| United Kingdom | FTSE | 0.2011 | 0.1150 | 18 | 0.0029 | 0.1445 | 18 | 3.6737 | *** | *** | |
| Country | Index | Notation | First | Mean | Min. | Max. | Stdev. | DF-z | |
| Argentina | MERVAL | ΔMERVd | 01/03/2000 | 0.0008 | -0.1295 | 0.1612 | 0.0215 | -61.7973 | *** |
| Australia | All ordinaries | ΔAORDd | 01/03/2000 | 0.0001 | -0.0855 | 0.0536 | 0.0096 | -63.7009 | *** |
| Belgium | BEL 20 | ΔBFXd | 01/03/2000 | 0.0001 | -0.0832 | 0.0933 | 0.0126 | -62.3348 | *** |
| Brazil | IBOVESPA | ΔBVSPd | 01/03/2000 | 0.0004 | -0.1210 | 0.1368 | 0.0181 | -64.4290 | *** |
| Canada | S & P/TSX composite index | ΔGSPTSEd | 01/03/2000 | 0.0001 | -0.0979 | 0.0937 | 0.0112 | -66.6040 | *** |
| Chile | IPSA | ΔIPSAd | 01/03/2002 | 0.0004 | -0.0724 | 0.1180 | 0.0098 | -51.7155 | *** |
| France | CAC 40 | ΔFCHId | 01/03/2000 | -0.0000 | -0.0947 | 0.1059 | 0.0147 | -68.4651 | *** |
| Germany | DAX | ΔGDAXId | 01/03/2000 | 0.0001 | -0.0734 | 0.1080 | 0.0151 | -67.2659 | *** |
| Hong Kong | Hang Seng index | ΔHSId | 01/03/2000 | 0.0001 | -0.1358 | 0.1341 | 0.0150 | -66.0974 | *** |
| India | S & P BSE SENSEX | ΔBSESNd | 01/03/2000 | 0.0004 | -0.1181 | 0.1599 | 0.0151 | -60.8319 | *** |
| Indonesia | Jakarta composite index | ΔJKSEd | 01/05/2000 | 0.0005 | -0.1131 | 0.0762 | 0.0139 | -57.6583 | *** |
| Japan | Nikkei 225 | ΔN225d | 01/05/2000 | -0.0000 | -0.1211 | 0.1323 | 0.0154 | -66.8286 | *** |
| Malaysia | FTSE Bursa Malaysia | ΔKLSEd | 01/03/2000 | 0.0002 | -0.0998 | 0.0450 | 0.0082 | -56.3397 | *** |
| Mexico | IPC | ΔMXXd | 01/03/2000 | 0.0004 | -0.0827 | 0.1044 | 0.0132 | -59.2160 | *** |
| New Zealand | S & P/NZX 50 index gross | ΔNZ50d | 01/06/2003 | 0.0003 | -0.0494 | 0.0581 | 0.0068 | -52.7519 | *** |
| Singapore | STI Index | ΔSTId | 01/03/2000 | 0.0001 | -0.0909 | 0.0753 | 0.0115 | -63.9297 | *** |
| South Korea | KOSPI composite Index | ΔKS11d | 01/05/2000 | 0.0002 | -0.1237 | 0.1128 | 0.0154 | -63.6613 | *** |
| Switzerland | ESTX50 EUR | ΔSTOXX50Ed | 01/03/2000 | -0.0001 | -0.0901 | 0.1044 | 0.0151 | -67.0996 | *** |
| Taiwan | TSEC weighted index | ΔTWIId | 01/05/2000 | 0.0000 | -0.0994 | 0.0652 | 0.0139 | -62.1012 | *** |
| United Kingdom | FTSE 100 | ΔFTSEd | 01/05/2000 | 0.0000 | -0.0926 | 0.0938 | 0.0120 | -68.4001 | *** |
| United States | S & P-500 | ΔSP500d | 01/05/2000 | 0.0001 | -0.0947 | 0.1096 | 0.0123 | -71.0393 | *** |
| United States | VIX | ΔVIXd | 01/05/2000 | -0.0006 | -0.3506 | 0.4960 | 0.0666 | -71.3406 | *** |
| Country | Index | ΔIndexd−1 | ΔIndexd−2 | ΔVIXd−1 | ΔVIXd−2 | ΔSP500d | Crisis | Constant | χ2 | N | |||||||
| Argentina | ΔMERVd | 0.0733 | *** | 0.0089 | -0.0046 | -0.0008 | 0.8775 | *** | -0.0007 | 0.0008 | *** | 2,339.38 | 4,164 | ||||
| Australia | ΔAORDd | -0.1054 | *** | 0.0206 | -0.0370 | *** | -0.0098 | *** | 0.2415 | *** | 0.0002 | 0.0003 | *** | 1,416.64 | 3,893 | ||
| Belgium | ΔBFXd | -0.0565 | *** | -0.0189 | -0.0328 | *** | -0.0035 | * | 0.5198 | *** | -0.0009 | ** | 0.0004 | *** | 3,027.19 | 4,346 | |
| Brazil | ΔBVSPd | -0.0029 | -0.0041 | -0.0086 | *** | 0.0045 | 0.9166 | *** | 0.0010 | * | 0.0001 | 3,368.37 | 4,214 | ||||
| Canada | ΔGSPTSEd | 0.0163 | -0.0165 | -0.0097 | *** | -0.0013 | 0.6097 | *** | 0.0002 | 0.0001 | 6,479.54 | 4,335 | |||||
| Chile | ΔIPSAd | 0.1711 | *** | -0.0445 | *** | -0.0068 | *** | -0.0008 | 0.3584 | *** | 0.0003 | 0.0004 | *** | 1,733.03 | 3,758 | ||
| France | ΔFCHId | -0.1342 | *** | -0.0486 | *** | -0.0414 | *** | -0.0092 | *** | 0.7145 | *** | -0.0003 | 0.0002 | 4,112.15 | 4,348 | ||
| Germany | ΔGDAXId | -0.0983 | *** | -0.0369 | *** | -0.0392 | *** | -0.0111 | *** | 0.7413 | *** | 0.0001 | 0.0003 | * | 4,091.56 | 4,328 | |
| Hong Kong | ΔHSId | -0.0521 | *** | 0.0006 | -0.0605 | *** | -0.0131 | *** | 0.2014 | *** | 0.0004 | 0.0002 | 1,003.37 | 4,198 | |||
| India | ΔBSESNd | 0.0406 | ** | -0.0113 | -0.0320 | *** | -0.0072 | *** | 0.2432 | *** | 0.0001 | 0.0006 | *** | 793.32 | 4,195 | ||
| Indonesia | ΔJKSEd | 0.0798 | *** | -0.0171 | -0.0377 | *** | 0.0045 | * | 0.0967 | *** | -0.0003 | 0.0008 | *** | 478.03 | 4,107 | ||
| Japan | ΔN225d | -0.0895 | *** | 0.0273 | * | -0.0739 | *** | -0.0183 | *** | 0.1624 | *** | -0.0006 | 0.0003 | * | 996.37 | 4,143 | |
| Malaysia | ΔKLSEd | 0.1176 | *** | 0.0164 | -0.0238 | *** | -0.0021 | * | 0.0483 | *** | 0.0004 | 0.0002 | *** | 602.95 | 4,172 | ||
| Mexico | ΔMXXd | 0.0797 | *** | -0.0302 | ** | -0.0048 | ** | 0.0008 | 0.6753 | *** | -0.0003 | 0.0004 | *** | 5,139.27 | 4,265 | ||
| New Zealand | ΔNZ50d | 0.0519 | *** | 0.0264 | -0.0013 | 0.0000 | 0.2828 | *** | -0.0006 | ** | 0.0004 | *** | 1,246.25 | 2,801 | |||
| Singapore | ΔSTId | -0.0333 | ** | 0.0044 | -0.0373 | *** | -0.0063 | *** | 0.1815 | *** | -0.0001 | 0.0002 | * | 894.90 | 4,269 | ||
| South Korea | ΔKS11d | -0.0465 | *** | -0.0118 | -0.0453 | *** | -0.0112 | *** | 0.1914 | *** | 0.0003 | 0.0004 | ** | 843.35 | 4,180 | ||
| Switzerland | ΔSTOXX50Ed | -0.1285 | *** | -0.0448 | *** | -0.0425 | *** | -0.0103 | *** | 0.7325 | *** | -0.0003 | 0.0001 | 3,765.37 | 4,224 | ||
| Taiwan | ΔTWIId | 0.0020 | 0.0144 | -0.0434 | *** | -0.0074 | *** | 0.1639 | *** | -0.0002 | 0.0003 | * | 857.67 | 4,164 | |||
| United Kingdom | ΔFTSEd | -0.1329 | *** | -0.0530 | *** | -0.0357 | *** | -0.0084 | *** | 0.5435 | *** | -0.0003 | 0.0001 | 3,834.85 | 4,318 | ||
| Country | Index (y) | VIX (x) | Fy→x | Fx→y | ||
| Argentina | ΔMERVd | ΔVIXd | 1.6352 | 3.1867 | ||
| Australia | ΔAORDd | ΔVIXd | 3.2358 | 433.9916 | *** | |
| Belgium | ΔBFXd | ΔVIXd | 4.1014 | 241.9900 | *** | |
| Brazil | ΔBVSPd | ΔVIXd | 6.1516 | ** | 7.0556 | ** |
| Canada | ΔGSPTSEd | ΔVIXd | 27.0668 | *** | 34.9830 | *** |
| Chile | ΔIPSAd | ΔVIXd | 2.3663 | 17.4938 | *** | |
| France | ΔFCHId | ΔVIXd | 5.6285 | * | 348.7822 | *** |
| Germany | ΔGDAXId | ΔVIXd | 4.2677 | 264.5841 | *** | |
| Hong Kong | ΔHSId | ΔVIXd | 0.1643 | 580.2403 | *** | |
| India | ΔBSESNd | ΔVIXd | 6.0031 | ** | 165.6883 | *** |
| Indonesia | ΔJKSEd | ΔVIXd | 1.0822 | 235.3652 | *** | |
| Japan | ΔN225d | ΔVIXd | 5.9154 | * | 667.4963 | *** |
| Malaysia | ΔKLSEd | ΔVIXd | 0.2587 | 293.2344 | *** | |
| Mexico | ΔMXXd | ΔVIXd | 8.3291 | ** | 4.1649 | |
| New Zealand | ΔNZ50d | ΔVIXd | 0.7931 | 1.6962 | ||
| Singapore | ΔSTId | ΔVIXd | 1.8026 | 407.6022 | *** | |
| South Korea | ΔKS11d | ΔVIXd | 0.6222 | 386.3308 | *** | |
| Switzerland | ΔSTOXX50Ed | ΔVIXd | 10.4257 | *** | 312.5596 | *** |
| Taiwan | ΔTWIId | ΔVIXd | 3.3380 | 330.6689 | *** | |
| United Kingdom | ΔFTSEd | ΔVIXd | 9.1058 | ** | 411.4937 | *** |
| Trading rule (x) | Buy-hold strategy (y) | ||||||||||
| Country | Index | μ | σ | N | μ | σ | N | t | x−y<0 | x−y≠0 | x−y>0 |
| Argentina | MERV | 0.1465 | 0.1567 | 18 | 0.1822 | 0.3779 | 18 | -0.4047 | |||
| Australia | AORD | 0.2102 | 0.0948 | 18 | 0.0267 | 0.1908 | 18 | 4.6212 | *** | *** | |
| Belgium | BFX | 0.1870 | 0.0941 | 18 | 0.0136 | 0.2417 | 18 | 2.6013 | ** | *** | |
| Brazil | BVSP | 0.0862 | 0.1585 | 18 | 0.0829 | 0.2905 | 18 | 0.0673 | |||
| Canada | GSPTSE | 0.0961 | 0.0860 | 18 | 0.0280 | 0.1585 | 18 | 1.9022 | * | ** | |
| Chile | IPSA | 0.1295 | 0.0908 | 16 | 0.0887 | 0.1987 | 16 | 0.8932 | |||
| France | FCHI | 0.2241 | 0.1225 | 18 | -0.0054 | 0.1922 | 18 | 3.4418 | *** | *** | |
| Germany | GDAXI | 0.1865 | 0.1106 | 18 | 0.0260 | 0.2331 | 18 | 2.6856 | ** | *** | |
| Hong Kong | HSI | 0.4084 | 0.2095 | 18 | 0.0244 | 0.2381 | 18 | 5.0133 | *** | *** | |
| India | BSESN | 0.2387 | 0.1683 | 18 | 0.0920 | 0.3139 | 18 | 1.8288 | * | ** | |
| Indonesia | JKSE | 0.2613 | 0.2044 | 18 | 0.1107 | 0.3196 | 18 | 1.9475 | * | ** | |
| Japan | N225 | 0.4337 | 0.1985 | 18 | -0.0033 | 0.2304 | 18 | 5.5651 | *** | *** | |
| Malaysia | KLSE | 0.1768 | 0.1060 | 18 | 0.0436 | 0.1839 | 18 | 2.9748 | *** | *** | |
| Mexico | MXX | 0.1255 | 0.1370 | 18 | 0.1033 | 0.1916 | 18 | 0.5793 | |||
| New Zealand | NZ50 | 0.0226 | 0.0723 | 15 | 0.0585 | 0.1510 | 15 | -1.2657 | |||
| Singapore | STI | 0.2614 | 0.1461 | 18 | 0.0218 | 0.2374 | 18 | 3.9074 | *** | *** | |
| South Korea | KS11 | 0.3682 | 0.1865 | 18 | 0.0429 | 0.2805 | 18 | 3.9891 | *** | *** | |
| Switzerland | STOXX50E | 0.2082 | 0.1125 | 18 | -0.0163 | 0.1999 | 18 | 3.4946 | *** | *** | |
| Taiwan | TWII | 0.2725 | 0.1649 | 18 | 0.0027 | 0.2910 | 18 | 3.9516 | *** | *** | |
| United Kingdom | FTSE | 0.2011 | 0.1150 | 18 | 0.0029 | 0.1445 | 18 | 3.6737 | *** | *** | |