Citation: N. Akhtar, S. Hasan. Assessment of the interaction between three collinear unequal straight cracks with unified yield zones[J]. AIMS Materials Science, 2017, 4(2): 302-316. doi: 10.3934/matersci.2017.2.302
| [1] | Geoffrey E. Tupholme . Moving row of antiplane shear cracks within one-dimensional piezoelectric quasicrystals. AIMS Materials Science, 2016, 3(4): 1365-1381. doi: 10.3934/matersci.2016.4.1365 |
| [2] | R.R.Bhargava, Kamlesh Jangid, Pavitra Tripathi . A Mode-III strip saturation model for two collinear semi-permeable cracks in a piezoelectric media. AIMS Materials Science, 2016, 3(4): 1507-1519. doi: 10.3934/matersci.2016.4.1507 |
| [3] | Enrico Troiani . Analytical evaluation of the Stress Intensity Factor in stiffened sheets with multiple side damage. AIMS Materials Science, 2016, 3(4): 1615-1622. doi: 10.3934/matersci.2016.4.1615 |
| [4] | A.-V. Phan . Dynamic stress intensity factor analysis of the interaction between multiple impact-loaded cracks in infinite domains. AIMS Materials Science, 2016, 3(4): 1683-1695. doi: 10.3934/matersci.2016.4.1683 |
| [5] | R. Citarella, M. Lepore, M. Perrella, C. Calì . Multiple crack propagation by DBEM in a riveted lap-joint. AIMS Materials Science, 2016, 3(4): 1577-1586. doi: 10.3934/matersci.2016.4.1577 |
| [6] | Kisaburo Azuma, Yinsheng Li . Interaction factors for two elliptical embedded cracks with a wide range of aspect ratios. AIMS Materials Science, 2017, 4(2): 328-339. doi: 10.3934/matersci.2017.2.328 |
| [7] | Yanmei Zhang, Mu Fan, Zhongmin Xiao . Nonlinear elastic-plastic stress investigations on two interacting 3-D cracks in offshore pipelines subjected to different loadings. AIMS Materials Science, 2016, 3(4): 1321-1339. doi: 10.3934/matersci.2016.4.1321 |
| [8] | Ahmed M. Abood, Haider Khazal, Abdulkareem F. Hassan . On the determination of first-mode stress intensity factors and T-stress in a continuous functionally graded beam using digital image correlation method. AIMS Materials Science, 2022, 9(1): 56-70. doi: 10.3934/matersci.2022004 |
| [9] | Pierre Dulieu, Valéry Lacroix . Mutual interaction of stress concentration and stress intensity factor between semi-circular notch and crack. AIMS Materials Science, 2016, 3(4): 1520-1533. doi: 10.3934/matersci.2016.4.1520 |
| [10] | Tomoya Kawabata, Shuji Aihara, Yukito Hagihara . Coalescence judgment criteria for the interaction between two close surface cracks by WES2805 and its safety margin for brittle fracture assessment. AIMS Materials Science, 2016, 3(4): 1665-1682. doi: 10.3934/matersci.2016.4.1665 |
Nomenclature
| ±a1,±b1,c1,d1 | crack tips |
| ±a,±b | tips of the developed yield plastic zones |
| Di (i=0,1,2,3) | constants of the problem |
| E | Young's modulus |
| F(θ,k),E(θ,k),Π(θ,α2,k) | incomplete elliptic integral of first, second, third kind, respectively |
| Li (i=1,2,3) | cracks |
| Pn(z) | polynomial of degree n |
| p(t),q(t) | applied stresses on the yield zones |
| u,v | components of displacement |
| Xx,Yy,Xy | components of stress |
| z=x+iy | complex variable |
| γ | Poisson's ratio |
| μ | shear modulus |
| δ(x) | crack-tip-opening displacement at the crack tip x |
| Ω(z)=ω′(z),Φ(z)=ϕ′(z) | complex stress functions |
| Γ′ | −12(N1−N2)e−2iα,N1 and N2 are the values of principal stresses at infinity, α be the angle between N1 and the ox-axis |
| Γi (i=1,2,…,6) | developed plastic/yield zones |
| κ | =3−γ1+γ for the plane-stress, =3−4γ for the plane-strain |
| σ∞ | remotely applied stress at infinite boundary of the plate |
| σye | yield stress of the plate |
Sometimes man-made structures are catastrophic due to decreasing the residual strength of the material used for the development and propagation of cracks or crack like defects [1]. Human life sometimes is in danger since these structures break unconditionally. A number of aircraft and ships were failed at initial stage due to the presence of multiple interacting cracks in the manufactured structures. Therefore, it becomes imperative to study the load bearing capacity of the plate containing defects. Dugdale [2] proposed a classical single crack model to evaluate load carrying capacity of a plate. This model was used and modified by Kanninen [3] for linear stress distribution. Effect of variable stress distribution on the rims of developed yield zones studied by Harrop [4].
Cracks in the structure increase initially as non-interacting and isolated defects. As the length of cracks increases (in other words distance between cracks decreases) a destructive interaction between cracks increases rapidly causes the failure of the structures. So the available results of single crack are not appropriate for the modelling of multiple site damage problem [5]. Hence, Collins et al. [6] and Chang et al. [7] applied Dugdale model to obtain the solution of two equal straight cracks in an infinite plate, they also discussed the conditions of coalescence of yield zones between cracks. Dugdale strip yield model for three collinear straight cracks was studied by Hasan et al. [8] and further modified the model for linear stress distribution [9].
Many efforts, in fact, have been made for the case of separated yield zones as far as multiple cracks are the concern. However, some efforts have been made by various researchers [10,11] to investigate the load bearing capacity of the cracked plate when yield zones were coalesced due to increase in applied stresses. As discussed by Gdoutos [12] that some of the structure fail at a stress, which is below the yield stress of the plate. Therefore, assuming the variable stress distribution acting on the rims of yield zones in order to arrest the cracks from further opening. Few cases of linear stress distribution have been considered by Tang et al. [13], Hasan [14] and Tada et al. [15]. Therefore, the purpose of this work is to study the interaction of multiple cracks when yield zones developed between two closely located cracks coalesced under the application of linearly varying stress distribution.
Components of stresses (Xx,Yy,Xy) and displacements (u,v) are same as given by Muskhelishvili [16] in terms of two complex potential functions Φ(z),Ω(z) as
| Xx+Yy=2[Φ(z)+¯Φ(z)], | (1) |
| Yy−iXy=Φ(z)+¯Ω(z)−(z−¯z)Φ′(z), | (2) |
| 2μ(u+iv)=χϕ(z)−z¯ϕ′(z)−¯ψ(z). | (3) |
where bar over the function or variable denotes its complex conjugate and prime its first order derivative.
If the stresses X±y,Y±y are applied over the rims of the cracks, where superscript (+) & (−) denotes the values of stress components on the upper and lower rims of the cracks, the boundary value problem may be expressed as
| Y+y−iX+y=Φ+(t)+Ω−(t), | (4) |
| Y−y−iX−y=Φ−(t)+Ω+(t), | (5) |
under the assumption limy→0yΦ′(t+iy)=0.
General solution of the boundary value problems given in Eqs. (4) and (5) is obtained using Sokhotski Plemelj formula and written as
| Φ(z)+Ω(z)=1πiX(z)∫LX(t)p(t)dtt−z+2Pn(z)X(z), | (6) |
| Φ(z)−Ω(z)=1πi∫Lq(t)dtt−z−¯Γ′, | (7) |
where
| p(t)=12[Y+y+Y−y]−i2[X+y+X−y],q(t)=12[Y+y−Y−y]−i2[X+y−X−y],X(z)=∏nj=1√z−aj√z−bj,Pn(z)=D0zn+D1zn−1+D2zn−2+⋯+Dn, |
aj,bj are the end points of jth crack.
Unknown constants Di (i=0,1,2,…,n) of polynomial Pn(z) may be obtained using condition of single-valuedness of displacement around the rims of the cracks,
| 2(χ+1)∫LiPn(t)X(t)dt+χ∫Li[Φ+0(t)−Φ−0(t)]dt+∫Li[Ω+0(t)−Ω−0(t)]dt=0. | (8) |
The present analysis is a modification of Dugdale model under the influence of linear stress distribution. Consider a plate is to be infinitely extended and contains three unequal cracks L1,L2,L3 of real lengths (−a1,−b1), (b1,c1), (d1,a1) along the x-axis. Boundary of the plate is subjected to uniform stress distribution, Yy=σ∞ opens the rims of the cracks in mode-I type deformation. As a result, at each crack tip yield zone develop and grow on increasing stresses at the boundary of the plate. Therefore, yield zones developed at interior tips of two closely located cracks get coalesced. These zone are denoted by Γi (i=1,2,3,…,6) and occupy the intervals (−a,−a1), (−b1,−b), (b,b1), (c1,d1), (a1,a) respectively, on ox-axis.
Further damage in the plate is seized by applying a linear stress distribution Yy=taσye over the rims of developed yield zones. The entire configuration of this problem is given in Figure 1.
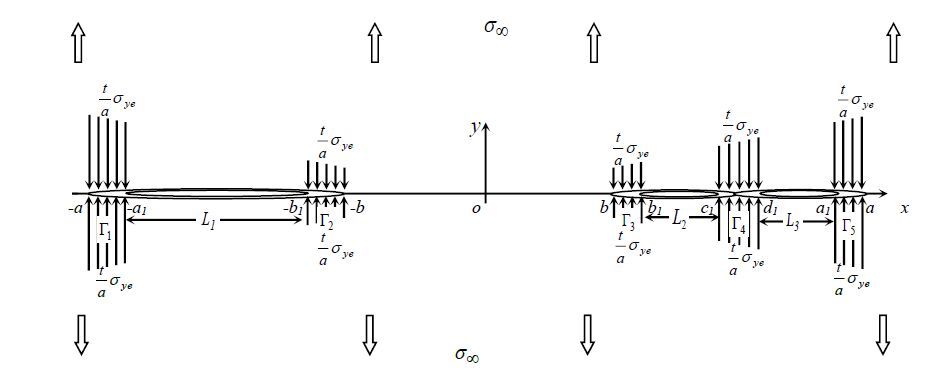 Figure 1. Configuration of the problem.
Figure 1. Configuration of the problem.The solution of the problem stated in Section 3 is obtained by superposition solution of two states of the problem. The first state is the tensile case when the infinite boundary is subjected to uniform stress distribution. The second state of the problem is to apply a linear stress distribution on the rims of the yield zones.
Consider, two cracks exist on the real axis in an infinite plate which is subjected to uniform tension, σ∞. The entire configuration is shown in Figure 2.
The problem of tensile case is solved under the following boundary conditions,
| Yy=σ∞,Xy=0,fory→±∞,−∞<x<∞ | (9) |
| Yy=Xy=0,fory=0,x∈2⋃i=1Ri | (10) |
The desired complex potential function ΦA(z) for this case is calculated using mathematical formulation given in Section 2 and boundary conditions (9) and (10) as,
| ΦA(z)=σ∞2[1X(z)(z2−a2λ2)−12] | (11) |
where λ2=E(k)F(k),k2=a2−b2a2 and F(k),E(k) are complete elliptical integrals of first and second kind, respectively, defined in Byrd [17].
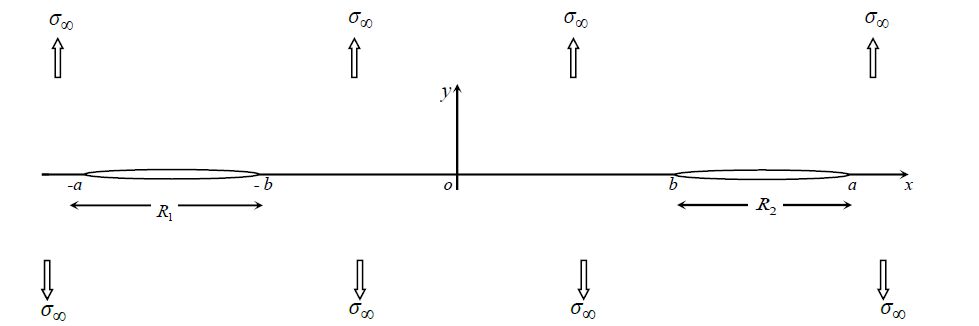 Figure 2. Configuration of the tensile case.
Figure 2. Configuration of the tensile case.Formula given here of calculating stress intensity factors for the configuration of Figure 2 at crack tip z=z1 is taken from [6],
| KI=2√2πlimz→z1√z−z1ΦA(z). | (12) |
Hence, opening mode stress intensity factors at crack tips a and b are determine by substituting ΦA(z) from Eq. (11) into Eq. (12) and after taking corresponding limits one can write
| (KAI)a=σ∞√πaa2√a2−b2(1−λ2), | (13) |
| (KAI)b=σ∞√πb1√a2−b2(b2−a2λ2), | (14) |
where subscript I refers to mode-I type deformation, and superscript A refers to sub-problem-A.
Displacement components for the tensile case is obtained on putting the value of ΦA(z) from Eq. (11) into Eq. (3) as,
| (v±A)a=±2aσ∞E[E(θ1,k)−λ2F(θ1,k)], | (15) |
| (v±A)b=∓2aσ∞E[E(θ2,k)−λ2F(θ2,k)−k2sinθ2cosθ2√1−k2sin2θ2], | (16) |
where θ1=sin−1√a2−a21a2−b2,θ2=sin−1√a2−b21a2−b2.
In this section, we aim to furnish a mathematical model for an isotropic infinite plate which is damaged by three collinear straight cracks with coalesced yield zones at the interior tip of two closely located cracks tips. These cracks are assumed to be located along ox-axis and denoted by Li (i=1,2,3). Cracks together with corresponding yield zones are treated as physical cracks of length (Γ1⋃L1⋃Γ2), and (Γ3⋃L2⋃Γ4⋃L3⋃Γ5). The model may be illustrated by means of Figure 3. Rims of developed yield zones are subjected to a compressive stress distribution to detain the cracks from further opening. According to Godoutos [12], in history, a number of failures occurred at a stress which is well below the yield strength of the plate. Therefore a linearly varying stress taσye (stress which is below the yield strength of the plate) distribution is considered over the rims of developed yield zones.
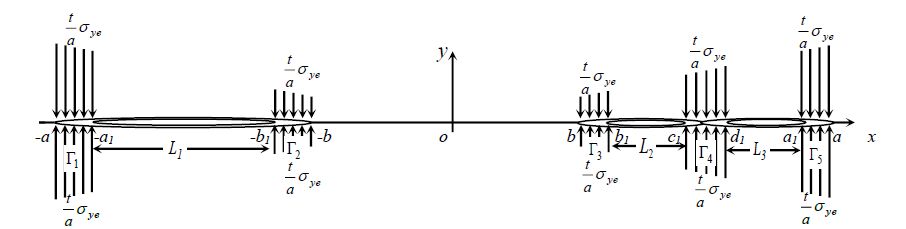 Figure 3. Configuration of the yield case.
Figure 3. Configuration of the yield case.The boundary conditions of the said problem are:
| Yy=0,Xy=0,fory→±∞,−∞<x<∞ | (17) |
| Yy=taσye,Xy=0,fory→0,x∈6⋃n=1Γn | (18) |
Using methodology given in Section 2 and boundary conditions (17) and (18), complex potential function for the sub-problem-B is obtained and written as:
| ΦB(z)=σye2πiX(z)[∫L′tX(t)dta(t−z)+iD2] | (19) |
where L′=[−a,−a1]⋃[−b1,−b]⋃[b,b1]⋃[c1,d1]⋃[a1,a], i.e., the loaded section in Figure 3. Using the fact that,
| X(t)=√t−a√t+a√t−b√t+b=i√a2−t2√t2−b2, | (20) |
| X(−t)=√−t−a√−t+a√−t−b√−t+b=−i√a2−t2√t2−b2. | (21) |
The integral shown in Eq. (19) may be evaluated as,
| ∫L′tX(t)dta(t−z)=2aa∫a1t2X(t)t2−z2dt+2ab1∫bt2X(t)t2−z2dt+1ad1∫c1(t2+tz)X(t)t2−z2dt,=2ia[a∫a1t2√a2−t2√t2−b2t2−z2dt+b1∫bt2√a2−t2√t2−b2t2−z2dt+12d1∫c1t2√a2−t2√t2−b2t2−z2dt+z2d1∫c1t√a2−t2√t2−b2t2−z2dt], |
Hence,
| ∫L′tX(t)dta(t−z)=2ia2k2[I1+(1−1n2)J1+za{I2+(1−1n2)J2}], | (22) |
where
| I1=i1(θ1)−i1(θ2)+i1(π2)+12(i1(θ3)−i1(θ4)),J1=j1(θ1)−j1(θ2)+j1(π2)+12(j1(θ3)−j1(θ4)),I2=12(i2(θ3)−i2(θ4)),J2=12(j2(θ3)−j2(θ4)),n2=a2−b2a2−z2,θ3=sin−1√a2−c21a2−b2,θ4=sin−1√a2−d21a2−b2,i1(ϕ)=13k2[(1−k2)F(ϕ,k)+(2k2−1)E(ϕ,k)−k2sinϕcosϕ√1−k2sin2ϕ],j1(ϕ)=[k2n2F(ϕ,k)−E(ϕ,k)+n2−k2n2Π(ϕ,n,k)],i2(ϕ)=12(ϕ−sinϕcosϕ),j2(ϕ)=[tanh−1(√n2−1tanϕ)√n2−1−ϕ]. |
Constant D2 is then obtained by using condition of single-valuedness of displacement around the rims of cracks given and written as ,
| D2=−2a2k2(T1−λ2T2), | (23) |
where
| T1=t1(θ1)−t1(θ2)+t1(π2)+12t1(θ3)−12t1(θ4),T2=t2(θ1)−t2(θ2)+t2(π2)+12t2(θ3)−12t2(θ4),t1(ϕ)=sinϕcosϕ√1−k2sin2ϕ6−1−k26k2F(ϕ,k)+(2−k23k2−1−k2sin2ϕ2k2)E(ϕ,k)t2(ϕ)=E(ϕ,k)2k2−1−k2sin2ϕ2k2F(ϕ,k). |
Thus, after a long mathematical calculations, final expressions for complex potential function ΦB(z) of closing case is obtained on substituting Eqs. (22) and (23) into Eq. (19),
| ΦB(z)=a2k2σyeπX(z)[I1+(1−1n2)J1+za(I2+(1−1n2)J2)−(T1−λ2T2)], | (24) |
Stress intensity factors at crack tips a, b for linear stress distribution is obtained by putting the value of ΦB(z) from Eq. (24) into Eq. (12), therefore
| (KBI)a=2a2k2σye√aπ√a2−b2(I1−E1+I2−ϕ1−(T1−λ2T2)), | (25) |
| (KBI)b=2a2k2σye√bπ√a2−b2(I1+baI2−(T1−λ2T2)). | (26) |
where
| E1=E(θ1,k)−E(θ2,k)+E(π2,k)+12E(θ3,k)−12E(θ4,k),ϕ1=12θ3−12θ4 |
Components of displacement are obtained on putting the value of ΦB(z) in Eq. (3),
| (v±B)a1=±4aπE(A1+A2), | (27) |
| (v±B)b1=∓4aπE(B1+B2), | (28) |
where
| A1={I1−(T1−λ2T2)}F(θ1,k)k2−F12{(1−k2)F(θ1,k)+k2S(θ1)}+I2k2θ1−ϕ1k22{sin2θ12+θ1}−E12{E(θ1,k)−2(1−k2)F(θ1,k)},A2=a31√a21−b22a3√a2−a21{Π(θ2,α2(a1),k)−Π(π2,α2(a1),k)−12Π(θ3,α2(a1),k)+12Π(θ4,α2(a1),k)}+12{12S(θ3)Λ1(c1)−S(θ2)Λ1(b1)−12S(θ4)Λ1(d1)}+12a2{Λ2(c1)−Λ2(d1)},B1={I1−(T1−λ2T2)}F(θ5,k)k2+F12{N(θ5)−(1−k2)F(θ5,k)}−k2ϕ12{π2−sin2θ22−θ2}−E12{M(θ5)−2(1−k2)F(θ5,k)}+k2I2{π2−θ2},B2=b31√b21−b22a3√a2−b21{Π(θ1,α2(b1),k)+Π(π2,α2(b1),k)+12Π(θ3,α2(b1),k)−12Π(θ4,α2(b1),k)}+b2k22{S(θ1)Λ3(a1)+12S(θ3)Λ3(c1)−12S(θ4)Λ3(d1)}+12a2{Λ4(c1)−Λ4(d1)},F1=F(θ1,k)−F(θ2,k)+F(π2,k)+12F(θ3,k)−12F(θ4,k),α21(t)=t2(a2−b2)a2(t2−b2),θ5=sin−1√a2(b21−b2)b21(a2−b2),S(θ)=sinθcosθ√1−k2sin2θ,α2(t)=a2−b2a2−t2,M(θ)=E(θ,k)−k2sinθcosθ√1−k2sin2θ,N(θ)=k2(1−k2)(1−k2sin2θ)32,Λ1(t)=(α2(t)−k2)π(θ1,α2(t),k)+k2F(θ1,k),Λ2(t)=−ξ(t,a1)−iX(t)2k2(k2θ1+ζ(t,θ1)),Λ3(t)=1t2−b2Π(θ5,α21(t),k),Λ4(t)=ξ(t,b1)−iX(t)2k2(k2(Π2−θ2)−ζ(t,θ2)),ξ(β,γ)=γ22tanh−1√(a2−β2)(γ2−b2)(a2−γ2)(β2−b2),ζ(β,γ)=(α2(β)−k2)tanh−1(√α2(β)−1tanγ)√α2(β)−1. |
The techniques outlined have been used to evaluate yield zone lengths and crack tip opening displacements for three cracks with coalesced yield zones under linearly varying stress distribution. Yield zone length at each crack tip is evaluated using Dugdale [2] hypothesis that the stresses remain finite in the vicinity of crack, hence
| K=KAI(z1)+KBI(z1)=0. | (29) |
On substituting the corresponding values of KAI from Eqs. (13) and (14) and the values of KBI from Eqs. (25) and (26) into Eq. (29), one may get two non-linear equations as given below.
| (σ∞σye)a(1−λ2)+2k2π[I1−E1+I2−ϕ1−(T1−λ2T2)]=0, | (30) |
| (σ∞σye)b(b2−a2λ2)+2a2k2π[I1+baI2−(T1−λ2T2)]=0. | (31) |
It is almost impossible to obtain yield zone length for prescribed values of σ∞σye, therefore σ∞σye is evaluated for given value of |b1−b|,|d1−c1| and |a−a1|. Figure 4 shows the variation between normalized load ratio σ∞σye and normalized yield zone length |a−a1||a1−b1| for different values of a1−b1a1+b1 (say △). It is clearly observed as the load applied at the boundary of the plate increases yield zone length |a−a1| increases as expected. The results of three collinear straight cracks with coalesced yield zones are compared with the results of two equal collinear cracks of the same length under same loading conditions, a significant difference is seen in yield zone length when cracks L1 and L2 are located far away from each other.
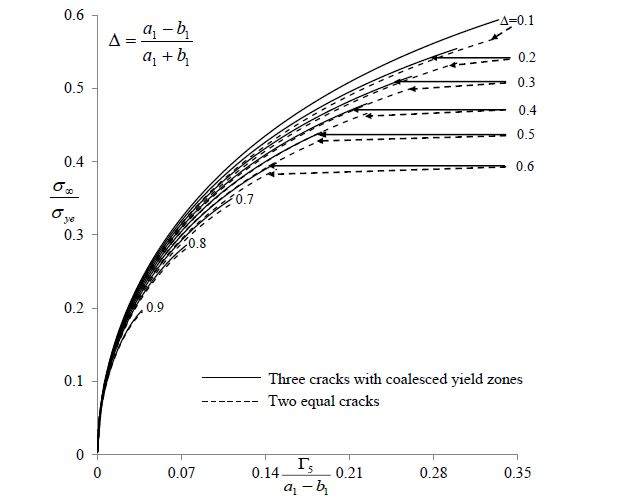 Figure 4. Variation between yield zone length and applied load ratio at the outer crack tip.
Figure 4. Variation between yield zone length and applied load ratio at the outer crack tip.Figure 5 shows the increase in the length of yield zone at crack tip b when applied stresses increases. It is seen from the graph, yield zone length |b1−b| is highly affected by the presence of another crack. The plate can bear more load when cracks are located far away (△=0.1) in comparison to closely located cracks (△=0.9). Furthermore, on comparing the results with two equal cracks it is clear that no significant difference is seen in bearing capacity for closely located cracks k=0.9.
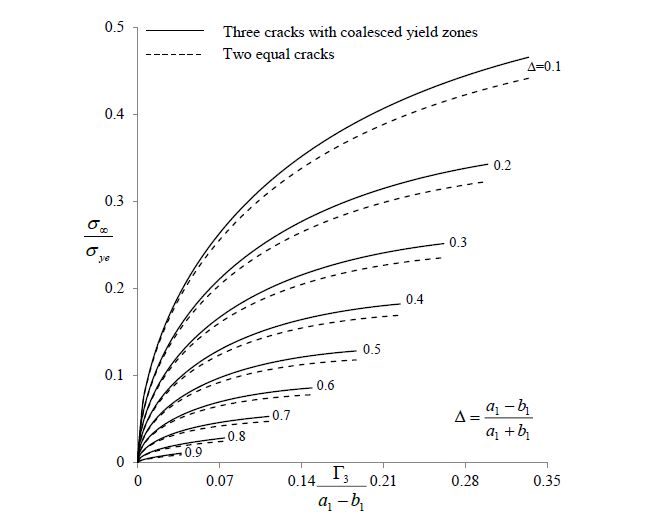 Figure 5. Variation between yield zone length and applied load ration at the inner crack tip.
Figure 5. Variation between yield zone length and applied load ration at the inner crack tip.Crack tip opening displacement (CTOD) is obtained using the formula given by Feng et al. [18],
| δ±(x)=v±A(x)−v±B(x), | (32) |
Hence, on substituting corresponding values of v±A and v±B from Eqs. (15) and (16) and Eqs. (27) and (28) into Eq. (32). One may get two non-linear equations for CTOD at crack tip a1 and b1, as
| δ±(a1)=±4aσyeE{(E(θ1,k)−λ2F(θ1,k))(σ∞σye)a−2π(A1+A2)}, | (33) |
| δ±(b1)=∓4aσyeE{(E(θ2,k)−λ2F(θ2,k)−k2sinθ2cosθ2√1−k2sin2θ2)(σ∞σye)b−2π(B1+B2)}. | (34) |
In order to investigated CTODs shown in Eqs. (33) and (34) at crack tips a1 and b1 ratio of CTODs to crack length has been plotted against applied load ratio. Figure 6 shows the said variation at crack tip a1 while Figure 7 at crack tip b1.
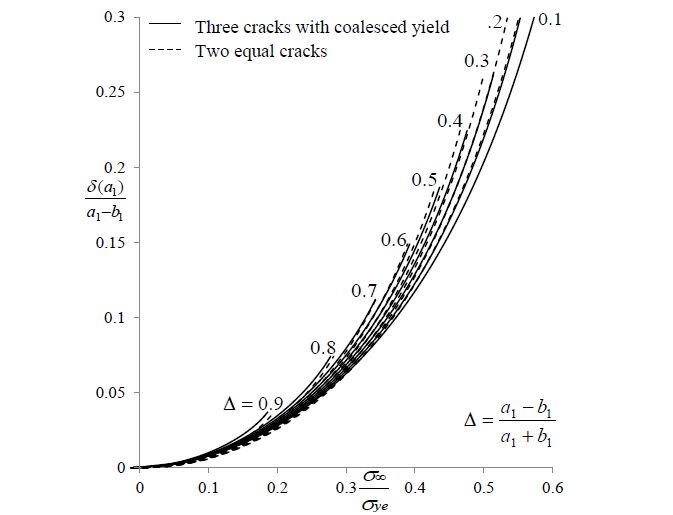 Figure 6. CTOD against applied load ratio at the crack tip a1.
Figure 6. CTOD against applied load ratio at the crack tip a1.As the load applied at the boundary of the plate increases cracks open in mode-I type deformation. In the case of △=0.9 the opening of cracks are almost same in comparison to the opening of two equal cracks. But the opening at crack tip a1 when △=0.1 is significantly different in the said comparison.
Figure 7 shows the behaviour of normalized CTOD with respect to applied load ratio σ∞σye at inner crack tip b1. CTOD at inner crack tip b1 is increasing gradually as the applied load at the boundary of the plate is increased. Moreover, a significant difference is seen in the CTOD of two configurations for different values of △.
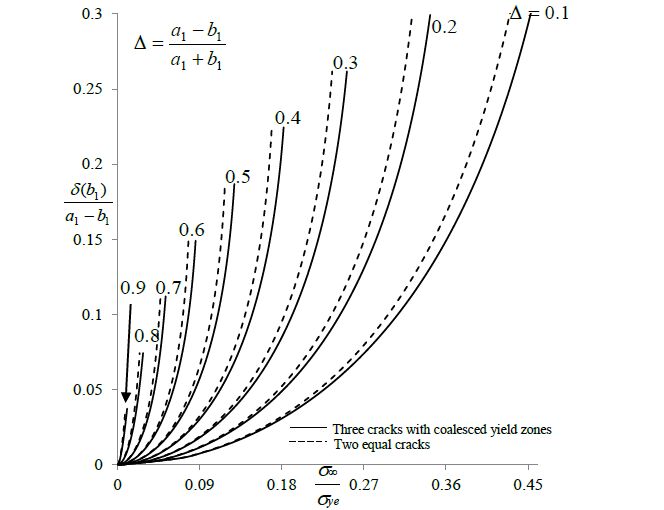 Figure 7. CTOD against applied load ratio at the crack tip b1.
Figure 7. CTOD against applied load ratio at the crack tip b1.A comparison of the results obtained is shown in Figure 8 and 9 with the results of single cracks given by Harrop [4]. It has been observed that as △=0.9, means cracks are located close to each other, behaviour of yield zone length and CTOD in case of configuration shown in Figure 1 is similar to single crack.
 Figure 8. Comparison of yield Zone length with single crack.
Figure 8. Comparison of yield Zone length with single crack.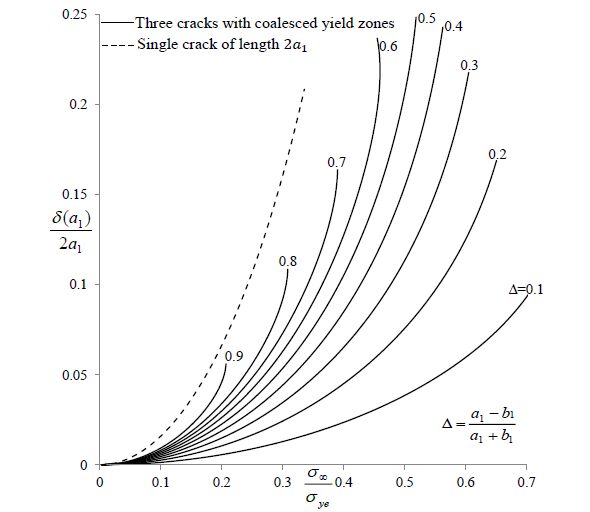 Figure 9. Comparison of CTOD with single crack.
Figure 9. Comparison of CTOD with single crack.1) Results of opening mode stress intensity factor for sub problem A given in Eqs. (13) and (14) are the same as given by Tada [15];
2) Expression of yield zone length given in Eq. (30) and crack-tip opening displacement in Eq. (33) are same as the expressions given by Harrop [4] taking b=b1=c1=d1=0 for a single crack.
The following set of conclusions may be drawn from the work that has been reported. The problem of three asymmetrical collinear straight cracks with coalesced yield zones in an infinite sheet is analyzed.
a) Analytical expression for stress intensity factors, yield zone length and CTODs have been obtained under linearly varying stress distribution using the complex variable method.
b) Numerical results for applied stresses, the length of yield zones and crack-tip opening displacements are plotted and reported graphically. These results are compared with the results of an equivalent configuration of two equal collinear straight cracks.
c) It has been observed that the plate can bear more load when the coalesced yield zone Γ5 bigger in size as compared to yield zone Γ3.
d) Less opening of cracks is seen in the presence of coalesced yield zones when results are compared with the results of two equal cracks.
First author is also grateful to University Grant Commission(UGC) for providing financial support under UGC-BSR scheme.
All authors declare no conflict of interest in this paper.
| [1] | Broek D (1982) Elementary Engineering Fracture Mechanics, The Netherlands: Martinus Nijhoff. |
| [2] | Dugdale DS (1960) Yielding of steel sheets containing slits. J Mech Phys Solids 8: 100–104. |
| [3] | Kanninen MF (1970) A solution for a Dugdale crack subjected to a linearly varying tensile loading. Int J Eng Sci 8: 85–95. |
| [4] | Harrop LP (1978) Application of a modified Dugdale model to the K vs COD relation. Eng Fract Mech 10: 807–816. |
| [5] | Chang D, Kotousov A (2012) A strip yield model for two collinear cracks in plates of arbitrary thickness. Int J Fracture 176: 39–47. |
| [6] | Collins RA, Cartwright DJ (2001) An analytical solution for two equal-length collinear strips yield cracks. Eng Fract Mech 68: 915–924. |
| [7] | Chang Dh, Kotousov A (2012) A strip yield model for two collinear cracks. Eng Fract Mech 90: 121–128. |
| [8] | Hasan S, Akhtar N (2015) Dugdale model for three equal collinear straight cracks: An analytical approach. Theor Appl Fract Mec 78: 40–50. |
| [9] | Hasan S, Akhtar N (2015) Mathematical model for three equal collinear straight cracks: A modified Dugdale approach. Strength Fract Complex 9: 211–232. |
| [10] | Nishimura T (1999) Strip Yield Analysis on Coalescence of Plastic Zones for Multiple Cracks in a Riveted Stiffened Sheet. ASME J Eng Mater Technol 121: 352–359. |
| [11] | Hasan S (2015) Dugdale model for three unequal collinear straight cracks with coalesced yield zones: a complex variable approach. Int J Pure Ap Mat 105: 311–323. |
| [12] | Gdoutos EE (2005) Fracture Mechanics–An Introduction, Springer. |
| [13] | Tang XS, Gao CH (2014) Macro–micro dual scale crack model linked by a restraining stress zone with a linear distribution. Theor Appl Fract Mec 71: 31–43. |
| [14] | Hasan S (2016) Modified Dugdale model for four collinear straight cracks with coalesced yield zones. Theor Appl Fract Mec 85: 227–235. |
| [15] | Tada H, Paris PC, Erwin GR (2000) The Stress Analysis of Cracks Handbook, New York: ASME Press. |
| [16] | Muskhelishvili NI (1963) Some Basic Problems of Mathematical Theory of Elasticity,Leiden: P. Noordhoff. |
| [17] | Byrd PF, Friedman MD (1971) Handbook of Elliptical Integrals for Engineers and Scientists, New York Heidelberg Berlin: Springer-Verlag. |
| [18] | Feng XQ, Gross D (2000) On the coalescence of collinear cracks in quasi-brittle materials. Eng Fract Mech 65: 511–524. |
| 1. | S. Shekhar, Naved Akhtar, S. Hasan, Study of load bearing capacity of an infinite sheet weakened by multiple collinear straight cracks with coalesced yield zones, 2021, 39, 2083-134X, 265, 10.2478/msp-2021-0023 | |
| 2. | N. Akhtar, S. Hasan, S. Shekhar, SOLUTION OF A MULTIPLE CRACK PROBLEM UNDER THE INFLUENCE OF A LINEAR STRESS DISTRIBUTION AND COALESCED YIELD ZONES, 2023, 64, 0021-8944, 118, 10.1134/S0021894423010133 | |
| 3. | Hongchuan Cheng, Xinhai Li, Guohui Ma, Xiafei Shi, Zhou Yang, Fatigue life and fatigue reliability mechanism of ball bearings, 2024, 40, 1811-8216, 79, 10.1093/jom/ufae006 | |
| 4. | Naved Akhtar, Shehzad Hasan, Sudhanshu Shekhar, Applications of modified Dugdale model to multiple collinear straight cracks with coalesced yield zones, 2024, 19, 1559-3959, 541, 10.2140/jomms.2024.19.541 |